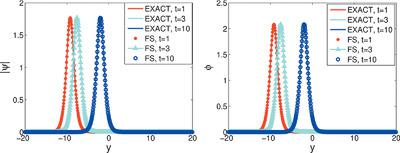
In this paper, we give an efficient numerical method for the nonlinear coupled space fractional Klein‐Gordon‐Schrödinger (NCSFKGS) equations, based on the Crank‐Nicolson method, the central difference method and the Fourier spectral method. As far as we know, no one has studied the Equations (5)–(6) in our paper, these equations are different from those in [39] which only considers space fractional Schrödinger equation while the Klein‐Gordon equation is classical, here, we consider the two equations which are both space fractional. In this paper, the Crank‐Nicolson method and the central difference method are used to discretize the space fractional Schrödinger equation and the space fractional Klein‐Gordon equation in time direction, respectively. The Fourier spectral method is used to discretize the NCSFKGS equations in space direction. This numerical method conserves the mass and energy in the discrete level. The convergence of the numerical method is of second order accuracy in time and spectral accuracy in space. Rigorous proofs are developed here for the conservation laws and the convergence of the numerical method. Numerical experiments are presented to confirm the theoretical results and they proved that our numerical method is very efficient (it only takes a few seconds to get the numerical solutions).

中文翻译：

---

非线性耦合空间分数阶Klein-Gordon-Schrödinger方程的傅立叶谱方法

在本文中，我们基于Crank-Nicolson方法，中心差分方法和傅立叶谱方法，为非线性耦合空间分数阶Klein-Gordon-Schrödinger（NCSFKGS）方程提供了一种有效的数值方法。据我们所知，没有人研究过方程式（5）-（6），这些方程式与[39]中的方程式不同，在文献[39]中仅考虑了空间分数薛定ding方程式，而克莱因-戈登方程式是经典的，这里，我们考虑两个都是空间分数的方程。本文使用Crank-Nicolson方法和中心差分法分别在时间方向上离散空间分数Schrödinger方程和空间分数Klein-Gordon方程。使用傅立叶谱方法在空间方向上离散化NCSFKGS方程。这种数值方法可以节省离散级的质量和能量。数值方法的收敛性是时间的二阶精度和空间的光谱精度。这里为守恒定律和数值方法的收敛性提供了严格的证明。数值实验证实了理论结果，并证明了我们的数值方法是非常有效的（仅需几秒钟即可得到数值解）。