Ferroelectric nonlinear anomalous Hall effect in few-layer WTe₂

Abstract

Under broken time reversal symmetry such as in the presence of external magnetic field or internal magnetization, a transverse voltage can be established in materials perpendicular to both longitudinal current and applied magnetic field, known as classical Hall effect. However, this symmetry constraint can be relaxed in the nonlinear regime, thereby enabling nonlinear anomalous Hall current in time-reversal invariant materials – an underexplored realm with exciting new opportunities beyond classical linear Hall effect. Here, using group theory and first-principles theory, we demonstrate a remarkable ferroelectric nonlinear anomalous Hall effect in time-reversal invariant few-layer WTe₂ where nonlinear anomalous Hall current switches in odd-layer WTe₂ except 1T′ monolayer while remaining invariant in even-layer WTe₂ upon ferroelectric transition. This even-odd oscillation of ferroelectric nonlinear anomalous Hall effect was found to originate from the absence and presence of Berry curvature dipole reversal and shift dipole reversal due to distinct ferroelectric transformation in even and odd-layer WTe₂. Our work not only treats Berry curvature dipole and shift dipole on an equal footing to account for intraband and interband contributions to nonlinear anomalous Hall effect, but also establishes Berry curvature dipole and shift dipole as new order parameters for noncentrosymmetric materials. The present findings suggest that ferroelectric metals and Weyl semimetals may offer unprecedented opportunities for the development of nonlinear quantum electronics.

Introduction

In classical linear Hall effect, a transverse voltage can be developed in materials with broken time-reversal symmetry only (e.g. in the presence of external magnetic field or internal magnetization) due to Onsager’s relation. Second and higher order conductivity tensors, however, are not subject to this constraint, thereby enabling nonlinear anomalous Hall effect (NAHE) in time-reversal invariant system.^1,2,3,4 NAHE was observed very recently in few-layer tungsten ditelluride (WTe₂),^{5,6,7,8,9,10,11} a layered material which also holds rich physics including high-temperature quantum spin Hall phase^12,13,14,15 and electrostatic gating induced superconductivity^16,17 in its 1T′ monolayer and type-II Weyl semimetallicity,¹⁸ large non-saturating magnetoresistance¹⁹ and ultrafast symmetry switching²⁰ in its bulk phase.

Monolayer 1T′ WTe₂ is centrosymmetric with vanishing even-order nonlinear current response, however vertical electric field can break its two-fold screw rotation symmetry, generate Berry curvature dipole (BCD), and induce second-order nonlinear anomalous Hall current.^5,6,7,8 In contrast to monolayer WTe₂, bilayer WTe₂ is naturally noncentrosymmetric due to the loss of two-fold screw rotation symmetry, resulting in intrinsic nontrivial BCD in bilayer WTe₂.^9,10,11 Surprisingly, ferroelectric switching was recently discovered in semimetallic bilayer and few-layer WTe₂,²¹ quite unusual as ferroelectricity and semimetallicity normally do not co-exist in the same material.²² The subtlety lies in the reduced screening along the out-of-plane direction which gives rise to finite out-of-plane ferroelectric polarization while preserving in-plane semimetallic nature. Conductance hysteresis persisting up to 300 K shows its great potential for room temperature device application. These recent studies combined reveal a striking feature of noncentrosymmetric few-layer WTe₂ – the coexistence of ferroelectricity and NAHE within a single material, enkindling a few fundamentally and technologically important questions: what’s the fundamental correspondence between NAHE and ferroelectricity in ferroelectric metals and Weyl semimetals? Compared to ferroelectric semiconductors,²³ what are the unique advantages of ferroelectric metals²¹ and ferroelectric Weyl semimetals?^24,25

Here using first-principles approach and group theoretical analysis we show an intriguing ferroelectric nonlinear anomalous Hall effect (FNAHE) in time-reversal invariant few-layer WTe₂. In particular, while both bilayer and trilayer WTe₂ possess switchable out-of-plane electric polarization, nonlinear transverse Hall current only switches in trilayer WTe₂ upon ferroelectric switching. The microscopic origin of FNAHE in trilayer WTe₂ is found to be rooted in the reversal of Berry curvature dipole and shift dipole upon ferroelectric transition, which reveals an exciting yet unexplored realm of ferroelectric metals and Weyl semimetals with potential applications in nonlinear electronics.

Results and discussion

Second-order dc current

Consider an oscillating electric field ${\boldsymbol{E}}\left( {{\boldsymbol{r}},t} \right) = {\boldsymbol{E}}\left( \omega \right)e^{i\left( {{\boldsymbol{k}} \cdot {\boldsymbol{r}} - \omega t} \right)} + {\boldsymbol{E}}\left( { - \omega } \right)e^{ - i\left( {{\boldsymbol{k}} \cdot {\boldsymbol{r}} - \omega t} \right)}$ with E(ω) = E^*(−ω) (e.g. under AC electric field or upon coherent light illumination), the second-order nonlinear dc current under minimal coupling approximation was derived by Sipe et al.²⁶, i.e., $j_a^0 = \chi _{abc}\left( {0;\omega , - \omega } \right)E_b\left( \omega \right)E_c( - \omega )$, where χ_abc are the dc photocurrent susceptibility. In general $j_a^0$ consists of two parts depending on the polarization of electric field/incident light, including linear photogalvanic effect (LPGE) and circular photogalvanic effect (CPGE),^2,26,27 i.e., $j_a^0 = j_a^L + j_a^C$. BCD-induced nonlinear photocurrent current was generalized to the multiple-band case by Morimoto et al.²⁸ using Floquet theory and Rostami et al.²⁹ using density matrix beyond semiclassical Boltzmann theory. Nonlinear photocurrent originating from CPGE is also known as injection current.²⁶

Both LPGE and CPGE have intraband and interband contributions. For the sake of completeness we include all the terms as follows,

$$j_{a}^{L} = j_{a,{\mathrm{intra}}}^{L} + j_{a,{\mathrm{inter}}}^{L}\left\{ {\begin{array}{*{20}{l}} {j_{a,{\mathrm{intra}}}^{L} = - 2\frac{{e^3}}{{\hbar ^2}}{\mathrm{Re}} \left( \frac{\tau }{{1 - i\omega \tau}} \right){\it{\epsilon }}_{adc}D_{bd}^{\mathrm{intra}}{\rm{Re}} \left( {E_{b}( \omega)E_{c}(- {\omega})} \right)}\\{j_{a,{\mathrm{inter}}}^{L} = - 2\frac{e^3}{\hbar ^2}\tau D_{a,bc}^{{\mathrm{L}},{\mathrm{inter}}}{\rm{Re}} \left( {E_{b}( \omega)E_{c}( -{\omega})} \right)} \end{array}} \right.$$

(1)

$$j_a^C = j_{a,{\mathrm{intra}}}^C + j_{a,{\mathrm{inter}}}^C\left\{ {\begin{array}{*{20}{l}} {j_{a,{\mathrm{intra}}}^C = - \frac{{e^3}}{{\hbar ^2}}{\rm{Im}} \left( {\frac{\tau }{{1 - i\omega \tau }}} \right)D_{ab}^{{\mathrm{intra}}}{\rm{Im}} \left( {{\boldsymbol{E}}\left( \omega \right) \times {\boldsymbol{E}}\left( { - \omega } \right)} \right)_b} \hfill \\ {j_{a,{\mathrm{inter}}}^C = - \frac{{e^3}}{{2\hbar ^2}}\tau D_{ab}^{{\mathrm{C}},{\mathrm{inter}}}{\rm{Im}} \left( {{\boldsymbol{E}}\left( \omega \right) \times {\boldsymbol{E}}\left( { - \omega } \right)} \right)_b} \hfill \end{array}} \right.$$

(2)

Here τ is relaxation time and ${\it{\epsilon }}_{adc}$ is the Levi–Civita symbol. $D_{ab}^{{\mathrm{intra}}}$ is the well-known BCD for intraband nonlinear process.² $D_{ab}^{{\mathrm{C}},{\mathrm{inter}}}$ is BCD for interband process associated with CPGE.⁹ $D_{a,bc}^{{\mathrm{L}},{\mathrm{inter}}}$ is shift dipole (SD), originated from the simultaneous displacement of wavepacket upon excitation. More specifically, they are given by

$$\left\{ {\begin{array}{*{20}{l}} {D_{ab}^{{\mathrm{intra}}}\left( \mu \right) = {\int}_{BZ} {f_0} \left( \mu \right)\partial _a{\mathrm{\Omega }}^b = {\int_{BZ}} \left[ {d{\boldsymbol{k}}} \right]\mathop {\sum}\nolimits_n {f_n} \left( \mu \right)v_n^a\left( {\boldsymbol{k}} \right){\mathrm{\Omega }}_n^b\left( {\boldsymbol{k}} \right)\delta (\hbar \omega _n({\boldsymbol{k}}) - \mu )} \hfill \\ {D_{ab}^{{\mathrm{C}},{\mathrm{inter}}}\left( {\mu ,\omega } \right) = {\int}_{BZ} {[d{\boldsymbol{k}}]} \mathop {\sum}\nolimits_{mn} {f_{nm}} \left( \mu \right){\mathrm{\Delta }}_{mn}^a\left( {\boldsymbol{k}} \right){\mathrm{\Omega }}_{nm}^b\left( {\boldsymbol{k}} \right){\rm{Re}} \left( {\frac{\tau }{{1 - i\left( {\omega - \omega _{mn}} \right)\tau }}} \right)} \hfill \\ {D_{a,bc}^{{\mathrm{L}},{\mathrm{inter}}}\left( {\mu ,\omega } \right) = {\int}_{{\mathrm{BZ}}} {[d{\boldsymbol{k}}]} \mathop {\sum}\nolimits_{mn} {f_{nm}} \left( \mu \right)R_{mn}^a\left( {\boldsymbol{k}} \right)\left\{ {r_{nm}^b,r_{mn}^c} \right\}{\rm{Re}} \left( {\frac{1}{{1 - i\left( {\omega - \omega _{mn}} \right)\tau }}} \right)} \hfill \end{array}} \right.$$

(3)

Here, $\hbar \omega _n({\boldsymbol{k}})$, $v_n^b\left( {\boldsymbol{k}} \right)$, and f_n(μ) are band energy, group velocity, and chemical-potential μ dependent Fermi-Dirac distribution, respectively. f_nm(μ) ≡ f_n(μ) − f_m(μ), and [dk] ≡ d^dk/(2π)^d for d-dimension integral. ${\mathrm{\Delta }}_{nm}^a \equiv v_n^a - v_m^a$ is the group velocity difference between two bands. $r_{nm}^a$ is interband Berry connection or dipole matrix element. ${\mathrm{\Omega }}_{nm}^c\left( {\boldsymbol{k}} \right)$ is the interband Berry curvature between two bands, defined as ${\mathrm{\Omega }}_{nm}^c\left( {\boldsymbol{k}} \right) \equiv i{\it{\epsilon }}_{abc}r_{nm}^ar_{mn}^b.$ ${\mathrm{\Omega }}_n^c\left( {\boldsymbol{k}} \right)$ is the intraband Berry curvature for band n, given by ${\mathrm{\Omega }}_n^c\left( {\boldsymbol{k}} \right) = \mathop {\sum}\nolimits_{n \ne m} {{\mathrm{\Omega }}_{mn}^c} \left( {\boldsymbol{k}} \right)$. In addition, $\left\{ {r_{nm}^b,r_{mn}^c} \right\} \equiv r_{nm}^br_{mn}^c + r_{mn}^cr_{nm}^b$. $R_{mn}^a$ is shift vector, given by $R_{mn}^a \equiv - \frac{{\partial \phi _{mn}\left( {\mathbf{k}} \right)}}{{\partial k^a}} + r_{mm}^a\left( {\boldsymbol{k}} \right) - r_{nn}^a\left( {\boldsymbol{k}} \right)$, where ϕ_mn(k) is the phase factor of the interband Berry connection and $r_{nn}^a$ is intraband Berry connection. ${\mathrm{\Omega }}_{nm}^b\left( {\boldsymbol{k}} \right)$, ${\mathrm{\Omega }}_n^b\left( {\boldsymbol{k}} \right)$ and $R_{mn}^a$ are all gauge invariant. For linearly polarized incident light/electric field, E_b = E_c, hence we denote $D_{ab}^{{\mathrm{L}},{\mathrm{inter}}} \equiv D_{a,bc}^{{\mathrm{L}},{\mathrm{inter}}}$. The intraband and interband BCDs ($D_{ab}^{{\mathrm{intra}}}$, $D_{ab}^{{\mathrm{C}},{\mathrm{inter}}}$) as well as SD $(D_{ab}^{{\mathrm{L}},{\mathrm{inter}}})$ have the same units of L^3−d for d-dimensional system. Thus, BCD and SD have units of length in 2D, but become dimensionless in 3D.

The appearance of relaxation time τ in the dc current from interband LPGE $(j_{a,{\mathrm{inter}}}^L)$ seems different from the widely-used τ-independent shift current formula by Sipe et al.²⁶, however the latter was derived for the clean limit when relaxation time τ approaches to infinite. In fact, as τ → ∞, $\tau {\mathrm{Re}}\left( {\frac{1}{{1 - i\left( {\omega - \omega _{mn}} \right)\tau }}} \right) \to \pi \delta \left( {\omega _{mn} - \omega } \right)$, and the original τ-time independent shift current susceptibility can be exactly recovered from the above $j_{a,{\mathrm{inter}}}^L$ formula. In reality, quasiparticles do have finite relaxation time, thus $j_{a,{\mathrm{inter}}}^L$ shall depend on relaxation time. More detailed derivation about the SD and BCD can be found in the Supplementary Information.

Moreover, it is worth to classify the contributions of LPGE/CPGE-induced dc current at the low/high frequency region. At the low frequency limit, ωτ → 0, hence $\frac{\tau }{{1 - i\omega \tau }} \to \tau$. In this case, the photocurrent due to both intraband and interband CPGE as well as interband LPGE will vanish, however a dc current from intraband LPGE will remain finite which is perpendicular to the applied electric field, thereby inducing static NAHE. At high frequency, CPGE (i.e. injection current) and interband LPGE (i.e. shift current) will have nontrivial contribution to total nonlinear photocurrent, referred as to dynamic NAHE.

It is important to note that the direction of nonlinear photocurrent induced by CPGE and LPGE have very different symmetry properties. The LPGE-induced dc current cannot flow normal to a mirror plane, however it is allowed for the CPGE-induced dc current. This distinct symmetric property of CPGE- and LPGE-induced dc current can be used to help distinguish different contributions. In fact, this is what we will see in bilayer and trilayer WTe₂.

More importantly, nonlinear dc current may switch their direction upon certain ferroelectric transition, giving rise to FNAHE which is the focus of this work. Below we will first reveal the fundamental difference between ferroelectric transitions in bilayer and trilayer WTe₂, then demonstrate a striking even-odd anomaly of NAHE, i.e. FNAHE, in bilayer and trilayer WTe₂ and provide an explanation using group theoretical analysis as well as its implication for potential FNAHE-based quantum devices.

Ferroelectric transition in bilayer and trilayer WTe₂

Both bilayer and trilayer WTe₂ were found to exhibit ferroelectric switching, however their transformation is fundamentally different, which plays a key role in their distinct NAHE. Crystal structures of monolayer, bilayer, and trilayer WTe₂ are shown in Fig. 1. Monolayer 1T′ WTe₂ has a C_2h point group with a mirror plane symmetry ${\cal{M}}_y$ perpendicular to y-axis and a two-fold screw rotation symmetry C_2y. This leads to inversion symmetry ${\cal{I}} = {\cal{M}}_yC_{2y}$ or $C_{2y}{\cal{M}}_y$. Upon van der Waals (vdW) T_d stacking, multilayer noncentrosymmetric T_d WTe₂ possesses mirror plane symmetry ${\cal{M}}_y$ only, and no longer holds C_2y symmetry as the rotation axes of different layers are not related by any symmetry operation in the point group. Consequently, multilayer T_d WTe₂ loses inversion center with C_s point group.

Fig. 1: Crystal structure of monolayer, bilayer, and trilayer WTe₂.

a Monolayer 1T′ WTe₂ with centrosymmetric C_2h point group. It has a mirror plane ${\cal{M}}_y$ and a screw rotation symmetry C_2y, which leads to the inversion symmetry ${\cal{I}} = {\cal{M}}_yC_{2y}$. b, c Bilayer and trilayer T_d WTe₂ with C_s point group. C_2y symmetry is broken, hence the inversion symmetry ${\cal{I}}$ is also broken in bilayer and trilayer WTe₂.

Ferroelectric transition pathways of bilayer and trilayer T_d WTe₂ are shown in Fig. 2. In both cases, two opposite ferroelectric (FE) states (Fig. 2a, c for bilayer, Fig. 2d, f for trilayer) can switch to each other by a small in-plane shift between adjacent layers along x by 2d_x (d_x ~ 20pm), passing through an intermediate paraelectric (PE) state. The intermediate PE state in bilayer WTe₂ (Fig. 2b) has a C_2v point group with additional $\left\{ {{\cal{M}}_{z}{{|}}\frac{1}{2}a} \right\}$ symmetry, thus its out-of-plane electric polarization P_z vanishes. While the ferroelectric transition is achieved by in-plane 2d_x shift, two FE states are related by a glide plane operation $\left\{ {{\cal{M}}_z{{|}}t_a} \right\}$ consisting of a mirror symmetry operation followed by a translation along x by a fractional translation t_a where $t_{a} = \frac{1}{2}a$. For this reason, we denote the two FE states of bilayer WTe₂ by −mFE and +mFE (Fig. 2a, c). In contrast, the two opposite FE states in trilayer WTe₂ are related by an inversion operation ${\cal{I}}$, denoted by −iFE and +iFE (Fig. 2d, f). Furthermore, its intermediate PE state has a C_2h point group with inversion symmetry, hence the out-of-plane polarization P_z of the PE state in trilayer WTe₂ vanishes as well.

Fig. 2: Ferroelectric transition in bilayer and trilayer WTe₂.

a–c ferroelectric transition in bilayer WTe₂. The two opposite ferroelectric states (−mFE and +mFE) in bilayer are transformed through a glide plane operation $\left\{ {{\cal{M}}_z{\mathrm{|}}t_a} \right\}$, that is, a mirror operation ${\cal{M}}_z$ followed by an in-plane shift along x by t_a with $t_a = \frac{1}{2}a$. The intermediate PE state C_2v point group, thus its out-of-plane polarization with vanishes due to the glide plane $\left\{ {{\cal{M}}_z|\frac{1}{2}a} \right\}$ symmetry. d–f ferroelectric transition in trilayer WTe₂. The two opposite ferroelectric states (−iFE and +iFE) in trilayer are related to each other through an inversion operation $\left\{ {{\cal{I}}{\mathrm{|}}0} \right\}$. The intermediate PE state of trilayer WTe₂ has C_2h point group, thus its out-of-plane polarization also vanishes due to inversion symmetry. The red and green vertical dashed lines show the relative shift ±d_x between adjacent WTe₂ layers. The corresponding in-plane shift is very small (d_x ≈ 20 pm), therefore it is exaggerated in the above plots for illustrative purpose.

Next, we calculate total electric polarization by summing the ionic and electronic contributions. Since we are interested in the polarization along the out of plane direction P_z, we can directly integrate the product between charge density/ionic charge and their corresponding position to obtain P_z without using Berry phase approach. More specifically, $P_{z} = \frac{1}{S}( {{\sum\nolimits_I} Q_{I} \cdot \left( {z_{I} - {\boldsymbol{R}}_{0}^z} \right) - e{\int_V} \rho \left( {\boldsymbol{r}} \right)\left( {z - {\boldsymbol{R}}_0^z} \right)d^3r})$, where S is the in-plane area of the unit cell, Q is ionic charge, ρ is electronic charge density, and R₀ is a reference point which is set to the origin of the unit cell in the present case. The equilibrium electronic charge density ρ(r) was obtained from first-principles density functional theory (DFT)^30,31 as implemented in the Vienna Ab initio Simulation Package (VASP).³² The calculated total electric polarization P_z is ±1.67 × 10⁻² nm μC/cm² for ±mFE in bilayer, and ±0.81 × 10⁻² nm μC/cm² for ±iFE in trilayer. This is in good agreement with experimentally measured vertical polarization in bilayer WTe₂ of ~10⁴ e cm⁻¹ (i.e. 1.60 × 10⁻² nm μC/cm²).²¹ Additionally, the intermediate PE state was recently observed in experiments.²⁰ In brief, the results from the DFT calculations confirmed the ferroelectricity in both bilayer and trilayer WTe₂, however the symmetry relations between the two FE states are very different in the bilayer and trilayer cases, i.e. −mFE ↔ PE ↔ +mFE and −iFE ↔ PE ↔ +iFE, which is essential for understanding their distinct NAHE upon ferroelectric switching we will discuss shortly.

NAHE in bilayer and trilayer WTe₂ upon ferroelectric switching

Now we proceed to discuss NAHE in few-layer WTe₂, in particular ferroelectric switching of NAHE (i.e. FNAHE) in odd-layer WTe₂, and reveal the intriguing connection between BCD/SD and ferroelectric order. We compute their electronic structure by first-principles DFT using hybrid exchange-correlation functional with spin-orbit coupling taken into account. Quasiatomic spinor Wannier functions and tight-binding Hamiltonian were obtained by rotating and optimizing the Bloch functions with a maximal similarity measure with respect to pseudoatomic orbitals.^33,34 Subsequently, first-principles tight-binding approach was applied to compute all the physical quantities such as band structure, BCD, SD, Berry curvature etc. More calculation details can also be found in Methods Section.

Electronic band structure of bilayer WTe₂ is presented in Fig. 3a, color-coded by total intraband Berry curvature of all occupied bands, that is, ${\mathrm{\Omega }}_{{\mathrm{occ}}}^{z,{\mathrm{intra}}}\left( {\boldsymbol{k}} \right) = \mathop {\sum }\limits_n f_n{\mathrm{\Omega }}_n^z\left( {\boldsymbol{k}} \right)$. It shows bilayer WTe₂ is a small gap insulator, and the intraband Berry curvature is odd with respect to Γ due to the presence of time-reversal symmetry. The k-dependent intraband Berry curvature ${\mathrm{\Omega }}_{{\mathrm{occ}}}^{z,{\mathrm{intra}}}\left( {\boldsymbol{k}} \right)$ are shown in Fig. 3c, d at two different chemical potentials of μ = ±50 meV. Alternatively, one may use the Kubo formula with the sum-over-states approach for Berry curvature (see Supplementary Fig. 2). Similarly, interband Berry curvature ${\mathrm{\Omega }}_{nm}^z\left( {\boldsymbol{k}} \right)$ with at frequency ω = 120 meV is displayed in Fig. 3e, f for two sets of occupied and unoccupied bands around the Fermi energy, ${\mathrm{\Omega }}_{{\mathrm{VBM}} - 1,{\mathrm{CBM}}}^{z,{\mathrm{inter}}}\left( {\boldsymbol{k}} \right)$ and ${\mathrm{\Omega }}_{{\mathrm{VBM}},{\mathrm{CBM}} - 1}^{z,{\mathrm{inter}}}\left( {\boldsymbol{k}} \right)$, respectively. VBM refers to valence band maximum, and CBM refers to conduction band minimum. The Berry curvature distribution plots confirm the presence of mirror symmetry ${\cal{M}}_{y}$ and time-reversal symmetry ${\cal{T}}$. Thus, the integral of the intraband Berry curvature over the full Brillouin zone vanishes, and linear anomalous Hall effect is absent. Furthermore, Fig. 3b shows the calculated BCD and SD tensor elements – $D_{yz}^{{\mathrm{intra}}}$, $D_{yz}^{{\mathrm{C}},{\mathrm{inter}}}$, and $D_{xy}^{{\mathrm{L}},{\mathrm{inter}}}$ – the key physical quantities governing NAHE. It clearly demonstrates the presence of finite BCD and SD and thus NAHE in bilayer WTe₂. The calculated BCD varies between 0 and 0.4 Å depending on the chemical potential, which is in nice agreement with the experimental values of 0.1–0.7 Å by Kang et al.¹⁰. Moreover, upon ferroelectric transition between −mFE and +mFE state, the Berry curvature, BCD and SD remain unchanged, thus nonlinear anomalous Hall current will not switch direction upon ferroelectric transition in bilayer WTe₂. Similarly, the out-of-plane spin polarization remains unflipped, while the in-plane spin polarization is expected to reverse (see Supplementary Figs. 3 and 4). Furthermore, Du et al. recently studied NAHE in bilayer WTe₂ using a model Hamiltonian and found that, as the SOC strength evolves, BCD becomes strong near tilted band anticrossings and band inversions.¹¹ Our first-principles results also show large Berry curvature near band anticrossings which is consistent with the conclusion from Du et al.’s analysis. The magnitude of the calculated Berry curvature is similar to that in Ma et al.⁹ for bilayer WTe₂ in the absence of electric field. The difference in the detailed band structure is mainly due to the electronic structure sensitive to DFT exchange-correlation functional, vdW functional, and the Wannier function construction. Nevertheless, both our results and the work by Ma et al.⁹ show the nontrivial BCD contribution to NAHE.

Fig. 3: Electronic structure and NAHE of bilayer WTe₂.

a Band structure of bilayer WTe₂ in ±mFE state color-coded by the z-component of intraband Berry curvature ${\mathrm{\Omega }}_n^z\left( {\boldsymbol{k}} \right)$. Spin-orbit coupling is included and hybrid HSE06 functional is employed. b BCD and SD tensor elements $D_{yz}^{{\mathrm{intra}}}\left( \mu \right)$, $D_{yz}^{{\mathrm{C}},{\mathrm{inter}}}\left( {\mu ,\omega } \right)$, and $D_{xy}^{{\mathrm{L}},{\mathrm{inter}}}\left( {\mu ,\omega } \right)$ as function of chemical potential μ. For interband BCD and SD, ω is set to 120 meV. c, d k-dependent distribution of intraband Berry curvature ${\mathrm{\Omega }}_{{\mathrm{occ}}}^{z,{\mathrm{intra}}}\left( {\boldsymbol{k}} \right)$ at μ = ±50 meV, respectively. e, f k-dependent distribution interband Berry curvature ${\mathrm{\Omega }}_{nm}^{z,{\mathrm{inter}}}\left( {\boldsymbol{k}} \right)$ for (n, m) = (VBM-1, CBM) and for (n, m) = (VBM-1, CBM), respectively. The results are the same for both +mFE and −mFE state, suggesting that nonlinear anomalous Hall current can be induced in bilayer WTe₂, but it will not switch sign upon ferroelectric transition.

Trilayer WTe₂ is quite different from bilayer WTe₂. Figure 4a, b show its electronic band structure of −iFE and +iFE state, respectively. In contrast to the bilayer case, intraband Berry curvature changes sign upon ferroelectric transition. The similar sign change is also evidenced in the opposite k-dependent intraband and interband Berry curvature ${\mathrm{\Omega }}_{{\mathrm{occ}}}^{z,{\mathrm{intra}}}\left( {\boldsymbol{k}} \right)$ and ${\mathrm{\Omega }}_{{\mathrm{VBM}} - 1,{\mathrm{CBM}}}^{z,{\mathrm{inter}}}\left( {\boldsymbol{k}} \right)$ as displayed in Fig. 4e–h. Consequently, the sign of BCD and SD flips upon ferroelectric transition between −iFE and +iFE, demonstrated in Fig. 4c, d. Therefore, in direct contrast to bilayer WTe₂, the nonlinear dc current in trilayer WTe₂ will switch its direction upon ferroelectric transition. The calculated BCD ranges from 0 to 0.7 Å depending on the chemical potential, also in good agreement with experiment.¹⁰ Moreover, there is a clear plateau in $D_{yz}^{{\mathrm{C}},{\mathrm{inter}}}$ marked by purple arrow in Fig. 4c. It is originated from the large joint density of state around 120 meV indicated by purple arrow in Fig. 4a, which remains constant when the chemical potential is located between the energy window. It is also worth to note that, like the bilayer case, the integral of Berry curvature of trilayer WTe₂ is also zero due to the presence of time-reversal symmetry, hence the linear anomalous Hall effect is absent. Both in-plane and out-of-plane spin polarizations are reversed (see Supplementary Figs. 5 and 6). Finally, the dc current susceptibility of bilayer and trilayer WTe₂ will be reversed in the trilayer case only. Figure 5 shows the interband LPGE susceptibility σ_abc of bilayer and trilayer WTe₂ at μ = 0, which is about 10 times higher than that in monolayer group IV monochalcogenides.²³ It is clear that in bilayer WTe₂ the two independent susceptibility tensor elements σ_xxx and σ_xyy of the ±mFE states remain invariant upon ferroelectric transition, while for trilayer WTe₂ both σ_xxx and σ_xyy of the ±iFE states flip the sign.

Fig. 4: Electronic structure and FNAHE of trilayer WTe₂.

a, b Band structure of trilayer WTe₂ in −iFE and +iFE state, respectively. Both are color-coded by the z-component of intraband Berry curvature ${\mathrm{\Omega }}_n^z\left( {\boldsymbol{k}} \right)$. c, d BCD tensor elements $D_{yz}^{{\mathrm{intra}}}\left( \mu \right)$ and $D_{yz}^{{\mathrm{C}},{\mathrm{inter}}}\left( {\mu ,\omega } \right)$, and SD tensor element $D_{xy}^{{\mathrm{L}},{\mathrm{inter}}}\left( {\mu ,\omega } \right)$ as function of chemical potential μ for −iFE and +iFE state, respectively. For interband BCD and SD, ω is set to 120 meV. e, f k-dependent distribution of intraband Berry curvature ${\mathrm{\Omega }}_{{\mathrm{occ}}}^{z,{\mathrm{intra}}}\left( {\boldsymbol{k}} \right)$ at μ = ±50 meV for −iFE and +iFE state, respectively. g, h k-dependent distribution interband Berry curvature ${\mathrm{\Omega }}_{nm}^{z,{\mathrm{inter}}}\left( {\boldsymbol{k}} \right)$ between (VBM-1,CBM) around the Fermi surface for −iFE and +iFE state, respectively. The results clearly show that nonlinear anomalous Hall current in trilayer WTe₂ will switch sign upon ferroelectric transition, in direct contrast to the bilayer case.

Fig. 5: Interband LPGE susceptibility σ_abc of bilayer and trilayer WTe₂ at μ = 0.

a σ_abc for the ±mFE states of bilayer WTe₂. b σ_abc for the ±iFE state of trilayer WTe₂. These two plots demonstrate that, under ferroelectric transition, the sign of LPGE-induced dc current susceptibility switches in the trilayer case, but remains invariant in the bilayer case.

The above electronic structure results demonstrate a striking difference between bilayer and trilayer WTe₂, that is, nonlinear anomalous Hall current flips its direction upon ferroelectric switching in trilayer WTe₂, but remains unchanged in bilayer WTe₂.

Group theoretical analysis of NAHE in bilayer and FNAHE in trilayer WTe₂

Here we provide a group theoretical analysis of NAHE in addition to the above first-principles calculations. Both bilayer and trilayer WTe₂ have C_s point group with a mirror symmetry M_y. For circularly polarized incident light propagating along z, (E(ω) × E(−ω))_z, shares the same A″ representation as axial vector R_z. Therefore, $\Gamma _{j_y} \otimes \Gamma _{{\boldsymbol{R}}_{{\boldsymbol{x}},{\boldsymbol{z}}}} = A^{\prime\prime} \otimes A^{\prime\prime} = A^{\prime}$, suggesting $\Gamma _{j_y} \otimes \Gamma _{{\boldsymbol{R}}_{{\boldsymbol{x}},{\boldsymbol{z}}}}$ includes total symmetric irreducible representation, and hence nonlinear CPGE current can be induced along y, i.e., perpendicular to the xz mirror plane. Furthermore, $\Gamma _{j_{x,z}} \otimes \Gamma _{{\boldsymbol{R}}_{{\boldsymbol{x}},{\boldsymbol{z}}}} = A^{\prime\prime}$, thus no CPGE current can be induced along x. In contrast, for linearly polarized incident light/electric field with in-plane polarization, we have ${\mathrm{\Gamma }}_{j_x} \otimes {\mathrm{\Gamma }}_{E_x} \otimes {\mathrm{\Gamma }}_{E_x} = A^{\prime} \otimes A^{\prime} \otimes A^{\prime} = A^{\prime}$, and ${\mathrm{\Gamma }}_{j_x} \otimes {\mathrm{\Gamma }}_{E_y} \otimes {\mathrm{\Gamma }}_{E_y} = A^{\prime} \otimes A^{\prime\prime} \otimes A^{\prime\prime} = A^{\prime}$, indicating that the LPGE current can be induced along x. However, ${\mathrm{\Gamma }}_{j_y} \otimes {\mathrm{\Gamma }}_{E_x} \otimes {\mathrm{\Gamma }}_{E_x} = {\mathrm{\Gamma }}_{j_y} \otimes {\mathrm{\Gamma }}_{E_y} \otimes {\mathrm{\Gamma }}_{E_y} = A^{\prime\prime}$, thus no LPGE current can be induced along y. This leads to a contrasting CPGE- and LPGE-based nonlinear anomalous Hall current in few-layer WTe₂ with C_s point group, that is, linearly polarized light/electric field with in-plane polarization will generate nonlinear anomalous Hall current along x only $(j_x^L\, \ne\, 0,j_y^L = 0)$, while circularly polarized light propagating along z axis will generate nonlinear anomalous Hall current along y only $(j_x^C = 0,j_y^C \,\ne\, 0)$.

The correlation between the irreducible representations of parent group C_2h and its noncentrosymmetric subgroups C₂, C_s, and C₁ is summarized in Supplementary Table 1. We start from monolayer 1T′ WTe₂ which has point group of C_2h, whose second order nonlinear current response vanishes due to the presence of inversion symmetry. Upon vdW stacking (e.g. few-layer and bulk T_d WTe₂), C_2y is broken with M_y left unchanged, which breaks the inversion symmetry and results in subgroup C_s. Consequently, as we analyzed above, $j_x^C = 0$, but $j_y^C \,\ne\, 0$ under circularly polarized light, while $j_x^L \,\ne\, 0$ but $j_y^L = 0$ under linearly polarized light/electric field with in-plane polarization. However, if M_y is broken with C_2y being preserved, it will fall into subgroup C₂. In this case, $j_x^C \,\ne\, 0$ and $j_y^C = 0$ under circularly polarized light, while $j_x^C = 0$ and $j_y^C \,\ne\, 0$ under linearly x/y-polarized light/electric field. Furthermore, if both M_y and C_2y are broken, it will end up with subgroup C₁, and enable all possible LPGE and CPGE current responses along different directions.

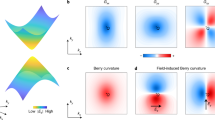
We now discuss the fundamental difference of NAHE between bilayer and trilayer WTe₂ upon ferroelectric switching. A general symmetry operator in Seitz notation is given by g = {R|t_R}, where R is point group symmetry operation and t_R is a translational vector. A time-reversal antisymmetric pseudovector (e.g. Berry curvature and spin polarization) transforms under operator g as follows, m’(k) = gm(k) = P_RP_TRm(k), where P_R and P_T are spatial and temporal parity associated with g, respectively. P_T = ±1 when Rk = ±k + K, where K is multiples of reciprocal lattice vector. For bilayer WTe₂, as aforementioned, two ferroelectric states can be related by a glide plane operation $\left\{{\mathcal{M}}_{z} | t_{a} \right\}$, where t_a refers to a fractional translation along x. Thus, P_R = −1, P_T = 1, and (m_x, m_y, m_z)^+mFE = (−m_x, −m_y, m_z)^−mFE. For trilayer WTe₂, the two ferroelectric states are related by an inversion operation $\left\{{\mathcal{I}} | 0 \right\}$, thus P_R = P_T = −1, subsequently (m_x, m_y, m_z)^+iFE = (−m_x, −m_y, −m_z)^−iFE. The above two conclusions are applicable to any time-reversal antisymmetric pseudovectors such as Berry curvature and spin polarization. For example, for intraband and interband Berry curvature, ${\mathcal{M}}_{z} {\mathrm{\Omega}}^{z}\left( {k_{x},k_{y}} \right) = {\mathrm{\Omega}}^{z}\left( {k_{x},k_{y}} \right)$ in bilayer WTe₂, and ${\mathcal{I}}{\mathrm{\Omega}}^{z}\left( {k_{x},k_{y}} \right) = {\mathrm{\Omega}}^{z}\left( {- k_{x}, - k_{y}} \right)\mathop { \to }\limits^{TRI} - {\Omega}^{z}\left( {k_{x},k_{y}} \right)$ in trilayer WTe₂, indicating that the sign of intraband and interband BCD ($D_{ab}^{{\mathrm{intra}}}$, $D_{ab}^{{\mathrm{C}},{\mathrm{inter}}}$) flips only in trilayer WTe₂ upon ferroelectric transition. This is in excellent agreement with the first-principles calculations shown in Fig. 3c–f and Fig. 4e–h. In addition, the in-plane spin polarization switches in both cases, and the out-of-plane spin polarization becomes reversed in trilayer WTe₂ while remaining unflipped for bilayer WTe₂, which also agrees with the calculations (Supplementary Figs. 3–6). Different from pseudovectors, polar vector p such as electric polarization and shift vector transforms as follows: p′ = Rp Therefore, both mirror ${\mathcal{M}}_{z}$ and inversion $\cal{I}$ operation will lead to vertical polarization reversal, i.e. $p_{z}^{\prime} = {\mathcal{M}}_{z}p_{z} = - p_{z}$ and $p_{z}^{\prime} = {\cal{I}}p_{z} = - p_{z}$, i.e. the out-of-plane electric dipole flips sign in both bilayer and trilayer WTe₂ upon ferroelectric transition. In addition, for in-plane shift vector $R_{mn}^{a}$ with a ∈ {x, y}, $\left(R_{mn}^{a}\right)^{\prime} = {\mathcal{M}}_{z} R_{mn}^{a} = R_{mn}^{a}$, and $\left(R_{mn}^{a}\right)^{\prime} = {\mathcal{I}}R_{mn}^{a} = - R_{mn}^{a}$, indicating that the in-plane shift vector $R_{mn}^{a}$ and thus SD $D_{yz}^{{\mathrm{L}},{\mathrm{inter}}}$ will flip only in trilayer WTe₂ upon ferroelectric transition. Consequently, the total $j_{x}^{L}$ and $j_{y}^{C}$ from CPGE and LPGE will switch direction upon ferroelectric transition, provoking FNAHE in time-reversal invariant semimetals. Moreover, it suggests that the BCD and SD can serve as distinct order parameters for noncentrosymmetric semimetals. Figure 6a presents an illustrative summary of the transformation of Berry curvature, spin polarization, and electric polarization under different symmetry operation, while Fig. 6b, c show the ferroelectric switching of nonlinear current in the −iFE and +iFE state of trilayer WTe₂. Upon the out-of-plane polarization switching, nonlinear Hall current $j_{x}^{L}$ generated via LPGE switches between −x and +x direction under the same external electric field with in-plane linear polarization. Moreover, nonlinear Hall current $j_{x}^{C}$ induced by CPGE switches between −y and +y direction under circularly-polarized light with normal incidence. It’s worth to emphasize that the intermediate PE state in bilayer and trilayer WTe₂ has noncentrosymmetric C_2v and centrosymmetric C_2h point group, respectively. Thus, despite that the out-of-plane electric polarization vanishes in both cases, nonlinear anomalous Hall current of the PE state vanishes in trilayer, but remains finite in bilayer.

Fig. 6: Transformation of pseudovectors and polar vectors under different symmetry operation and ferroelectric switching of LPGE and CPGE nonlinear current.

a Transformation of Berry curvature, spin polarization, shift vector, BCD and SD as well as LPGE and CPGE nonlinear current under different symmetry operation between two ferroelectric states in time-reversal invariant few-layer WTe₂. Berry curvature and spin polarization transform as time-reversal antisymmetric pseudovectors. Under a mirror symmetry operation ${\cal{M}}_{z}$ for the ferroelectric states in bilayer WTe₂, most quantities remain invariant, except in-plane spin and Berry curvature component. Under inversion symmetry operation ${\cal{I}}$ for the −iFE and +iFE state states in trilayer WTe₂, all quantities, including Berry curvature, spin polarization, shift vector, BCD and SD, flip the sign in the presence of time-reversal symmetry, giving rise to FNAHE in trilayer WTe₂. b, c Ferroelectric switching of nonlinear current in the −iFE and +iFE state of trilayer WTe₂, respectively. Upon the polarization P_z switching, nonlinear anomalous Hall current $j_{x}^{L}$ from LPGE switches between −x and +x direction under external field with in-plane linear polarization, while nonlinear anomalous Hall current $j_{y}^{C}$ from CPGE switches between −y and +y direction under circularly-polarized light with normal incidence.

The present work considers intrinsic NAHE due to BCD. Disorder however can play an important role in NAHE as pointed out by Du et al.³⁵ and Isobe et al.³⁶ particularly in the dc limit due to side jump and skew scattering.³⁷ As disorder scattering depends on scattering potential and defect density, further experimental studies are required to understand the nature of defects in bilayer and trilayer WTe₂. The transformation behavior of BCD-induced NAHE in multilayer WTe₂ upon ferroelectric transition may be utilized to distinguish itself from the disorder scattering-induced NAHE. For example, upon ferroelectric transition, the change in defect scattering potential may behave very differently from the change in crystal structure, thereby potentially helping differentiate the two NAHE contributions.

In conclusion, using first-principles calculations and group theoretical analyses we investigated the NAHE in bilayer and trilayer WTe₂ and, more importantly, the underlying microscopic origin of FNAHE (i.e., ferroelectric switching of NAHE) in trilayer WTe₂. Although both bilayer and trilayer WTe₂ exhibit ferroelectric transition with similar electric polarization, they behave very differently in NAHE. In the trilayer case, the nonlinear anomalous Hall current flips direction upon ferroelectric switching due to the reversal of BCD and SD under an effective inversion operation of the two ferroelectric states. In contrast, the two ferroelectric states in bilayer WTe₂ are related effectively by a glide plane operation which does not flip the BCD/SD, thus its nonlinear anomalous Hall current will not flip upon ferroelectric switching. In addition, NAHE is expected to vanish in the PE state of trilayer WTe₂, but remains nontrivial for the PE state of the bilayer case. The above conclusions are applicable to any even and odd layer WTe₂ (except monolayer 1T′ WTe₂ as it is centrosymmetric with vanishing second order NAHE) as long as the two opposite ferroelectric states have the same relationship as the bilayer and trilayer case. The theoretical approaches presented here can also be applied to other materials such as Weyl semimetals.^24,25

More importantly, our results imply that BCD and SD can serve as new order parameters for noncentrosymmetric materials, which opens up the possibility to explore nonlinear multiferroicity based on the coupling of BCD/SD and ferroelectric order. Ferroelectric metals may be advantageous as their vanishing bandgap will not only bring intraband contributions to nonlinear anomalous Hall current that is absent in semiconductors/insulators, but also significantly enhance the interband contributions due to the reduced gap of nonlinear interband processes. For example, the calculated nonlinear anomalous Hall current from interband LPGE in bilayer and trilayer WTe₂ is about one order of magnitude higher than that in ferroelectric GeS.²³ Moreover, FNAHE provides a facile approach for direct readout of ferroelectric states, which, combined with vertical ferroelectric writing, may allow for realizing nonlinear multiferroic memory. In addition, the distinct ferroelectric transformation pathway may provide potential routes to realizing non-abelian reciprocal braiding of Weyl nodes.³⁸ The present findings therefore reveal an underexplored realm beyond classical linear Hall effect and conventional ferroelectrics with exciting new opportunities for FNAHE-based nonlinear quantum electronics using ferroelectric metals and Weyl semimetals.

Methods

First-principles calculations of atomistic and electronic structure

First-principles calculations for structural relaxation and electric polarization were performed using density-functional theory^30,31 as implemented in the Vienna Ab initio Simulation Package (VASP)³² with the projector-augmented wave method.³⁹ We employed the generalized-gradient approximation of exchange-correlation functional in the Perdew–Burke–Ernzerhof form,⁴⁰ a plane-wave basis with an energy cutoff of 300 eV, a Monkhorst–Pack k-point sampling of 6 × 12 × 1 for the Brillouin zone integration, and optB88-vdW functional⁴¹ to account for dispersion interactions. Ground state crystal structures were obtained by fully relaxing both atomic positions and in-plane lattice parameters while keeping a large vacuum region of ~20 Å along the out-of-plane direction to reduce the periodic image interactions. The convergence criteria for maximal residual force was <0.005 eV/Å, and the convergence criteria for electronic relaxation is 10⁻⁶ eV. We have tested higher energy cutoff of 400 eV, and the difference in the lattice constants is <0.04%. Crystal structures of bilayer and trilayer WTe₂ can be found in the Supplementary Information. In addition, total electric polarization was calculated by directly integrating the product of charge density/ionic charge and their corresponding position without using Berry phase approach.

First-principles electronic structure calculations of NAHE

To compute the NHLE-related quantities, we first construct quasiatomic spinor Wannier functions and tight-binding Hamiltonian from Kohn–Sham wavefunctions and eigenvalues under the maximal similarity measure with respect to pseudoatomic orbitals.^33,34 Spin-orbit coupling is taken into account, and hybrid exchange-correlation energy functional HSE06⁴² is employed with the range-separation parameter λ = 0.2 (see Supplementary Information for more details). Total 112 and 168 quasiatomic spinor Wannier functions were obtained for bilayer and trilayer WTe₂, respectively. Using the developed tight-binding Hamiltonian we then compute CPGE and LPGE susceptibility tensor with a modified WANNIER90 code⁴³ using a dense k-point sampling of 600 × 600 × 1 for both bilayer and trilayer WTe₂. A small imaginary smearing factor η of 0.05 eV is applied to fundamental frequency, and Sokhotski–Plemelj theorem is employed for the Dirac delta function integration. In addition, we tested the range separation parameter λ in the hybrid HSE functional. Although the values of Berry curvature etc. can change with respect to λ, the presence (absence) of Berry curvature switching in trilayer (bilayer) remains the same (see Supplementary Fig. 3 for HSE functional with λ = 0.4). We also checked the convergence of the k-point sampling by increasing it to 1000 × 1000 × 1 (Supplementary Fig. 7). Finally, since few-layer WTe₂ is either semimetallic or having very small gap, the dielectric screening is large, thus the effect of the Coulombic interaction between electrons and holes is negligible.