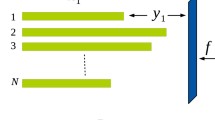Abstract
We study the velocity-force (V-F) relation for a Brownian ratchet consisting of a linear rigid polymer growing against a diffusing barrier, acted upon by a opposing constant force (F). Using a careful mathematical analysis, we derive the V-F relations in the extreme limits of fast and slow barrier diffusion. In the first case, V depends exponentially on the load F, in agreement with the well-known formula proposed by Peskin, Odell and Oster (1993), while the relationship becomes linear in the second case. For a bundle of two filaments growing against a common barrier, equal sharing of load in the corresponding V-F relation is predicted by a mean-field argument in both limits. However, the scaling behaviour of velocity with the number of filaments is different for the two cases. In the limit of large D, the validity of the mean-field approach is tested, and partially supported by a detailed and rigorous analysis. Our principal predictions are also verified in numerical simulations.
Graphic abstract




Similar content being viewed by others
References
T.L. Hill, M.W. Kirschner, Int. Rev. Cytol. 78, 1–125 (1982)
C.S. Peskin, G.M. Odell, G.F. Oster, Biophys. J. 65(1), 316–324 (1993)
G.S. van Doorn, C. Tănase, B.M. Mulder, M. Dogterom, Eur Biophys J. 29(1), 2–6 (2000)
S. Inoué, E.D. Salmon, Collected Works Of Shinya InouÉ: Microscopes, Living Cells, and dynamic molecules. (2008);p. 749–770
S. Dumont, T.J. Mitchison, Curr Biol. 19(17), R749–R761 (2009)
N. Pavin, I.M. Tolić, Ann. Rev. Biophys. 45, 279–298 (2016)
H. Hotani, H. Miyamoto, Adv Biophys. 26, 135–156 (1990)
T.D. Pollard, J.A. Cooper, Science 326(5957), 1208–1212 (2009)
D. Bray, Cell movements: from molecules to motility. Garland Science; (2000)
V. Argiro, M. Bunge, M. Johnson, J. Neurosci. Res. 13(1–2), 149–162 (1985)
J.A. Theriot, T.J. Mitchison, L.G. Tilney, D.A. Portnoy, Nature 357(6375), 257–260 (1992)
L.G. Tilney, D.A. Portnoy, J. Cell Biol. 109(4), 1597–1608 (1989)
G.A. Dabiri, J.M. Sanger, D.A. Portnoy, F.S. Southwick, Proc. Natl. Acad. Sci. USA 87(16), 6068–6072 (1990)
J. Sanger, J. Sanger, F. Southwick, Infect Immun. 60(9), 3609–3619 (1992)
K. Tsekouras, D. Lacoste, K. Mallick, J.F. Joanny, New. J. Phys. 13(10), 103032 (2011)
X. Li, A.B. Kolomeisky, J. Phys. Chem. B. 119, 4653–4661 (2015)
R. Wang, A. Carlsson, New J. Phys. 16(11), 113047 (2014)
D. Das, D. Das, R. Padinhateeri, New J. Phys. 16(6), 063032 (2014)
M. Dogterom, B. Yurke, Science 278(5339), 856–860 (1997)
D. Démoulin, M.F. Carlier, J. Bibette, J. Baudry, Proc. Natl. Acad. Sci. USA 111(50), 17845–17850 (2014)
D.R. Kovar, T.D. Pollard, Proc. Natl. Acad. Sci. USA 101(41), 14725–14730 (2004)
M.J. Footer, J.W. Kerssemakers, J.A. Theriot, M. Dogterom, Proc. Natl. Acad. Sci. USA 104(7), 2181–2186 (2007)
N.J. Burroughs, D. Marenduzzo, J. Phys. Condens Matter. 18(14), S357 (2006)
M.E. Fisher, A.B. Kolomeisky, Proc. Natl. Acad. Sci. USA 98(14), 7748–7753 (2001)
E.B. Stukalin, A.B. Kolomeisky, J. Chem. Phys. 121(2), 1097–1104 (2004)
J. Krawczyk, J. Kierfeld, EPL. 93, 28006 (2011)
J. Valiyakath, M. Gopalakrishnan, Sci. Rep. 8(1), 2526 (2018)
C.L. Cole, H. Qian, Biophys. Rev. Lett. (2011);6(01n02):59–79
A. Perilli, C. Pierleoni, G. Ciccotti, J.P. Ryckaert, J. Chem. Phys. 148(9), 095101 (2018)
A.E. Carlsson, Biophys. J. 81(4), 1907–1923 (2001)
A. Mogilner, G. Oster, Biophys. J. 71(6), 3030–3045 (1996)
F. Gerbal, P. Chaikin, Y. Rabin, J. Prost, Biophys. J. 79(5), 2259–2275 (2000)
Y. Inoue, T. Adachi, Biomech. Model Mechanobiol. 10(4), 495–503 (2011)
Acknowledgements
VY would like to thank the computational facilities provided by the High-Performance Computational Environment (HPCE), IIT Madras.
Author information
Authors and Affiliations
Contributions
Both the authors contributed equally to conceptualization and implementation of the work, as well as the mathematical calculations. V.Y developed the numerical codes for simulations.
Corresponding author
Appendix A: On load-sharing properties of a two-filament bundle
Appendix A: On load-sharing properties of a two-filament bundle
Here, we explore the question of load sharing in a two-filament bundle, outside the MFA. Consider the reflecting boundary condition \(J(x_1=0,x_2)=0\) imposed at the first barrier, which implies the relation
After differentiating Eq. (A.1) with respect to \(x_2\), and putting \(x_2=0\), we arrive at
Let us define the function \(\psi (x)=\theta (x,0)=\theta (0,x)\), where the second equality follows from symmetry of \(\theta (x_1,x_2)\) with respect to exchange of \(x_1\) and \(x_2\). This definition, when used in Eq. A.2, gives
Next, use the reflecting boundary condition at the second barrier, i.e. \(J(x_1,x_2=0)\), which provides the relation
which, when used in Eq. A.3, yields the following useful relation between the second derivatives:
Let us now revisit Eq. 31 for the two-filament bundle, and put \(x_2=0\). It then follows that the function \(\psi (x)\) satisfies the equation
where we have used the exact result \(T(x,0)=0\) for any x (see Eq. 32). In Laplace space, Eq. A.6 has the solution
where we have used the reflecting boundary condition \(2D\psi ^{\prime }(0) +f\psi (0)=0\) for \(\psi (x)\), which follows from \(J(x_1=0,x_2=0)=0\). The inverse Laplace transform of Eq. A.7 gives
where \(\alpha _1=f/D\). Taylor expansion of Eq. A.8 around \(x=0\) yields the expression
It is confirmed readily by observation that Eq. 38 and Eq. 45, derived under the MFA, are consistent with Eq. A.9 up to \({{\mathcal {O}}}(x)\).
Rights and permissions
About this article
Cite this article
Yadav, V., Gopalakrishnan, M. Force-velocity relation and load-sharing in the linear polymerization ratchet revisited: the effects of barrier diffusion. Eur. Phys. J. E 45, 35 (2022). https://doi.org/10.1140/epje/s10189-022-00190-6
Received:
Accepted:
Published:
DOI: https://doi.org/10.1140/epje/s10189-022-00190-6