Skip to main content
SpringerLink
Log in

Analytic Derivation of the Longitudinal Proton Structure Function FL(x, Q2) and the Reduced Cross Section σr(x, Q2) at the Leading Order and the Next-to-leading Order Approximations

  • Published:
International Journal of Theoretical Physics Aims and scope Submit manuscript

Abstract

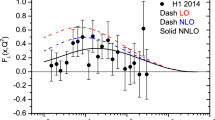
We present a set of formulas to extract the longitudinal proton structure function FL(x, Q2) and the reduced cross section σr(x, Q2) by using Dokshitzer–Gribov–Lipatov–Altarelli–Parisi (DGLAP) evolution equations and Altarelli-Martinelli equation at the leading order (LO) and the next-to-leading order (NLO) approximations in perturbative quantum chromodynamics (QCD). These formulas are obtained by the Laplace transform method at the virtualities higher than \({Q_{0}^{2}}\). We show that, the obtained equations for FL and σr depend only on the parton distribution functions (PDF’s) at the initial scale \({Q_{0}^{2}}\). We obtain the corresponding numerical results in a range of the virtuality \({Q_{0}^{2}}\leq Q^{2} \leq 800\) GeV2 and Bjorken scale 10− 4 ≤ x ≤ 1 and compare them with the results achieved by MSTW2008 (Martin et al. Eur. Phys. J 63(2), 189–285, 2009), WT (White and Thorne Phy. Rev. D 75(3), 034005, 2007) and Dipole (b-Sat) model (H. Kowalski, L. Motyka, G. Watt, Phys. Rev. D 74(7), 074016, 2006) predictions and also with data released by the Hadron Electron Ring Accelerator (HERA). Our numerical results show an acceptable agreement with the deep inelastic scattering (DIS) experimental data throughout over a wide range of the Bjorken scale x and the virtuality Q2, and then can be applied in analyses of the Large Hadron Collider and Future Circular Collider projects.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

Notes

  1. Here and subsequently, the subscript (1) and (2) denote the LO and NLO approximations, respectively.

References

  1. Glück, M., Reya, E., Vogt, A.: Dynamical parton distributions of the proton and small-x physics. Zeitschrift für Physik C Particles and Fields 67(3), 433–447 (1995)

    Article  ADS  Google Scholar 

  2. Martin, A.D., Stirling, W.J., Thorne, R.S., Watt, G.: Parton distributions for the LHC. Eur. Phys. J. C 63(2), 189–285 (2009)

    Article  ADS  Google Scholar 

  3. Khanpour, H., Mirjalili, A., Atashbar Tehrani, S.: Analytic derivation of the next-to-leading order proton structure function \({F_{2}^{p}} (x, Q^{2})\) based on the Laplace transformation. Phys. Rev. C 95(3), 035201 (2017)

    Article  ADS  Google Scholar 

  4. Zarrin, S., Boroun, G.R.: Solution of QCD ⊗ QED coupled DGLAP equations at NLO. Nuclear Physics B 922, 126–147 (2017)

    Article  ADS  Google Scholar 

  5. Martin, A.D.: Proton structure, Partons, QCD, DGLAP and beyond. arXiv:0802.0161 (2008)

  6. Boroun, G.R., Zarrin, S., Teimoury, F.: Decoupling of the DGLAP evolution equations by Laplace method. The European Physical Journal Plus 130 (10), 1–6 (2015)

    Article  Google Scholar 

  7. Navelet, H., Peschanski, R., Royon, C.h., Wallon, S.: Proton structure functions in the dipole picture of BFKL dynamics. Phys. Lett. B 385(1-4), 357–364 (1996)

    Article  ADS  Google Scholar 

  8. Hentschinski, M., Sabio Vera, A., Salas, C.: F2 and FL at small x using a collinearly improved BFKL resummation. Phys. Rev. D 87(7), 07600 (2013)

    Article  Google Scholar 

  9. Rezaei, B., Boroun, G.R.: Longitudinal structure function from the parton parameterization. The European Physical Journal A 56(10), 1–15 (2020). (ok)

    Article  Google Scholar 

  10. Boroun, G.R.: Hard-pomeron behavior of the longitudinal structure function FL in the next-to-leading order at low x. Int. J. Mod. Phys. E 18, 131 (2009)

    Article  ADS  Google Scholar 

  11. White, C.D., Thorne, R.S.: Global t to scattering data with next-to-leading logarithmic BFKL resummations. Phys. Rev. D 75(3), 034005 (2007)

    Article  ADS  Google Scholar 

  12. Baruah, N., Sarma, J.K.: Evolutions of longitudinal structure function FL upto Next-to-Next-to-Leading orders at small-x. Int. J. Theor. Phys. 53(7), 2492–2504 (2014)

    Article  Google Scholar 

  13. Kotikov, A.V., Parente, G.: The longitudinal structure function FL as a function of f2 and \(df_{2}/d \ln q^{2}\) at small x. The Next-to-Leading analysis. Modern Phys. Lett. A 12(13), 963–973 (1997)

    Article  ADS  Google Scholar 

  14. Rezaei, B., Boroun, G.R.: Analytical solution of the longitudinal structure function FL in the leading and next-to-leading-order analysis at low x with respect to Laguerre polynomials method. Nucl. Phys. A 857(1), 42–47 (2011)

    Article  ADS  Google Scholar 

  15. Kaptari, L.P., Kotikov, A.V., Chernikova, N.Yu., Zhang, P.: Longitudinal structure function fl at small x extracted from the Berger–Block–Tan parametrization of f2. JETP Lett. 109(5), 281–285 (2019)

    Article  ADS  Google Scholar 

  16. Kaptari, L.P., Kotikov, A.V., Yu Chernikova, N., Zhang, P.: Extracting the longitudinal structure function FL(x, Q2) at small x from a Froissart-bounded parametrization of F2(x, Q2). Phys. Rev. D 99(9), 096019 (2019)

    Article  ADS  Google Scholar 

  17. Block, M.M., Durand, L., Ha, P., McKay, D. W.: Applications of the leading-order Dokshitzer-Gribov-Lipatov-Altarelli-Parisi evolution equations to the combined HERA data on deep inelastic scattering. Phys. Rev. D 84(9), 094010 (2011)

    Article  ADS  Google Scholar 

  18. Block, M.M., Durand, L., Ha, P.: Connection of the virtual γ∗ p cross section of e p deep inelastic scattering to real γ p scattering, and the implications for ν N and ep total cross sections. Phys. Rev. D 89(9), 094027 (2014)

    Article  ADS  Google Scholar 

  19. Boroun, G.R.: Nonlinear correction to the longitudinal structure function at small x. The European Physical Journal A 43(3), 335–338 (2010)

    Article  ADS  Google Scholar 

  20. Baruah, N., Das, M.K., Sarma, J.K.: Longitudinal structure function F L of proton from regge like behaviour of structure function at small-x. Few-Body Systems 55(11), 1061–1071 (2014)

    Article  ADS  Google Scholar 

  21. Boroun, G.R.: Longitudinal structure function from logarithmic slopes of F 2 at low x. Phys. Rev. C 97(1), 015206 (2018)

    Article  ADS  Google Scholar 

  22. Golec-Biernat, K., Staśto, A. M.: FL proton structure function from the unified DGLAP/BFKL approach. Phys. Rev. D 80(1), 014006 (2009)

    Article  ADS  Google Scholar 

  23. Andreev, V., Baghdasaryan, A., Baghdasaryan, S., Begzsuren, K., Belousov, A., Belov, P., Boudry, V., et al.: Measurement of inclusive ep cross sections at high Q2 at \(\sqrt s= 225\) and 252GeV and of the longitudinal proton structure function fL at HERA. Eur. Phys. J. C 74(4), 2814 (2014)

    Article  ADS  Google Scholar 

  24. Aaron, F.D., Abramowicz, H., Abt, I., Adamczyk, L., Adamus, M., Al-daya Martin, M., Alexa, C., et al.: Combined measurement and QCD analysis of the inclusive e±p scattering cross sections at HERA. Journal of High Energy Physics 2010(1), 109 (2010)

    Article  Google Scholar 

  25. Abramowicz, H., Abt, I., Adamczyk, L., Adamus, M., Aggarwal, R., Antonelli, S., Arslan, O., et al.: Deep inelastic cross-section measurements at large y with the ZEUS detector at HERA. Phys. Rev. D 90(7), 072002 (2014)

    Article  ADS  Google Scholar 

  26. Aaron, F.D., Alexa, C., Andreev, V., Antunovic, B., Aplin, S., Asmone, A., Astvatsatourov, A., et al.: Measurement of the proton structure function fL(x, q2) at Low x. Phys. Lett. B 665(4), 139–146 (2008)

    Article  ADS  Google Scholar 

  27. White, C.D., Thorne, R.S.: Global fit to scattering data with next-to-leading logarithmic BFKL resummations. Phys. Rev. D 75(3), 034005 (2007)

    Article  ADS  Google Scholar 

  28. Kowalski, H., Motyka, L., Watt, G.: Exclusive diffractive processes at HERA within the dipole picture. Phys. Rev. D 74(7), 074016 (2006)

    Article  ADS  Google Scholar 

  29. Moch, S., Vermaseren, J.A., Vogt, A.: The longitudinal structure function at the third order. Phys. Lett. B 606(1-2), 123–129 (2005)

    Article  ADS  Google Scholar 

  30. Dokshitzer, Y.L.: Calculation of the structure functions for deep inelastic scattering and e+e− annihilation by perturbation theory in quantum chromodynamics. Zh. Eksp. Teor. Fiz 73, 1216 (1977)

    Google Scholar 

  31. Altarelli, G., Parisi, G.: Asymptotic freedom in parton language. Nucl. Phys. B 126(2), 298–318 (1977). (23)

    Article  ADS  Google Scholar 

  32. Gribov, V.N., Lipatov, L.N.: Deep Inelastic Ep-Scattering in a Perturbation Theory. Inst. of Nuclear Physics, Leningrad (1972)

    Google Scholar 

  33. Altarelli, G., Martinelli, G.: Transverse momentum of jets in electroproduction from quantum chromodynamics. Phys. Lett. B 76(1), 89–94 (1978)

    Article  ADS  Google Scholar 

  34. Furmanski, W., Petronzio, R.: Singlet parton densities beyond leading order. Phys. Lett. B 97(CERN-TH-2933), 437–442 (1980)

    Article  ADS  Google Scholar 

  35. Curci, G., Furmanski, W., Petronzio, R.: Evolution of parton densities beyond leading order the non-singlet case. Nucl. Phys. B 175(1), 27–92 (1980)

    Article  ADS  Google Scholar 

  36. Block, M.M., Durand, L., Ha, P., McKay. D.W.: Analytic solution to leading order coupled DGLAP evolution equations A new perturbative QCD tool. Phys. Rev. D 83(5), 054009 (2011)

    Article  ADS  Google Scholar 

  37. Vermaseren, J.A.M., Vogt, A., Moch, S.: The third-order QCD corrections to deep-inelastic scattering by photon exchange. Nucl. Phys. B 724(1-2), 3–182 (2005)

    Article  ADS  MathSciNet  Google Scholar 

Download references

Acknowledgements

We would like to thank Dr. M. Enayati for his help and for productive discussions.

Author information

Authors and Affiliations

  1. Department of Physics, University of Sistan and Baluchestan, Zahedan, Iran

    S. Zarrin & S. Dadfar

Authors
  1. S. Zarrin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. Dadfar
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. Zarrin.

Additional information

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix A: The Coefficients of the Longitudinal Proton Structure Function and their Laplace Transforms

The coefficients \(k_{ij}^{(1)}\) (i, j=f, g) and \(k_{ffns}^{(1)}\) in (17), (18) and (19) are as follows:

$$ k_{ff}^{(1)}(s,\tau(Q^{2},{Q^{2}_{0}})=\exp \left( \frac{1}{2}\tau({\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)})\right) \left( \cosh \left( \frac{T \tau}{2}\right)+\frac{({\Phi}_{f}^{(1)}-{\Phi}_{g}^{(1)}) \sinh \left( \frac{T \tau}{2}\right)}{T}\right), $$
(A1)
$$ k_{fg}^{(1)}(s,\tau(Q^{2},{Q^{2}_{0}}))=\exp \left( \frac{1}{2} \tau ({\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)})\right)\frac{2 {\Phi}_{f}^{(1)} \sinh \left( \frac{T \tau}{2}\right)} {T}, $$
(A2)
$$ k_{gf}^{(1)}(s,\tau(Q^{2},{Q^{2}_{0}})=\exp \left( \frac{1}{2} \tau ({\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)})\right)\frac{2 {\Phi}_{g}^{(1)} \sinh \left( \frac{T \tau}{2}\right) }{T}, $$
(A3)
$$ k_{gg}^{(1)}(s,\tau(Q^{2},{Q^{2}_{0}}) =\exp \left( \frac{1}{2} \tau ({\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)})\right) \left( \cosh \left( \frac{T \tau}{2}\right)-\frac{({\Phi}_{f}^{(1)}-{\Phi}_{g}^{(1)}) \sinh \left( \frac{T \tau}{2}\right)}{T}\right), $$
(A4)
$$ k_{ffns}^{(1)}(s,\tau(Q^{2},{Q^{2}_{0}}) = \exp \left( \tau{\Phi}_{nsf}^{(1)}\right) $$
(A5)

where \(T=\sqrt {({\Phi }_{f}^{(1)}-{\Phi }_{g}^{(1)})^{2}+4 {\Phi }_{f}^{(1)} {\Phi }_{g}^{(1)}}\). The coefficients \(K_{L,i}^{(2)}(x,Q^{2}) \) (i = ns, s, g) in (28), are as follows:

$$ \begin{array}{@{}rcl@{}} K_{L,ns}^{(2)}(x,Q^{2})&=& \frac{\alpha_{s}^{(2)}(Q^{2})}{\pi}C_{F}x^{2}+\bigg(\frac{\alpha_{s}^{(2)}(Q^{2})}{4\pi}\bigg)^{2}\\ &&x \bigg[\frac{128}{9}x\times\ln(1 - x)^{2} - 46.50x\ln(1 - x) - 84.094\ln(x)\ln(1 - x) - 37.338 \\ &&+89.53x+33.82x^{2}+x\ln(x)(32.90 + 18.41\ln(x))-\frac{128}{9}\ln(x)\\ &&-0.012\delta(x_{1})+\frac{16}{27}n_{f}(6x\ln(1-x)-12x\ln(x)-25x+6)\bigg], \end{array} $$
(A6)
$$ \begin{array}{@{}rcl@{}} K_{L,s}^{(2)}(x,Q^{2})&=&K_{L,ns}^{(2)}(x,Q^{2})+\bigg(\frac{\alpha_{s}^{(2)}(Q^{2})}{4\pi}\bigg)^{2}xn_{f}\bigg[(15.94-5.212x)(1-x)^{2}\ln(1-x)\\ &&+(0.421+1.520x)\ln(x)^{2} \\ &&+28.09(1-x)\ln(x)-(2.370x^{-1}-19.27)(1-x)^{3}\bigg], \end{array} $$
(A7)
$$ \begin{array}{@{}rcl@{}} K_{L,g}^{(2)}(x,Q^{2})&=& \frac{\alpha_{s}^{(2)}(Q^{2})}{\pi}2n_{f}x^{2}(1-x) + \bigg(\frac{\alpha_{s}^{(2)}(Q^{2})}{4\pi}\bigg)^{2}\\ &&xn_{f}\bigg[(94.74-49.20x)(1-x)\ln(1-x)^{2}+864.8(1-x)\ln(1-x) \\ &&+1161x\ln(1-x)\ln(x)+60.06(1-x)\ln(x)^{2}+39.66(1-x)\ln(x)\\ &&-5.333(x^{-1}-1)\bigg]. \end{array} $$
(A8)

And, the Laplace transforms of \(K_{L,i}^{(2)}(x,Q^{2}) \) (i = ns, s, g), which we used in (34) are as:

$$ \begin{array}{@{}rcl@{}} k_{L,ns}^{(2)}(s,Q^{2})&=&\frac{{\alpha_{s}^{2}}(Q^{2})C_{F}}{\pi} \frac{1}{ (s+2)}+ \bigg(\frac{\alpha_{s}(Q^{2})}{4\pi}\bigg)^{2}\bigg[-0.012+\frac{128}{9(1+s)^{2}}-\frac{37.338}{(1+s)}\\ &&+\frac{32n_{f}}{9(1+s)} +\frac{36.82}{(2+s)^{3}}-\frac{32.9}{(2+s)^{2}} +\frac{64n_{f}}{9(2+s)^{2}}+\frac{89.53}{(2+s)}-\frac{400n_{f}}{27(2+s)}\\ &&+\frac{33.82}{(3+s)}+\frac{46.5H_{(2+s)}}{(2+s)}-\frac{32n_{f}H_{(2+s)}}{9(2+s)}-\frac{84.094}{(1+s)^{3}}\\ &&\bigg(2+\gamma_{E}+\gamma_{E}s +(1+s)\psi(1+s)-(1+s)^{2}\psi{'}(1+s)\bigg)\\ &&+\frac{128(\pi^{2}+6H_{(2+s)}^{2}-6\psi{'}(3+s)}{9(12+6s)}\bigg], \end{array} $$
(A9)
$$ \begin{array}{@{}rcl@{}} k_{L,s}^{(2)}(s,Q^{2})&=&\frac{{\alpha_{s}^{2}}(Q^{2})C_{F}}{\pi} \frac{1}{ (s+2)}+ \bigg(\frac{\alpha_{s}(Q^{2})}{4\pi}\bigg)^{2}\bigg[-0.012-\frac{2.371n_{f}}{s}+\frac{0.842n_{f}}{(1+s)^{3}}\\ &&+\frac{128}{9(1+s)^{2}}-\frac{28.09n_{f}}{(1+s)^{2}}-\frac{37.338}{(1+s)} \\ &&+\frac{29.9386n_{f}}{1+s}+\frac{36.82}{(2+s)^{3}}+\frac{3.04n_{f}}{(2+s)^{3}}-\frac{32.9}{(2+s)^{2}}+\frac{35.201n_{f}}{(2+s)^{2}}+\frac{89.53}{(2+s)}\\ &&-\frac{79.7378n_{f}}{(2+s)}+\frac{33.82}{(3+s)}+\frac{60.181n_{f}}{(3+s)}-\frac{19.27n_{f}}{(4+s)} \\ &&-\frac{15.49n_{f}H_{(1+s)}}{(1+s)}+\frac{46.5H_{(2+s)}}{(2+s)}+\frac{32.6364n_{f}H_{(2+s)}}{(2+s)}-\frac{25.914n_{f}H_{(3+s)}}{(3+s)}\\ &&+\frac{5.212n_{f}H_{(4+s)}}{(4+s)}-\frac{84.094}{(1+s)^{3}}\\ &&\bigg(2+\gamma_{E}+\gamma_{E}s +(1+s)\psi(1+s)-(1+s)^{2}\psi{'}(1+s)\bigg)\\ &&+\frac{128(\pi^{2}+6H_{(2+s)}^{2}-6\psi{'}(3+s)}{9(12+6s))}\bigg], \end{array} $$
(A10)
$$ \begin{array}{@{}rcl@{}} k_{L,g}^{(2)}(s,Q^{2})&=&\frac{2\alpha_{s}(Q^{2})n_{f}}{\pi}\left( \frac{1}{ (s+2)}-\frac{1}{ (s+3)} \right)+n_{f} \bigg(\frac{\alpha_{s}(Q^{2})}{4\pi}\bigg)^{2}\\ &&\bigg[-\frac{5.333}{s}-\frac{39.66}{(1+s)^{2}}+\frac{5.333}{(1+s)}+\frac{120.12}{(2+s)^{3}}+\frac{39.66}{(2+s)^{2}} \\ &&-\frac{864.8H_{(1+s)}}{(1+s)}+\frac{864.8H_{(2+s)}}{(2+s)}+\frac{1161}{(1+s)^{2}(2+s)^{3}}\\ &&\bigg(5+\gamma_{E}(1 + s)^{2}(2 + s)+2s(3+s)+(1+s)^{2}(2+s)\psi(3+s)-(1+s)^{2} \\ &&\times(2+s)^{2}\psi{'}(1+s)\bigg)\frac{94.74(\pi^{2}+6H_{(1+s)}^{2}-6\psi{'}(2+s))}{(6+6s)}\\ &&-\frac{143.94(\pi^{2}+6H_{(2+s)}^{2}-6\psi{'}(3+s))}{(12+6s)} \\ &&+\frac{49.2(\pi^{2}+6H_{(3+s)}^{2}-6\psi{'}(4+s))}{(18+6s)}\bigg], \end{array} $$
(A11)

where ψ(s) is defined by \(\psi (s)=\frac {d}{ds}{\Gamma }(s)\), H(s) is the s-th harmonic number, \(C_{F}=\frac {4}{3}\) and γE is the Euler-Lagrange constant. The coefficients of \(k_{i,j}^{(2)}(s,\tau (Q^{2},{Q^{2}_{0}})) \) (i, j = f, g) in (41) and (42) and the coefficient of \(k_{ffns}^{(2)}(s,\tau (Q^{2},{Q^{2}_{0}})) \) in (43) are as:

$$ \begin{array}{@{}rcl@{}} k_{ff}^{(2)}(s,\tau(Q^{2},{Q^{2}_{0}}))&=&\bigg[ - e^{\frac{1}{2} \tau \left( -2 b+{\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)}-T\right)} \bigg(a b^{2} \bigg({\Phi}_{f}^{(1)} {\Phi}_{f}^{(2)} \left( - \left( e^{b \tau}-1\right)\right) \left( e^{\tau T}-1\right)\\ &&+{\Phi}_{f}^{(2)} \left( e^{b \tau}-1\right) \bigg({\Phi}_{g}^{(1)} \left( e^{\tau T}-1\right) \\ &&-T \left( e^{\tau T}+1\right)\bigg)+2 \left( e^{\tau T}-1\right) \left( {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}-{\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} e^{b \tau}\right)\bigg)\\ &&+b \bigg(e^{\tau \left( b+T\right)} \bigg({\Phi}_{f}^{(1)} (T^{2}-a ({\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+{\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)})) \\ &&+2 a {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+{\Phi}_{g}^{(1)} (a {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+a {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}-T^{2})\\ &&+a T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}-a T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}+T^{3}\bigg)+e^{b \tau} \bigg({\Phi}_{f}^{(1)} (a {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} \\ &&+a {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}-T^{2})+2 a {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})\\ &&+{\Phi}_{g}^{(1)} (T^{2}-a ({\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+{\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}))+a T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}-a T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}+T^{3}\bigg)+a e^{\tau T} \\ &&\times \bigg(-{\Phi}_{f}^{(1)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}-{\Phi}_{f}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)} +2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})\\ &&+{\Phi}_{g}^{(1)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+{\Phi}_{g}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}-T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)\\ &&+a \bigg({\Phi}_{f}^{(1)} \times {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+{\Phi}_{f}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)} -2 {\Phi}_{f}^{(2)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)}-{\Phi}_{g}^{(1)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}\\ &&-{\Phi}_{g}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}+2 {\Phi}_{g}^{(2)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)}-T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)\bigg)+a T \end{array} $$
$$ \begin{array}{@{}rcl@{}} &&\times \left( e^{b \tau}-1\right) \bigg({\Phi}_{f}^{(1)} {\Phi}_{f}^{(2)} T \left( e^{\tau T}-1\right)+{\Phi}_{f}^{(1)} \bigg(e^{\tau T}+1\bigg) ({\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+{\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}) \\ &&-{\Phi}_{f}^{(2)} {\Phi}_{g}^{(1)} T e^{\tau T} +{\Phi}_{f}^{(2)} {\Phi}_{g}^{(1)} T+{\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} (2 {\Phi}_{g}^{(2)}-2 {\Phi}_{f}^{(2)}) \\ &&\times e^{\tau T}+{\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} (2 {\Phi}_{g}^{(2)}-2 \times{\Phi}_{f}^{(2)}) +{\Phi}_{f}^{(2)} T^{2} e^{\tau T}+{\Phi}_{f}^{(2)} T^{2}-{\Phi}_{g}^{(1)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} e^{\tau T}\\ &&-{\Phi}_{g}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)} e^{\tau T}-{\Phi}_{g}^{(1)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}-{\Phi}_{g}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}+T \\ &&\times{\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} e^{\tau T}+T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)} e^{\tau T}-T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} -T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)\\ &&-b^{3} e^{b \tau} \left( {\Phi}_{f}^{(1)} \left( e^{\tau T}-1\right)-{\Phi}_{g}^{(1)} e^{\tau T}+{\Phi}_{g}^{(1)}+T \left( e^{\tau T}+1\right)\right)\bigg)\bigg] \\ &&\Bigg/\bigg[2 T \left( b^{3}-b T^{2}\right)\bigg], \end{array} $$
(A12)
$$ \begin{array}{@{}rcl@{}} k_{fg}^{(2)}(s,\!\tau(Q^{2},\!{Q^{2}_{0}}))\!&=&\bigg[e^{\frac{1}{2} \tau \left( -2 b+{\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)}-3 T\right)} \bigg(a b^{2} e^{\tau T} \bigg(e^{b \tau} \left( {\Theta}_{f}^{(2)} \left( {\Phi}_{f}^{(1)}-{\Phi}_{g}^{(1)}-T\right)\right.\\ &&\left.+2 {\Phi}_{g}^{(2)} {\Theta}_{f}^{(1)}\right)-e^{\tau \left( b+T\right)} \bigg({\Theta}_{f}^{(2)} \bigg({\Phi}_{f}^{(1)} -{\Phi}_{g}^{(1)}+T\bigg)+2 {\Phi}_{g}^{(2)} {\Theta}_{f}^{(1)}\bigg)\\ &&+{\Theta}_{f}^{(2)} e^{\tau T} (-{\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)}+T)+{\Theta}_{f}^{(2)} \left( {\Phi}_{f}^{(1)}-{\Phi}_{g}^{(1)}+T\right)\\ &&+2 {\Phi}_{f}^{(2)} {\Theta}_{f}^{(1)} \left( e^{\tau T}-1\right)\bigg)+b \bigg(a \bigg(- e^{\tau \left( b+T\right)} \bigg({\Phi}_{f}^{(1)} {\Theta}_{f}^{(1)} ({\Phi}_{g}^{(2)} - {\Phi}_{f}^{(2)})\\ &&-{\Phi}_{f}^{(1)} T {\Theta}_{f}^{(2)}+{\Phi}_{g}^{(1)} {\Theta}_{f}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+T {\Theta}_{f}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})\\ &&+{\Phi}_{g}^{(1)} T {\Theta}_{f}^{(2)}+T^{2} {\Theta}_{f}^{(2)}-2 {\Theta}_{f}^{(1)2} {\Theta}_{g}^{(2)} -2 {\Theta}_{f}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)\\ &&+e^{\tau \left( b+2 T\right)}\! \bigg(\!{\Phi}_{f}^{(1)} {\Theta}_{f}^{(1)} ({\Phi}_{g}^{(2)} - {\Phi}_{f}^{(2)}) + {\Phi}_{f}^{(1)} T {\Theta}_{f}^{(2)} + {\Phi}_{g}^{(1)} {\Theta}_{f}^{(1)} \!(\!{\Phi}_{f}^{(2)}\! - {\Phi}_{g}^{(2)})\\ &&+T {\Theta}_{f}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)}) \\ &&-{\Phi}_{g}^{(1)} T {\Theta}_{f}^{(2)}+T^{2} {\Theta}_{f}^{(2)}-2 {\Theta}_{f}^{(1)2} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)\\ &&+e^{2 \tau T} \bigg({\Phi}_{f}^{(1)} {\Theta}_{f}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})-{\Phi}_{f}^{(1)} T {\Theta}_{f}^{(2)}+{\Phi}_{g}^{(1)} {\Theta}_{f}^{(1)} ({\Phi}_{f}^{(2)} \\ &&-{\Phi}_{g}^{(2)})+T {\Theta}_{f}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+{\Phi}_{g}^{(1)} T {\Theta}_{f}^{(2)}+T^{2} {\Theta}_{f}^{(2)}-2 {\Theta}_{f}^{(1)2} {\Theta}_{g}^{(2)}\\ &&-2 {\Theta}_{f}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)-e^{\tau T} \bigg({\Phi}_{f}^{(1)} {\Theta}_{f}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})+{\Phi}_{f}^{(1)} T {\Theta}_{f}^{(2)} \\ &&+{\Phi}_{g}^{(1)} {\Theta}_{f}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+T {\Theta}_{f}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})-{\Phi}_{g}^{(1)} T {\Theta}_{f}^{(2)}\\ &&+T^{2} {\Theta}_{f}^{(2)}-2 {\Theta}_{f}^{(1)2} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)\bigg)+2 T^{2} {\Theta}_{f}^{(1)} \\ &&\times\left( e^{\tau T}-1\right) e^{\tau \left( b+T\right)}\bigg)+a T {\Theta}_{f}^{(1)} \bigg(e^{\tau \left( b+2 T\right)} \bigg({\Phi}_{f}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})\\ &&+{\Phi}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})+T ({\Phi}_{f}^{(2)}+{\Phi}_{g}^{(2)})+2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} \end{array} $$
$$ \begin{array}{@{}rcl@{}} &&+2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)-e^{\tau \left( b+T\right)} \left( {\Phi}_{f}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})+{\Phi}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+T ({\Phi}_{f}^{(2)}+{\Phi}_{g}^{(2)})\right.\\ &&\left.-2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\right)-e^{2 \tau T} \bigg({\Phi}_{f}^{(1)} ({\Phi}_{f}^{(2)} \\ &&-{\Phi}_{g}^{(2)})+{\Phi}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})+T ({\Phi}_{f}^{(2)}+{\Phi}_{g}^{(2)})+2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)\\ &&+e^{\tau T} \bigg({\Phi}_{f}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})+{\Phi}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+T ({\Phi}_{f}^{(2)}+{\Phi}_{g}^{(2)}) \\ &&-2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)\bigg)-2 b^{3} {\Theta}_{f}^{(1)} \left( e^{\tau T}-1\right) e^{\tau \left( b+T\right)}\bigg)\bigg]\Bigg/\bigg[2 T \left( b^{3}-b T^{2}\right)\bigg],\\ \end{array} $$
(A13)
$$ \begin{array}{@{}rcl@{}} k_{gf}^{(2)}(s,\tau(Q^{2},{Q^{2}_{0}}))&=&-\bigg[e^{\frac{1}{2} \tau \left( -2 b+{\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)}-3 T\right)} \bigg(a b^{2} e^{\tau T} \bigg(-e^{\tau \left( b+T\right)} \left( {\Theta}_{g}^{(2)} \left( -{\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)}+T\right)\right.\\ &&\left.+2 {\Phi}_{f}^{(2)} {\Theta}_{g}^{(1)}\right) +e^{b \tau} \left( 2 {\Phi}_{f}^{(2)} {\Theta}_{g}^{(1)}-{\Theta}_{g}^{(2)} \left( {\Phi}_{f}^{(1)}-{\Phi}_{g}^{(1)}+T\right)\right)\\ &&+e^{\tau T} \left( {\Theta}_{g}^{(2)} \left( {\Phi}_{f}^{(1)}-{\Phi}_{g}^{(1)}+T\right)+2 {\Phi}_{g}^{(2)} {\Theta}_{g}^{(1)}\right)+{\Theta}_{g}^{(2)} \left( -{\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)}+T\right)\\ &&-2 {\Phi}_{g}^{(2)}\times {\Theta}_{g}^{(1)}\bigg)+b \bigg(a \bigg(e^{\tau \left( b+2 T\right)} \bigg({\Phi}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})-{\Phi}_{f}^{(1)} T {\Theta}_{g}^{(2)}\\ &&+{\Phi}_{g}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+T {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+{\Phi}_{g}^{(1)} T {\Theta}_{g}^{(2)}+T^{2} {\Theta}_{g}^{(2)} \\ &&-2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)2}\bigg)-e^{\tau \left( b+T\right)} \bigg({\Phi}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})\\ &&+{\Phi}_{f}^{(1)} T {\Theta}_{g}^{(2)}+{\Phi}_{g}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+T {\Theta}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)}) \\ &&-{\Phi}_{g}^{(1)} T {\Theta}_{g}^{(2)}+T^{2} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)2}\bigg)\\ &&-e^{\tau T} \bigg({\Phi}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})-{\Phi}_{f}^{(1)} T {\Theta}_{g}^{(2)}+{\Phi}_{g}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})\\ &&+T {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+{\Phi}_{g}^{(1)} T {\Theta}_{g}^{(2)}+T^{2} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} {\Theta}_{g}^{(2)}\\ &&-2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)2}\bigg)+e^{2 \tau T} \bigg({\Phi}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})+{\Phi}_{f}^{(1)} T {\Theta}_{g}^{(2)}+{\Phi}_{g}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)} \\ &&-{\Phi}_{g}^{(2)})+T {\Theta}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})-{\Phi}_{g}^{(1)} T {\Theta}_{g}^{(2)}+T^{2} {\Theta}_{g}^{(2)}\\ &&-2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)2}\bigg)\bigg)+2 T^{2} {\Theta}_{g}^{(1)} \left( e^{\tau T}-1\right) e^{\tau \left( b+T\right)}\bigg)+a T {\Theta}_{g}^{(1)} \\ &&\times\bigg(e^{\tau \left( b+2 T\right)} \left( {\Phi}_{f}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+{\Phi}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})+T ({\Phi}_{f}^{(2)}+{\Phi}_{g}^{(2)})\right.\\ &&\left.+2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\right)-e^{\tau \left( b+T\right)} \bigg({\Phi}_{f}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)}) \\ &&+{\Phi}_{g}^{(1)}({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+T ({\Phi}_{f}^{(2)}+{\Phi}_{g}^{(2)})-2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)\\ &&-e^{2 \tau T} \bigg({\Phi}_{f}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+{\Phi}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})+T ({\Phi}_{f}^{(2)}+{\Phi}_{g}^{(2)}) \end{array} $$
$$ \begin{array}{@{}rcl@{}} &&+2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)+e^{\tau T} \left( {\Phi}_{f}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})+{\Phi}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})\right.\\ &&\left.+T ({\Phi}_{f}^{(2)}+{\Phi}_{g}^{(2)})-2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}-2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\right)\bigg) \\ &&-2 b^{3} {\Theta}_{g}^{(1)} \left( e^{\tau T}-1\right) e^{\tau \left( b+T\right)}\bigg)\bigg)\Bigg/\bigg[2 T \left( b^{3}-b T^{2}\right)\bigg], \end{array} $$
(A14)
$$ \begin{array}{@{}rcl@{}} k_{gg}^{(2)}(s,\tau(Q^{2},{Q^{2}_{0}}))&=&-\bigg[e^{\frac{1}{2} \tau \left( -2 b+{\Phi}_{f}^{(1)}+{\Phi}_{g}^{(1)}-T\right)} \bigg(-a b^{2} \bigg({\Phi}_{f}^{(1)} {\Phi}_{g}^{(2)} \left( -\left( e^{b \tau}-1\right)\right) \left( e^{\tau T}-1\right)\\ &&+{\Phi}_{g}^{(1)} {\Phi}_{g}^{(2)} \left( e^{b \tau}-1\right) \left( e^{\tau T}-1\right) \\ &&+{\Phi}_{g}^{(2)} T e^{\tau \left( b+T\right)}+{\Phi}_{g}^{(2)} T \left( e^{b \tau}-1\right)+2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)} e^{\tau \left( b+T\right)}\\ &&-2 {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)} e^{b \tau}-{\Phi}_{g}^{(2)} T e^{\tau T}-2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} e^{\tau T}+2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}\bigg) \\ &&+b \bigg(e^{b \tau} \bigg({\Phi}_{f}^{(1)} (T^{2}-a ({\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+{\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}))+2 a {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})\\ &&+{\Phi}_{g}^{(1)} (a {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+a {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}-T^{2})-a T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} \\ &&+a T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}+T^{3}\bigg)+e^{\tau \left( b+T\right)} \bigg({\Phi}_{f}^{(1)} (a {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+a {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}-T^{2})\\ &&+2 a {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{g}^{(2)}-{\Phi}_{f}^{(2)})+{\Phi}_{g}^{(1)} (T^{2}-a ({\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+{\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)})) \\ &&-a T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+a T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}+T^{3}\bigg)-a e^{\tau T} \bigg(-{\Phi}_{f}^{(1)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}\\ &&-{\Phi}_{f}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}+2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+{\Phi}_{g}^{(1)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} \\ &&+{\Phi}_{g}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}-T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)+a \bigg(-{\Phi}_{f}^{(1)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}\\ &&-{\Phi}_{f}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}+2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})+{\Phi}_{g}^{(1)} {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} \\ &&+{\Phi}_{g}^{(1)} {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)} +T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}-T {\Theta}_{f}^{(2)}{\Theta}_{g}^{(1)}\bigg)\bigg)+a T \left( e^{b \tau}-1\right)\\ && \bigg(-{\Phi}_{f}^{(1)} \left( {\Phi}_{g}^{(2)} T \left( e^{\tau T}-1\right)+\left( e^{\tau T}+1\right) ({\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+{\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)})\right) \\ &&+2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)}) e^{\tau T}+2 {\Theta}_{f}^{(1)} {\Theta}_{g}^{(1)} ({\Phi}_{f}^{(2)}-{\Phi}_{g}^{(2)})\\ &&+{\Phi}_{g}^{(1)} {\Phi}_{g}^{(2)} T \left( e^{\tau T}-1\right)+{\Phi}_{g}^{(1)} \left( e^{\tau T}+1\right) ({\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}+{\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}) \\ &&+{\Phi}_{g}^{(2)} T^{2} e^{\tau T}+{\Phi}_{g}^{(2)} T^{2}+T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)} e^{\tau T} +T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)} e^{\tau T}\\ &&-T {\Theta}_{f}^{(1)} {\Theta}_{g}^{(2)}-T {\Theta}_{f}^{(2)} {\Theta}_{g}^{(1)}\bigg)-b^{3} e^{b \tau} \bigg(-{\Phi}_{f}^{(1)} e^{\tau T}+{\Phi}_{f}^{(1)} \\ &&+{\Phi}_{g}^{(1)} \left( e^{\tau T}-1\right)+T \left( e^{\tau T}+1\right)\bigg)\bigg)\bigg) \Bigg/\bigg[2 T \left( b^{3}-b T^{2}\right)\bigg], \end{array} $$
(A15)
$$ k_{ffns}^{(2)}(s,\tau(Q^{2},{Q^{2}_{0}}))= \exp\bigg[\frac{a{\Phi}_{nsf}^{(2)}(s)}{b}(1+e^{-b\tau(Q^{2},{Q^{2}_{0}})}) +{\Phi}_{nsf}^{(1)}(s)\tau(Q^{2},{Q^{2}_{0}})\bigg]. $$
(A16)

Appendix B: The Coefficients of the Proton Structure Function and their Laplace Transforms

The coefficients of \(C^{(j)}_{i}(s,\tau (Q^{2},{Q^{2}_{0}}))\) (i = ns, s, g and j = 1, 2) in (49) and their Laplace transforms in (50) are as:

$$ C^{(1)}_{ns}(x,\tau(Q^{2},{Q_{0}^{2}})) = \delta(1\!-x)x,\!\ \ C^{(1)}_{s}(x,\tau(Q^{2},{Q_{0}^{2}})) = \delta(1-x)x,\!\ \ C^{(1)}_{g}(x,\tau(Q^{2},{Q_{0}^{2}}))=0, $$
(B1)
$$ \begin{array}{@{}rcl@{}} C^{(2)}_{ns}(x,\tau(Q^{2},{Q_{0}^{2}}))&=&\delta(1-x)x+xC_{F}\frac{\tau}{4\pi}\bigg(4D_{1}-3D_{0}-(9+4\zeta_{2})\delta(1-x)\\ &&-2(1+x)(\ln(1-x)-\ln(x)) -4(1-x)^{-1}\ln(x)+6+4x\bigg),\\ \end{array} $$
(B2)
$$ C^{(2)}_{s}(x,\tau(Q^{2},{Q_{0}^{2}}))=C^{(2)}_{ns}(x,\tau(Q^{2},{Q_{0}^{2}})), $$
(B3)
$$ C^{(2)}_{g}(x,\tau(Q^{2},{Q_{0}^{2}}))=n_{f}x\left( (2-4x(1-x))(\ln(1-x)-\ln(x))-2+16x(1-x)\right), $$
(B4)
$$ c^{(1)}_{ns}(s,\tau(Q^{2},{Q_{0}^{2}}))=1,\ \ c^{(1)}_{s}(s,\tau(Q^{2},{Q_{0}^{2}}))=1,\ \ c^{(1)}_{g}(s,\tau(Q^{2},{Q_{0}^{2}}))=0, $$
(B5)
$$ \begin{array}{@{}rcl@{}} c^{(2)}_{ns}(s,\tau(Q^{2},{Q_{0}^{2}}))&=&1+C_{F}\frac{\tau}{4\pi}\bigg(-9-\frac{2\pi^{2}}{3}-\frac{2}{(1+s)^{2}}+\frac{6}{(1+s)}-\frac{2}{(2+s)^{2}}\\ &&+\frac{4}{(2+s)}+3 H_{(s)}+\frac{2 H_{(1+s)}}{1+s}+\frac{2 H_{(2+s)}}{2+s} \\ &&+\frac{1}{3}\left( \pi^{2}+6H_{(s)}^{2}-6\psi{'}(1+s)\right)+4\psi{'}(1+s)\bigg), \end{array} $$
(B6)
$$ c^{(2)}_{s}(x,\tau(Q^{2},{Q_{0}^{2}}))=c^{(2)}_{ns}(s,\tau(Q^{2},{Q_{0}^{2}})), $$
(B7)
$$ \begin{array}{@{}rcl@{}} c^{(2)}_{g}(s,\tau(Q^{2},{Q_{0}^{2}}))&=&n_{f} \left( -\frac{2 H_{(1+s)}}{1+s}+\frac{4 H_{(2+s)}}{2+s}-\frac{4 H_{(3+s)}}{3+s}-\frac{2}{1+s}+\frac{2}{(1+s)^{2}}\right.\\ &&\left.+\frac{16}{2+s}-\frac{4}{(2+s)^{2}}-\frac{16}{3+s}+\frac{4}{(3+s)^{2}}\right). \end{array} $$
(B8)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zarrin, S., Dadfar, S. Analytic Derivation of the Longitudinal Proton Structure Function FL(x, Q2) and the Reduced Cross Section σr(x, Q2) at the Leading Order and the Next-to-leading Order Approximations. Int J Theor Phys 60, 3822–3849 (2021). https://doi.org/10.1007/s10773-021-04947-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10773-021-04947-1

Keywords

Search

Navigation