Skip to main content
SpringerLink
Log in

Bipedal Gait Costs: a new case study of mathematical explanation in science

  • Paper in General Philosophy of Science
  • Published:
European Journal for Philosophy of Science Aims and scope Submit manuscript

Abstract

In this paper I present a case study of mathematical explanation in science that is new to the philosophical literature, and that arises in the context of estimating the energetic costs of running in bipedal animals. I refer to this as the Bipedal Gait Costs explanation (BGC). I argue that it is important for examples of applied mathematics to be driven not just by philosophical and mathematical concerns but also by scientific concerns. After a detailed presentation of the BGC case study, I discuss ways in which it is different from standard examples of MES. One distinctive aspect of BGC is that substantive mathematics occurs at two different levels. I argue for a distinction to be drawn between the mathematical modeling that occurs at one level, which is non-explanatory, and the mathematical theorem which is applied to results gleaned from these models, which is explanatory. I conclude by drawing some connections with broader indispensability-based arguments for platonism in the philosophy of mathematics.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Notes

  1. This terminology was introduced in Baker (2012). Note that just because the motivating puzzle in [Konigsberg], for example, was not scientific (and thus the explanation is not “science-driven”) does not mean that the general mathematical result concerning Eulerian graphs that appears in the explanation does not have other, scientifically useful applications.

  2. Colyvan [2001, p. 49]

  3. Although even [Cicadas] has been criticized by philosophers for not being faithful to scientific practice. See e.g. Wakil and Justus (2017).

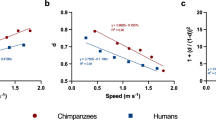
  4. The focus here is on running rather than walking. For terminological purposes, typically a gait is defined as running if the fraction of time for which each foot is in contact with the ground is less than 0.5, and as walking if this fraction is greater than 0.5. This proportion is referred to as the duty factor.

  5. Marsh et al. (2004)

  6. op. cit., p. 80

  7. op. cit., p. 81

  8. op. cit., p. 80

  9. op. cit., p. 82

  10. ibid.

  11. Rubenson and Marsh (2009, p. 1628). See also Daley and Biewener (2003) and Winter (1990) for further arguments in favor of these common modeling assumptions.

  12. Marsh et al. (2004, p. 82). See Armstrong and Laughlin (1983) for experimental details.

  13. This corresponds to the case in which p+ = 0, so there is no ‘bounce back’ of the center of mass following the collision of the leg with the ground.

  14. Ruina et al. (2005, p. 186). Data on human walking comes from Bertram (2005).

  15. For full details of this derivation, see Doke et al. (2005, p. 440).

  16. op. cit., pp. 442 – 3

  17. Here g1(v) and g2(v) are place-holders to indicate that the energy costs are also dependent on running velocity, v. As will become apparent, the precise nature of this relation does not matter to the overall explanation.

  18. The basics of this explanation are taken from Srinivisan (2006, pp. 103 – 106).

  19. For example, y = 100x4 + x-2 and z = x4 + 100x-2 both achieve their minimum value when the two summed power laws are in the ratio 2:1.

  20. This ratio is a positive constant, because (as noted earlier) δ > 0 and β < 0.

  21. Baron (2016, p. 459)

  22. ibid.

  23. Melia (2000, p. 473)

  24. The Level 1 / Level 2 distinction is not supposed to be an intrinsic feature of the type of mathematics involved, but only relative to the role played by a particular kind of mathematical apparatus in a given explanation. Also I am not claiming here that the Level 1 mathematics is definitely non-explanatory, just that the possibility of it being non-explanatory allows for an indexing view of kinematics and a commitment to the explanatoriness of the BGC case study to be simultaneously maintained.

  25. Baker (2017, p. 201)

  26. [Konigsberg] is another case where Level 1 mathematics is present, albeit minimally. In this case, it involves the mathematical modeling of the system of bridges as a mathematical graph with landmasses as nodes and bridges as links.

  27. I don’t mean to suggest that there is some essential connection between the presence of Level 1 mathematics and being rooted in scientific practice. However, I do think that there is a correlation between these two features.

  28. e.g. Frigg (2010), Bokulich (2012).

  29. By contrast, philosophers less sympathetic to the force of the Indispensability Argument (in either its original Quinean form or its more recent Enhanced formulation) have often cited idealizations as a problematic issue. See e.g. Maddy (1992), Batterman (2010).

  30. Ruina et al. (2005, p. 174)

  31. op. cit., p. 173

References

  • Armstrong, R., & Laughlin, H. (1983). Blood flows within and among rat muscles as function of time during high speed treadmill exercise. Journal of Physiology, 344, 189–208.

    Article  Google Scholar 

  • Baker, A. (2005). Are there genuine mathematical explanations of physical phenomena? Mind, 114, 223–238.

    Article  Google Scholar 

  • Baker, A. (2009). Mathematical explanation in science. British Journal for the Philosophy of Science, 60, 611–633.

    Article  Google Scholar 

  • Baker, A. (2012). Science-driven mathematical explanation. Mind, 121, 243–267.

    Article  Google Scholar 

  • Baker, A. (2017). Mathematics and explanatory generality. Philosophia Mathematica, 25, 194–209.

    Google Scholar 

  • Baron, S. (2014). Optimization and mathematical explanation: Doing the Lévy Walk. Synthese, 191, 459–479.

    Article  Google Scholar 

  • Baron, S. (2016). Explaining mathematical explanation. Philosophical Quarterly, 66, 458–480.

    Article  Google Scholar 

  • Batterman, R. (2002). The devil in the details. Oxford University Press.

    Google Scholar 

  • Batterman, R. (2010). On the explanatory role of mathematics in empirical science. British Journal for the Philosophy of Science, 61, 1–25.

    Article  Google Scholar 

  • Bertram, J. (2005). Constrained optimization in human walking: Cost minimization and gait plasticity. Journal of Experimental Biology, 208, 979–991.

    Article  Google Scholar 

  • Bokulich, A. (2012). Distinguishing explanatory from nonexplanatory fictions. Philosophy of Science, 79, 725–737.

    Article  Google Scholar 

  • Charles, W., et al. (2016). Comparison of point foot, collisional and smooth rolling contact models on the bifurcations and stability of bipedal walking. European Journal of Computational Mechanics, 25, 273–293.

    Article  Google Scholar 

  • Chirimuuta, M. (2018). Explanation in computation neuroscience: Causal and non-causal. British Journal for the Philosophy of Science, 69, 849–880.

    Article  Google Scholar 

  • Daley, M., & Biewener, A. (2003). Muscle force-length dynamic during level versus incline locomotion: A comparison of in vivo performance of two guinea fowl ankle extensors. Journal of Experimental Biology, 206, 2941–2958.

    Article  Google Scholar 

  • Doke, et al. (2005). Mechanics and energetics of swinging the human leg. Journal of Experimental Biology, 208, 439–445.

    Article  Google Scholar 

  • Fedak, M., et al. (1982). Energetics and mechanics of terrestrial locomotion: II. Total mechanical energy changes of the limbs and body as a function of speed and body size in birds and mammals. Journal of Experimental Biology, 79, 57–66.

    Google Scholar 

  • Frigg, R. (2010). Models and fiction. Synthese, 172, 251–268.

    Article  Google Scholar 

  • Lange, M. (2013). What makes a scientific explanation distinctively mathematical? British Journal for the Philosophy of Science, 64, 485–511.

    Article  Google Scholar 

  • Lange, M. (2015). Because without cause: Non-causal explanation in science and mathematics. Oxford University Press.

    Google Scholar 

  • Lyon, A., & Colyvan, M. (2008). The explanatory power of phase spaces. Philosophia Mathematica, 16, 227–243.

    Article  Google Scholar 

  • Maddy, P. (1992). Indispensability and practice. Journal of Philosophy, 89, 275–289.

    Article  Google Scholar 

  • Marsh, et al. (2004). Partitioning the energetics of walking and running: Swinging the limbs is expensive. Science, 303(5654), 80–83.

    Article  Google Scholar 

  • Melia, J. (2000). Weaseling away the indispensability argument. Mind, 109, 455–480.

    Article  Google Scholar 

  • Pincock, C. (2007). Mathematical idealization. Philosophy of Science, 74, 957–967.

    Article  Google Scholar 

  • Pincock, C. (2012). Mathematics and scientific representation. Oxford University Press.

    Book  Google Scholar 

  • Rubenson, J., & Marsh, R. (2009). Mechanical efficiency of limb swing during walking and running in guinea fowl. Journal of Applied Physiology, 106, 1618–1630.

    Article  Google Scholar 

  • Ruina, A., et al. (2005). A collisional model of the energetic cost of support work. Journal of Theoretical Biology, 237, 170–192.

    Article  Google Scholar 

  • Srinivisan. (2006). Why walk and run: Energetic costs and energetic optimality in simple mechanics-based models of a bipedal animal, Ph.D. Dissertation (Cornell University).

  • Usherwood, J. (2020). An extension to the collisional model of the energetic cost of support qualitatively explains trotting and the trot-canter transition. Journal of Experimental Zoology, 333, 9–19.

    Article  Google Scholar 

  • Wakil, S., & Justus, J. (2017). Mathematical explanation and the biological optimality fallacy. Philosophy of Science, 84, 916–930.

    Article  Google Scholar 

  • Winter, D. (1990). Biomechanics and motor control of human movement. Wiley.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Philosophy, Swarthmore College, Swarthmore, PA, 19081, USA

    Alan Baker

Authors
  1. Alan Baker
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alan Baker.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

This article belongs to the Topical Collection: Dimensions of Applied Mathematics

Guest Editors: Matthew W. Parker, Davide Rizza

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Baker, A. Bipedal Gait Costs: a new case study of mathematical explanation in science. Euro Jnl Phil Sci 11, 95 (2021). https://doi.org/10.1007/s13194-021-00411-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s13194-021-00411-3

Keywords

Search

Navigation