1 INTRODUCTION

Creep, which refers to the slow deformation with time effect, means that under the condition of constant temperature and constant stress, the deformation of material will increase slowly with the increase of time; and even when the stress is less than the yield strength of the material, the creep deformation is still irreversible. As early as the 18th century, creep phenomenon has attracted people’s attention. In 1883, Vicat carried out experiments on steel cables and made quantitative analysis. In 1910, Andrade first proposed the item ‘creep’, which is still in use today [1].

The creep deformation is closely related to the stress level and temperature range. When the temperature reaches 0.3 times the melting point of the material, the creep phenomenon is obvious. Therefore, when the temperature is greater than or equal to 0.5 times of the material melting point, the creep deformation can never be ignored. But there are many factors affecting creep, and the creep mechanism is complex. It is very difficult to get a unified creep formula. Therefore, some assumptions are put forward to reflect the main factors of creep, and the creep theories are established. These theories mainly include aging theory, time hardening theory, strain hardening theory and plastic hysteresis theory.

At present, researchers mainly analyze the creep experimental data of some materials, and modify the parameters or coefficients based on some basic creep theories put forward by predecessors, so as to fit the creep constitutive equations of these materials. The well-known Dorn equation was used to describe the high temperature creep rate for steady-state creep [2]. A new approach to dislocation creep was proposed based on a reappraisal of diffusion controlled glide from microcosmic point of view [3]. The θ-projection method was used to characterize the creep deformation of DZ125 under different temperatures and stress levels. And a modified θ-projection method was employed to promote the adaptability of the original model to both steady state and transient temperature fields [4]. A material constitutive model and creep damage model considering the Orowan effect and the dislocation effect were established based on the crystal plasticity theory [5].

As to the parts working under high temperature for long term, the second stage of creep is the main object of study. Norton power law model is widely used, and the constitutive equations of some different materials are fitted [6–10]. As a new kind of superalloy, the experimental research on creep behavior of GH3128 under high temperature is not sufficient. Therefore, based on the high temperature tensile creep test of GH3128, the Norton power law model and another strain-time model which can reflect the first two stages of creep are used to fit the corresponding constitutive equations. And the calculated values of the constitutive equations are compared with the test values, so as to obtain the constitutive equations which can be used to estimate the creep deformation within the test ranges.

2 MATERIALS AND METHODS

GH3128 is a solution strengthening nickel base superalloy with tungsten and molybdenum as strengthening elements [11]. Due to its high plasticity, durable creep strength, good oxidation resistance and other properties, it is widely utilized in the manufacture of aero-engine combustor flame tube, afterburner housing, and other parts which work for long time at high temperature [12].

The specimens for high temperature tensile creep test were cut from an air-cooled billet with solid solution treatment for 2 hours at 1160°C. The composition content is shown in Table 1.

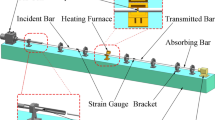
Table 1. Composition content of GH3128 specimens (wt %)
Full size table

Gleeble-3800 thermal-mechanical physical simulation machine was used to carry out high temperature tensile test. According to the dimensional requirement for tensile specimen of Gleeble-3800 test machine, a 10 mm diameter bar by 120 mm long with screw thread on both ends was used for the tensile test. When the specimen was fixed in the test machine, the gauge of specimen was 30 mm as shown in Fig. 1.

Fig. 1.
figure 1

The dimension of tensile specimen.

Full size image

Ni–Cr thermocouples welded in the middle of the specimens were used to measure and monitor the actual temperature during the test. The thermoplastic test of tensile specimen was carried out at the strain rate of 0.001 /s under the temperature of 850°C, 900°C and 950°C, respectively. Because there are no obvious yield platforms of the stress-strain curves at these temperatures, σ0.2 is chosen as the yield strength value.

Referring to the yield strength values obtained, the tensile stress value was set below the yield stress so that merely creep deformation occurred rather than plastic deformation. During the tensile creep test, the specimen was heated to test temperature at the rate of 10°C/s and was stretched after holding for 5 minutes. To study the creep deformation under high temperature and high stress, the specific parameters of tensile creep test were listed in Table 2.

Table 2. Parameters of creep tensile test
Full size table

As the steady-state creep was the main object of research, the duration of tensile test was set as 4 hours, because under the test temperature and stress, the data collected could be used to fit the constitutive equations.

3 RESULTS AND DISCUSSION

3.1 Creep Strain-Time Curve

As the applied stress is less than the yield stress, the deformation of the specimen is elastic deformation and creep deformation without plastic deformation. Figs. 2a, 2b, and 2c show the creep strain-time curves under different stress at the temperature of 850°C, 900°C and 950°C, respectively.

Fig. 2.
figure 2

Creep strain-time curves at different stress under the temperature of: (a) 850°C; (b) 900°C; (c) 950°C.

Full size image

The instantaneous strain produced at the moment of loading is elastic strain. As it was shown in Fig. 2, when the time is 0, the corresponding strain of each curve is elastic strain. But the values are much smaller than the creep strains. It can be observed from Fig. 2, when creep occurred at relatively low temperature of 850 °C, the specimens under various stress loads were mainly in the steady-state creep stage. The transition stage at the beginning of creep occupied the test time for about 2980 s, 1970 s, 1550 s and 1180 s, respectively under the stress of 50 MPa, 65 MPa, 80 MPa, and 100 MPa. There was no accelerated stage and no fracture occurred in the samples. When the test temperature was increased to 900°C, under the stress of 100 MPa, the sample entered the third stage of creep deformation at about 6500 s, and then fracture occurred at about 7100 s. When the test temperature was increased to 950°C, the specimens under the tensile loads of 80 MPa and 100 MPa began to enter the third stage of creep at about 7800 s and 3000 s, and then the fracture occurred at about 9000 s and 3500 s, respectively. From the test results it can be found that under the same temperature, with the increase of stress, the steady-stage creep time is shortened rapidly. Meanwhile the creep rate is obviously increased, and the material will enter the accelerated fracture stage earlier.

As can be seen from Fig. 2, the creep strain-time curves above accorded with the classical creep curves. And with the increase of test temperature and tensile load, the deformation caused by creep changed obviously. It also illustrates that the temperature and the stress loaded on the material have an important effect on the creep deformation and its rate, which is the key factor in the study of creep.

3.2 Creep Constitutive Equations

In order to solve the creep problem in engineering, it is often necessary to calculate the creep deformation of the object, and the creep constitutive equation is the basis. However, due to the complex creep mechanism and many factors affecting creep, it is difficult to get a unified equation to express creep deformation. As for long-term working parts at high temperature, the second stage of creep is the main research object and the constant rate creep theory is often applied [1]. Consequently, according to the constant rate creep theory, two creep constitutive equations based on strain-time creep formula and the Norton power-law creep formula were fitted by the data obtained from the tensile creep test.

In terms of the relationship between strain and time, the formula brought forward by Shuji Taira [13] is shown as follow:

$$\varepsilon = {{\varepsilon }_{0}} + b{{t}^{n}} + kt,$$
(3.1)

where, ε is stain; ε0 is the instantaneous elastic strain; btn is the strain caused by transition creep; and kt is the strain occurred in steady-state creep stage; n is a constant less than 1; b and k are parameters related with load and temperature; t is time (s). The above equation is taken the derivative of time as:

$$\dot {\varepsilon } = bn{{t}^{{n - 1}}} + k,$$
(3.2)

where, \(\dot {\varepsilon }\) is strain rate. In Eq. (3.2), the first term on the right plays a major role in the first stage of creep, which indicates that the strain rate decreases with the increase of time. At the second stage of creep, the second term plays a key role, which implies that the creep rate tends to be a constant value and there is a linear relationship between creep strain and time. Therefore, the value of parameter k can be obtained by linear regression.

Discarding the instantaneous elastic strain generated during loading, the logarithm is taken for Eq. (3.1), then it is changed as follow:

$$\ln \left( {\varepsilon - kt} \right) = \ln b + n\ln t.$$
(3.3)

Based on this equation, the logarithmic time-logarithmic strain curves at 850°C, 900°C and 950°C are shown in Figs. 3a, 3b and 3c, where εc represents the creep strain and its value equals to ε–kt in Eq. (3.3).

Fig. 3.
figure 3

Logarithmic creep strain-logarithmic time curves under the temperature of: (a) 850°C; (b) 900°C; (c) 950°C.

Full size image

The linear correlation coefficients (R2) are all up to 0.99, which indicates that the linear fitting degree is good. As shown in Table 3 (a, b, c), the values of n, lnb, and k under different stresses at various temperatures were listed.

Table 3. (a) Creep parameters at 850°C
Full size table

The parameter n in the Tables is taken average as n = 0.743. The parameters b and k can be expressed by the power exponent function of the stress as follows:

$$b = {{b}_{0}}{{\sigma }^{c}},$$
(3.4)
$$k = {{k}_{0}}{{\sigma }^{{c'}}},$$
(3.5)

where, c and c' are constant. The following two equations are obtained by taking logarithms on both sides of the above two equations:

$$\ln b = c\ln \sigma + \ln {{b}_{0}},$$
(3.6)
$$\ln k = c'\ln \sigma + \ln {{k}_{0}}.$$
(3.7)

In order to explore the relationship between lnb, lnk, stress σ and temperature T, the test data were fitted by linear regression, as shown in Figs. 4a and 4b.

Fig. 4.
figure 4

Relationship between (a) lnb, (b) lnk and lnσ.

Full size image

Figure 4a shows there is a linear relationship between lnb and logarithmic stress lnσ, and the correlation coefficients are more than 0.99. The arithmetic average of each slope is 1.322, that is, the value of parameter c is 1.322. Then the relationship between lnb0 and temperature T is obtained as Eq. (3.8) shown:

$$\ln {{b}_{0}} = 0.0006T - 17.183.$$
(3.8)

Similarly, Fig. 4b illustrates the linear relationship between lnσ and logarithmic steady-state creep rate lnk, and the correlation coefficients are more than 0.98. The arithmetic average of each slope is 4.128, that is, the value of c' is 4.128. Then the relationship between lnk0 and temperature T is got as Eq. (3.9) shown:

$$\ln {{k}_{0}} = 0.03229T - 60.839.$$
(3.9)

To sum up, the creep equation of stress, time, and temperature based on the strain-time formula can be expressed as Eq. (3.10):

$${{\varepsilon }_{c}} = \exp (0.0006T - 17.183){{\sigma }^{{1.322}}}{{t}^{{0.743}}} + \exp (0.03229T - 60.839){{\sigma }^{{4.128}}}t$$
(3.10)

At the conditions of 850°C–50 MPa, 950°C–65 MPa, 900°C–80 MPa, 950°C–100 MPa, the values εc calculated by Eq. (3.10) are compared with the test data, which are shown in Fig. 5.

Fig. 5.
figure 5

Comparison between test value and calculated value based on strain-time formula.

Full size image

It can be observed from Fig. 5 that under the conditions of 850°C–50 MPa, 950°C–65 MPa, and 950°C–100 MPa, the values εc calculated by Eq. (3.10) are all obviously greater than those obtained in creep test. Under the condition of 900°C–80 MPa, the calculation value of Eq. (3.10) is also higher than that of creep test. But at the end of creep test, the test value gradually exceeds the calculated value. This indicates that the constitutive Eq. (3.10) only can roughly reflect the first two creep stages of GH3128 at high temperature. On the other hand, it is safer and more reliable to estimate the service life of this material in the steady-state creep stage.

The Norton power law creep equation [14] shown as Eq. (3.11) is simplified from the Mukherjee-Bird-Dorn equation [15], which is put forward based on the microscopic mechanism of material. It is considered that the creep at high temperature is mainly a diffusion phenomenon.

$${{\dot {\varepsilon }}_{c}} = A{{\sigma }^{{n'}}}\exp \left( { - \frac{{{{Q}_{c}}}}{{R{{T}_{h}}}}} \right).$$
(3.11)

where, A is the dimensionless constant related to the microstructure of the material; Qc is the apparent activation energy (J); R is the gas Avogadro constant, 8.314 J/(mol · K); Th is the thermodynamic temperature (K); and \({{\dot {\varepsilon }}_{c}}\) is the strain rate of steady-state creep (the same as the values of k in Table 3 (a, b, c)).

Take the logarithm on both sides of the Eq. (3.11), the Eq. (3.12) is obtained.

$$\ln {{\dot {\varepsilon }}_{c}} = \ln A + n'\ln \sigma - \frac{{{{Q}_{c}}}}{{RTh}}.$$
(3.12)

It can be seen from Eq. (3.12) that it is transformed into a general linear equation when \(\ln {{\dot {\varepsilon }}_{c}}\) is the dependent variable and lnσ is independent variable. According to the tensile creep test data at temperatures of 850°C, 900°C and 950°C, the relationship between \(\ln {{\dot {\varepsilon }}_{c}}\) and lnσ are shown in Fig. 6a.

Fig. 6.
figure 6

Relationship between \({\text{lnln}}~{{\dot {\varepsilon }}_{c}}~\) and (a) lnσ; (b) 1/Th.

Full size image

Figure 6a shows that there is a good linear relationship between logarithmic stress and logarithmic steady-state creep rate. R2 in the graph is the correlation coefficient and its values are all above 0.99, which implies the fitting effect is excellent. The slope of the line in Fig. 6 is the value of n'. The stress index n' is averaged and 4.0913 is appropriate.

Similarly, Eq. (3.12) can also be transformed into a linear equation when \(\ln {{\dot {\varepsilon }}_{c}}\) is the dependent variable and 1/Th is the independent variable. The relationship between 1/Th and is shown in Fig. 6b.

The slope of the line in Fig. 6b is the value of –Qc/R, where R is the gas Avogadro constant. Through the average calculation of Qc, the apparent activation energy Qc in the equation can be got as 328.57 kJ/mol. When parameters n' and Qc are determined, then taken into Eq. (3.11), the parameter A is solved according to the value of steady-state creep rate, and its arithmetic average value is taken. Finally, the parameter A is determined to be 8.26 × 106. After each parameter is substituted into Eq. (3.11), the expression of steady-state creep rate can be described as follow:

$${{\dot {\varepsilon }}_{c}} = 8.26 \times {{10}^{6}}{{\sigma }^{{4.0913}}}\exp \left( { - \frac{{39520.1}}{{{{T}_{h}}}}} \right).$$
(3.13)

The constitutive equation can express the mathematical relationship between stress and strain rate, and it is a comprehensive reflection of the macroscopic mechanical properties of materials. Furthermore, to express the constitutive equation between stress and strain, the Eq. (3.14) [16] is taken as reference.

$${{\varepsilon }_{c}} = \frac{1}{{a + b{{t}^{{c - 1}}}}},$$
(3.14)

where, a, b, and c are all parameters related to temperature and stress. Tensile creep test data were taken into Eq. (3.14) to calculate the values of parameter a, b, and c at different temperature and stress, respectively. Afterwards, the relationship between ln(\({{\dot {\varepsilon }}_{c}}{\text{/}}A{{\dot {\varepsilon }}_{c}}{\text{/}}A\)) and 1/a, 1/b, c is shown as Figs. 7a and 7b.

Fig. 7.
figure 7

Relationship between ln(\({{\dot {\varepsilon }}_{c}}{\text{/}}A\)) and parameter of: (a) 1/a, 1/b; (b) c.

Full size image

Figure 7 illustrates that there is an apparent linear relationship between ln(\({{\dot {\varepsilon }}_{c}}{\text{/}}A\)) and 1/a, 1/b, c, respectively. It can be seen from the correlation coefficient that the fitting effect is excellent. The expressions of 1/a, 1/b, and c are shown as Eq. (3.15), Eq. (3.16), and Eq. (3.17), respectively.

$$\frac{1}{a} = 2.26E( - 5) + 1.19E( - 6)\ln \left( {{{\sigma }^{{4.0913}}}\exp \left( { - \frac{{39520.1}}{{{{T}_{h}}}}} \right)} \right),$$
(3.15)
$$\frac{1}{b} = - 2.3{\text{1}}E( - 5) - 1.24E( - 6)\ln \left( {{{\sigma }^{{4.0913}}}\exp \left( { - \frac{{39520.1}}{{{{T}_{h}}}}} \right)} \right),$$
(3.16)
$$c = 1.00085 + 4.19E( - 5)\ln \left( {{{\sigma }^{{4.0913}}}\exp \left( { - \frac{{39520.1}}{{{{T}_{h}}}}} \right)} \right).$$
(3.17)

The creep constitutive equation of GH3128 is obtained by substituting the expression of parameters a, b, and c into Eq. (3.14) respectively. The test data of 850°C–50 MPa, 950°C–65 MPa, 900°C–80 MPa, 950°C–100 MPa were compared with the calculated values as shown in Fig. 8.

Fig. 8.
figure 8

Comparison between test value and calculated value based on Norton power law creep equation.

Full size image

It can be observed from Fig. 8 that the calculated values are all greater than the test values in the first creep stage. While after the first stage, the calculated values are in good agreement with the experimental data under the same conditions. This creep equation can more accurately reflect the creep behaviour in second stage of GH3128 at high temperature.

The constitutive Eq. (3.10) is based on the strain-time model, and constitutive Eq. (3.14) is based on the Norton power law creep model. To compare the accuracy of the two constitutive equations, the calculated values of the two constitutive equations and the tensile creep test data are illustrated in Fig. 9.

Fig. 9.
figure 9

Comparison of calculated and test values based on two different models.

Full size image

In Fig. 9, the calculated value1 represents the calculated value of the creep constitutive Eq. (3.10) based on strain-time formula; the calculated value2 represents the calculated value of the creep constitutive Eq. (3.14) based on the Norton power law creep formula. Figure 9 shows the calculated value2 are more consistent with the actual test values than the calculated value1 under the same temperature and stress condition. Especially under the test condition of 850°C–50 MPa, the calculated value1 is obviously larger than the test value.

The tensile creep test in this paper was carried out under the conditions of high temperature and high stress. In this case, the movement of atoms was accelerated and the diffusion degree was intensified. According to the theoretical basis of the Norton power law creep formula, creep process is regarded as the diffusion process of atoms. While the strain-time creep formula regards time as the most important factor to the creep process. Therefore, compared with the calculated values based on the strain-time creep equation, the calculated values based on the Norton power law creep equation are closer to the test values.

4 CONCLUSIONS

In this paper, the creep constitutive equations of nickel base alloy GH3128 at high temperature are constructed based on the tensile creep test. The creep constitutive equation based on the strain-time theory only can roughly reflect the creep behaviour of GH3128 at high temperature. While the creep constitutive equation based on the Norton power law creep theory are in good agreement with the test values in the first two creep stages.

By contrast, the calculated values based on the Norton power law creep theory are much closer to the test values. The reason is inferred that the theoretical basis of the Norton power law creep formula is accord with the creep mechanism under high temperature and high stress. While the strain-time creep formula regards time as the most important factor to the creep process. Therefore, the Norton power law model is more suitable to determine the high temperature creep equation of GH3128 superalloy.

The creep constitutive equation of GH3128 deduced in this study reflects the creep constitutive relation of the material at high temperature well. This equation can be used to study the creep behaviour of GH3128 at the temperature of 850°C to 950°C and the stress of 50 MPa to 100 MPa. But owing to obtain the constitutive equations describing the first two stages of creep deformation, the test duration was only set as 4 hours. It only could be used to estimate the creep deformation of the material in the range of experimental temperature when the stress is relatively high. Therefore, this study merely fills the narrow gap and provides research basis of GH3128 under high temperature and high stress. However, for the fracture failure caused by creep under high stress, a large number of long-term creep tests are needed.