A Split-form, Stable CG/DG-SEM for Wave Propagation Modeled by Linear Hyperbolic Systems

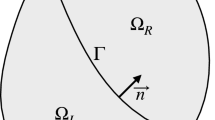
Abstract

We present a hybrid continuous and discontinuous Galerkin spectral element approximation that leverages the advantages of each approach. The continuous Galerkin approximation is used on interior element faces where the equation properties are continuous. A discontinuous Galerkin approximation is used at physical boundaries and if there is a jump in properties at a face. The approximation uses a split form of the equations and two-point fluxes to ensure stability for unstructured quadrilateral/hexahedral meshes with curved elements. The approximation is also conservative and constant state preserving on such meshes. Spectral accuracy is obtained for all examples, which include wave scattering at a discontinuous medium boundary.

Proof of Physical Boundary Dissipation

We show that (93) holds. As for the continuous problem, let be the normal coefficient matrix. The physical boundary can be viewed as being between two identical media so the numerical flux reduces to the standard upwind flux (75),

$$\begin{aligned} \mathbf{F}^{s,*}\left( \mathbf{U}_{L}, \mathbf{U}_{R}\right) = {A}^{s,+}\mathbf{U}_{L} + {A}^{s,-}\mathbf{U}_{R} = \frac{1}{2}\left( \mathbf{F}^{s}_{L} + \mathbf{F}^{s}_{R} \right) - \frac{1}{2}\left| {A}^{s}\right| \left( \mathbf{U}_{R} - \mathbf{U}_{L}\right) . \end{aligned}$$

(120)

Then

(121)

Since $\mathbf{U}^{s}$ is the value taken from the interior, i.e. $\mathbf{U}_{L}$,

(122)

But $\left| {A}^{s}\right| = {A}^{s,+}- {A}^{s,-}$, so

(123)

Also, ${A}^{s,-} = -\left| {A}^{s,-}\right|$, so

(124)

The last two terms are non-negative.

To simplify the next few steps, we define $\bar{\mathbf{U}} \equiv \sqrt{\left| {A}^{s,-}\right| }\mathbf{U}$. Then

(125)

Completing the square,

(126)

Replacing the external state $\bar{\mathbf{U}}_{R}$ with a boundary condition $\bar{\mathbf{g}}$,

(127)

Therefore,

(128)

When we return to the original variables, we get the desired result, (93).