Skip to main content
SpringerLink
Log in

An inertial Halpern-type CQ algorithm for solving split feasibility problems in Hilbert spaces

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

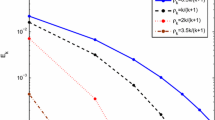
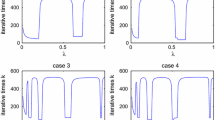
In this work, we introduce an inertial Halpern-type CQ algorithm for solving split feasibility problems in Hilbert spaces. The main advantages of the proposed algorithm are that the introduced stepsize is bounded away from zero and a strong convergence theorem for our method is established without assuming Lipschitz continuity of the gradient operator. Some preliminary numerical experiments are provided for illustration and comparison.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

References

  1. Agarwal, R.P., Regan, D.O., Sahu, D.R.: Fixed Point Theory for Lipschitzian-Type Mappings with Applications, Topological Fixed Point Theory and Its Applications. Springer, New York (2009)

    Google Scholar 

  2. Alvarez, F., Attouch, H.: An inertial proximal method for maximal monotone operators via discretization of a nonlinear oscillator with damping. Set-Valued Anal. 9, 3–11 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  3. Anh, P.K., Vinh, N.T., Dung, V.T.: A new self-adaptive CQ algorithm with an application to the lasso problem. J. Fixed Point Theory Appl. 20, 142 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  4. Antipin, A.S.: On a method for convex programs using a symmetrical modification of the Lagrange function. Ekon. Mat. Metody 12, 1164–1173 (1976)

    Google Scholar 

  5. Bauschke, H.H., Combettes, P.L.: A weak-to-strong convergence principle for fej\(\acute{e}\)r-monotone methods in Hilbert spaces. Math. Oper. Res. 26, 248–264 (2001)

    Article  MathSciNet  Google Scholar 

  6. Bauschke, H.H., Combettes, P.L.: Convex Analysis and Monotone Operator Theory in Hilbert Spaces. Springer, New York (2011)

    Book  MATH  Google Scholar 

  7. Byrne, C.: Iterative oblique projection onto convex sets and the split feasibility problem. Inverse Probl. 18, 441–453 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  8. Byrne, C.: A unified treatment of some iterative algorithms in signal processing and image reconstruction. Inverse Probl. 20, 103–120 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  9. Ceng, L.C., Ansari, Q.H., Yao, J.C.: Mann type iterative methods for finding a common solution of split feasibility and fixed point problems. Positivity 16, 471–495 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  10. Censor, Y., Elfving, T.: A multiprojection algorithm using Bregman projection in a product space. Numer. Algorithms 8, 221–239 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  11. Censor, Y., Elfving, T., Kopf, N., Bortfeld, T.: The multiple-sets split feasibility problem and its applications for inverse problems. Inverse Probl 21, 2071–2084 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  12. Cholamjiak, P., Sunthrayuth, P.: A Halpern-type iteration for solving the split feasibility problem and the fixed point problem of Bregman relatively non- expansive semigroup in Banach spaces. Filomat 32, 3211–3227 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  13. Dang, Y., Sun, J., Xu, H.: Inertial accelerated algorithm for solving a split feasibility problem. J. Ind. Manag. Optim. 13, 1383–1394 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  14. Dong, Q.L., Yao, Y., He, S.: Weak convergence theorems of the modified relaxed projection algorithms for the split feasibility problem in Hilbert spaces. Optim. Lett. 8, 1031–1046 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  15. Dong, Q.L., Tang, Y.C., Cho, Y.J., Rassias, Th.M.: ’ ’Optimal“ choice of the step length of the projection and contraction methods for solving the split feasibility problem. J. Global Optim. 71, 341–360 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  16. Dong, Q.L., Yuan, H.B., Cho, Y.J., Rassias, Th.M.: Modified inertial Mann algorithm and inertial CQ-algorithm for nonexpansive mappings. Optim. Lett. 12, 87–102 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  17. Ertürk, M., Gürsoy, F., Ansari, Q.H., Karakaya, V.: Modified Picard type iterative algorithm for nonexpansive mappings. J. Nonlinear Convex Anal. 19, 919–933 (2018)

    MathSciNet  MATH  Google Scholar 

  18. Ertürk, M., Gürsoy, F., Ansari, Q.H., Karakaya, V.: Picard type iterative method with applications to minimization problems and split feasibility problems. J. Nonlinear Convex Anal. 21, 943–951 (2020)

    MathSciNet  MATH  Google Scholar 

  19. Faikgürsoy, Sahu, D.R., Ansari, Q.H.: S-iteration process for variational inclusions and its rate of convergence. J. Nonlinear Convex Anal. 17, 1753–1767 (2016)

    MathSciNet  MATH  Google Scholar 

  20. Fukushima, M.: A relaxed projection method for variational inequalities. Math. Program. 35, 58–70 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  21. Gibali, A., Liu, L.W., Tang, Y.C.: Note on the modified relaxation CQ algorithm for the split feasibility problem. Optim. Lett. 12, 817–830 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  22. Gibali, A., Mai, D.T., Vinh, N.T.: A new relaxed CQ algorithm for solving split feasibility problems in Hilbert spaces and its applications. J. Ind. Manag. Optim. 15, 963–984 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  23. Halpern, B.: Fixed points of nonexpanding maps. Bull. Am. Math. Soc. 73, 957–961 (1967)

    Article  MathSciNet  MATH  Google Scholar 

  24. Kesornprom, S., Pholasa, N., Cholamjiak, P.: On the convergence analysis of the gradient-CQ algorithms for the split feasibility problem. Numer. Algorithms 84, 997–1017 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  25. Korpelevich, G.M.: The extragradient method for finding saddle points and other problems. Ekon. Mate. Metody 12, 747–756 (1976)

    MathSciNet  MATH  Google Scholar 

  26. López, G., Martin, V., Wang, F., Xu, H.K.: Solving the split feasibility problem without prior knowledge of matrix norms. Inverse Probl. 28, 085004 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  27. Osilike, M.O., Aniagbosor, S.C.: Weak and strong convergence theorems for fixed points of asymptotically nonexpansive mappings. Math. Comput. Model. 32, 1181–1191 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  28. Qu, B., Xiu, N.: A note on the CQ algorithm for the split feasibility problem. Inverse Probl. 21, 1655–1665 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  29. Sahu, D.R., Cho, Y.J., Dong, Q.L., Kashyap, M.R., Li, X.H.: Inertial relaxed CQ algorithms for solving a split feasibility problem in Hilbert spaces. Numer. Algorithms (2020). https://doi.org/10.1007/s11075-020-00999-2

    Article  MATH  Google Scholar 

  30. Shehu, Y., Gibali, A.: New inertial relaxed method for solving split feasibilities. Optim. Lett. (2020). https://doi.org/10.1007/s11590-020-01603-1

    Article  MATH  Google Scholar 

  31. Suantai, S., Pholasa, N., Cholamjiak, P.: The modified inertial relaxed CQ algorithm for solving the split feasibility problems. J. Ind. Manag. Optim. 23, 1595–1615 (2018)

    Article  MathSciNet  Google Scholar 

  32. Suantai, S., Kesornprom, S., Cholamjiak, P.: A new hybrid CQ algorithm for the split feasibility problem in Hilbert spaces and its applications to compressed sensing. Mathematics 7, 789 (2019)

    Article  Google Scholar 

  33. Suantai, S., Witthayarat, U., Shehu, Y., Cholamjiak, P.: Iterative methods for the split feasibility problem and the fixed point problem in Banach spaces. Optimization 68, 955–980 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  34. Tibshirani, R.: Regression shrinkage and selection via the Lasso. J. R. Stat. Soc. Ser B 58, 267–288 (1996)

    MathSciNet  MATH  Google Scholar 

  35. Vinh, N., Cholamjiak, P., Suantai, S.: A new CQ algorithm for solving split feasibility problems in Hilbert spaces. Bull. Malays. Math. Sci. Soc. 42, 2517–2534 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  36. Wang, F.: On the convergence of CQ algorithm with variable steps for the split equality problem. Numer. Algorithms 74, 927–935 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  37. Xu, H.K.: Iterative methods for solving the split feasibility in infinite-dimensional Hilbert spaces. Inverse Probl. 26, 105018 (2010)

    Article  MATH  Google Scholar 

  38. Yang, Q.: On variable-step relaxed projection algorithm for variational inequalities. J. Math. Anal. Appl. 302, 166–179 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  39. Yen, L.H., Huyen, N.T.T., Muu, L.D.: A subgradient algorithm for a class of nonlinear split feasibility problems: application to jointly constrained Nash equilibrium models. J. Global Optim. 73, 849–868 (2019)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Funding

The second author was supported by National Natural Science Foundation of China (Grant No. 11801430).

Author information

Authors and Affiliations

  1. Xidian University, Xi’an, 710126, Shaanxi, China

    Xiaojun Ma & Hongwei Liu

Authors
  1. Xiaojun Ma
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hongwei Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xiaojun Ma.

Ethics declarations

Conflicts of interest

There are no conflicts of interest to this work.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ma, X., Liu, H. An inertial Halpern-type CQ algorithm for solving split feasibility problems in Hilbert spaces. J. Appl. Math. Comput. 68, 1699–1717 (2022). https://doi.org/10.1007/s12190-021-01585-y

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-021-01585-y

Keywords

Mathematics Subject Classification

Search

Navigation