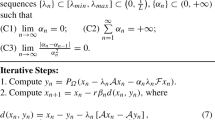Skip to main content
SpringerLink
Log in

New Bregman projection methods for solving pseudo-monotone variational inequality problem

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

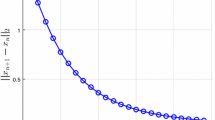
In this work, we introduce two Bregman projection algorithms with self-adaptive stepsize for solving pseudo-monotone variational inequality problem in a Hilbert space. The weak and strong convergence theorems are established without the prior knowledge of Lipschitz constant of the cost operator. The convergence behavior of the proposed algorithms with various functions of the Bregman distance are presented. More so, the performance and efficiency of our methods are compared to other related methods in the literature.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Anh, P.K., Thong, D.V., Vinh, N.T.: Improved inertial extragradient methods for solving pseudomonotone variational inequalities. Optimization (2020). https://doi.org/10.1080/02331934.2020.1808644

    Article  Google Scholar 

  2. Antipin, A.S.: On a method for convex programs using a symmetrical modification of the Lagrange function. Ekon. Mat. Metody 12, 1164–1173 (1976)

    Google Scholar 

  3. Baiocchi, C., Capelo, A.: Variational and Quasi-variational Inequalities. Wiley, New York (1984)

    MATH  Google Scholar 

  4. Barbu, V., Precupanu, T.: Convexity and Optimization in Banach Spaces. Springer, Dordrecht (2010)

    MATH  Google Scholar 

  5. Bnouhachem, A., Noor, M.A., Hao, Z.: Some new extragradient iterative methods for variational inequalities. Nonlinear Anal. 70, 1321–1329 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  6. Beck, A.: First-Order Methods in Optimization. Society for Industrial and Applied Mathematics, Philadelphia (2017)

    Book  MATH  Google Scholar 

  7. Bot, R.I., Csetnek, E.R., Vuong, P.T.: The forward–backward–forward method from continuous and discrete perspective for pseudo-monotone variational inequalities in Hilbert spaces. Eur. J. Oper. Res. 287, 49–60 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  8. Bregman, L.M.: The relaxation method of finding the common point of convex sets and its application to the solution of problems in convex programming. USSR Comput. Math. Math. Phys. 7, 200–217 (1967)

    Article  MathSciNet  MATH  Google Scholar 

  9. Butnariu, D., Resmerita, E.: Bregman distances, totally convex functions and a method for solving operator equations in Banach spaces. Abstr. Appl. Anal. 2006, pp. 1–39 (Art. ID 84919) (2006)

  10. Censor, Y., Gibali, A., Reich, S.: The subgradient extragradient method for solving variational inequalities in Hilbert space. J. Optim. Theory Appl. 148, 318–335 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  11. Cottle, R.W., Yao, J.C.: Pseudo-monotone complementarity problems in Hilbert space. J. Optim. Theory Appl. 75, 281–295 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  12. Dafermos, S.: Exchange price equilibria and variational inequalities. Math. Program. 46, 391–402 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  13. Gibali, A.: A new Bregman projection method for solving variational inequalities in Hilbert spaces. Pure Appl. Funct. Anal. 3, 403–415 (2018)

    MathSciNet  MATH  Google Scholar 

  14. Hieu, D.V., Cholamjiak, P.: Modified extragradient method with Bregman distance for variational inequalities. Appl. Anal. (2020). https://doi.org/10.1080/00036811.2020.1757078

    Article  MATH  Google Scholar 

  15. Hieu, D.V., Reich, S.: Two Bregman projection methods for solving variational inequalities. Optimization (2020). https://doi.org/10.1080/02331934.2020.1836634

    Article  Google Scholar 

  16. Hieu, D.V., Anh, P.K., Muu, L.D.: Modified hybrid projection methods for finding common solutions to variational inequality problems. Comput. Optim. Appl. 66, 75–96 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  17. Iusem, A.N., Svaiter, B.F.: A variant of Korpelevich’s method for variational inequalities with a new search strategy. Optimization 42, 309–321 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  18. Jolaoso, L.O., Aphane, M.: Weak and strong convergence Bregman extragradient schemes for solving pseudo-monotone and non-Lipschitz variational inequalities. J. Inequ. Appl. 2020, 195 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  19. Jolaoso, L.O., Aphane, M., Khan, S.H.: Two Bregman projection methods for solving variational inequality problems in Hilbert spaces with applications to signal processing. Symmetry 2020, 12 (2007). https://doi.org/10.3390/sym12122007

    Article  Google Scholar 

  20. Khanh, P.D., Vuong, P.T.: Modified projection method for strongly pseudo-monotone variational inequalities. J. Glob. Optim. 58, 341–350 (2014)

    Article  MATH  Google Scholar 

  21. Khanh, P.Q., Thong, D.V., Vinh, N.T.: Versions of the subgradient extragradient method for pseudomonotone variational inequalities. Acta Appl. Math. 170, 319–345 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  22. Kikuchi, N., Oden, J.T.: Contact Problems in Elasticity. SIAM, Philadelphia (1988)

    Book  MATH  Google Scholar 

  23. Kinderlehrer, D., Stampacchia, G.: An Introduction to Variational Inequalities and Their Applications. Academic Press, New York (1980)

    MATH  Google Scholar 

  24. Korpelevich, G.M.: The extragradient method for finding saddle points and other problems. Matecon 12, 747–756 (1976)

    MathSciNet  MATH  Google Scholar 

  25. Liu, L., Qin, X.: Strong convergence of an extragradient-like algorithm involving pseudo-monotone mappings. Numer. Algorithms 83, 1577–1590 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  26. Nakajo, K., Takahashi, W.: Strong convergence theorems for nonexpansive mappings and nonexpansive semigroups. J. Math. Anal. Appl. 279, 372–379 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  27. Noor, M.A.: New approximation schemes for general variational inequalities. J. Math. Anal. Appl. 251, 217–229 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  28. Noor, M.A.: Projection–splitting algorithms for general mixed variational inequalities. J. Comput. Anal. Appl. 4, 47–61 (2002)

    MathSciNet  MATH  Google Scholar 

  29. Noor, M.A.: Variational Inequalities and Applications, Lecture Notes. Mathematics Department, COMSATS Institute of Information Technology, Islamabad (2007)

    Google Scholar 

  30. Noor, M.A., Noor, K.I.: Self-adaptive projection algorithms for general variational inequalities. Appl. Math. Comput. 151, 659–670 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  31. Noor, M.A., Noor, K.I., Rassias, T.M.: Some aspects of variational inequalities. J. Comput. Appl. Math. 47, 285–312 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  32. Noor, M.A., Huang, Z.: Wiener–Hopf equation technique for variational inequalities and nonexpansive mappings. Appl. Math. Comput. 191, 504–510 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  33. Popov, L.D.: A modification of the Arrow–Hurwicz method for searching for saddle points. Mat. Zametki 28, 777–784 (1980)

    MathSciNet  MATH  Google Scholar 

  34. Reich, S., Sabach, S.: A strong convergence theorem for a proximal-type algorithm in reflexive Banach spaces. J. Nonlinear Convex Anal. 10, 471–485 (2009)

    MathSciNet  MATH  Google Scholar 

  35. Reich, S., Thong, D.V., Dong, Q.L., Xiao, H.L., Dung, V.T.: New algorithms and convergence theorems for solving variational inequalities with non-Lipschitz mappings. Numer. Algorithms (2020). https://doi.org/10.1007/s11075-020-00977-8

    Article  MATH  Google Scholar 

  36. Stampacchia, G.: Formes bilinéaires coercitives sur les ensembles convexes. C. R. Acad. Sci. Paris 258, 4413–4416 (1964)

    MathSciNet  MATH  Google Scholar 

  37. Thong, D.V., Gibali, A.: Two strong convergence subgradient extragradient methods for solving variational inequalities in Hilbert spaces. Jpn. J. Ind. Appl. Math. 36, 299–321 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  38. Thong, D.V., Hieu, D.V.: Weak and strong convergence theorems for variational inequality problems. Numer. Algorithms 78, 1045–1060 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  39. Thong, D.V., Vuong, P.T.: Modified Tseng’s extragradient methods for solving pseudo-monotone variational inequalities. Optimization 68, 2207–2226 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  40. Thong, D.V., Shehu, Y., Iyiola, O.S., Thang, H.V.: New hybrid projection methods for variational inequalities involving pseudomonotone mappings. Optim. Eng. 22, 363–386 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  41. Thong, D.V., Yang, J., Cho, Y.J., Rassias, T.M.: Explicit extragradient-like method with adaptive stepsizes for pseudomonotone variational inequalities. Optim. Lett. (2021). https://doi.org/10.1007/s11590-020-01678-w

    Article  MathSciNet  MATH  Google Scholar 

  42. Thong, D.V., Vinh, N.T., Hieu, D.V.: Accelerated hybrid and shrinking projection methods for variational inequality problems. Optimization 68, 981–998 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  43. Tseng, P.: A modified forward–backward splitting method for maximal monotone mapping. SIAM J. Control. Optim. 38, 431–446 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  44. Yang, J., Liu, H.: Strong convergence result for solving monotone variational inequalities in Hilbert space. Numer. Algorithms 80, 741–752 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  45. Yang, J., Liu, H., Li, G.: Convergence of a subgradient extragradient algorithm for solving monotone variational inequalities. Numer. Algorithms 84, 389–405 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  46. Yang, J., Liu, H., Liu, Z.: Modified subgradient extragradient algorithms for solving monotone variational inequalities. Optimization 67, 2247–2258 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  47. Yao, Y., Noor, M.A.: On viscosity iterative methods for variational inequalities. J. Math. Anal. Appl. 325, 776–787 (2007)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Funding

P. Cholamjiak thanks School of Science, University of Phayao and Thailand Science Research and Innovation (IRN62W0007).

Author information

Authors and Affiliations

  1. Department of Mathematics and Computer Science, Faculty of Science and Technology, Rajamangala University of Technology Thanyaburi (RMUTT), Thanyaburi, Pathumthani, 12110, Thailand

    Pongsakorn Sunthrayuth

  2. Department of Mathematics and Applied Mathematics, Sefako Makgatho Health Sciences University, Pretoria, South Africa

    Lateef Olakunle Jolaoso

  3. School of Science, University of Phayao, Mae Ka, Phayao, 56000, Thailand

    Prasit Cholamjiak

Authors
  1. Pongsakorn Sunthrayuth
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Lateef Olakunle Jolaoso
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Prasit Cholamjiak
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Prasit Cholamjiak.

Ethics declarations

Conflict of interest

There is no conflicts of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sunthrayuth, P., Jolaoso, L.O. & Cholamjiak, P. New Bregman projection methods for solving pseudo-monotone variational inequality problem. J. Appl. Math. Comput. 68, 1565–1589 (2022). https://doi.org/10.1007/s12190-021-01581-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-021-01581-2

Keywords

Mathematics Subject Classification

Search

Navigation