Skip to main content
SpringerLink
Log in

An Efficient Numerical Algorithm for Constructing the Wigner Function of a Quantum System with a Polynomial Potential in Phase Space

  • Published:
Physics of Particles and Nuclei Aims and scope Submit manuscript

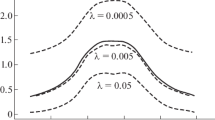
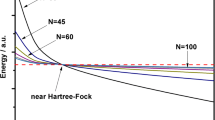
Abstract

When considering quantum systems in phase space, the Wigner function is used as a function of quasi-probability density. Finding the Wigner function is related to the calculation of a Fourier transform of a certain composition of wave functions of the corresponding quantum system. As a rule, knowledge of the Wigner function is not the final goal, and the calculation of mean values of different quantum characteristics of the system is required. An explicit solution of the Schrödinger equation can be obtained only for a narrow class of potentials, therefore, in the majority of cases, numerical methods must be used to find wave functions. As a result, finding the Wigner function is related to the numerical integration of grid wave functions. When considering a one-dimensional system, the calculation of N2 Fourier integrals of the grid wave function is needed. To provide the necessary accuracy for wave functions, corresponding to the higher states of a quantum system, a larger number of grid nodes is needed. The goal of this work was to construct the numerical-analytical method for finding the Wigner function, which allows the number of computational operations to be considerably reduced. The quantum systems with polynomial potentials were considered, for which the Wigner function is represented as a series in some known functions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1.
Fig. 2.
Fig. 3.
Fig. 4.
Fig. 5.
Fig. 6.
Fig. 7.
Fig. 8.
Fig. 9.
Fig. 10.
Fig. 11.
Fig. 12.
Fig. 13.
Fig. 14.

Similar content being viewed by others

REFERENCES

  1. E. P. Wigner, “On the quantum correction for thermodynamic equilibrium,” Phys. Rev. 40, 749–759 (1932).

    Article  ADS  MATH  Google Scholar 

  2. H. Weyl, The Theory of Groups and Quantum Mechanics (Dover, New York, 1931).

    MATH  Google Scholar 

  3. Causality Problems in Quantum Mechanics. Collection of Translations, Ed. by Ya. P. Terletsky and A. A. Gusev (IL, Moscow, 1955).

    Google Scholar 

  4. N. L. Balazs and B. K. Jennings, “Wigner’s functions and other distribution functions in mock phase spaces,” Phys. Rep. 104, 347–391 (1984).

    Article  ADS  MathSciNet  Google Scholar 

  5. M. Hillery, R. F. O’Connell, M. O. Scully, and E. P. Wigner, “Distribution functions in physics: Fundamentals,” Phys. Rep. 106, 121–167 (1984).

    Article  ADS  MathSciNet  Google Scholar 

  6. B.-G. Englert, “On the operator bases underlying Wigner’s, Kirkwood’s and Glauber’s phase space functions,” J. Phys. A 22, 625–640 (1989).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  7. I. Bialynicki-Birula, M. Cieplak, and J. Kaminski, Theory of Quanta (Oxford University Press, 1992).

    Google Scholar 

  8. A. M. Ozorio de Almeida, “The Weyl representation in classical and quantum mechanics,” Phys. Rep. 295, 265–342 (1998).

    Article  ADS  MathSciNet  Google Scholar 

  9. E. Scheibe, Die Reduktion physikalischer Theorien, Teil II (Springer, Heidelberg, 1999).

  10. Y. Kano, “A new phase-space distribution function in the statistical theory of the electromagnetic field,” J. Math. Phys. 6, 1913–1915 (1965).

    Article  ADS  MathSciNet  Google Scholar 

  11. R. J. Glauber, “Photon correlations,” Phys. Rev. Lett. 10, 84–86 (1963).

    Article  ADS  MathSciNet  Google Scholar 

  12. E. C. G. Sudarshan, “Equivalence of semiclassical and quantum mechanical descriptions of statistical light beams,” Phys. Rev. Lett. 10, 277–279 (1963).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  13. K. E. Cahill and R. J. Glauber, “Density operators and quasiprobability distributions,” Phys. Rev. A 177, 1882–1902 (1969).

    Article  ADS  Google Scholar 

  14. H. J. Groenewold, “On the principles of elementary quantum mechanics,” Physica 12, 405–460 (1946).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  15. G. S. Agarwal and E. Wolf, “Calculus for functions of noncommuting operators and general phase-space methods in quantum mechanics. II. Quantum mechanics in phase space,” Phys. Rev. D 2, 2187–2205 (1970).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  16. Valentino A. Simpao, “Real wavefunction from generalised Hamiltonian Schrodinger equation in quantum phase space via HOA (Heaviside Operational Ansatz): Exact analytical results,” J. Math. Chem. 52, 1137–1155 (2014).

    Article  MathSciNet  MATH  Google Scholar 

  17. D. B. Fairliet and C. A. Manoguei, “The formulation of quantum mechanics in terms of phase space functions—the third equation,” J. Phys. A: Math. Gen. 24, 3807–3815 (1991).

    Article  ADS  MathSciNet  Google Scholar 

  18. K. B. Møller, T. G. Jørgensen, and G. Torres-Vega, “On coherent-state representations of quantum mechanics: Wave mechanics in phase space,” J. Chem. Phys. 106, 7228–7240 (1997).

    Article  ADS  Google Scholar 

  19. D. T. Smithey, M. Beck, M. G. Raymer, and A. Faridani, “Measurement of the Wigner distribution and the density matrix of a light mode using optical homodyne tomography: Application to squeezed states and the vacuum,” Phys. Rev. Lett. 70, 1244–1247 (1993).

    Article  ADS  Google Scholar 

  20. J. Radon, “Fiber die Bestimmung von Funktionen durch ihre Integralwerte Langs gewisser Mannigfaltigkeiten,” Ber. Verh. Sachs. Akad. Wiss. Leipzig, Math.-Nat. Kl. 69, 262–277 (1917).

    Google Scholar 

  21. G. M. D’Ariano, M. G. A. Paris, and M.F. Sacchi, “Quantum tomography,” in Advances in Imaging and Electron Physics, Ed. by P.W. Hawkes (Elsevier, 2003), Vol. 128, pp. 205–308.

    Google Scholar 

  22. K. Vogel and H. Risken, “Determination of quasiprobability distributions in terms of probability distributions for the rotated quadrature phase,” Phys. Rev. A 40, 2847–2849 (1989).

    Article  ADS  Google Scholar 

  23. A. Casado, S. Guerra, and J. Plácido, “From stochastic optics to the Wigner formalism: The role of the vacuum field in optical quantum communication experiments,” Atoms 7, 76 (2019).

    Article  ADS  Google Scholar 

  24. A. Casado, S. Guerra, and J. Plácido, “Wigner representation for experiments on quantum cryptography using two-photon polarization entanglement produced in parametric down-conversion,” J. Phys. B 41, 045501 (2008).

    Article  ADS  Google Scholar 

  25. R. P. Rundle, T. Tilma, J. H. Samson, V. M. Dwyer, R.F. Bishop, and M. J. Everitt, “General approach to quantum mechanics as a statistical theory,” Phys. Rev. A 99, 012115 (2019).

    Article  ADS  Google Scholar 

  26. I. I. Arkhipov, A. Barasiński, and J. Svozilík, “Negativity volume of the generalized Wigner function as an entanglement witness for hybrid bipartite states,” Sci. Rep. 8, 16955 (2018).

    Article  ADS  Google Scholar 

  27. U. Andersen, J. Neergaard-Nielsen, P. van Loock, et al., “Hybrid discrete- and continuous-variable quantum information,” Nature Physics 11, 713–719 (2015).

    Article  ADS  Google Scholar 

  28. L. Cohen, Time-Frequency Analysis (Prentice Hall, Englewood Cliffs, 1995).

    Google Scholar 

  29. A. Zayed, “A new perspective on the two-dimensional fractional Fourier transform and its relationship with the Wigner distribution,” J. Fourier. Anal. Appl. 25, 460–487 (2019).

    Article  MathSciNet  MATH  Google Scholar 

  30. T. A. C. M. Claasen and W. F. G. Mecklenbräuker, “The Wigner distribution—a tool for time-frequency signal analysis. II: Discrete-time signals, part 2,” Philips J. Res. 35, 276–300 (1980).

    MathSciNet  MATH  Google Scholar 

  31. D. Brandon, N. Saad, and Shi-Hai Dong, “On some polynomial potentials in d-dimensions,” J. Math. Phys. 54, 082106 (2013).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  32. F. J. Gomez and J. Sesma, “Quantum anharmonic oscillators: A new approach,” J. Phys. A 38, 3193–3202 (2005).

    Article  MathSciNet  MATH  Google Scholar 

  33. F. Pan, J. R. Klauder, and J. P. Draayer, “Quasi-exactly solvable cases of an N-dimensional symmetric decatic anharmonic oscillator,” Phys. Lett. A 262, 131 (1999).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  34. M. Bansal, S. Srivastava, and Vishwamittar, “Energy eigenvalues of double-well oscillator with mixed quartic and sextic anharmonicities,” Phys. Rev. A 44, 8012 (1991).

    Article  ADS  Google Scholar 

  35. R. N. Chaudhuri and M. Mondal, “Improved Hill determinant method: General approach to the solution of quantum anharmonic oscillators,” Phys. Rev. A 43, 3241 (1991).

    Article  ADS  MathSciNet  Google Scholar 

  36. R. L. Hall and N. Saad, “Bounds on Schrodinger eigenvalues for polynomial potentials in N-dimensions,” J. Math. Phys. 38, 4909 (1997).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  37. M. Vishwarmittar, “Energy eigenvalues for anharmonic and double-well oscillators with even power polynomial potential,” Physica A 216, 452–458 (1995).

    Article  ADS  Google Scholar 

  38. E. Z. Liverts and V. B. Mandelzweig, “Approximate analytic solutions of the Schrodinger equation for the generalized anharmonic oscillator,” Phys. Scr. 77, 025003 (2008).

    Article  MATH  Google Scholar 

  39. E. E. Perepelkin, B. I. Sadovnikov, N. G. Inozemtseva, and E. V. Burlakov, “Wigner function of a quantum system with polynomial potential,” J. Stat. Mech. Theory Exp. 2020. 053105 (2020).

    Article  MathSciNet  MATH  Google Scholar 

  40. B. V. Numerov, “Note on the numerical integration of \({{{{d}^{2}}x} \mathord{\left/ {\vphantom {{{{d}^{2}}x} {d{{t}^{2}}}}} \right. \kern-0em} {d{{t}^{2}}}} = f\left( {x,t} \right)\),” Astronomische Nachrichten 230, 359–364 (1927).

    Article  ADS  Google Scholar 

  41. E. E. Perepelkin, B. I. Sadovnikov, N. G. Inozemtseva, and E. V. Burlakov, “Explicit form for the kernel operator matrix elements in eigenfunction basis of harmonic oscillator,” J. Stat. Mech. Theory Exp. 2020, 023109 (2020).

    Article  MathSciNet  MATH  Google Scholar 

  42. B. I. Sadovnikov, N. G. Inozemtseva, and E. E. Perepelkin, “Generalized phase space and conservative systems,” Doklady Mathematics 88, 457–459 (2013).

    Article  MathSciNet  MATH  Google Scholar 

  43. E. E. Perepelkin, B. I. Sadovnikov, and N. G. Inozemtseva, Generalized Phase Space (Faculty of Physics, MSU, Moscow, 2014), 164 p.

  44. B. I. Sadovnikov, E. E. Perepelkin, and N. G. Inozemtseva, “Coordinate uncertainty principle in a generalized phase space,” Doklady Mathematics 90, 628–630 (2014).

    Article  MathSciNet  MATH  Google Scholar 

  45. E. E. Perepelkin, B. I. Sadovnikov, and N. G. Inozemtseva, “The quantum mechanics of high-order kinematic values,” Ann. Phys. 401, 59–90 (2019).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  46. E. E. Perepelkin, B. I. Sadovnikov, and N. G. Inozemtseva, “The new modified Vlasov equation for the systems with dissipative processes,” J. Stat. Mech. Theory Exp. 2017, 053207 (2017).

    Article  MathSciNet  MATH  Google Scholar 

  47. A. A. Vlasov, The Theory of Many Particles (URSS, Moscow, 2011) [In Russian].

    Google Scholar 

  48. A. A. Vlasov, Statistical Distribution Functions (Nauka, Moscow, 1966) [In Russian].

    Google Scholar 

  49. E. E. Perepelkin, B. I. Sadovnikov, and N.G. Inozemtseva, “The properties of the first equation of the Vlasov chain of equations,” J. Stat. Mech. Theory Exp. 2015, 05019 (2015).

    Article  MathSciNet  MATH  Google Scholar 

  50. E. E. Perepelkin, B. I. Sadovnikov, and N. G. Inozemtseva, “Paradigm of Infinite Dimensional Phase Space,” in Understanding the Schrödinger Equation: Some [Non]Linear Perspectives (Nova Science Publishers, Inc., United States, 2020), pp. 248–288.

  51. D. Bohm, “A suggested interpretation of the quantum theory in terms of “hidden” variables I and II," Phys. Rev. 85, 166–193 (1952).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  52. D. Bohm, B. J. Hiley, and P. N. Kaloyerou, “An ontological basis for the quantum theory,” Phys. Rep. 144, 321–375 (1987).

    Article  ADS  MathSciNet  Google Scholar 

  53. D. Bohm and B. J. Hiley, The Undivided Universe: An Ontological Interpretation of Quantum Theory (Routledge, London, 1993).

    Google Scholar 

  54. L. de Broglie, Une Interprétation Causale et non Linéaire de la Mécanique Ondulatoire: La Théorie de la Double Solution (Gauthiers-Villiars, Paris, 1956).

    MATH  Google Scholar 

  55. W. P. Schleich, Quantum Optics in Phase Space (Wiley-VCH, Berlin, 2001) 718 p.

    Book  MATH  Google Scholar 

  56. Hudson R.L. “When is the Wigner quasi-probability density non-negative?,” Rep. Math. Phys. 6, 240–252 (1974).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  57. K. Husimi, “Some formal properties of the density matrix,” Proc. Phys. Math. Soc. Jpn. 22, 264–314 (1940).

    MATH  Google Scholar 

  58. Y. Kano, “A new phase-space distribution function in the statistical theory of the electromagnetic field,” J. Math. Phys. 6, 1913–1915 (1965).

    Article  ADS  MathSciNet  Google Scholar 

  59. R. J. Glauber, “Photon correlations,” Phys. Rev. Lett. 10, 84–86 (1963).

    Article  ADS  MathSciNet  Google Scholar 

  60. E. C. G. Sudarshan, “Equivalence of semiclassical and quantum mechanical descriptions of statistical light beams,” Phys. Rev. Lett. 10, 277–279 (1963).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  61. K. E. Cahill and R. J. Glauber, “Density operators and quasiprobability distributions,” Phys. Rev. A 177, 1882–1902 (1969).

    Article  ADS  Google Scholar 

  62. Go. Torres-Vega and J. H. Frederick, “A quantum mechanical representation in phase space,” J. Chem. Phys. 98, 3103–3120 (1993).

    Article  ADS  Google Scholar 

  63. Go. Torres-Vega and J. H. Frederick, “Quantum mechanics in phase space: New approaches to the correspondence principle,” J. Chem. Phys. 93, 8862–8874 (1990).

    Article  ADS  MathSciNet  Google Scholar 

  64. N. Wiener “Hermitian polynomials and Fourier analysis,” J. Math. Phys. 8, 70–73 (1929).

    Article  MATH  Google Scholar 

  65. W. Koepf, “Identities for families of orthogonal polynomials and special functions,” Integral Transforms and Special Functions 5, 69–102 (1997).

    Article  MathSciNet  MATH  Google Scholar 

  66. W. A. Al-Salam, “Operational representations for Laguerre and other polynomials,” Duke Math. J. 31, 127–142 (1964).

    Article  MathSciNet  MATH  Google Scholar 

  67. E. E. Perepelkin, B. I. Sadovnikov, and N. G. Inozemtseva, “Riemann surface and quantization,” arXiv: 1606.02013v1 (2016).

  68. A. N. Kolmogorov and S. V. Fomin, Elements of the Theory of Functions and Functional Analysis (Nauka, Moscow, 1976; Dover Publ., 1999).

Download references

Funding

This work was supported by the Russian Foundation for Basic Research (grant No. 18-29-10014).

This research has been supported by the Interdisciplinary Scientific and Educational School of Moscow University “Photonic and Quantum Technologies. Digital Medicine.”

Author information

Authors and Affiliations

  1. Joint Institute for Nuclear Research, 141980, Dubna, Moscow oblast, Russia

    E. E. Perepelkin & R. V. Polyakova

  2. Faculty of Physics, Moscow State University, 119991, Moscow, Russia

    E. E. Perepelkin, B. I. Sadovnikov & E. V. Burlakov

  3. Dubna University, 141982, Dubna, Moscow oblast, Russia

    E. E. Perepelkin & N. G. Inozemtseva

  4. Moscow Technical University of Communication and Informatics, 111024, Moscow, Russia

    E. E. Perepelkin, N. G. Inozemtseva & E. V. Burlakov

Authors
  1. E. E. Perepelkin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. B. I. Sadovnikov
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. N. G. Inozemtseva
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. E. V. Burlakov
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. R. V. Polyakova
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to E. E. Perepelkin or R. V. Polyakova.

Additional information

Translated by M. Samokhina

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Perepelkin, E.E., Sadovnikov, B.I., Inozemtseva, N.G. et al. An Efficient Numerical Algorithm for Constructing the Wigner Function of a Quantum System with a Polynomial Potential in Phase Space. Phys. Part. Nuclei 52, 438–476 (2021). https://doi.org/10.1134/S1063779621030072

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1063779621030072

Keywords:

Search

Navigation