Skip to main content
SpringerLink
Log in

Provably physical-constraint-preserving discontinuous Galerkin methods for multidimensional relativistic MHD equations

  • Published:
Numerische Mathematik Aims and scope Submit manuscript

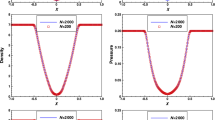
Abstract

We propose and analyze a class of robust, uniformly high-order accurate discontinuous Galerkin (DG) schemes for multidimensional relativistic magnetohydrodynamics (RMHD) on general meshes. A distinct feature of the schemes is their physical-constraint-preserving (PCP) property, i.e., they are proven to preserve the subluminal constraint on the fluid velocity and the positivity of density, pressure, and internal energy. This is the first time that provably PCP high-order schemes are achieved for multidimensional RMHD. Developing PCP high-order schemes for RMHD is highly desirable but remains a challenging task, especially in the multidimensional cases, due to the inherent strong nonlinearity in the constraints and the effect of the magnetic divergence-free condition. Inspired by some crucial observations at the PDE level, we construct the provably PCP schemes by using the locally divergence-free DG schemes of the recently proposed symmetrizable RMHD equations as the base schemes, a limiting technique to enforce the PCP property of the DG solutions, and the strong-stability-preserving methods for time discretization. We rigorously prove the PCP property by using a novel “quasi-linearization” approach to handle the highly nonlinear physical constraints, technical splitting to offset the influence of divergence error, and sophisticated estimates to analyze the beneficial effect of the additional source term in the symmetrizable RMHD system. Several two-dimensional numerical examples are provided to further confirm the PCP property and to demonstrate the accuracy, effectiveness and robustness of the proposed PCP schemes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Notes

  1. The PCP property defined in this paper refers only to the preservation of the subluminal constraint on fluid velocity and the positivity of density, pressure, and internal energy. Our PCP definition does not include the divergence-free constraint (4). The numerical schemes proposed in this paper only maintain a locally divergence-free property for the magnetic field.

References

  1. Balsara, D.S.: Second-order-accurate schemes for magnetohydrodynamics with divergence-free reconstruction. Astrophys. J. Suppl. Ser. 151, 149–184 (2004)

    Article  Google Scholar 

  2. Balsara, D.S., Kim, J.: A subluminal relativistic magnetohydrodynamics scheme with ADER-WENO predictor and multidimensional Riemann solver-based corrector. J. Comput. Phys. 312, 357–384 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  3. Balsara, D.S., Spicer, D.: A staggered mesh algorithm using high order Godunov fluxes to ensure solenoidal magnetic fields in magnetohydrodynamic simulations. J. Comput. Phys. 149, 270–292 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  4. Chandrashekar, P.: A global divergence conforming DG method for hyperbolic conservation laws with divergence constraint. J. Sci. Comput. 79(1), 79–102 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  5. Christlieb, A.J., Liu, Y., Tang, Q., Xu, Z.: Positivity-preserving finite difference weighted ENO schemes with constrained transport for ideal magnetohydrodynamic equations. SIAM J. Sci. Comput. 37(4), A1825–A1845 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  6. Cockburn, B., Hou, S., Shu, C.W.: The Runge–Kutta local projection discontinuous Galerkin finite element method for conservation laws. IV. The multidimensional case. Math. Comput. 54(190), 545–581 (1990)

    MathSciNet  MATH  Google Scholar 

  7. Cockburn, B., Li, F., Shu, C.W.: Locally divergence-free discontinuous Galerkin methods for the Maxwell equations. J. Comput. Phys. 194(2), 588–610 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  8. Cockburn, B., Shu, C.W.: TVB Runge–Kutta local projection discontinuous Galerkin finite element method for conservation laws. II. General framework. Math. Comput. 52(186), 411–435 (1989)

    MathSciNet  MATH  Google Scholar 

  9. Dedner, A., Kemm, F., Kröner, D., Munz, C.D., Schnitzer, T., Wesenberg, M.: Hyperbolic divergence cleaning for the MHD equations. J. Comput. Phys. 175(2), 645–673 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  10. Del Zanna, L., Zanotti, O., Bucciantini, N., Londrillo, P.: ECHO: a Eulerian conservative high-order scheme for general relativistic magnetohydrodynamics and magnetodynamics. Astron. Astrophys. 473(1), 11–30 (2007)

    Article  Google Scholar 

  11. Du, J., Shu, C.W.: Positivity-preserving high-order schemes for conservation laws on arbitrarily distributed point clouds with a simple WENO limiter. Int. J. Numer. Anal. Model. 15, 1–25 (2018)

    MathSciNet  MATH  Google Scholar 

  12. Evans, C.R., Hawley, J.F.: Simulation of magnetohydrodynamic flows: a constrained transport method. Astrophys. J. 332, 659–677 (1988)

    Article  Google Scholar 

  13. Fu, P., Li, F., Xu, Y.: Globally divergence-free discontinuous Galerkin methods for ideal magnetohydrodynamic equations. J. Sci. Comput. 77(3), 1621–1659 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  14. Godunov, S.K.: Symmetric form of the equations of magnetohydrodynamics. Numer. Methods Mech. Contin. Medium 1, 26–34 (1972)

    Google Scholar 

  15. Gottlieb, S., Shu, C.W., Tadmor, E.: Strong stability-preserving high-order time discretization methods. SIAM Rev. 43(1), 89–112 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  16. Guermond, J.L., Nazarov, M., Popov, B., Tomas, I.: Second-order invariant domain preserving approximation of the Euler equations using convex limiting. SIAM J. Sci. Comput. 40(5), A3211–A3239 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  17. He, P., Tang, H.: An adaptive moving mesh method for two-dimensional relativistic magnetohydrodynamics. Comput. Fluids 60, 1–20 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  18. van der Holst, B., Keppens, R., Meliani, Z.: A multidimensional grid-adaptive relativistic magnetofluid code. Comput. Phys. Commun. 179(9), 617–627 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  19. Hu, X.Y., Adams, N.A., Shu, C.W.: Positivity-preserving method for high-order conservative schemes solving compressible Euler equations. J. Comput. Phys. 242, 169–180 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  20. Jiang, Y., Liu, H.: Invariant-region-preserving DG methods for multi-dimensional hyperbolic conservation law systems, with an application to compressible Euler equations. J. Comput. Phys. 373, 385–409 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  21. Komissarov, S.S.: A Godunov-type scheme for relativistic magnetohydrodynamics. Mon. Not. R. Astron. Soc. 303(2), 343–366 (1999)

    Article  Google Scholar 

  22. Krivodonova, L., Xin, J., Remacle, J.F., Chevaugeon, N., Flaherty, J.E.: Shock detection and limiting with discontinuous Galerkin methods for hyperbolic conservation laws. Appl. Numer. Math. 48(3–4), 323–338 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  23. Li, F., Shu, C.W.: Locally divergence-free discontinuous Galerkin methods for MHD equations. J. Sci. Comput. 22(1–3), 413–442 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  24. Li, F., Xu, L., Yakovlev, S.: Central discontinuous Galerkin methods for ideal MHD equations with the exactly divergence-free magnetic field. J. Comput. Phys. 230(12), 4828–4847 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  25. Liang, C., Xu, Z.: Parametrized maximum principle preserving flux limiters for high order schemes solving multi-dimensional scalar hyperbolic conservation laws. J. Sci. Comput. 58(1), 41–60 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  26. Ling, D., Duan, J., Tang, H.: Physical-constraints-preserving Lagrangian finite volume schemes for one- and two-dimensional special relativistic hydrodynamics. J. Comput. Phys. 396, 507–543 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  27. Mignone, A., Bodo, G.: An HLLC riemann solver for relativistic flows—II. magnetohydrodynamics. Mon. Not. R. Astron. Soc. 368(3), 1040–1054 (2006)

    Article  Google Scholar 

  28. Mishra, S., Tadmor, E.: Constraint preserving schemes using potential-based fluxes. III. Genuinely multi-dimensional schemes for MHD equations. ESAIM: Math. Model. Numer. Anal. 46(3), 661–680 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  29. Powell, K.G.: An approximate Riemann solver for magnetohydrodynamics (that works in more than one dimension). Technical Report. ICASE Report No. 94-24, NASA Langley, VA (1994)

  30. Powell, K.G., Roe, P.L., Linde, T.J., Gombosi, T.I., Zeeuw, D.L.D.: A solution-adaptive upwind scheme for ideal magnetohydrodynamics. J. Comput. Phys. 154(2), 284–309 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  31. Qin, T., Shu, C.W., Yang, Y.: Bound-preserving discontinuous Galerkin methods for relativistic hydrodynamics. J. Comput. Phys. 315, 323–347 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  32. Qiu, J., Shu, C.W.: Runge–Kutta discontinuous Galerkin method using WENO limiters. SIAM J. Sci. Comput. 26(3), 907–929 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  33. Radice, D., Rezzolla, L., Galeazzi, F.: High-order fully general-relativistic hydrodynamics: new approaches and tests. Class. Quantum Gravity 31(7), 075012 (2014)

    Article  MATH  Google Scholar 

  34. Shu, C.W.: Bound-preserving high-order schemes for hyperbolic equations: survey and recent developments. In: Klingenberg, C., Westdickenberg, M. (eds.) Theory, Numerics and Applications of Hyperbolic Problems II, pp. 591–603. Springer, Cham (2018)

    Chapter  Google Scholar 

  35. Sun, Z., Shu, Cw.: Strong stability of explicit Runge–Kutta time discretizations. SIAM J. Numer. Anal. 57(3), 1158–1182 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  36. Tadmor, E.: A minimum entropy principle in the gas dynamics equations. Appl. Numer. Math. 2(3), 211–219 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  37. Torrilhon, M.: Locally divergence-preserving upwind finite volume schemes for magnetohydrodynamic equations. SIAM J. Sci. Comput. 26(4), 1166–1191 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  38. Tóth, G.: The \(\nabla \cdot {B} = 0\) constraint in shock-capturing magnetohydrodynamics codes. J. Comput. Phys. 161(2), 605–652 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  39. Wu, K.: Design of provably physical-constraint-preserving methods for general relativistic hydrodynamics. Phys. Rev. D 95(10), 103001 (2017)

    Article  MathSciNet  Google Scholar 

  40. Wu, K.: Positivity-preserving analysis of numerical schemes for ideal magnetohydrodynamics. SIAM J. Numer. Anal. 56(4), 2124–2147 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  41. Wu, K.: Minimum principle on specific entropy and high-order accurate invariant region preserving numerical methods for relativistic hydrodynamics. arXiv preprint arXiv:2102.03801 (2021)

  42. Wu, K., Shu, C.W.: A provably positive discontinuous Galerkin method for multidimensional ideal magnetohydrodynamics. SIAM J. Sci. Comput. 40(5), B1302–B1329 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  43. Wu, K., Shu, C.W.: Provably positive high-order schemes for ideal magnetohydrodynamics: analysis on general meshes. Numer. Math. 142(4), 995–1047 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  44. Wu, K., Shu, C.W.: Entropy symmetrization and high-order accurate entropy stable numerical schemes for relativistic MHD equations. SIAM J. Sci. Comput. 42(4), A2230–A2261 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  45. Wu, K., Tang, H.: High-order accurate physical-constraints-preserving finite difference WENO schemes for special relativistic hydrodynamics. J. Comput. Phys. 298, 539–564 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  46. Wu, K., Tang, H.: Admissible states and physical-constraints-preserving schemes for relativistic magnetohydrodynamic equations. Math. Models Methods Appl. Sci. 27(10), 1871–1928 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  47. Wu, K., Tang, H.: Physical-constraint-preserving central discontinuous Galerkin methods for special relativistic hydrodynamics with a general equation of state. Astrophys. J. Suppl. Ser. 228(1), 3 (2017)

    Article  MathSciNet  Google Scholar 

  48. Wu, K., Tang, H.: On physical-constraints-preserving schemes for special relativistic magnetohydrodynamics with a general equation of state. Z. Angew. Math. Phys. 69(3), 84 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  49. Xu, Z.: Parametrized maximum principle preserving flux limiters for high order schemes solving hyperbolic conservation laws: one-dimensional scalar problem. Math. Comput. 83(289), 2213–2238 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  50. Xu, Z., Liu, Y.: New central and central discontinuous Galerkin schemes on overlapping cells of unstructured grids for solving ideal magnetohydrodynamic equations with globally divergence-free magnetic field. J. Comput. Phys. 327, 203–224 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  51. Zanotti, O., Fambri, F., Dumbser, M.: Solving the relativistic magnetohydrodynamics equations with ADER discontinuous Galerkin methods, a posteriori subcell limiting and adaptive mesh refinement. Mon. Not. R. Astron. Soc. 452(3), 3010–3029 (2015)

    Article  Google Scholar 

  52. Zhang, X.: On positivity-preserving high order discontinuous Galerkin schemes for compressible Navier–Stokes equations. J. Comput. Phys. 328, 301–343 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  53. Zhang, X., Shu, C.W.: On maximum-principle-satisfying high order schemes for scalar conservation laws. J. Comput. Phys. 229(9), 3091–3120 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  54. Zhang, X., Shu, C.W.: On positivity-preserving high order discontinuous Galerkin schemes for compressible Euler equations on rectangular meshes. J. Comput. Phys. 229(23), 8918–8934 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  55. Zhang, X., Shu, C.W.: A minimum entropy principle of high order schemes for gas dynamics equations. Numer. Math. 121(3), 545–563 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  56. Zhang, X., Xia, Y., Shu, C.W.: Maximum-principle-satisfying and positivity-preserving high order discontinuous Galerkin schemes for conservation laws on triangular meshes. J. Sci. Comput. 50(1), 29–62 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  57. Zhao, J., Tang, H.: Runge–Kutta discontinuous Galerkin methods for the special relativistic magnetohydrodynamics. J. Comput. Phys. 343, 33–72 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  58. Zou, S., Yu, X., Dai, Z.: A positivity-preserving Lagrangian discontinuous Galerkin method for ideal magnetohydrodynamics equations in one-dimension. J. Comput. Phys. 405, 109144 (2020)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Southern University of Science and Technology, Shenzhen, 518055, Guangdong Province, People’s Republic of China

    Kailiang Wu

  2. Division of Applied Mathematics, Brown University, Providence, RI, 02912, USA

    Chi-Wang Shu

Authors
  1. Kailiang Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Chi-Wang Shu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Kailiang Wu.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Research of C.-W. Shu is supported in part by NSF grants DMS-1719410 and DMS-2010107, and AFOSR grant FA9550-20-1-0055.

Appendices

Proof of Proposition 1

Due to the assumption that the exact smooth solution exists for \({\varvec{x}} \in {{\mathbb {R}}}^d\) and \(0\le t \le T\), the Lorentz factor W does not blow up, and then \(|\mathbf{v }( {\varvec{x}}, t )|<1\) for \(\forall {\varvec{x}} \in {{\mathbb {R}}}^d\) and \(0\le t \le T\). For any \(\left( {\bar{\varvec{x}}}, {\bar{t}} \right) \in {\mathbb {R}}^d \times {\mathbb {R}}^+\), we denote by \({\varvec{x}}={\varvec{x}}(t;\bar{\varvec{x}},{\bar{t}})\) the integral curve of \( \frac{d {\varvec{x}}}{d t} = \mathbf{v } ({\varvec{x}},t) \) through the point \(\left( {\bar{\varvec{x}}}, {\bar{t}} \right) \). Define \({\varvec{x}}_0( \bar{\varvec{x}},{\bar{t}} ):= {\varvec{x}}(0;\bar{\varvec{x}},{\bar{t}})\). It can be observed that the curve passes through the point \(\left( {\varvec{x}}_0( \bar{\varvec{x}},{\bar{t}} ),0 \right) \) at the initial time \(t=0\). For strong solutions, we can reformulate the continuity equation of (10) for \(\rho W\) as \( \frac{ {\mathcal {D}}(\rho W) }{{\mathcal {D}}t} = - \rho W \nabla \cdot \mathbf{v }, \) where \(\frac{{\mathcal {D}}}{{\mathcal {D}}t}:= \frac{\partial }{\partial t} + \mathbf{v }({\varvec{x}},t) \nabla \cdot \) denotes the derivative along the integral curve. Integration of this reformulated continuity equation from \(t=0\) to \({\bar{t}}\) along the curve implies

$$\begin{aligned} \rho W ( {\bar{\varvec{x}}}, {\bar{t}} ) = \rho _0 W_0 ( {\varvec{x}}_0( \bar{\varvec{x}},{\bar{t}} ) ) \exp \left( - \int _{0}^{{\bar{t}}} \nabla \cdot \mathbf{v }( {\varvec{x}}(t;\bar{\varvec{x}},{\bar{t}}), t ) dt \right) > 0, \end{aligned}$$

which, along with \(W ( {\bar{\varvec{x}}}, {\bar{t}} ) \ge 1\), imply \(\rho ( {\bar{\varvec{x}}}, {\bar{t}} )>0\) for all \(\left( {\bar{\varvec{x}}}, {\bar{t}} \right) \in {\mathbb {R}}^3 \times {\mathbb {R}}^+\). For smooth solutions of the modified RMHD system (10), one can derive that

$$\begin{aligned} \frac{ {\mathcal {D}}\left( p \rho ^{-\varGamma } \right) }{{\mathcal {D}}t} = \frac{\partial }{\partial t} \left( p\rho ^{-\varGamma } \right) + \mathbf{v } \cdot \nabla \left( p\rho ^{-\varGamma } \right) = 0, \end{aligned}$$
(60)

which implies \( p \rho ^{-\varGamma } ( {\bar{\varvec{x}}}, {\bar{t}} ) = p_0 \rho ^{-\varGamma }_0 ( {\varvec{x}}_0( \bar{\varvec{x}},{\bar{t}} ) ) > 0. \) It follows that \(p ( {\bar{\varvec{x}}}, {\bar{t}} ) >0,~\forall \left( {\bar{\varvec{x}}}, {\bar{t}} \right) \in {\mathbb {R}}^3 \times {\mathbb {R}}^+\). Using the ideal EOS (3) with \(\varGamma \in (1,2]\) gives \(e ({\bar{\varvec{x}}}, {\bar{t}}) = \frac{1}{\varGamma -1} p ({\bar{\varvec{x}}}, {\bar{t}})/\rho ({\bar{\varvec{x}}}, {\bar{t}}) >0\), \(\forall \left( {\bar{\varvec{x}}}, {\bar{t}} \right) \in {\mathbb {R}}^3 \times {\mathbb {R}}^+\). It has been shown in [44] that, for smooth solutions of (10), the quantity \(\frac{\nabla \cdot \mathbf{B }}{\rho W}\) satisfies

$$\begin{aligned} \frac{\partial }{\partial t} \left( \frac{\nabla \cdot \mathbf{B }}{\rho W} \right) + \mathbf{v } \cdot \nabla \left( \frac{\nabla \cdot \mathbf{B }}{\rho W} \right) = 0, \end{aligned}$$

which implies that \(\frac{\nabla \cdot \mathbf{B }}{\rho W}\) remains constant along the integral curve \({\varvec{x}}={\varvec{x}}(t;\bar{\varvec{x}},{\bar{t}})\), and further yields (13). The proof is completed. \(\square \)

Proof of Theorem 2

For the first-order DG method (\(k=0\)), \(\mathbf{U }_h |_K ({\varvec{x}}) \equiv {{\bar{\mathbf{U }}}}_K\), \(\forall K \in {{\mathcal {T}}}_h\), and

$$\begin{aligned} {\widetilde{\varvec{\mathcal {J}}}}_K^{(1)} ( \mathbf{U }_h )&= - \frac{1}{2|K|} \sum _{ {{\mathscr {E}}} \in \partial K } \Big [ |{{\mathscr {E}}}| \Big ( \big \langle \mathbf{n }_{{{\mathscr {E}}},K}, \mathbf{F } ( {{\bar{\mathbf{U }}}}_K ) + \mathbf{F } ( {{\bar{\mathbf{U }}}}_{K_{{\mathscr {E}}}} ) \big \rangle - a ( {{\bar{\mathbf{U }}}}_{K_{{\mathscr {E}}}} - {{\bar{\mathbf{U }}}}_{K} ) \Big ) \Big ] \nonumber \\&= - \frac{1}{2|K|} \sum _{ {{\mathscr {E}}} \in \partial K } \Big [ |{{\mathscr {E}}}| \Big ( \big \langle \mathbf{n }_{{{\mathscr {E}}},K}, \mathbf{F } ( {{\bar{\mathbf{U }}}}_{K_{{\mathscr {E}}}} ) \big \rangle - a ( {{\bar{\mathbf{U }}}}_{K_{{\mathscr {E}}}} - {{\bar{\mathbf{U }}}}_{K} ) \Big ) \Big ], \end{aligned}$$
(61)
$$\begin{aligned} {\widetilde{\varvec{\mathcal {J}}}}_K^{(2)} ( \mathbf{U }_h)&= -\frac{1}{2|K|} \sum _{ {{\mathscr {E}}} \in \partial K } \Big ( |{{\mathscr {E}}}| \left\langle \mathbf{n }_{{{\mathscr {E}}},K}, \bar{\mathbf {B}}_{ K_{{\mathscr {E}}}} - \bar{\mathbf {B}}_{ K} \right\rangle \mathbf{S } \left( {{\bar{\mathbf{U }}}}_K \right) \Big ) \nonumber \\&= - \frac{1}{2|K|} \sum _{ {{\mathscr {E}}} \in \partial K } \Big ( |{{\mathscr {E}}}| \left\langle \mathbf{n }_{{{\mathscr {E}}},K}, \bar{\mathbf {B}}_{ K_{{\mathscr {E}}}} \right\rangle \mathbf{S } \left( {{\bar{\mathbf{U }}}}_K \right) \Big ), \end{aligned}$$
(62)

where the identity \(\sum \limits _{ {{\mathscr {E}}} \in \partial K } |{{\mathscr {E}}}| \mathbf{n }_{{{\mathscr {E}}},K} = \mathbf{0 }\) has been used. In order to prove the PCP property (31), it suffices to show

$$\begin{aligned} {{\bar{\mathbf{U }}}}_K^{\varDelta t} := {{\bar{\mathbf{U }}}}_K + \varDelta t {\widetilde{\varvec{\mathcal {J}}}}_K ( \mathbf{U }_h ) \in {{\mathcal {G}}},\qquad \forall K \in {{\mathcal {T}}}_h, \end{aligned}$$
(63)

under the CFL type condition (52) and the condition that \({{\bar{\mathbf{U }}}}_K \in {{\mathcal {G}}},~\forall K \in {{\mathcal {T}}}_h\). In the following, we prove (63) by using the second equivalent form \({{\mathcal {G}}}_2={{\mathcal {G}}}\) in Lemma 2 and verifying that \({{\bar{\mathbf{U }}}}_K^{\varDelta t} \in {{\mathcal {G}}}_2\), \(\forall K \in {{\mathcal {T}}}_h\).

We first show that the mass density \({\bar{D}}_K^{\varDelta t}>0\). Recalling that the first component of \(\mathbf{S }(\mathbf{U })\) is zero, we know that the first component of \(\widetilde{{\mathcal {J}}}_K^{(2)}\) is zero. Then, we obtain

$$\begin{aligned} {\bar{D}}_K^{\varDelta t}&= {\bar{D}}_K - \frac{\varDelta t}{2|K|} \sum _{ {{\mathscr {E}}} \in \partial K } \Big [ | {{\mathscr {E}}} | \Big ( {\bar{D}}_{ K_{{\mathscr {E}}}} \langle \mathbf{n }_{{{\mathscr {E}}},K}, {\bar{\mathbf{v }}}_{ K_{{\mathscr {E}}}} \rangle - a ( {\bar{D}}_{K_{{\mathscr {E}}}} - {\bar{D}}_{K} ) \Big ) \Big ] \\&= \left( 1 - \frac{a \varDelta t }{2 |K|} \sum _{ {{\mathscr {E}}} \in \partial K } | {{\mathscr {E}}} | \right) {\bar{D}}_{K} + \frac{\varDelta t}{2|K|} \sum _{ {{\mathscr {E}}} \in \partial K } \Big [ | {{\mathscr {E}}} | \left( a - \langle \mathbf{n }_{{{\mathscr {E}}},K}, {\bar{\mathbf{v }}}_{ K_{{\mathscr {E}}}} \rangle \right) {\bar{D}}_{K_{{\mathscr {E}}}} \Big ] \ge 0, \end{aligned}$$

where we have used the CFL condition (52) and \(\langle \mathbf{n }_{{{\mathscr {E}}},K}, {\bar{\mathbf{v }}}_{ K_{{\mathscr {E}}}} \rangle \le | {\bar{\mathbf{v }}}_{ K_{{\mathscr {E}}}} | < 1 = c =a\).

We then prove that \({{\bar{\mathbf{U }}}}_K^{\varDelta t} \cdot {{ {\varvec{\xi }}^*}} + {p^*_m} >0\) for any auxiliary variables \(\mathbf{B }^* \in {\mathbb {R}}^3 \) and \(\mathbf{v }^* \in {\mathbb {B}}_1(\mathbf{0 })\), where \({\varvec{\xi }}^*\) and \({p^*_m}\) are functions of \((\mathbf{B }^*,\mathbf{v }^*)\) as defined in Lemma 2. Using the inequality (23) in Lemma 6 gives

$$\begin{aligned} \left( {{\bar{\mathbf{U }}}}_{ K_{{\mathscr {E}}}} \!-\! \frac{1}{a} \big \langle \mathbf{n }_{{{\mathscr {E}}},K}, {\mathbf {F}}(\bar{{\mathbf {U}}}_{ K_{{\mathscr {E}}}}) \big \rangle \right) \!\cdot \! {\varvec{\xi }}^* \!+\! p_{m}^* \!\ge \! \frac{1}{a} \left( \langle \mathbf{n }_{{{\mathscr {E}}},K}, \mathbf{v }^*\rangle p_{m}^* - \langle \mathbf{n }_{{{\mathscr {E}}},K}, \bar{\mathbf{B }}_{ K_{{\mathscr {E}}}} \rangle ({\mathbf {v}}^* \cdot {\mathbf {B}}^*)\right) . \end{aligned}$$

It, along with (61), imply that

$$\begin{aligned} {\widetilde{\varvec{\mathcal {J}}}}_K^{(1)} ( \mathbf{U }_h ) \cdot {\varvec{\xi }}^*&= - \frac{ a }{ 2|K| } \sum _{ {{\mathscr {E}}} \in \partial K } \left[ |{{\mathscr {E}}}| \left( {{\bar{\mathbf{U }}}}_{ K} \cdot {\varvec{\xi }}^* + p_{m}^* \right) \right] \\&\quad + \frac{ a }{ 2 |K| } \sum _{ {{\mathscr {E}}} \in \partial K } \left\{ |{{\mathscr {E}}}| \left[ \left( {{\bar{\mathbf{U }}}}_{ K_{{\mathscr {E}}}} - \frac{1}{a} \big \langle \mathbf{n }_{{{\mathscr {E}}},K}, {\mathbf {F}}(\bar{{\mathbf {U}}}_{ K_{{\mathscr {E}}}}) \big \rangle \right) \cdot {\varvec{\xi }}^* + p_{m}^* \right] \right\} \\&\ge - \frac{ a }{ 2|K| } \sum _{ {{\mathscr {E}}} \in \partial K } \left[ |{{\mathscr {E}}}| \left( {{\bar{\mathbf{U }}}}_{ K} \cdot {\varvec{\xi }}^* + p_{m}^* \right) \right] \\&\quad + \frac{ 1 }{ 2 |K| } \sum _{ {{\mathscr {E}}} \in \partial K } \Big \{ |{{\mathscr {E}}}| \Big [ \langle \mathbf{n }_{{{\mathscr {E}}},K}, \mathbf{v }^*\rangle p_{m}^* - \langle \mathbf{n }_{{{\mathscr {E}}},K}, \bar{\mathbf{B }}_{ K_{{\mathscr {E}}}} \rangle ({\mathbf {v}}^* \cdot {\mathbf {B}}^*) \Big ] \Big \} \\&= - \frac{ a }{ 2|K| } \sum _{ {{\mathscr {E}}} \in \partial K } \left( |{{\mathscr {E}}}| \left( {{\bar{\mathbf{U }}}}_{ K} \cdot {\varvec{\xi }}^* + p_{m}^* \right) \right) - \frac{ {\mathbf {v}}^* \cdot {\mathbf {B}}^* }{ 2 |K| } \sum _{ {{\mathscr {E}}} \in \partial K } |{{\mathscr {E}}}| \langle \mathbf{n }_{{{\mathscr {E}}},K}, \bar{\mathbf{B }}_{ K_{{\mathscr {E}}}} \rangle , \end{aligned}$$

where the identity \(\sum \limits _{ {{\mathscr {E}}} \in \partial K } |{{\mathscr {E}}}| \mathbf{n }_{{{\mathscr {E}}},K} = \mathbf{0 }\) has been used in the last equality. Combining (62) and the above estimate, we obtain

$$\begin{aligned} {{\bar{\mathbf{U }}}}_K^{\varDelta t} \cdot {{ {\varvec{\xi }}^*}} + {p^*_m}&= {{\bar{\mathbf{U }}}}_K \cdot {{ {\varvec{\xi }}^*}} + {p^*_m} + \varDelta t {\widetilde{\varvec{\mathcal {J}}}}_K^{(1)} ( \mathbf{U }_h ) \cdot {\varvec{\xi }}^* + \varDelta t {\widetilde{\varvec{\mathcal {J}}}}_K^{(2)} ( \mathbf{U }_h ) \cdot {\varvec{\xi }}^* \\&\ge {{\bar{\mathbf{U }}}}_K \cdot {{ {\varvec{\xi }}^*}} + {p^*_m} - \frac{ a \varDelta t }{ 2|K| } \sum _{ {{\mathscr {E}}} \in \partial K } |{{\mathscr {E}}}| \left( {{\bar{\mathbf{U }}}}_{ K} \cdot {\varvec{\xi }}^* + p_{m}^* \right) \\&\quad - \frac{ \varDelta t }{ 2 |K| } \sum _{ {{\mathscr {E}}} \in \partial K } |{{\mathscr {E}}}| \langle \mathbf{n }_{{{\mathscr {E}}},K}, \bar{\mathbf{B }}_{ K_{{\mathscr {E}}}} \rangle \left( \mathbf{S } \left( {{\bar{\mathbf{U }}}}_K \right) \cdot {\varvec{\xi }}^*+ {\mathbf {v}}^* \cdot {\mathbf {B}}^* \right) \\&\ge \left( 1-\frac{ a \varDelta t }{ 2|K| } \sum _{ {{\mathscr {E}}} \in \partial K } |{{\mathscr {E}}}| \right) \left( {{\bar{\mathbf{U }}}}_{ K} \cdot {\varvec{\xi }}^* + p_{m}^* \right) \\&\quad -\frac{ \varDelta t }{ 2 |K| } \left| \sum _{ {{\mathscr {E}}} \in \partial K } |{{\mathscr {E}}}| \langle \mathbf{n }_{{{\mathscr {E}}},K}, \bar{\mathbf{B }}_{ K_{{\mathscr {E}}}} \rangle \right| \left| \mathbf{S } \left( {{\bar{\mathbf{U }}}}_K \right) \cdot {\varvec{\xi }}^*+ {\mathbf {v}}^* \cdot {\mathbf {B}}^* \right| . \end{aligned}$$

Thanks to Lemma 4, we obtain

$$\begin{aligned} {{\bar{\mathbf{U }}}}_K^{\varDelta t} \cdot {{ {\varvec{\xi }}^*}} + {p^*_m}&\ge \left( 1-\frac{ a \varDelta t }{ 2|K| } \sum _{ {{\mathscr {E}}} \in \partial K } |{{\mathscr {E}}}| \right) \left( {{\bar{\mathbf{U }}}}_{ K} \cdot {\varvec{\xi }}^* + p_{m}^* \right) \\&\quad -\frac{ \varDelta t }{ 2 |K| } \left| \sum _{ {{\mathscr {E}}} \in \partial K } |{{\mathscr {E}}}| \langle \mathbf{n }_{{{\mathscr {E}}},K}, \bar{\mathbf{B }}_{ K_{{\mathscr {E}}}} \rangle \right| \frac{1}{ \sqrt{ {\bar{\rho }}_K \bar{H}_K} } \left( {{\bar{\mathbf{U }}}}_{ K} \cdot {\varvec{\xi }}^* + p_{m}^* \right) \\&= \left( 1 - \frac{a \varDelta t }{2|K|} \sum _{ {{\mathscr {E}}} \in \partial K } \big | {{\mathscr {E}}} \big | - \varDelta t \frac{ \left| \mathrm{div} _{K} \mathbf{B }_h\right| }{ \sqrt{{\bar{\rho }}_K {\bar{H}}_K } } \right) \left( {{\bar{\mathbf{U }}}}_{ K} \cdot {\varvec{\xi }}^* + p_{m}^* \right) > 0, \end{aligned}$$

where the identity \(\sum \limits _{ {{\mathscr {E}}} \in \partial K } |{{\mathscr {E}}}| \mathbf{n }_{{{\mathscr {E}}},K} = \mathbf{0 }\) has been used in the equality, and the CFL condition (52) is used in the last inequality. Therefore, we have

$$\begin{aligned} {{\bar{\mathbf{U }}}}_K^{\varDelta t} \cdot {{ {\varvec{\xi }}^*}} + {p^*_m} >0, \qquad \forall \mathbf{B }^* \in {\mathbb {R}}^3,~~\forall \mathbf{v }^* \in {\mathbb {B}}_1(\mathbf{0 }), \end{aligned}$$

which, along with \({\bar{D}}_K^{\varDelta t}>0\), yield \({{\bar{\mathbf{U }}}}_K^{\varDelta t} \in {{\mathcal {G}}}_2 = {{\mathcal {G}}}\). The proof is completed. \(\square \)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wu, K., Shu, CW. Provably physical-constraint-preserving discontinuous Galerkin methods for multidimensional relativistic MHD equations. Numer. Math. 148, 699–741 (2021). https://doi.org/10.1007/s00211-021-01209-4

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00211-021-01209-4

Mathematics Subject Classification

Search

Navigation