Skip to main content
SpringerLink
Log in

Linear and energy stable schemes for the Swift–Hohenberg equation with quadratic-cubic nonlinearity based on a modified scalar auxiliary variable approach

  • Published:
Journal of Engineering Mathematics Aims and scope Submit manuscript

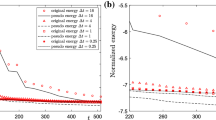
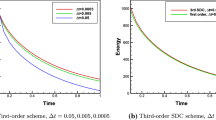
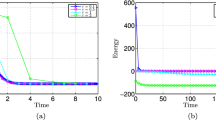
Abstract

In this study, we develop linear and energy stable numerical schemes for the Swift–Hohenberg equation with quadratic-cubic nonlinearity. A modified scalar auxiliary variable (SAV) approach is used to construct the temporally first- and second-order accurate discretizations. Different from the classical SAV approach, the proposed schemes permit us to solve the governing equations in a step-by-step manner, i.e., the calculation of inner product is not needed. We analytically prove the energy stability. We solve the resulting system of discrete equations using the linear multigrid method. We perform various numerical examples to show the accuracy and energy stability of the proposed method. The pattern formations in two- and three-dimensional spaces are also simulated.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Swift J, Hohenberg PC (1977) Hydrodynamic fluctuation at the convective instability. Phys Rev A 15:319–328

    Article  Google Scholar 

  2. Haken H (1983) Advanced synergetics. Springer, Berlin

    Book  MATH  Google Scholar 

  3. Lee HG (2019) An energy stable method for the Swift–Hohenberg equation with quadratic-cubic nonlinearity. Comput Methods Appl Mech Eng 343:40–51

    Article  MathSciNet  MATH  Google Scholar 

  4. Hutt A, Atay FM (2005) Analysis nonlocal neural fields for both general and gamma-distributed connectivities. Physica D 203:30–54

    Article  MathSciNet  MATH  Google Scholar 

  5. Su J, Fang W, Yu Q, Li Y (2019) Numerical simulation of Swift–Hohenberg equation by the fourth-order compact scheme. Comput Appl Math 38:54

    Article  MathSciNet  MATH  Google Scholar 

  6. Kudryashov NA, Sinelshchikov DI (2012) Exact solutions of the Swift–Hohenberg equation with dispersion. Commun Nonlinear Sci Numer Simul 17:26–34

    Article  MathSciNet  MATH  Google Scholar 

  7. Xi H, Viñals J, Gunton JD (1991) Numerical solution of the Swift–Hohenberg equation in two dimensions. Physica A 177:356–365

    Article  Google Scholar 

  8. Christov CI, Pontes J (2002) Numerical scheme for Swift–Hohenberg equation with strict implementation of Lyapunov functional. Math Comput Model 35:87–99

    Article  MathSciNet  MATH  Google Scholar 

  9. Gomez H, Nogueira X (2012) A new space-time discretization for the Swift–Hohenberg equation that strictly respects the Lyapunov functional. Commun Nonlinear Sci Numer Simul 17:4930–4946

    Article  MathSciNet  MATH  Google Scholar 

  10. Nikolay NA, Ryabov PN (2016) Analytical and numerical solutions of the generalized dispersive Swift–Hohenberg equation. Appl Math Comput 286:171–177

    MathSciNet  MATH  Google Scholar 

  11. Dehghan M, Abbaszadeh M (2017) The meshless local collocation method for solving multi-dimensional Cahn–Hilliard, Swift–Hohenberg and phase field crystal equations. Eng Anal Bound Elem 78:49–64

    Article  MathSciNet  MATH  Google Scholar 

  12. Abbaszadeh M, Khodadadian A, Parvizi M, Dehghan M, Heitzinger C (2019) A direct meshless local collocation method for solving stochastic Cahn–Hilliard-Cook and stochastic Swift–Hohenberg equations. Eng Anal Bound Elem 98:253–264

    Article  MathSciNet  MATH  Google Scholar 

  13. Sarmiento AF, Espath LFR, Vignal P, Dalcin L, Parsani M, Calo VM (2018) An energy-stable generalized-\(\alpha \) method for the Swift–Hohenberg equation. J Comput Appl Math 344:836–851

    Article  MathSciNet  MATH  Google Scholar 

  14. Lee HG (2017) A semi-analytical Fourier spectral method for the Swift–Hohenberg equation. Comput Math Appl 74:1885–1896

    Article  MathSciNet  MATH  Google Scholar 

  15. Wise SM, Wang C, Lowengrub JS (2009) An energy-stable and convergent finite-difference scheme for the phase field crystal equation. SIAM J Numer Anal 47(3):2269–2288

    Article  MathSciNet  MATH  Google Scholar 

  16. Hu Z, Wise SM, Wang C, Lowengrub JS (2009) Stable and efficient finite-difference nonlinear-multigrid schemes for the phase field crystal equation. J Comput Phys 228:5323–5339

    Article  MathSciNet  MATH  Google Scholar 

  17. Guan Z, Heinonen V, Lowengrub J, Wang C, Wise SM (2016) An energy stable, hexagonal finite difference scheme for the 2D phase field crystal amplitude equations. J Comput Phys 321(15):1026–1054

    Article  MathSciNet  MATH  Google Scholar 

  18. Wang C, Wise SM (2011) An energy stable and convergent finite-difference scheme for the modified phase field crystal equation. SIAM J Numer Anal 49(3):945–969

    Article  MathSciNet  MATH  Google Scholar 

  19. Baskaran A, Hu Z, Lowengrub JS, Wang C, Wise SM, Zhou P (2013) Energy stable and efficient finite-difference nonlinear multigrid schemes for the modified phase field crystal equation. J Comput Phys 250(1):270–292

    Article  MathSciNet  MATH  Google Scholar 

  20. Cheng K, Wang C, Wise SM (2019) An energy stable BDF2 Fourier pseudo-spectral numerical scheme for the square phase field crystal equation. Commun Comput Phys 26(5):1335–1364

    Article  MathSciNet  Google Scholar 

  21. Baskaran A, Lowengrub JS, Wang C, Wise SM (2013) Convergence analysis of a second order convex splitting scheme for the modified phase field crystal equation. SIAM J Numer Anal 51(5):2851–2873

    Article  MathSciNet  MATH  Google Scholar 

  22. Dong L, Feng W, Wang C, Wise SM, Zhang Z (2018) Convergence analysis and numerical implementation of a second order numerical scheme for the three-dimensional phase field crystal equation. Comput Math Appl 75(6):1912–1928

    Article  MathSciNet  MATH  Google Scholar 

  23. Long J, Luo C, Yu Q, Li Y (2019) An unconditionally stable compact fourth-order finite difference scheme for three dimensional Allen–Cahn equation. Comput Math Appl 77(4):1042–1054

    Article  MathSciNet  MATH  Google Scholar 

  24. Hou T, Tang T, Yang J (2017) Numerical analysis of fully discretized Crank–Nicolson scheme for fractional-in-space Allen–Cahn equations. J Sci Comput 72:1214–1231

    Article  MathSciNet  MATH  Google Scholar 

  25. Liao HL, Tang T, Zhou T (2020) A second-order and nonuniform time-stepping maximum-principle preserving scheme for time-fractional Allen–Cahn equations. J Comput Phys 414(1):109473

    Article  MathSciNet  MATH  Google Scholar 

  26. Grillo A, Carfagna M, Federico S (2018) An Allen–Cahn approach to the remodelling of fibre-reinforced anisotropic materials. J Eng Math 109:139–172

    Article  MathSciNet  MATH  Google Scholar 

  27. Cheng K, Feng W, Wang C, Wise SM (2019) An energy stable fourth order finite difference scheme for the Cahn–Hilliard equation. J Comput Appl Math 362(15):574–595

    Article  MathSciNet  MATH  Google Scholar 

  28. Yang J, Li Y, Lee C, Jeong D, Kim J (2019) A conservative finite difference scheme for the \(N\)-component Cahn–Hilliard system on curved surfaces in 3D. J Eng Math 119:149–166

    Article  MathSciNet  MATH  Google Scholar 

  29. Li Q, Mei L, You B (2018) A second-order, unqiuely solvable, energy stable BDF numerical scheme for the phase field crystal model. Appl Numer Math 134:46–65

    Article  MathSciNet  MATH  Google Scholar 

  30. Guillén-González F, Tierra G (2013) On linear schemes for the Cahn–Hilliard diffuse interface model. J Comput Phys 234:140–171

    Article  MathSciNet  MATH  Google Scholar 

  31. Liu Z, Li X (2019) Efficient modified stabilized invariant energy quadratization approaches for phase-field crystal equation. Numer Algorithms 85:107–132

    Article  MathSciNet  MATH  Google Scholar 

  32. Li Q, Mei L (2020) Efficient, decoupled, and second-order unconditionally energy stable numerical schemes for the coupled Cahn–Hilliard system in copolymer/homopolymer mixtures. Comput Phys Commun 260:107290

    Article  MathSciNet  Google Scholar 

  33. Yang X, Han D (2017) Linearly first- and second-order, unconditionally energy stable schemes for the phase field crystal model. J Comput Phys 330(1):1116–1134

    Article  MathSciNet  MATH  Google Scholar 

  34. Liu Z (2019) Efficient invariant energy quadratization and scalar auxiliary variable approaches without bounded below restriction for phase field models. arXiv preprint

  35. Liu Z, Li X (2019) Step-by-step solving schemes based on scalar auxiliary variable and invariant energy quadratization approaches for gradient flows. arXiv preprint

  36. Yang J, Kim J (2020) An improved scalar auxiliary variable (SAV) approach for the phase-field surfactant model. Appl Math Model 90:11–29

    Article  MathSciNet  Google Scholar 

  37. Espath L, Calo VM, Fried E (2020) Generalized Swift–Hohenberg and phase-field-crystal equations based on a second-gradient phase-field theory. Meccanica 55:1853–1868

    Article  MathSciNet  Google Scholar 

  38. Shen J, Xu J (2018) Convergence and error analysis for the scalar auxiliary variable (SAV) schemes to gradient flows. SIAM J Numer Anal 56:2895–2912

    Article  MathSciNet  MATH  Google Scholar 

  39. Kim J, Kang K, Lowengrub J (2004) Conservative multigrid methods for the Cahn–Hilliard fluids. J Comput Phys 193:511–543

    Article  MathSciNet  MATH  Google Scholar 

  40. Wise S, Kim J, Lowengrub J (2007) Solving the regularized, strongly anisotropic Cahn–Hilliard equation by an adaptive nonlinear multigrid method. J Comput Phys 226:414–446

    Article  MathSciNet  MATH  Google Scholar 

  41. Feng W, Guo Z, Lowengrub JS, Wise SM (2018) A mass-conservative adaptive FAS multigrid solver for cell-centered finite difference methods on block-structured locally-cartesian grids. J Comput Phys 352:463–497

    Article  MathSciNet  MATH  Google Scholar 

  42. Yang J, Kim J (2020) An unconditionally stable second-order accurate method for systems of Cahn–Hilliard equations. Commun Nonlinear Sci Numer Simul 87:105276

    Article  MathSciNet  MATH  Google Scholar 

  43. Baskaran A, Hu Z, Lowengrub JS, Wang C, Wise SM, Zhou P (2013) Energy stable and efficient finite-difference nonlinear multigrid schemes for the modified phase field crystal equation. J Comput Phys 250:270–292

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

J. Yang is supported by China Scholarship Council (201908260060). The corresponding author (J.S. Kim) was supported by Basic Science Research Program through the National Research Foundation of Korea (NRF) funded by the Ministry of Education (NRF-2019R1A2C1003053). The authors appreciate the reviewers for their constructive comments, which have improved the quality of this paper.

Author information

Authors and Affiliations

  1. Department of Mathematics, Korea University, Seoul, 02841, Republic of Korea

    Junxiang Yang & Junseok Kim

Authors
  1. Junxiang Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Junseok Kim
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Junseok Kim.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yang, J., Kim, J. Linear and energy stable schemes for the Swift–Hohenberg equation with quadratic-cubic nonlinearity based on a modified scalar auxiliary variable approach. J Eng Math 128, 21 (2021). https://doi.org/10.1007/s10665-021-10122-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10665-021-10122-6

Keywords

Search

Navigation