Micromechanics-based elasto-plastic–damage energy formulation for strain gradient solids with granular microstructure

Abstract

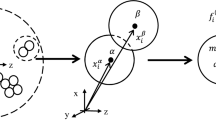
This paper is devoted to the development of a continuum theory for materials having granular microstructure and accounting for some dissipative phenomena like damage and plasticity. The continuum description is constructed by means of purely mechanical concepts, assuming expressions of elastic and dissipation energies as well as postulating a hemi-variational principle, without incorporating any additional postulate like flow rules. Granular micromechanics is connected kinematically to the continuum scale through Piola’s ansatz. Mechanically meaningful objective kinematic descriptors aimed at accounting for grain–grain relative displacements in finite deformations are proposed. Karush–Kuhn–Tucker (KKT)-type conditions, providing evolution equations for damage and plastic variables associated with grain–grain interactions, are derived solely from the fundamental postulates. Numerical experiments have been performed to investigate the applicability of the model. Cyclic loading–unloading histories have been considered to elucidate the material hysteretic features of the continuum, which emerge from simple grain–grain interactions. We also assess the competition between damage and plasticity, each having an effect on the other. Further, the evolution of the load-free shape is shown not only to assess the plastic behavior, but also to make tangible the point that, in the proposed approach, plastic strain is found to be intrinsically compatible with the existence of a placement function.

Appendix: Justification of normal and tangent relative displacement definitions

Let us assume that the grain p is at the origin ($\varvec{X}_{p}=0$) and the grain n at $\varvec{X}_{n}=L\hat{c}$.

For the placement field indicating an elongation along the intergranular axis $\hat{c}$, i.e.,

$$\begin{aligned} \chi \left( \varvec{X}\right) =\varvec{X}+\alpha \left( \varvec{X}\cdot \hat{c}\right) \hat{c}, \end{aligned}$$

on the one hand, the grain p does not displace ($\chi \left( \varvec{X}_{p}\right) =0=u\left( \varvec{X}_{p}\right)$), the grain n places at $\chi \left( \varvec{X}_{n}\right) =L\hat{c}+\alpha L\hat{c}$ with a displacement $u\left( \varvec{X}_{n}\right) =\alpha L\hat{c}$. Thus, the displacement of one grain with respect to the other along the intergranular axis $\hat{c}$ is

$$\begin{aligned} \left[ u\left( \varvec{X}_{n}\right) -u\left( \varvec{X}_{p}\right) \right] \cdot \hat{c}=\alpha L, \end{aligned}$$

and no relative displacement occurs in the transverse direction. On the other hand, the deformation gradient,

$$\begin{aligned} F=\nabla \varvec{\chi }=I+\alpha \hat{c}\otimes \hat{c}=F^{T} \end{aligned}$$

is symmetric and the objective relative displacement yields,

$$\begin{aligned} u^{np}=\left[ I+\alpha \hat{c}\otimes \hat{c}\right] \left( L\hat{c}+\alpha L\hat{c}\right) -L\hat{c}=2\alpha L\hat{c}+\alpha ^{2}L\hat{c}. \end{aligned}$$

Thus, for small deformation ($\alpha \ll 1$), the definition (11)$_{1}$ of the normal displacement is justified, i.e., $u_{\eta }={\frac{1}{2}}u^{np}\cdot \hat{c}.$

For the placement field indicating a shear deformation transverse (e.g., in a direction $\hat{d}$) to the intergranular axis $\hat{c}$,

$$\begin{aligned} \chi \left( \varvec{X}\right) =\varvec{X}+\alpha \left( \varvec{X}\cdot \hat{c}\right) \hat{d},\qquad \hat{c}\cdot \hat{d}=0, \end{aligned}$$

On the one hand, the grain p does not displace ($\chi \left( \varvec{X}_{p}\right) =u\left( \varvec{X}_{p}\right) =0$), the grain n places at $\chi \left( \varvec{X}_{n}\right) =L\hat{c}+\alpha L\hat{d}$ with a displacement $u\left( \varvec{X}_{n}\right) =\alpha L\hat{d}$. Thus, the displacement of one grain with respect to the other along the direction $\hat{d}$ orthogonal to the intergranular axis $\hat{c}$ is

$$\begin{aligned} \left[ u\left( \varvec{X}_{n}\right) -u\left( \varvec{X}_{p}\right) \right] \cdot \hat{d}=\alpha L, \end{aligned}$$

and no relative displacement occurs along $\hat{c}$. On the other hand, the deformation gradient

$$\begin{aligned} F=\nabla \varvec{\chi }=I+\alpha \hat{d}\otimes \hat{c} \end{aligned}$$

is not symmetric and the objective relative displacement yields,

$$\begin{aligned} u^{np}=\left[ I+\alpha \hat{c}\otimes \hat{d}\right] \left( L\hat{c}+\alpha L\hat{d}\right) -L\hat{c}=L\hat{c}+\alpha L\hat{d}+\alpha \hat{c}\alpha L-L\hat{c}=\alpha L\hat{d}+\alpha ^{2}L\hat{c}. \end{aligned}$$

Thus, for small deformation ($\alpha \ll 1$) the definition (11)$_{2}$ of the tangent displacement is justified, i.e., $u_{\tau }=u^{np}-\left( u^{np}\cdot \hat{c}\right) \hat{c}.$