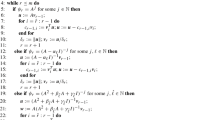Skip to main content
SpringerLink
Log in

Gauss–Laurent-type quadrature rules for the approximation of functionals of a nonsymmetric matrix

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

This paper is concerned with the approximation of matrix functionals of the form wTf(A)v, where \(A\in \mathbb {R}^{n\times n}\) is a large nonsymmetric matrix, \(\boldsymbol {w},\boldsymbol {v}\in \mathbb {R}^{n}\), and f is a function such that f(A) is well defined. We derive Gauss–Laurent quadrature rules for the approximation of these functionals, and also develop associated anti-Gauss–Laurent quadrature rules that allow us to estimate the quadrature error of the Gauss–Laurent rule. Computed examples illustrate the performance of the quadrature rules described.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Alqahtani, H., Reichel, L.: Simplified anti-Gauss quadrature rules with applications in linear algebra. Numer. Algorithms 77, 577–602 (2018)

    Article  MathSciNet  Google Scholar 

  2. Alqahtani, H., Reichel, L.: Generalized block anti-Gauss quadrature rules. Numer. Math. 143, 605–648 (2019)

    Article  MathSciNet  Google Scholar 

  3. Barkouki, H., Bentbib, A. H., Jbilou, K.: A matrix rational Lanczos method for model reduction in large-scale first- and second-order dynamical systems. Numer. Linear Algebra Appl. 24, Art.e2077 (2017)

    Article  MathSciNet  Google Scholar 

  4. Bentbib, A. H., El Ghomari, M., Jagels, C., Jbilou, K., Reichel, L.: The extended global Lanczos method for matrix function approximation. Electron. Trans. Numer. Anal. 50, 144–163 (2018)

    Article  MathSciNet  Google Scholar 

  5. Calvetti, D., Reichel, L., Sgallari, F.: Application of anti-Gauss quadrature rules in linear algebra. In: Gautschi, W., Golub, G.H., Opfer, G. (eds.) Applications and Computation of Orthogonal Polynomials, pp 41–56. Birkhäuser, Basel (1999)

  6. Deckers, K., Bultheel, A.: The existence and construction of rational Gauss-type quadrature rules. Appl. Math. Comput. 218, 10299–10320 (2012)

    MathSciNet  MATH  Google Scholar 

  7. Fenu, C., Martin, D., Reichel, L., Rodriguez, G.: Block Gauss and anti-Gauss quadrature with application to networks. SIAM J. Matrix Anal. Appl. 34, 1655–1684 (2013)

    Article  MathSciNet  Google Scholar 

  8. Gallivan, K., Grimme, E., Van Dooren, P.: A rational Lanczos algorithm for model reduction. Numer. Algorithms 12, 33–66 (1996)

    Article  MathSciNet  Google Scholar 

  9. Gautschi, W., Polynomials, Orthogonal: Computation and approximation, Oxford University Press Oxford (2004)

  10. Golub, G. H., Meurant, G.: Matrices, moments and quadrature with applications. Princeton University Press, Princeton (2010)

    MATH  Google Scholar 

  11. Gonchar, A. A., López Lagomasino, G.: On Markov’s theorem for multipoint Padé approximants. Math. USSR Sb. 34, 449–459 (1978)

    Article  Google Scholar 

  12. Heyouni, M., Jbilou, K.: An extended block Arnoldi algorithm for large-scale solutions of the continuous-time algebraic Riccati equation. Electron. Trans. Numer. Anal. 33, 53–62 (2009)

    MathSciNet  MATH  Google Scholar 

  13. Jagels, C., Jbilou, K., Reichel, L.: The extended global Lanczos method, Gauss–Radau quadrature, and matrix function approximation. J. Comput. Appl. Math. 381, Art.113027 (2021)

    Article  MathSciNet  Google Scholar 

  14. Jagels, C., Mach, T., Reichel, L., Vandebril, R.: Convergence rates for inverse-free rational approximation of matrix functions. Linear Algebra Appl. 510, 291–310 (2016)

    Article  MathSciNet  Google Scholar 

  15. Jagels, C., Reichel, L.: Recursion relations for the extended Krylov subspace method. Linear Algebra Appl. 434, 1716–1732 (2011)

    Article  MathSciNet  Google Scholar 

  16. Jagels, C., Reichel, L.: The structure of matrices in rational Gauss quadrature. Math. Comp. 82, 2035–2060 (2013)

    Article  MathSciNet  Google Scholar 

  17. Knizhnerman, L., Simoncini, V.: A new investigation of the extended Krylov subspace method for matrix function evaluations. Numer. Linear Algebra Appl. 17, 615–638 (2010)

    MathSciNet  MATH  Google Scholar 

  18. Laurie, D. P.: Anti-Gaussian quadrature formulas. Math. Comp. 65, 739–747 (1996)

    Article  MathSciNet  Google Scholar 

  19. Mach, T., Pranić, M. S., Vandebril, R.: Computing approximate extended Krylov subspaces without explicit inversion. Electron. Trans. Numer. Anal. 40, 414–435 (2013)

    MathSciNet  MATH  Google Scholar 

  20. Mach, T., Pranić, M. S., Vandebril, R.: Computing approximate (block) rational Krylov subspaces without explicit inversion with extensions to symmetric matrices. Electron. Trans. Numer. Anal. 43, 100–124 (2014)

    MathSciNet  MATH  Google Scholar 

  21. Moret, I., Novati, P.: RD-rational approximations of the matrix exponential. BIT Numer. Math. 44, 595–615 (2004)

    Article  MathSciNet  Google Scholar 

  22. Pozza, S., Pranić, M. S., Strakoš, Z.: Gauss quadrature for quasi-definite linear functionals. IMA J. Numer. Anal. 37, 1468–1495 (2017)

    MathSciNet  MATH  Google Scholar 

  23. Pozza, S., Pranić, M. S., Strakoš, Z.: The Lanczos algorithm and computing complex Gauss quadrature. Electron. Trans. Numer. Anal. 50, 1–18 (2018)

    Article  MathSciNet  Google Scholar 

  24. Pranić, M. S., Reichel, L.: Rational Gauss quadrature. SIAM J. Numer. Anal. 52, 832–851 (2014)

    Article  MathSciNet  Google Scholar 

  25. Pranić, M. S., Reichel, L.: Generalized anti-Gauss quadrature rules. J. Comput. Appl. Math. 284, 235–243 (2015)

    Article  MathSciNet  Google Scholar 

  26. Pranić, M., Reichel, L., Rodriguez, G., Wang, Z., Yu, X.: A rational Arnoldi process with applications. Numer. Linear Algebra Appl. 23, 1007–1022 (2016)

    Article  MathSciNet  Google Scholar 

  27. Schweitzer, M.: A two-sided short-recurrence extended Krylov subspace method for nonsymmetric matrices and its relation to rational moment matching. Numer. Algorithms 76, 1–31 (2016)

    Article  MathSciNet  Google Scholar 

  28. Van Buggenhout, N., Van Barel, M., Vandebril, R.: Biorthogonal rational Krylov subspace methods. Electron. Trans. Numer. Anal. 51, 451–468 (2019)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

The authors would like to thank a referee for carefully reading the manuscript and for comments that lead an improved presentation. This work was begun while L.R. visited the University of Banja Luka. He would like to thank M.P. for making this visit possible and enjoyable.

Funding

H.A. was supported by the Deanship of Scientific Research (DSR), King Abdulaziz University, Jeddah, under grant no. G-111-665-1441. L.R. was supported by NSF grant DMS-1729509.

Author information

Authors and Affiliations

  1. Department of Mathematical Sciences, Kent State University, Kent, OH, 44242, USA

    J. Alahmadi & L. Reichel

  2. Department of Mathematics, Faculty of Science and Arts, King Abdulaziz University, Rabigh, Saudi Arabia

    H. Alqahtani

  3. Department of Mathematics and Informatics, Faculty of Science, University of Banja Luka, M. Stojanovića 2, 51000, Banja Luka, Bosnia and Herzegovina

    M. S. Pranić

Authors
  1. J. Alahmadi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. H. Alqahtani
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. M. S. Pranić
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. L. Reichel
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to J. Alahmadi.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Alahmadi, J., Alqahtani, H., Pranić, M.S. et al. Gauss–Laurent-type quadrature rules for the approximation of functionals of a nonsymmetric matrix. Numer Algor 88, 1937–1964 (2021). https://doi.org/10.1007/s11075-021-01101-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-021-01101-0

Keywords

Search

Navigation