Evolutionary microdynamics of employee profit sharing as productivity-enhancing device

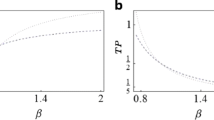
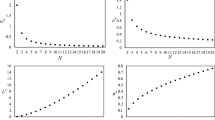
Abstract

We devise an analytical framework where the distribution of factor income across agents and the distribution of labor earnings across workers is influenced by the possibility of profit sharing with workers. Firms choose periodically to compensate workers with only a real base wage or a share of profits in addition to this real base wage as alternative productivity-enhancing strategies. As supported by robust empirical evidence, labor productivity is higher in profit-sharing firms than in non-sharing firms. The frequency distribution of productivity-enhancing strategies and labor productivity across firms are co-evolutionarily time-varying, which impacts on the distribution of factor income between profits and wages and thereby on the rates of employment and output growth, as well as on the distribution of labor earnings across workers. Heterogeneity in productivity-enhancing strategies across firms (and hence labor earnings inequality across workers) may be a stable long-run equilibrium outcome. This polymorphic equilibrium features the frequency of profit-sharing firms and the employment rate varying positively (negatively) with the real base wage (profit-sharing coefficient). Yet the employment rate is path dependent in the polymorphic equilibrium. As shown analytically and with numerical simulations, the microdynamics landscape is shaped by the real base wage and the profit-sharing coefficient as bifurcation parameters.

Appendices

Appendix 1

1.1 Proof of Proposition 2 on the stability properties of the long-run equilibrium

The Jacobian matrix evaluated around the monomorphic equilibrium $\left(\overline{\alpha},0\right)\subset \Theta$ is:

(32)

where ${\pi}_s^c\left(\overline{\alpha}\right)=\left(1-\delta \right)\left(1-\frac{v}{\overline{\alpha}}\right)$ and π_n = 1 − v.

Let ξ be an eigenvalue of the Jacobian matrix in (32). The eigenvalues of the Jacobian matrix in (32) are given by:

$$ {\xi}_1=\delta \mathrm{and}{\xi}_2=1+\left[{\pi}_s^c\ \left(\overline{\alpha}\right)-{\pi}_n\right]k. $$

(33)

Given that 0 < δ < 1, it follows that |ξ₁| < 1.

Let us investigate the absolute value of ξ₂. We want to find out under what condition(s) it follows that −1 < ξ₂ < 1. Per (33), it follows that −1 < ξ₂ < 1 obtains if, and only if, $-2<\left[{\pi}_s^c\left(\overline{\alpha}\right)-{\pi}_n\right]k<0.$

Given that $0<{\pi}_s^c\left(\overline{\alpha}\right)<1$ and 0 < π_n < 1, we find that $-1<{\pi}_s^c\left(\overline{\alpha}\right)-{\pi}_n<1$. Since the output to capital ratio, k, typically satisfies the condition given by 0 < k < 1 (see, e.g., Caselli 2005, and Caselli and Feyrer 2007), it follows that $-2<-k<\left[{\pi}_s^c\left(\overline{\alpha}\right)-{\pi}_n\right]k$. Note that ${\pi}_s^c\left(\alpha \right)-{\pi}_n=\left(1-\delta \right)\left(1-\frac{v}{\alpha}\right)-\left(1-v\right)$ is increasing in α. Given (20), it then follows that ${\pi}_s^c\left({\alpha}^{\ast}\right)-{\pi}_n=0$. Since $\overline{\alpha}-{\alpha}^{\ast }=0\ \mathrm{at}\ \delta =\overline{\delta}$ and ${\left.\frac{\partial \left(\overline{\alpha}-{\alpha}^{\ast}\right)}{\partial \delta}\right|}_{\delta =\overline{\delta}}=\frac{-{\left({v}^2-2v+\beta \right)}^3}{{\left(v-1\right)}^3v\left(v-\beta \right)}<0$ for all v ∈ (0, 1) ⊂ ℝ and β > 1, if $\delta >\overline{\delta}$, we then obtain $\overline{\alpha}<{\alpha}^{\ast }$, so that ${\pi}_s^c\left(\overline{\alpha}\right)-{\pi}_n<0$ and hence $\left[{\pi}_s^c\left(\overline{\alpha}\right)-{\pi}_n\right]k<0$. Thus, it follows from $\delta >\overline{\delta}$ that |ξ₂| < 1. Meanwhile, if $\delta >\overline{\delta}$, so that $\overline{\alpha}>{\alpha}^{\ast }$, it follows that ${\pi}_s^c\left(\overline{\alpha}\right)-{\pi}_n>0$ and hence $\left[{\pi}_s^c\left(\overline{\alpha}\right)-{\pi}_n\right]k<0$. Thus, it follows from $\delta <\overline{\delta}$ that |ξ₂| > 1. As it turns out, at $\delta =\overline{\delta}$ the system undergoes a bifurcation characterized by a change in both the stability property of the monomorphic equilibrium $\left(\overline{\alpha},0\right)\subset \Theta$ and the existence status of the polymorphic equilibrium (α^∗, λ^∗) ⊂ Θ. If the threshold given by $\delta =\overline{\delta}$ is crossed from below (above), the bifurcation is characterized by the monomorphic equilibrium $\left(\overline{\alpha},0\right)\subset \Theta$ ceasing to be a repulsor (an attractor) to become an attractor (a repulsor), and by the polymorphic equilibrium (α^∗, λ^∗) ⊂ Θ ceasing (coming) to exist. In any case, this completes the demonstration that the long-run equilibrium with no firm playing the profit-sharing strategy, $\left(\overline{\alpha},0\right)\subset \Theta$, is an attractor if $\delta >\overline{\delta}$ and a repulsor if $\delta <\overline{\delta}$.

The Jacobian matrix evaluated around the monomorphic equilibrium $\left(\overset{\sim }{\alpha },1\right)\subset \Theta$ is:

(34)

where ${\pi}_s^c\left(\beta \right)=\left(1-\delta \right)\left[1-\left(v/\beta \right)\right]$ and π_n = 1 − v.

Let ξ be an eigenvalue of the Jacobian matrix in (34). In this case, the eigenvalues of the Jacobian matrix is (34) are also easily computed:

$$ {\xi}_1=0\mathrm{and}{\xi}_2=b=1-\left[{\pi}_s^c\left(\beta \right)-{\pi}_n\right]k. $$

(35)

Therefore, the local stability of $\left(\overset{\sim }{\alpha },1\right)\subset \Theta$ depends on the absolute value of ξ₂. Given (35), it follows that −1 < ξ₂ < 1 obtains if, and only if, $0<\left[{\pi}_s^c\left(f(0)\right)-{\pi}_n\right]k<2$.

Since $0<{\pi}_s^c\left(\beta \right)<1\ \mathrm{and}\ 0<{\pi}_n<1$, it follows that $-1<{\pi}_s^c\left(\beta \right)-{\pi}_n<1$. Therefore, given that it is typically the case that 0 < k < 1, we can infer that $\left[{\pi}_s^c\left(\beta \right)-{\pi}_n\right]k<2$.

It can be seen that ${\pi}_s^c\left(\alpha \right)-{\pi}_n=\left(1-\delta \right)\left(1-\frac{v}{\alpha}\right)-\left(1-v\right)$ is increasing in α. Given (20), it follows that ${\pi}_s^c\left({\alpha}^{\ast}\right)-{\pi}_n=0$. Given that $\beta -{\alpha}^{\ast }=0\ \mathrm{at}\ \delta =\underline{\delta}$ and $\frac{\partial \left(\beta -{\alpha}^{\ast}\right)}{\partial \delta }=\frac{-\left(1-v\right)v}{{\left(v-\delta \right)}^2}<0$ for all v ∈ (0, 1) ⊂ ℝ and $\delta \ne v,\mathrm{if}\ \delta >\underline{\delta}$, we then obtain β < α^∗, so that ${\pi}_s^c\left(\beta \right)-{\pi}_n<0$ and therefore $\left[{\pi}_s^c\left(\beta \right)-{\pi}_n\right]k<0$. Thus, it follows from $\delta >\underline{\delta}$ that |ξ₂| > 1. Meanwhile, if $\delta <\underline{\delta}$, so that β > α^∗, it follows that ${\pi}_s^c\left(\beta \right)-{\pi}_n>0$ and hence $\left[{\pi}_s^c\left(\beta \right)-{\pi}_n\right]k>0$. Thus, it follows from $\delta <\underline{\delta}$ that |ξ₂| < 1. As it turns out, at $\delta =\underline{\delta}$ the system undergoes a bifurcation characterized by a change in both the stability property of the monomorphic equilibrium $\left(\overset{\sim }{\alpha },1\right)\subset \Theta$ and the existence status of the polymorphic equilibrium (α^∗, λ^∗) ⊂ Θ. In fact, if the threshold given by $\delta =\underline{\delta}$ is crossed from below (above), the bifurcation is characterized by the monomorphic equilibrium $\left(\overset{\sim }{\alpha },1\right)\subset \Theta$ ceasing to be an attractor (a repulsor) to become a repulsor (an attractor), and by the polymorphic equilibrium (α^∗, λ^∗) ⊂ Θ coming (ceasing) to exist. In any case, this completes the demonstration that the long-run equilibrium with all firms playing the profit-sharing strategy, $\left(\overset{\sim }{\alpha },1\right)\subset \Theta$, is an attractor if $\delta <\underline{\delta}$ and a repulsor if $\delta >\underline{\delta}$.

The Jacobian matrix evaluated around the polymorphic equilibrium (α^∗, λ^∗) ⊂ Θ is:

(36)

Let ξ be an eigenvalue of the Jacobian matrix in (36). We can set the characteristic equation of the linearization around the equilibrium as:

(37)

where a ≡ δ(1 − λ^∗) > 0, b ≡ δ(α^∗ − v) > 0, and $c\equiv {\lambda}^{\ast}\left(1-{\lambda}^{\ast}\right)\left(1-\delta \right)\frac{v}{{\left({\alpha}^{\ast}\right)}^2}k>0$.

We can use the Samuelson stability conditions for a second order characteristic equation to determine under what conditions the two eigenvalues are inside the unit circle. Based on Farebrother (1973, p. 396, inequalities 2.4 and 2.5), we can establish the following set of simplified Samuelson conditions for the quadratic polynomial in (37):

$$ 1+a+ bc>\left|-\left(a+1\right)\right|=a+1\mathrm{and}\mathrm{a}+\mathrm{bc}<1. $$

(38)

Let us prove that these conditions are satisfied if a + bc < 1.

First, note that 1 + a + bc > a + 1 simplifies to bc > 0, which is trivially satisfied given that b > 0 and c > 0.

Meanwhile, the second inequality, a + bc < 1, can be expressed as follows:

$$ a+ bc=\delta \left(1-{\lambda}^{\ast}\right)\left\{1+\frac{v\left(1-\delta \right){\lambda}^{\ast}\left({\alpha}^{\ast }-v\right)k}{{\left({\alpha}^{\ast}\right)}^2}\right\}<1. $$

(39)

Substitution of (20) and (21) in the expression a + bc yields:

$$ a+ bc=\frac{\left[-\beta \delta +v\left(\beta -1+\delta \right)\right]\left\{v\left(\delta -1\right)\delta +k\left(v-\delta \right)\left[{v}^2\delta +\beta \delta -v\left(\beta -1+2\delta \right)\right]\right\}}{\left(1-v\right){v}^2\left(1-\delta \right)\delta }. $$

(39a)

Note that at δ = v it follows that a + bc = 1. Besides, we have that:

$$ \frac{\partial \left(a+ bc\right)}{\partial \delta }=\frac{\left(\beta -v\right)\left\{\left[1+k\left(2-v\right)\right]v-\beta k\right\}+\frac{k{\left(v-1\right)}^3\left(v-\beta \right)\beta }{{\left(\delta -1\right)}^2}+\frac{kv^3{\left(\beta -1\right)}^2}{\delta^2}}{\left(1-v\right){v}^2}>0 $$

(40)

if we assume that the following condition is satisfied:

$$ \beta <\frac{\left[1+k\left(2-v\right)\right]v}{k}. $$

(41)

Thus, since a + bc = 1 at δ = v and given (40), it follows that the inequality in (39) holds for all δ ∈ (0, v) ⊂ ℝ. As a result, by assuming that (41) is satisfied, we can conclude that for all $\delta \in \left(\underline{\delta},\overline{\delta}\right)\subset \left(0,v\right)\subset \mathbb{R}$ the stability condition in (39) is satisfied as well, so that the polymorphic equilibrium represented by (α^∗, λ^∗) ⊂ Θ is an attractor.

Appendix 2

1.1 A specific derivation of the replicator dynamic in (17)

In order to specifically deal with the employment rate in the polymorphic equilibrium, we can derive the replicator dynamic in (1) as follows. Let us consider that the probability with which a type n firm contemplates the convenience of switching productivity-enhancing strategy is determined by the popularity of the alternative, profit-sharing strategy, which is measured by λ_t. Having a type n firm become a potential strategy switcher, the probability with which the actual strategy switch materializes is assumed to be given by $\mathit{\operatorname{Max}}\left\{{r}_{s,t}^c-{r}_n,0\right\}= kMax\left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}$, where we assume that 0 < k < 1, as we have already done in Appendix 1. As a result, assuming that these two events are independent, the probability that a type n firm switches strategy to become a type s firm in a certain period t is represented by ${\lambda}_t kMax\left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}$. It follows that the estimated inflow to the subpopulation of type s firms is given by:

$$ \left(1-{\lambda}_t\right){\lambda}_t kMax\left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}. $$

(42)

Analogously, the estimated inflow to the subpopulation of type n firms in a given period t is given by:

$$ {\lambda}_t\left(1-{\lambda}_t\right) kMax\left\{{\pi}_n-{\pi}_{s,t}^c,0\right\}. $$

(43)

Therefore, the net inflow to the subpopulation of type s firms in a given period t can be obtained by subtracting (43) from (42), which yields:

$$ {\lambda}_{t+1}-{\lambda}_t=\left(1-{\lambda}_t\right){\lambda}_t kMax\left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}-{\lambda}_t\left(1-{\lambda}_t\right) kMax\left\{{\pi}_n-{\pi}_{s,t}^c,0\right\}={\lambda}_t\left(1-{\lambda}_t\right)\left({\pi}_{s,t}^c-{\pi}_n\right)k. $$

(44)

Notice that the resulting expression in (44) corresponds precisely to the replicator dynamic in (17). Moreover, it follows that $\left(1-{\lambda}_t\right){\lambda}_t kMax\left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}=0$ and ${\lambda}_t\left(1-{\lambda}_t\right) kMax\left\{{\pi}_n-{\pi}_{s,t}^c,0\right\}>0$ if ${\pi}_n>{\pi}_{s,t}^c$, and that $\left(1-{\lambda}_t\right){\lambda}_t kMax\left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}>0$ and ${\lambda}_t\left(1-{\lambda}_t\right) kMax\left\{{\pi}_n-{\pi}_{s,t}^c,0\right\}=0$ if ${\pi}_{s,t}^c>{\pi}_n$. Therefore, the specification in (44) describes an evolutionary dynamic characterized only by inflow of firms to the strategy yielding the highest net profit rate. Also, this inflow varies positively with the excess of the net profit rate of the respective more profitable strategy over the net profit rate of the alternative strategy.

Let us now describe the flows of capital across productivity-enhancing strategies that go along with the flow of firms across these strategies, recalling there is neither capital mobility across firms nor depreciation of individual capital stocks. Let us consider that the distribution of the aggregate capital stock across strategies in a certain period t, (K_{s, t}, K_{n, t}), is given, with K_t = K_{s, t} + K_{n, t}. Considering (9), the capital stock of the subpopulation of type n firms will have grown at a rate given by γkπ_n = γk(1 − v) ≡ g_n in period t + 1, whereas per (11) the capital stock of the subpopulation of type s firms will have grown at a rate represented by $\gamma k{\pi}_{s,t}^c=\gamma k\left(1-\delta \right)\left(1-\frac{v}{\alpha_t}\right)\equiv {g}_s^c\left({\alpha}_t\right)$. Thus, by the end of period t (and before that any flows across strategies will have materialized), the capital stocks of the subpopulations of type n and type s firms are respectively the following:

$$ \left(1+{g}_n\right){K}_{n,t}; $$

(45)

and:

$$ \left[1+{g}_s^c\left({\alpha}_t\right)\right]{K}_{s,t}. $$

(46)

The capital stock in the subpopulation of type s firms in period t + 1 is represented by the proportion of firms that have remained following the profit-sharing strategy across periods t and t + 1 multiplied by their capital stock in period t + 1, plus the proportion of type n firms in period t that have become of type s in period t + 1 multiplied by their capital stock in period t + 1. Using (42), (43), (45) and (46), the capital stock of the subpopulation of type s firms in period t + 1 is then formally represented by:

$$ {\displaystyle \begin{array}{c}{K}_{s,t+1}=\left[1-{\lambda}_t\left(1-{\lambda}_t\right) kMax\left\{{\pi}_n-{\pi}_{s,t}^c,0\right\}\right]\left[1+{g}_s^c\left({\alpha}_t\right)\right]{K}_{s,t}+\left[\left(1-{\lambda}_t\right){\lambda}_t kMax\left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}\right]\left(1+{g}_n\right){K}_{n,t}\\ {}=\left[1+{g}_s^c\left({\alpha}_t\right)\right]{K}_{s,t}+{\lambda}_t\left(1-{\lambda}_t\right) kMax\left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}\left[\left[1+{g}_s^c\left({\alpha}_t\right)\right]{K}_{s,t}+\left(1+{g}_n\right){K}_{n,t}\right].\end{array}} $$

(47)

Analogously, the capital stock in the subpopulation of type n firms in period t+1 is formally given by:

$$ {\displaystyle \begin{array}{c}{K}_{n,t+1}=\left[1-\left(1-{\lambda}_t\right){\lambda}_t kMax\left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}\right]\left(1+{g}_n\right){K}_{n,t}+\left[{\lambda}_t\left(1-{\lambda}_t\right) kMax\left\{{\pi}_n-{\pi}_{s,t}^c,0\right\}\right]\left[1+{g}_s^c\left({\alpha}_t\right)\right]{K}_{s,t}\\ {}=\left(1+{g}_n\right){K}_{n,t}-{\lambda}_t\left(1-{\lambda}_t\right) kMax\left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}\left[\left[1+{g}_s^c\left({\alpha}_t\right)\right]{K}_{s,t}+\left(1+{g}_n\right){K}_{n,t}\right].\end{array}} $$

(48)

Dividing both sides in (47) and (48) by N_t + 1 and rearranging, we obtain:

$$ {\kappa}_{s,t+1}=\frac{1+{g}_s^c\left({\alpha}_t\right)}{1+g\left({\alpha}_t,{\lambda}_t\right)}{\kappa}_{s,t}+{\lambda}_t\left(1-{\lambda}_t\right)\kern0.2em kMax\kern0.2em \left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}\left[\frac{\left[1+{g}_s^c\left({\alpha}_t\right)\right]{\kappa}_{s,t}+\left(1+{g}_n\right){\kappa}_{n,t}}{1+g\left({\alpha}_t,{\lambda}_t\right)}\right], $$

(49)

and:

$$ {\kappa}_{n,t+1}=\frac{1+{g}_n}{1+g\left({\alpha}_t,{\lambda}_t\right)}{\kappa}_{n,t}-{\lambda}_t\left(1-{\lambda}_t\right)\kern0.2em kMax\kern0.2em \left\{{\pi}_{s,t}^c-{\pi}_n,0\right\}\left[\frac{\left[1+{g}_s^c\left({\alpha}_t\right)\right]{\kappa}_{s,t}+\left(1+{g}_n\right){\kappa}_{n,t}}{1+g\left({\alpha}_t,{\lambda}_t\right)}\right], $$

(50)

where κ_{i, t} = K_{i, t} / N_t denotes the total amount of capital of firms of type i = s, n as a proportion of the supply of labor in period t. In arriving at (49) and (50), we have made use of our assumption that N_t + 1 = [1 + g(α_t, λ_t)]N_t, where it is to be remembered that g(α_t, λ_t) is the short-run equilibrium average output growth rate defined in (14).

As demonstrated in Appendix 1, and summarized in Propositions 1 and 2, the coevolution of the frequency distribution of productivity-enhancing strategies and labor productivity across firms takes the economy to one of three evolutionary equilibria. Each one of these evolutionary equilibria features a different configuration in terms of the total amount of capital owned by firms of type i = s, n as a proportion of the supply of labor. If $0<\delta <\underline{\delta}$, the economy converges to the monomorphic equilibrium represented by ${E}_1=\left(\overline{\alpha},0\right)=\left(\frac{\beta - v\delta}{1-\delta },0\right)\in \Theta$, which features no firm following the profit-sharing compensation strategy. Since the average output growth rate is $g\left(\overline{\alpha},0\right)={g}_n=\gamma k\left(1-v\right)$, it follows from (49)-(50) that κ_s,t + 1 = κ_s,t = 0 and ${\kappa}_{n,t+1}={\kappa}_{n,t}={\overline{\kappa}}_s>0$ for all t ∈ {t₀, t₀ + 1, t₀ + 2, …} when the economy is at the monomorphic equilibrium E₁ in period t₀. If $\overline{\delta}<\delta <1$, the economy converges to the monomorphic equilibrium represented by ${E}_2=\left(\overset{\sim }{\alpha },1\right)=\left(\beta, 1\right)\in \Theta$, which is characterized by all firms following the profit-sharing compensation strategy. Given that the average output growth rate is represented by $g\left(\overset{\sim }{\alpha },1\right)={g}_s^c\left(\beta, 1\right)=\gamma k\left(1-\delta \right)\left(1-\frac{v}{\beta}\right)$, it results from (49)-(50) that ${\kappa}_{s,t+1}={\kappa}_{s,t}={\overset{\sim }{\kappa}}_s>0$ and κ_{n, t + 1} = κ_{n, t} = 0 for all t ∈ {t₀, t₀ + 1, t₀ + 2, …} when the economy is at the monomorphic equilibrium E₂ in period t₀. Meanwhile, if $\underline{\delta}<\delta <\overline{\delta}$, the economy converges to the polymorphic equilibrium represented by ${E}_3=\left({\alpha}^{\ast },{\lambda}^{\ast}\right)=\left({\alpha}^{\ast },\frac{\beta - v\delta -\left(1-\delta \right){\alpha}^{\ast }}{\delta \left({\alpha}^{\ast }-v\right)}\right)\in \Theta$, at which the average output growth rate is given by $g\left(\overline{\alpha},0\right)={g}_n=\gamma k\left(1-v\right)$. Therefore, it follows from (49)-(50) that ${\overset{\frown }{\kappa}}_{s,t+1}={\overset{\frown }{\kappa}}_{s,t}={\kappa}_s^{\ast }>0$ and ${\overset{\frown }{\kappa}}_{n,t+1}={\overset{\frown }{\kappa}}_{n,t}={\kappa}_n^{\ast }>0$ for all t ∈ {t₀, t₀ + 1, t₀ + 2, …} when the economy is at the polymorphic equilibrium E₃ in period t₀.