Modeling of elastoplastic behavior of freestanding square thin films under bulge testing

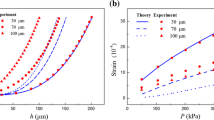
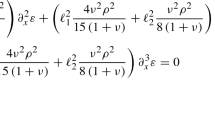Abstract

Bulge testing is an experimental technique applied to the characterization of thin films, with which the mechanical properties are obtained employing numerical and analytical approaches. For rectangular and circular thin films, classical methods have been well adopted to calculate the stresses and strains at the central point without the dependency of its properties. It is due to that the equilibrium conditions are dependent on the curvatures generated at the maximum deflection point. Therefore, the kinematics of the bulged surface can be represented by a spherical cap and cylindrical shapes in these films. For square thin films, the deflected surface presents a complex displacement field that implies the development of analytical models for the estimation of these. In this sense, equations to represent the equibiaxial stress state (central point) for freestanding square thin films are developed and applied in this study. The curvatures are determined using a reviewed nonlinear deflection field. Virtual experiments were driven by applying finite element analysis to validate the stress and strain models through parametric analysis. In order to impose the residual stress in thin films, two approaches of modeling for the simulations are described. In order to validate the applicability of the proposed equations, different cases were presented for the linear elastic analysis, and two examples were developed to characterize elastoplastic behavior (bilinear and nonlinear) from load–deflection curves. The obtained results show that the developed models for square thin films are in good agreement with the finite element simulations since these predicted the stress and strain states with good accuracy.

Appendix A

The strain functions depend on the rotations (first-order derivation) that are obtained as follows:

$$\frac{\partial w(x,y)}{{\partial x}} = \left( {\frac{{2w_{2} xy^{2} }}{{a^{4} }} + \frac{{2w_{1} x}}{{a^{2} }}} \right)\cos \left( {\frac{\pi x}{{2a}}} \right)\cos \left( {\frac{\pi y}{{2a}}} \right) - \frac{\pi }{2a}\sin \left( {\frac{\pi x}{{2a}}} \right)\cos \left( {\frac{\pi y}{{2a}}} \right)\left( {\frac{{w_{2} x^{2} y^{2} }}{{a^{4} }} + \frac{{w_{1} \left( {x^{2} + y^{2} } \right)}}{{a^{2} }} + w_{0} } \right).$$

(A1)

$$\frac{\partial w(x,y)}{{\partial y}} = \left( {\frac{{2w_{2} x^{2} y}}{{a^{4} }} + \frac{{2w_{1} y}}{{a^{2} }}} \right)\cos \left( {\frac{\pi x}{{2a}}} \right)\cos \left( {\frac{\pi y}{{2a}}} \right) - \frac{\pi }{2a}\cos \left( {\frac{\pi x}{{2a}}} \right)\sin \left( {\frac{\pi y}{{2a}}} \right)\left( {\frac{{w_{2} x^{2} y^{2} }}{{a^{4} }} + \frac{{w_{1} \left( {x^{2} + y^{2} } \right)}}{{a^{2} }} + w_{0} } \right).$$

(A2)

Also, the curvatures (second-order derivation) are computed for the $x$-direction:

$$\begin{aligned} \frac{{\partial w^{2} (x,y)}}{{\partial x^{2} }} & = - \frac{{\pi^{2} }}{{4a^{2} }}\cos \left( {\frac{\pi x}{{2a}}} \right)\cos \left( {\frac{\pi y}{{2a}}} \right)\left( {w_{0} + w_{1} \frac{{(x^{2} + y^{2} )}}{{a^{2} }} + w_{2} \frac{{x^{2} y^{2} }}{{a^{4} }}} \right) + \left( {\frac{{2w_{2} y^{2} }}{{a^{4} }} + \frac{{2w_{1} }}{{a^{2} }}} \right)\cos \left( {\frac{\pi x}{{2a}}} \right)\cos \left( {\frac{\pi y}{{2a}}} \right) \\ & \quad - \frac{\pi }{a}\cos \left( {\frac{\pi y}{{2a}}} \right)\sin \left( {\frac{\pi x}{{2a}}} \right)\left( {\frac{{2w_{2} xy^{2} }}{{a^{4} }} + \frac{{2w_{1} x}}{{a^{2} }}} \right). \\ \end{aligned}$$

(A3)

and for the $y$-direction:

$$\begin{aligned} \frac{{\partial w^{2} (x,y)}}{{\partial y^{2} }} & = - \frac{{\pi^{2} }}{{4a^{2} }}\cos \left( {\frac{\pi x}{{2a}}} \right)\cos \left( {\frac{\pi y}{{2a}}} \right)\left( {w_{0} + w_{1} \frac{{(x^{2} + y^{2} )}}{{a^{2} }} + w_{2} \frac{{x^{2} y^{2} }}{{a^{4} }}} \right) + \left( {\frac{{2w_{2} x^{2} }}{{a^{4} }} + \frac{{2w_{1} }}{{a^{2} }}} \right)\cos \left( {\frac{\pi x}{{2a}}} \right)\cos \left( {\frac{\pi y}{{2a}}} \right) \\ & \quad -\frac{\pi }{a}\cos \left( {\frac{\pi x}{{2a}}} \right)\sin \left( {\frac{\pi y}{{2a}}} \right)\left( {\frac{{2w_{2} x^{2} y}}{{a^{4} }} + \frac{{2w_{1} y}}{{a^{2} }}} \right). \\ \end{aligned}$$

(A4)

For the $x - y$ plane, the mixed curvature is determined as:

$$\begin{aligned} \frac{{\partial w^{2} (x,y)}}{\partial x\partial y} & = - \frac{4}{{a^{4} }}w_{2} xy\cos \left( {\frac{\pi x}{{2a}}} \right)\cos \left( {\frac{\pi y}{{2a}}} \right) + \pi^{2} \sin \left( {\frac{\pi x}{{2a}}} \right)\sin \left( {\frac{\pi y}{{2a}}} \right)\left( {\frac{{w_{2} x^{2} y^{2} }}{{a^{4} }} + \frac{{w_{1} \left( {x^{2} + y^{2} } \right)}}{{a^{2} }} + w_{0} } \right) \\ & \quad - \frac{\pi }{2a}\sin \left( {\frac{\pi x}{{2a}}} \right)\cos \left( {\frac{\pi y}{{2a}}} \right)\left( {\frac{{2w_{2} x^{2} y}}{{a^{4} }} + \frac{{2w_{1} y}}{{a^{2} }}} \right) - \frac{\pi }{2a}\cos \left( {\frac{\pi x}{{2a}}} \right)\sin \left( {\frac{\pi y}{{2a}}} \right)\left( {\frac{{2w_{2} xy^{2} }}{{a^{4} }} + \frac{{2w_{1} x}}{{a^{2} }}} \right). \\ \end{aligned}$$

(A5)

To determine a set of kinematic equations in the x-direction, the derivatives should be evaluated at $y = 0$ such that

$$\frac{\partial w(x,0)}{{\partial x}} = \left( {\frac{{2w_{1} x}}{{a^{2} }}} \right)\cos \left( {\frac{\pi x}{{2a}}} \right) - \frac{\pi }{2a}\sin \left( {\frac{\pi x}{{2a}}} \right)\left( {\frac{{x^{2} }}{{a^{2} }} + w_{0} } \right),$$

(A6)

$$\frac{{\partial w^{2} (x,0)}}{{\partial x^{2} }} = - \frac{{\pi^{2} }}{{4a^{2} }}\cos \left( {\frac{\pi x}{{2a}}} \right)\left( {w_{0} + w_{1} \frac{{x^{2} }}{{a^{2} }}} \right) + \left( {\frac{{2w_{1} }}{{a^{2} }}} \right)\cos \left( {\frac{\pi x}{{2a}}} \right) - \frac{\pi }{a}\sin \left( {\frac{\pi x}{{2a}}} \right)\left( {\frac{{2w_{1} x}}{{a^{2} }}} \right),$$

(A7)

$$\frac{{\partial w^{3} (x,0)}}{{\partial x^{3} }} = - \frac{{w_{1} x\pi^{2} }}{{a^{4} }}\cos \left( {\frac{\pi x}{{2a}}} \right) + \left( {\frac{{\pi^{3} }}{{8a^{3} }}w_{0} - w_{1} \frac{3\pi }{{a^{3} }} + w_{1} \frac{{\pi^{3} x^{2} }}{{8a^{5} }}} \right)\sin \left( {\frac{\pi x}{{2a}}} \right),$$

(A8)

$$\frac{{\partial w^{4} (x,0)}}{{\partial x^{4} }} = - w_{1} \frac{{3x\pi^{3} }}{{4a^{5} }}\sin \left( {\frac{\pi x}{{2a}}} \right) + \left( {\frac{{\pi^{4} }}{{16a^{4} }}w_{0} - w_{1} \frac{{5\pi^{2} }}{{2a^{4} }} + w_{1} \frac{{\pi^{4} x^{2} }}{{16a^{6} }}} \right)\cos \left( {\frac{\pi x}{{2a}}} \right).$$

(A9)