Small Permanent Charge Effects on Individual Fluxes via Poisson–Nernst–Planck Models with Multiple Cations

Abstract

A quasi-one-dimensional Poisson–Nernst–Planck system for ionic flow through a membrane channel is studied. Nonzero but small permanent charge, the major structural quantity of an ion channel, is included in the model. The system includes three ion species, two cations with the same valences and one anion, which provides more correlations/interactions between ions compared to the case included only two oppositely charged particles. The cross-section area of the channel is included in the system, which provides certain information of the geometry of the three-dimensional channel. This is crucial for our analysis. Under the framework of geometric singular perturbation theory, more importantly, the specific structure of the model, the existence and local uniqueness of solutions to the system for small permanent charges is established. Furthermore, treating the permanent charge as a small parameter, through regular perturbation analysis, we are able to derive approximations of the individual fluxes explicitly, and this allows us to examine the small permanent charge effects on ionic flows in detail. Of particular interest is the competition between two cations, which is related to the selectivity phenomena of ion channels. Critical potentials are identified and their roles in characterizing ionic flow properties are studied. Some critical potentials can be estimated experimentally, and this provides an efficient way to adjust/control boundary conditions (electric potential and concentrations) to observe distinct qualitative properties of ionic flows. Mathematical analysis further indicates that to optimize the effect of permanent charges, a short and narrow filter, within which the permanent charge is confined, is expected, which is consistent with the typical structure of an ion channel.

Appendix: Proofs of Some Results

1.1 Proof of Proposition 2.3

We will provide a detailed proof for statement (i), and the second statement can be argued in a similar way. To get started, we assume

$$\begin{aligned} z(\xi )=(\phi (\xi ), u(\xi ), c_1(\xi ), c_2(\xi ), c_3(\xi ), J_1(\xi ), J_2(\xi ), J_3(\xi ), \tau (\xi )) \end{aligned}$$

is a solution of the limiting fast system (2.3) from $B_{j-1}$ to ${{\mathcal {Z}}}_j$; namely, $z(\xi )\in N^{[j-1,r]}=M^{[j-1,r]}\cap W^s({{\mathcal {Z}}}_j)$. It follows that $J_1(\xi ), J_2(\xi ), J_3(\xi )$ are constants and $\tau (\xi )=x_{j-1}$. Notice that $z(0)\in B_{j-1}$ and $\lim _{\xi \rightarrow +\infty }z(\xi )=z(+\infty )\in {{\mathcal {Z}}}_j.$ One has $\phi (0)=\phi ^{[j-1]},\ c_k(0)=c_k^{[j-1]}, \ u(+\infty )=0,$ and $z_1c_1(+\infty )+z_2c_2(+\infty )+z_3c_3(+\infty )+Q_j=0.$ Define $u(0)=u^{[j-1,r]}$. By the integrals in Proposition 2.2, we get

$$\begin{aligned} \ln c_k(\xi )+z_k\phi (\xi )=\ln c_k^{[j-1]}+z_k\phi ^{[j-1]}. \end{aligned}$$

Hence,

$$\begin{aligned} c_k(\xi )=c_k^{[j-1]}e^{-z_k\big (\phi (\xi )-\phi ^{[j-1]}\big )}. \end{aligned}$$

(6.1)

Now the first two equations in the limiting fast system (2.3) read

$$\begin{aligned} \begin{aligned} \phi '=&u,\quad u'=-\sum _{k=1}^3z_kc_k^{[j-1]}e^{-z_k\big (\phi -\phi ^{[j-1]}\big )}-Q_j, \end{aligned} \end{aligned}$$

(6.2)

which is a Hamiltonian system with a Hamiltonian function given by

$$\begin{aligned} H(\phi , u)=&\frac{1}{2}u^2-\sum _{k=1}^3c_k^{[j-1]}e^{-z_k\big (\phi -\phi ^{[j-1]}\big )}+Q_j\phi . \end{aligned}$$

Not difficult to see that the above Hamiltonian function is exactly the integral $H_4$ in Proposition 2.2 with the relation (6.1). The equilibria of (6.2) are given by

$$\begin{aligned} u=0,\quad \sum _{k=1}^3z_kc_k^{[j-1]}e^{-z_k\big (\phi -\phi ^{[j-1]}\big )}+Q_j=0. \end{aligned}$$

(6.3)

We now claim that $\phi ^{[j-1,r]}$ is the unique solution of the second equation in (6.3). To get started, we let

$$\begin{aligned} f(\phi )=\sum _{k=1}^3z_kc_k^{[j-1]}e^{z_k\big (\phi ^{[j-1]}-\phi \big )}+Q_j. \end{aligned}$$

(6.4)

It is easy to see that $f'(\phi )=-\sum _{k=1}^3z_k^2c_k^{[j-1]}e^{z_k\big (\phi ^{[j-1]}-\phi \big )}<0,$ which implies that $f(\phi )$ is a decreasing function. Note that in our set-up, $z_1>0,\ z_2>0,\ z_3<0$ and $c_k^{[j-1]}$’s are positive, one has $f(\phi )\rightarrow -\infty$ as $\phi \rightarrow +\infty$ and $f(\phi )\rightarrow +\infty$ as $\phi \rightarrow -\infty$. Correspondingly, (6.3) has a unique solution.

Let $c_k(+\infty )=c_k^{[j-1,r]}$, then, from (6.1), one has $c_k^{[j-1,r]}=c_k^{[j-1]}e^{-z_k\big (\phi ^{[j-1,r]}-\phi ^{[j-1]}\big )}.$ Evaluating the integral $H_4$ in Proposition 2.2 at $\xi =0$ and $\xi \rightarrow +\infty$, we have

$$\begin{aligned} \frac{1}{2}u^2(0)-\sum _{k=1}^3c_k^{[j-1]}+Q_j\phi ^{[j-1]}=- \sum _{k=1}^3c_k^{[j-1]}e^{-z_k\big (\phi ^{[j-1,r]}-\phi ^{[j-1]}\big )}+Q_j\phi ^{[j-1,r]}, \end{aligned}$$

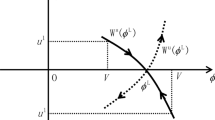
which gives the expression for $u^{[j-1,r]}$. The choice of the sign can be determined from the phase portrait sketched in Fig. 2.

Fig. 2

The phase portrait for the Hamiltonian system (6.2). The sign of $u^{[j-1,r]}$ agrees with the sign of $\phi ^{[j-1,r]}-\phi ^{[j-1]}$

We now claim that the expressions under the square root in $u^{[j-1,r]}$ and $u^{[j,l]}$ are non-negative. We just provide the proof for the expression in $u^{[j-1,r]}$. Let

$$\begin{aligned} F(\phi )=\sum _{k=1}^3c_k^{[j-1]}\big (1-e^{z_k(\phi ^{[j-1]}-\phi )} \big )-Q_j\big (\phi ^{[j-1]}-\phi \big ). \end{aligned}$$

Notice that $F'(\phi )=f(\phi )$ and $F''(\phi )=f'(\phi )$ where $f(\phi )$ is defined in (6.4). Since $f'(\phi )<0$, one has $F(\phi )$ is concave down. Together with $F'(\phi ^{[j-1,r]})=f(\phi ^{[j-1,r]})=0$, one has $F(\phi ^{[j-1,r]})$ is the unique maximal value of $F(\phi )$, and in particular, $F(\phi ^{[j-1,r]})\ge F(\phi ^{[j-1]})=0.$

Finally, we consider the transversal intersection of the stable manifold $W^s(\mathcal {Z}_j)$ and $B_{j-1}$ at points $\big (\phi ^{[j-1]}, u^{[j-1,r]}, c_1^{[j-1]}, c_2^{[j-1]}, c_3^{[j-1]}, J_1, J_2, J_3, x_{j-1}\big ).$ From the above argument, they do intersect at the specified points, and one only need to verify the intersection is transversal. Since the stable manifold is completely characterized, one can compute its tangent space at each intersection point (via the complete set of first integrals obtained in Proposition 2.2) to verify the transversality of the intersection. It is slightly complicated but straightforward. We would like to omit the detail here. This completes the proof.

1.2 Proof of Proposition 2.8

Plugging (2.27) into (2.18), the zeroth-order system in $Q_0$ reads

$$\begin{aligned} 0&=zc_{10}^{[1]}e^{z(\phi _0^{[1]}-\phi _0^{[1,r]})}+zc_{20}^{[1]} e^{z(\phi _0^{[1]}-\phi _0^{[1,r]})}+z_3c_{30}^{[1]}e^{z_3(\phi _0^{[1]} -\phi _0^{[1,r]})},\nonumber \\ 0&=zc_{10}^{[2]}e^{z(\phi _0^{[2]}-\phi _0^{[2,l]})}+zc_{20}^{[2]}e^{z(\phi _0^{[2]}- \phi _0^{[2,l]})}+z_3c_{30}^{[2]}e^{z_3(\phi _0^{[2]}-\phi _0^{[2,l]})},\nonumber \\ 0&=C_0^{[1]}\Big (e^{z(\phi _0^{[1]}-\phi _0^{[1,r]})}-e^{z(\phi _0^{[1]}-\phi _0^{[1,l]})} \Big )+c_{30}^{[1]}\Big (e^{z_3(\phi _0^{[1]}-\phi _0^{[1,r]})}-e^{z_3(\phi _0^{[1]} -\phi _0^{[1,l]})}\Big ),\nonumber \\ 0&=C_0^{[2]}\Big (e^{z(\phi _0^{[2]}-\phi _0^{[2,r]})}-e^{z(\phi _0^{[2]}-\phi _0^{[2,l]})} \Big )+c_{30}^{[2]}\Big (e^{z_3(\phi _0^{[2]}-\phi _0^{[2,r]})}-e^{z_3(\phi _0^{[2]} -\phi _0^{[2,l]})}\Big ),\nonumber \\ J_{10}&=\frac{C^{[0,r]}-C_0^{[1,l]}}{\ln C^{[0,r]}-\ln C_0^{[1,l]}}\cdot \frac{\ln C^{[0,r]}-\ln C_0^{[1,l]}e^{z(\phi _0^{[1,l]}-\phi ^{[0,r]})}}{C^{[0,r]}-C_0^{[1,l]} e^{z(\phi _0^{[1,l]}-\phi ^{[0,r]})}}\nonumber \\&\quad \cdot \frac{c_1^{[0,r]}-c_{10}^{[1,l]}e^{z(\phi _0^{[1,l]}-\phi ^{[0,r]})}}{H(x_1)}\nonumber \\&=\frac{C_0^{[2,r]}-C^{[3,l]}}{\ln C_0^{[2,r]}-\ln C^{[3,l]}}\cdot \frac{\ln C_0^{[2,r]}-\ln C^{[3,l]}e^{z(\phi ^{[3,l]}-\phi _0^{[2,r]})}}{C_0^{[2,r]}-C^{[3,l]} e^{z(\phi ^{[3,l]}-\phi _0^{[2,r]})}}\nonumber \\&\quad \cdot \frac{c_{10}^{[2,r]}-c_1^{[3,l]}e^{z(\phi ^{[3,l]}-\phi _0^{[2,r]})}}{H(1)-H(x_2)},\nonumber \\ J_{20}&=\frac{C^{[0,r]}-C_0^{[1,l]}}{\ln C^{[0,r]}-\ln C_0^{[1,l]}}\cdot \frac{\ln C^{[0,r]}-\ln C_0^{[1,l]}e^{z(\phi _0^{[1,l]}-\phi ^{[0,r]})}}{C^{[0,r]}-C_0^{[1,l]} e^{z(\phi _0^{[1,l]}-\phi ^{[0,r]})}}\nonumber \\&\quad \cdot \frac{c_2^{[0,r]}-c_{20}^{[1,l]}e^{z(\phi _0^{[1,l]}-\phi ^{[0,r]})}}{H(x_1)}\nonumber \\&=\frac{C_0^{[2,r]}-C^{[3,l]}}{\ln C_0^{[2,r]}-\ln C^{[3,l]}}\cdot \frac{\ln C_0^{[2,r]}-\ln C^{[3,l]}e^{z(\phi ^{[3,l]}-\phi _0^{[2,r]})}}{C_0^{[2,r]}-C^{[3,l]} e^{z(\phi ^{[3,l]}-\phi _0^{[2,r]})}}\nonumber \\&\quad \cdot \frac{c_{20}^{[2,r]}-c_2^{[3,l]}e^{z(\phi ^{[3,l]} -\phi _0^{[2,r]})}}{H(1)-H(x_2)},\nonumber \\ J_{30}&=-\frac{z}{z_3}\frac{C^{[0,r]}-C_0^{[1,l]}}{\ln C^{[0,r]}-\ln C_0^{[1,l]}}\frac{\ln C^{[0,r]}-\ln C_0^{[1,l]}e^{z(\phi _0^{[1,l]}-\phi ^{[0,r]})}}{H(x_1)}\nonumber \\&=-\frac{z}{z_3}\frac{C_0^{[2,r]}-C^{[3,l]}}{\ln C_0^{[2,r]}-\ln C^{[3,l]}} \frac{\ln C_0^{[2,r]}-\ln C^{[3,l]}e^{z(\phi ^{[3,l]}-\phi _0^{[2,r]})}}{H(1)-H(x_2)},\nonumber \\ \phi _0^{[2]}&=\phi _0^{[1]}-T_0^cy_{00}, \quad c_{10}^{[2,l]}=\frac{J_{20}c_{10}^{[1,r]}-J_{10}c_{20}^{[1,r]}}{J_{10} +J_{20}}e^{zT_0^cy_{00}}+\frac{J_{10}C_0^{[1,r]}}{J_{10}+J_{20}}e^{zz_3T_0^my_{00}},\nonumber \\ c_{20}^{[2,l]}&=\frac{J_{10}c_{20}^{[1,r]}-J_{20}c_{10}^{[1,r]}}{J_{10} +J_{20}}e^{zT_0^cy_{00}}+\frac{J_{20}C_0^{[1,r]}}{J_{10}+J_{20}}e^{zz_3T_0^my_{00}},\nonumber \\ T_0^m&=\frac{z_3-z}{z_3}\frac{C_0^{[1]}-C_0^{[2]}}{H(x_2)-H(x_1)}. \end{aligned}$$

(6.5)

Recall that on $\mathcal {Z}_j$, one has $z_1c_1+z_2c_2+z_3c_3+Q_j=0$. Plugging (2.27) into it, the zeroth-order terms in $Q_0$ gives

$$\begin{aligned} c_{30}^{[1]}=-\frac{z}{z_{3}}C_0^{[1]},\quad c_{30}^{[2]}=-\frac{z}{z_{3}}C_0^{[2]}. \end{aligned}$$

(6.6)

Plugging (6.6) into the first two equations of (6.5) gives

$$\begin{aligned} \begin{aligned} \phi _{0}^{[1]}=\phi _0^{[1,r]}\quad \text{ and }\quad \phi _{0}^{[2]}=\phi _0^{[2,l]}. \end{aligned} \end{aligned}$$

From (2.19), one then has

$$\begin{aligned} \begin{aligned} \phi ^{[0,r]}&=V-\frac{1}{z-z_3}\ln \frac{-z_3L_3}{zL},\ c_{1}^{[0,r]}=L_1\Big (\frac{-z_3L_3}{zL}\Big )^{\frac{z}{z-z_3}},\ c_2^{[0,r]}=L_2\Big (\frac{-z_3L_3}{zL}\Big )^{\frac{z}{z-z_3}},\\ c_3^{[0,r]}&=L_3\Big (\frac{-z_3L_3}{zL}\Big )^{\frac{z_3}{z-z_3}},\ \phi ^{[3,l]}=-\frac{1}{z-z_3}\ln \frac{-z_3R_3}{zR},\ c_{1}^{[3,l]}=R_1\Big (\frac{-z_3R_3}{zR}\Big )^{\frac{z}{z-z_3}},\\ c_2^{[3,l]}&=R_2\Big (\frac{-z_3R_3}{zR}\Big )^{\frac{z}{z-z_3}},\ c_3^{[3,l]}=R_3\Big (\frac{-z_3R_3}{zR}\Big )^{\frac{z_3}{z-z_3}},\\ \phi _{0}^{[1,l]}&=\phi _{0}^{[1,r]}=\phi _{0}^{[1]}, \ \phi _{0}^{[2,l]}=\phi _{0}^{[2,r]}=\phi _{0}^{[2]},\ c_{k0}^{[1,l]}=c_{k0}^{[1,r]}=c_{k0}^{[1]}, \ c_{k0}^{[2,l]}=c_{k0}^{[2,r]}=c_{k0}^{[2]}, \end{aligned} \end{aligned}$$

for $k=1,2,3$, and further, from (6.5), we have

$$\begin{aligned} \begin{aligned} J_{10}&=\frac{C^{[0,r]}-C_0^{[1]}}{\ln C^{[0,r]}-\ln C_0^{[1]}}\frac{\ln C^{[0,r]}-\ln C_0^{[1]}e^{z(\phi _0^{[1]}-\phi ^{[0,r]})}}{C^{[0,r]}-C_0^{[1]}e^{z(\phi _0^{[1]} -\phi ^{[0,r]})}}\frac{c_1^{[0,r]}-c_{10}^{[1]}e^{z(\phi _0^{[1]} -\phi ^{[0,r]})}}{H(x_1)}\\&=\frac{C_0^{[2]}-C^{[3,l]}}{\ln C_0^{[2]}-\ln C^{[3,l]}}\frac{\ln C_0^{[2]}- \ln C^{[3,l]}e^{z(\phi ^{[3,l]}-\phi _0^{[2]})}}{C_0^{[2]}-C^{[3,l]}e^{z(\phi ^{[3,l]} -\phi _0^{[2]})}}\frac{c_{10}^{[2]}-c_1^{[3,l]}e^{z(\phi ^{[3,l]} -\phi _0^{[2]})}}{H(1)-H(x_2)},\\ J_{20}&=\frac{C^{[0,r]}-C_0^{[1]}}{\ln C^{[0,r]}-\ln C_0^{[1]}}\frac{\ln C^{[0,r]}-\ln C_0^{[1]}e^{z(\phi _0^{[1]}-\phi ^{[0,r]})}}{C^{[0,r]}-C_0^{[1]}e^{z(\phi _0^{[1]} -\phi ^{[0,r]})}}\frac{c_2^{[0,r]}-c_{20}^{[1]}e^{z(\phi _0^{[1]}- \phi ^{[0,r]})}}{H(x_1)}\\&=\frac{C_0^{[2]}-C^{[3,l]}}{\ln C_0^{[2]}-\ln C^{[3,l]}}\frac{\ln C_0^{[2]}-\ln C^{[3,l]}e^{z(\phi ^{[3,l]}-\phi _0^{[2]})}}{C_0^{[2]}-C^{[3,l]}e^{z(\phi ^{[3,l]} -\phi _0^{[2]})}}\frac{c_{20}^{[2]}-c_2^{[3,l]}e^{z(\phi ^{[3,l]}- \phi _0^{[2]})}}{H(1)-H(x_2)},\\ J_{30}&=-\frac{z}{z_3}\frac{C^{[0,r]}-C_0^{[1]}}{\ln C^{[0,r]}-\ln C_0^{[1]}}\frac{\ln C^{[0,r]}-\ln C_0^{[1]}e^{z(\phi _0^{[1]}-\phi ^{[0,r]})}}{H(x_1)}\\&=-\frac{z}{z_3}\frac{C_0^{[2]}-C^{[3,l]}}{\ln C_0^{[2]}-\ln C^{[3,l]}}\frac{\ln C_0^{[2]}-\ln C^{[3,l]}e^{z(\phi ^{[3,l]}-\phi _0^{[2]})}}{H(1)-H(x_2)},\\ \phi _0^{[2]}&=\phi _0^{[1]}-T_0^cy_{00},\ \quad C_0^{[2]}=C_0^{[1]}e^{zz_{3}T_0^my_{00}},\\&J_{10}\left( C_0^{[2]}-C_0^{[1]}e^{zT_0^cy_{00}}\right) =(J_{10}+J_{20}) \left( c_{10}^{[2]}-c_{10}^{[1]}e^{zT_0^cy_{00}}\right) ,\\ T_0^m&=\frac{z_3-z}{z_3}\frac{C_0^{[1]}-C_0^{[2]}}{H(x_2)-H(x_1)}. \end{aligned} \end{aligned}$$

(6.7)

Adding $J_{10},\ J_{20}$ and $J_{30}$ in (6.7), together with the last equation in (6.7), one has

$$\begin{aligned} \begin{aligned}&T_0^m=\frac{z_3-z}{z_3}\frac{C^{[0,r]}-C_0^{[1]}}{H(x_1)}=\frac{z_3-z}{z_3} \frac{C_0^{[2]}-C^{[3,l]}}{H(x_2)-H(x_1)}=\frac{z_3-z}{z_3}\frac{C_0^{[1]}-C_0^{[2]}}{H(x_2)-H(x_1)}, \end{aligned} \end{aligned}$$

(6.8)

from which

$$\begin{aligned} \begin{aligned}&\frac{C^{[0,r]}-C_0^{[1]}}{H(x_1)}=\frac{C_0^{[2]}-C^{[3,l]}}{H(x_2)-H(x_1)} =\frac{C_0^{[1]}-C_0^{[2]}}{H(x_2)-H(x_1)}=\frac{C^{[0,r]}-C^{[3,l]}}{H(1)}, \end{aligned} \end{aligned}$$

(6.9)

which implies

$$\begin{aligned} C_0^{[1]}=(1-\alpha )C^{[0,r]}+\alpha C^{[3,l]}, \ C_0^{[2]}=(1-\beta )C^{[0,r]}+\beta C^{[3,l]} \end{aligned}$$

and

$$\begin{aligned} T_0^m=\frac{z_3-z}{z_3}\frac{C^{[0,r]}-C^{[3,l]}}{H(1)}. \end{aligned}$$

It follows from the fifth equation in (6.7) that

$$\begin{aligned} \begin{aligned}&y_{00}=\frac{H(1)\big (\ln C_0^{[2]}-\ln C_0^{[1]}\big )}{z(z-z_{3})\left( C^{[0,r]}-C^{[3,l]}\right) }. \end{aligned} \end{aligned}$$

(6.10)

Adding the first two equations in (6.7), one has

$$\begin{aligned} \begin{aligned} J_{10}+J_{20}=&\frac{C^{[0,r]}-C_0^{[1]}}{H(x_1)}\bigg (1-\frac{z(\phi _0^{[1]} -\phi _0^{[0]})}{\ln C^{[0,r]}-\ln C_0^{[1]}}\bigg )\\ =&\frac{C_0^{[2]}-C^{[3,l]}}{H(1)-H(x_2)}\bigg (1-\frac{z(\phi ^{[3,l]} -\phi _0^{[2]})}{\ln C_0^{[2]}-\ln C^{[3,l]}} \bigg ). \end{aligned} \end{aligned}$$

(6.11)

Equations (6.9), (6.10), (6.11), together with the fourth equation in (6.7) yield

$$\begin{aligned} \begin{aligned}&\frac{\phi _0^{[1]}-\phi _0^{[0]}}{\ln C_0^{[1]}-\ln C^{[0,r]}} =\frac{\phi ^{[3,l]}-\phi _0^{[2]}}{\ln C^{[3,l]}-\ln C_0^{[2]}}=\frac{\phi _0^{[2]}-\phi _0^{[1]}}{\ln C_0^{[2]}-\ln C_0^{[1]}}=\frac{\phi ^{[3,l]}-\phi _0^{[0]}}{\ln C^{[3,l]}-\ln C^{[0,r]}}. \end{aligned} \end{aligned}$$

(6.12)

It now follows that

$$\begin{aligned} \begin{aligned}&\phi _{0}^{[1]}=\frac{\ln C_0^{[1]}-\ln C^{[3,l]}}{\ln C^{[0,r]} -\ln C^{[3,l]}}\phi ^{[0,r]}+\frac{\ln C^{[0,r]}-\ln C_0^{[1]}}{\ln C^{[0,r]} -\ln C^{[3,l]}}\phi ^{[3,l]},\\&\phi _{0}^{[2]}=\frac{\ln C_0^{[2]}-\ln C^{[3,l]}}{\ln C^{[0,r]} -\ln C^{[3,l]}}\phi ^{[0,r]}+\frac{\ln C^{[0,r]}-\ln C_0^{[2]}}{\ln C^{[0,r]} -\ln C^{[3,l]}}\phi ^{[3,l]}.\\ \end{aligned} \end{aligned}$$

From the first two equations and the penultimate equation in (6.7), one has

$$\begin{aligned} \begin{aligned} \frac{J_{10}}{J_{10}+J_{20}}=&\frac{c_{1}^{[0,r]}-c_{10}^{[1]}e^{z(\phi _0^{[1]} -\phi ^{[0,r]})}}{C^{[0,r]}-C_0^{[1]}e^{z(\phi _0^{[1]}-\phi ^{[0,r]})}}= \frac{c_{10}^{[2]}-c_{1}^{[3,l]}e^{z(\phi ^{[3,l]}-\phi _0^{[2]})}}{C_0^{[2]} -C^{[3,l]}e^{z(\phi ^{[3,l]}-\phi _0^{[2]})}}\\ =&\frac{c_{10}^{[2]}-c_{10}^{[1]}e^{z(\phi _0^{[1]}-\phi _0^{[2]})}}{C_0^{[2]} -C_0^{[1]}e^{z(\phi _0^{[1]}-\phi _0^{[2]})}}, \end{aligned} \end{aligned}$$

from which, one obtains the expressions of $c_{10}^{[1]}, \ c_{20}^{[1]}, \ c_{10}^{[2]}$ and $c_{20}^{[2]}$. The expressions for $J_{10}$ and $J_{20}$ then follow. This completes the proof.

1.3 Proof of Lemma 3.5

$N>0$ follows from the fact that both M and $\ln \frac{\omega (\beta )}{\omega (\alpha )}$ have the same sign with that of $R-L$. Rewrite $1-N$ as

$$\begin{aligned} \begin{aligned} 1-N=\frac{g(\beta )}{(\beta -\alpha )(t-1)^{2}},\ \ \text{ where }\ \ g(\beta )=\omega (\alpha )\omega (\beta )\ln t \ln \frac{\omega (\beta )}{\omega (\alpha )}+(\beta -\alpha )(t-1)^{2}. \end{aligned} \end{aligned}$$

One then can easily obtain $\lim _{t\rightarrow 1}(1-N)=0$.

For the other statements, we just established statement (i) for $t>1$, and those for $t<1$ can be proved similarly. Direct computation yields $\frac{\mathrm{d}(1-N)}{\mathrm{d} \beta }=\frac{g_{1}(\beta )}{(\beta -\alpha )^{2}(t-1)^{2}},$ where $g_{1}(\beta )=-\omega ^{2}(\alpha )\ln t \ln \frac{\omega (\beta )}{\omega (\alpha )} +(\beta -\alpha )(t-1)^{2}\left( (\alpha -\gamma (t))\ln t-1\right) ,$ and further

$$\begin{aligned} \begin{aligned}&g_{1}'(\beta )=\omega ^{2}(\alpha )\frac{t-1}{\omega (\beta )}\ln t +(t-1)^{2}\left( (\alpha -\gamma (t))\ln t-1\right) ,\ g_{1}''(\beta )=\frac{\omega ^2(\alpha )}{\omega ^2(\beta )}(1-t)^{2}\ln t. \end{aligned} \end{aligned}$$

It then follows that for $t>1$, $g_{1}(\beta )$ is concave upward. Furthermore, one has

$$\begin{aligned} \lim _{\beta \rightarrow \alpha }g_{1}(\beta )=0 \ \text{ and }\ \lim _{\beta \rightarrow \alpha }g_{1}'(\beta )=0, \end{aligned}$$

which implies $g_{1}(\beta )>0$ for $\beta >\alpha$. Note that $\displaystyle {\lim _{\beta \rightarrow \alpha }\frac{\mathrm{d}(1-N)}{\mathrm{d} \beta }=\ln \frac{t}{2}>0}\ \text{ for }\ t>1.$ We have $\frac{\mathrm{d}(1-N)}{\mathrm{d} \beta }>0$ for $\beta >\alpha$, and $1-N$ is strictly increasing on $(\alpha ,+\infty )$. Additionally, since $\displaystyle {\lim _{\beta \rightarrow \alpha }(1-N)=(\alpha -\gamma (t))\ln t,}$ one has, for $t>1$,

• (i1) if $\alpha \ge \gamma (t)$, then $\frac{z}{z_{3}}<0<1-N$, which yields $V_{1}<0<V_{2}$;

• (i2) For $\alpha <\gamma (t)$, we first claim that there exists a unique $\beta _{1}\in (\alpha ,1)$ such that $1-N=0$ for $\beta =\beta _{1}$. In fact, based on the facts that

  $$\begin{aligned} \lim _{\beta \rightarrow \alpha }(1-N)=(\alpha -\gamma (t))\ln t<0\end{aligned}$$

  and $1-N$ is strictly increasing on $(\alpha ,+\infty )$, one just need to show that $1-N>0$ for $\beta =1$, which is yielded by $g(1)>0$. For convenience, for $t>1$, we set

  $$\begin{aligned} \begin{aligned} g_{2}(\alpha ):=g(1)=-\omega (\alpha )\ln t \ln \omega (\alpha )+(1-\alpha )(t-1)^{2}. \end{aligned} \end{aligned}$$

  Note that $g_{2}''(\alpha )=-\frac{(1-t)^{2}\ln t}{\omega (\alpha )}<0$, which indicates that $g_{2}(\alpha )$ is concave downward for $t>1$. Note also that $g_2(1)=0$. To show that $g(1)>0$, we claim $g_{2}(0)\ge 0$. To get started, we set

  $$\begin{aligned} \begin{aligned} g_{3}(t):=g_{2}(0)=-t(\ln t)^{2}+(t-1)^{2}. \end{aligned} \end{aligned}$$

  Direct calculation gives $g_{3}'(t)=-(\ln t)^{2}-2\ln t+2(t-1)$ and $g_{3}''(t)=\frac{2}{t}(t-1-\ln t)>0$ for all $t>1$. Together with $g_{3}(1)=g_{3}'(1)=0$, one has $g_{3}(t)=g_{2}(0)>0$.

  If $\alpha < \gamma (t)$ and $\frac{z}{z_{3}}<(\alpha -\gamma (t))\ln t$, which is equivalent to $\alpha< \gamma (t)<\alpha -\frac{z}{z_3\ln t}$, then, one has $\frac{z}{z_{3}}<1-B$ for all $\beta >\alpha$, and more specifically, $\frac{z}{z_{3}}<1-N<0$, which implies $V_{2}<V_{1}<0$ for $\beta \in (\alpha ,\beta _{1})$; $\frac{z}{z_{3}}<1-N=0$ for $\beta =\beta _{1}$; $\frac{z}{z_{3}}<0<1-N$, which indicates $V_{1}<0<V_{2}$, for $\beta \in (\beta _{1},1)$.

• (i3) if $\gamma (t)>\alpha -\frac{z}{z_3\ln t}$, then, the straight line $w=\frac{z}{z_{3}}$ and $w=1-N$ have a unique intersection point $(\beta _{1}^{*}, w(\beta _{1}^{*}))$, which indicates that there exists a unique $\beta _{1}^{*}\in (\alpha ,\beta _{1})$ such that $1-N<\frac{z}{z_{3}}<0$, which suggests $V_{1}<V_{2}<0$ for $\beta \in (\alpha ,\beta _{1}^{*})$; $1-N=\frac{z}{z_{3}}<0$ and further $V_{2}=V_{1}<0$ for $\beta =\beta _{1}^{*}$; $\frac{z}{z_{3}}<1-N<0$, which yields $V_{2}<V_{1}<0$ for $\beta \in (\beta _{1}^{*},\beta _{1})$; $\frac{z}{z_{3}}<1-N=0$ for $\beta =\beta _{1}$; and $\frac{z}{z_{3}}<0<1-N$, which indicates $V_{1}<0<V_{2}$ for $\beta \in (\beta _{1},1)$.

1.4 Proof of Theorem 3.18

$$\begin{aligned}&\text{ Rewrite }\ \ J_{11}\ \text{ as }\ \ J_{11}\\&\quad =\frac{Mzz_{3}(1-N)}{(z-z_3)H(1)\left( \ln L-\ln R\right) ^{2}}\frac{\left( V-V_{1}\right) \left( V-V_{2}\right) }{L-Re^{-zV}}\left( L_{1}-R_{1}e^{-zV}\right) .\\&\text{ Direct } \text{ calculation } \text{ yields }\qquad \frac{\mathrm{d}J_{11}}{\mathrm{d} V}\\&\quad =\frac{Mzz_{3}(1-N)}{(z-z_3)H(1)\left( \ln L-\ln R\right) ^{2}}\frac{e^{-zV}}{\left( L-Re^{-zV}\right) ^{2}}f_{11}(V), \end{aligned}$$

where

$$\begin{aligned} \begin{aligned} f_{11}(V)=&\left( 2V-V_{1}-V_{2}\right) \left( L-Re^{-zV}\right) \left( L_1-R_1e^{-zV}\right) \\&+z\left( R_{1}L-L_{1}R\right) \left( V-V_{1}\right) \left( V-V_{2}\right) . \end{aligned} \end{aligned}$$

It follows that

$$\begin{aligned} \begin{aligned} f_{11}'(V)=\frac{\mathrm{d}f_{11}}{\mathrm{d} V}=e^{zV}\left( L-Re^{-zV}\right) g_{11}(V), \end{aligned} \end{aligned}$$

where $g_{11}(V)=2\left( L_1-R_1e^{-zV}\right) +z\left( 2V-V_{1}-V_{2}\right) \left( L_1+R_1e^{-zV}\right) .$ For $g_{11}(V)$, one has

$$\begin{aligned} \begin{aligned} g_{11}'(V)&=\frac{\mathrm{d}g_{11}}{\mathrm{d} V}=4zR_{1}e^{-zV}+2zL_{1}-z^{2}R_{1}e^{-zV}\left( 2V-V_{1}-V_{2}\right) ,\\ g_{11}''(V)&=\frac{\mathrm{d}^{2}g_{11}}{\mathrm{d} V^{2}}=z^{2}R_{1}e^{-zV}\left( z\left( 2V-V_{1}-V_{2}\right) -6\right) . \end{aligned} \end{aligned}$$

(6.13)

From (6.13), we obtain a unique zero of $g_{11}''(V)$ given by $V_{0}=\frac{1}{2}\left( \frac{6}{z}+V_{1}+V_{2}\right)$, which is the minimum point of $g_{11}'(V)$. Note that $\displaystyle {\lim _{V\rightarrow +\infty }g_{11}'(V)=2zL_{1}>0}$ and $\displaystyle {\lim _{V\rightarrow -\infty }g_{11}'(V)=+\infty .}$ If the minimal value $g_{11}'(V_{0})=2z\left( L_{1}-R_{1}e^{-zV_{0}}\right) \ge 0$, i.e., $V_{0}\le V_{3}$, then, $g_{11}'(V)\le 0$ for all V. In this case, $g_{11}(V)$ has a unique zero, since $\displaystyle {\lim _{V\rightarrow +\infty }g_{11}(V)=+\infty }$ and $\displaystyle {\lim _{V\rightarrow -\infty }g_{11}=-\infty .}$ If the minimal value $g_{11}'(V_{0})=2z\left( L_{1}-R_{1}e^{-zV_{0}}\right) < 0$ i.e., $V_{0}< V_{3}$, then $g_{11}'(V)$ has two zeros. Suppose $V_{e}$ is a zero point of $g_{11}'(V)$, one has $2V_{e}-V_{1}-V_{2}=\frac{4R_{1}+2L_{1}e^{zV_{e}}}{zR_{1}}$ and the extreme value of $g_{11}(V)$ given by $g_{11}(V_{e})=\frac{2}{R_{1}}\left( L_{1}^{2}e^{zV_{e}}+R_{1}^{2}e^{-zV_{e}} +4L_{1}R_{1}\right) >0,$ which indicates that $g_{11}(V)$ has a unique zero point. Therefore, no matter $V_{0}\le V_{3}$ or $V_{0}< V_{3}$, $g_{11}(V)$ always has a unique zero denoted by $V_{z}$. Furthermore, $f_{11}'(V)$ has two zeros $V_{1}$ and $V_{z}$. To determine the order of $V_{1}$ and $V_{z}$, one just need to determine the sign of $g_{11}(V_{1})$. In fact, if $g_{11}(V_{1})>0$, then $V_{1}>V_{z}$, if $g_{11}(V_{1})=0$, then $V_{1}=V_{z}$, if $g_{11}(V_{1})<0$, then $V_{1}<V_{z}$.

Note that $g_{11}(V_{1}) =\frac{1}{zL_{1}LR}\left[ C\frac{R_{1}}{L_{1}}+\frac{1}{(1-N)t}\Big (\frac{z}{z_{3}}\ln t-(\ln t-2)(1-N)\Big )\right] ,$ where C is defined in (3.8) and the sign of the quantity C has been studied in Lemma 3.17. It then follows that , with $\Delta =\frac{\frac{z}{z_{3}}\ln t-(\ln t-2)(1-N)}{\frac{z}{z_{3}}\ln t-(\ln t+2)(1-N)},$

• for $C>0$, one has $g_{11}(V_{1})>0$ if $\frac{R_1}{L_1}>-\frac{\Delta }{t}$, and $g_{11}(V_{1})<0$ if $\frac{R_1}{L_1}<-\frac{\Delta }{t}$.

• for $C<0$, one has $g_{11}(V_{1})>0$ if $\frac{R_1}{L_1}<-\frac{\Delta }{t}$, and $g_{11}(V_{1})<0$ if $\frac{R_1}{L_1}>-\frac{\Delta }{t}$.

• for $C=0$, one has $g_{11}(V_{1})>0$.

In particular, $g_{11}(V_{1})=0$ if $\frac{R_1}{L_1}=-\frac{\Delta }{t}$ with $C\ne 0$.

If $V_{1}<V_{z}$, then $f_{11}$ is increasing on $(-\infty , V_1)$, decreasing on $(V_{1},V_{2})$, and increasing on $(V_2,\infty )$. Note that $f_{11}(V_{1})=0$, which is a local maximum of $f_{11}$, $\lim _{V\rightarrow -\infty }f_{11}=-\infty$ and $\lim _{V\rightarrow \infty }f_{11}=\infty$, $f_{11}$ has the other zero $V_{11}^{1}$ with $V_{11}^{1}>V_{1}$. Furthermore, $\displaystyle {\lim _{V\rightarrow V_{1}}\frac{\mathrm{d}J_{11}}{\mathrm{d} V}=\frac{Mz_{3}(1-N)e^{zV_{1}}}{2(z-z_3)H(1)\left( \ln L-\ln R\right) ^{2}R}g_{11}(V_{1})<0,}$ since $g_{11}(V_{1})<g_{11}(V_{z})=0$, which can be obtained from the fact that $g_{11}$ is increasing on $(-\infty ,V_{z})$ and $V_{1}<V_{z}$. Therefore, when $V>V_{11}^{1}$, $\frac{\mathrm{d}J_{11}}{\mathrm{d} V}>0$, and when $V<V_{11}^{1}$, $\frac{\mathrm{d}J_{11}}{\mathrm{d} V}<0$. This result also hold for the case when $V_{1}\ge V_{z}$, which can be proved in a similar way. Consequently the statement (i) follows.

The statements for $J_{21}$ and $J_{31}$ can be proved similarly.

1.5 Proof of Theorem 4.6

We will just prove the first statement. Statement (ii) can be proved by a similar argument. For $\frac{L_{2}}{L_{1}}<\frac{D_{1}}{D_{2}}<\frac{R_{2}}{R_{1}}$, one has

$$\begin{aligned} \begin{aligned} {{\mathcal {J}}}_{1,2}^1&=\frac{Mzz_{3}(1-N)}{(z-z_3)H(1)\left( \ln L-\ln R\right) ^{2}}\frac{\left( V-V_{1}\right) \left( V-V_{2}\right) }{L-Re^{-zV}} \left( L_d^{-}-R_d^{-}e^{-zV}\right) . \end{aligned} \end{aligned}$$

Direct calculation yields

$$\begin{aligned} \begin{aligned} \frac{\mathrm{d}{{\mathcal {J}}}_{1,2}^1}{\mathrm{d} V}=\frac{Mzz_{3}(1-N)}{(z-z_3)H(1)\left( \ln L-\ln R\right) ^{2}}\frac{e^{-zV}}{\left( L-Re^{-zV}\right) ^{2}}f_{d3}(V), \end{aligned} \end{aligned}$$

and further

$$\begin{aligned} \begin{aligned} f_{d3}'(V)=e^{zV}\left( L-Re^{-zV}\right) g_{d3}(V), \end{aligned} \end{aligned}$$

(6.14)

where $f_{d3}(V)$ and $g_{d3}(V)$ are given in (4.5).

For $g_{d3}(V)$, we have

$$\begin{aligned} \begin{aligned} g_{d3}'(V)=&2zL_d^{-}+4zR_d^{-}e^{-zV}-z^{2}R_d^{-}e^{-zV}\left( 2V-V_{1}-V_{2}\right) ,\\ g_{d3}''(V)=&-z^{2}R_d^{-}e^{-zV}\left( 6-z\left( 2V-V_{1}-V_{2}\right) \right) . \end{aligned} \end{aligned}$$

(6.15)

From (6.15), we obtain a unique zero of $g_{d3}''(V)$ given by $V_{0}=\frac{1}{2}\left( \frac{6}{z}+V_{1}+V_{2}\right)$, which actually is the maximum value point of $g_{d3}'(V)$. Note that

$$\begin{aligned} \lim _{V\rightarrow +\infty }g_{d3}'(V)=2zL_d^{-}>0,\ \lim _{V\rightarrow -\infty }g_{d3}'(V)=-\infty , \end{aligned}$$

and the maximal value $g_{d3}'(V_{0})=2z\left( L_d^{-}-R_d^{-}e^{-zV_{0}}\right) > 0.$ Hence, $g_{d3}'(V)$ has a unique zero denoted by $V_{d3}$ and $V_{d3}$ is actually the minimum value point of $g_{d3}(V)$. From $g_{d3}'(V)=0$, one immediately has $2V_{d3}-V_{1}-V_{2}=\frac{4R_d^{-}+2L_d^{-}e^{zV_{d3}}}{zR_d^{-}}$ and the corresponding extreme value of $g_{d3}(V)$, i.e., $g_{d3}(V_{d3})=-\frac{2}{R_d^{-}}h(V_{d3})$. From lemma 4.5, $g_{d3}(V_{d3})>0$ for $V_{z}^{1}<V_{d3}<V_{z}^{2}$, $g_{d3}(V_{d3})=0$ for $V_{d3}=V_{z}^{1}$ or $V_{d3}=V_{z}^{2}$, and $g_{d3}(V_{d3})<0$ for $V_{d3}<V_{z}^{1}$ or $V_{d3}>V_{z}^{2}$.

If $g_{d3}(V_{d3})>0$, then, $g_{d3}(V)>0$ for all V. It follows that $f_{d3}'(V)$ has a unique zero $V_{1}$, $f_{d3}'(V)>0$ for $V>V_{1}$, and $f_{d3}'(V)<0$ for $V<V_{1}$. Therefore, the minimal value of $f_{d3}(V)$ is $f_{d3}(V_{1})=0$. Note that $\displaystyle {\lim _{V\rightarrow V_{1}}\frac{\mathrm{d}{{\mathcal {J}}}_{1,2}^1}{\mathrm{d} V}=\frac{Mz_{3}(1-N)g_{d3}(V_{1})}{2(z-z_3)H(1)\left( \ln L-\ln R\right) ^{2}L}>0.}$ Then, $\frac{\mathrm{d}{{\mathcal {J}}}_{1,2}^1}{\mathrm{d} V}>0$ follows, which yields ${{\mathcal {J}}}_{1,2}^1(V)$ always increases.

If $g_{d3}(V_{d3})=0$, then $g_{d3}(V)$ has the unique zero $V_{d3}$. From (6.14), $f_{d3}'(V)$ has two zeros $V_{1}$ and $V_{d3}$. To determine the position relation of $V_{1}$ and $V_{d3}$, one just need to determine the sign of $g_{d3}'(V_{1})=2zR_d^{-}\bigg (\frac{L_d^{-}}{R_d^{-}}+\frac{t\big ((4+\ln t)(1-N)-\frac{z}{z_{3}}\ln t\big )}{2(1-N)}\bigg ).$ In fact, if $g_{d3}'(V_{1})<0$, then $V_{1}<V_{d3}$, if $g_{d3}'(V_{1})=0$, then $V_{1}=V_{d3}$, if $g_{d3}'(V_{1})>0$, then $V_{1}>V_{d3}$. However, no matter $V_{1}<V_{d3}$ or $V_{1}\ge V_{d3}$, from (6.14), one always has $f_{d3}'\le 0$ for $V<V_{1}$ and $f_{d3}'\ge 0$ for $V>V_{1}$. Hence, the minimal value of $f_{d3}$ is $f_{d3}(V_{1})=0$. Note that $\displaystyle {\lim _{V\rightarrow V_{1}}\frac{\mathrm{d}{{\mathcal {J}}}_{1,2}^1}{\mathrm{d} V}=\frac{Mz_{3}(1-N)g_{d3}(V_{1})}{2(z-z_3)H(1)\left( \ln L-\ln R\right) ^{2}L}>0.}$ Then, $\frac{\mathrm{d}{{\mathcal {J}}}_{1,2}^1}{\mathrm{d} V}>0$ follows, and hence, ${{\mathcal {J}}}_{1,2}^1(V)$ always increases.

If $g_{d3}(V_{d3})<0$, then, $g_{d3}(V)$ has two zeros denoted by $V_{z}^{3}$ and $V_{z}^{4}$ with $V_{z}^{3}<V_{z}^{4}$, since $\displaystyle {\lim _{V\rightarrow \pm \infty }g_{d3}=+\infty }$. It follows that $f_{d3}'(V)$ has three zeros $V_{1}$, $V_{z}^{3}$ and $V_{z}^{4}$. To determine the position relation of $V_{1}$, $V_{z}^{3}$ and $V_{z}^{4}$, one just need to determine the sign of $g_{d3}(V_{1})$ and $g_{d3}'(V_{1})$. In fact, if $g_{d3}(V_{1})<0$, then $V_{z}^{3}<V_{1}<V_{z}^{4}$, if $g_{d3}(V_{1})>0$ and $g_{d3}'(V_{1})<0$, then $V_{1}<V_{z}^{3}<V_{z}^{4}$, and if $g_{d3}(V_{1})>0$ and $g_{d3}'(V_{1})>0$, then $V_{z}^{3}<V_{z}^{4}<V_{1}$.

Note that $g_{d3}(V_{1})=\frac{\frac{z}{z_{3}}\ln t-(\ln t-2)(1-N)}{(1-N)R_d^{-}}\bigg (\frac{L_d^{-}}{R_d^{-}}+\frac{1}{t\Delta }\bigg ).$ It follows that

• For $\frac{1}{1-N}\Big (\frac{z}{z_{3}}\ln t-(\ln t-2)(1-N)\Big )>0$, one has $g_{d3}(V_{1})>0$ (resp. $g_{d3}(V_{1})<0$) if $\frac{L_d^{-}}{R_d^{-}}<-\frac{1}{t\Delta }$ (resp. $\frac{L_d^{-}}{R_d^{-}}>-\frac{1}{t\Delta }$);

• For $\frac{1}{1-N}\left( \frac{z}{z_{3}}\ln t-(\ln t-2)(1-N)\right) <0$, one has $g_{d3}(V_{1})>0$ (resp. $g_{d3}(V_{1})<0$) if $\frac{L_d^{-}}{R_d^{-}}>-\frac{1}{t\Delta }$ (resp. $\frac{L_d^{-}}{R_d^{-}}<-\frac{1}{t\Delta }$);

• For $\frac{1}{1-N}\left( \frac{z}{z_{3}}\ln t-(\ln t-2)(1-N)\right) \ne 0$, one has $g_{d3}(V_{1})=0$ if $\frac{L_d^{-}}{R_d^{-}}=-\frac{1}{t\Delta }$;

• For $\frac{1}{1-N}\left( \frac{z}{z_{3}}\ln t-(\ln t-2)(1-N)\right) =0$, one has $g_{d3}(V_{1})>0$.

If $V_{z}^{3}<V_{1}<V_{z}^{4}$, then, $f_{d3}(V)$ decreases on $(-\infty , V_{z}^{3})$, increases on $(V_{z}^{3},V_{1})$, decreases on $(V_{1},V_{z}^{4})$, and increases on $(V_{z}^{4},\infty )$. Note that $f_{d3}(V_{1})=0$, which is a local maximum of $f_{d3}(V)$, one has $f_{d3}(V_{z}^{3})<0$ and $f_{d3}(V_{z}^{4})<0$. Since

$$\begin{aligned} \lim _{V\rightarrow V_{1}}\frac{\mathrm{d}{{\mathcal {J}}}_{1,2}^1}{\mathrm{d} V}=\frac{Mz_{3}(1-N)g_{d3}(V_{1})}{2(z-z_3)H(1)\left( \ln L-\ln R\right) ^{2}L}<0, \end{aligned}$$

$\frac{\mathrm{d}{{\mathcal {J}}}_{1,2}^1}{\mathrm{d} V}$ has two zeros denoted by $V_{c}^{51}$ and $V_{c}^{52}$ with $V_{c}^{51}<V_{c}^{52}$ such that $\frac{\mathrm{d}{{\mathcal {J}}}_{1,2}^1}{\mathrm{d} V}>0$ for $V<V_{c}^{51}$ or $V>V_{c}^{52}$, and $\frac{\mathrm{d}{{\mathcal {J}}}_{1,2}^1}{\mathrm{d} V}<0$ for $V_{c}^{51}<V<V_{c}^{52}$, that is, ${{\mathcal {J}}}_{1,2}^1$ increases on $(-\infty , V_{c}^{51})$, decreases on $(V_{c}^{51},V_{c}^{52})$, and increases on $(V_{c}^{52},\infty )$.

Similar discussions can be applied to the cases with $V_{1}<V_{z}^{3}<V_{z}^{4}$ and $V_{z}^{3}<V_{z}^{4}<V_{1}$, respectively. This completes the proof of the first statement.