Skip to main content
SpringerLink
Log in

Variational Bayesian inversion for the reaction coefficient in space-time nonlocal diffusion equations

  • Published:
Advances in Computational Mathematics Aims and scope Submit manuscript

Abstract

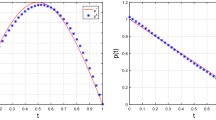
In the paper, a variational Bayesian method is used to identify the reaction coefficient for space-time nonlocal diffusion equations using nonlocal averaged flux data. To show the posterior measure to be well-defined, we rigorously prove that the forward operator is continuous with respect to the unknown reaction field. Then, gradient-based prior information is proposed to explore oscillation features in the reaction coefficient. Moreover, the Bayesian inverse problem is shown to be well-posed in Hellinger distance. To accurately characterize the posterior density using uncorrelated samples, an efficient variational Bayesian method is used to estimate the reaction coefficient in the nonlocal models. A few numerical results are presented to illustrate the efficacy of the proposed approach and confirm some theoretic discoveries.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Similar content being viewed by others

References

  1. Acosta, G., Bersetche, F.M., Borthagaray, J.P.: A short FE implementation for a 2d homogeneous Dirichlet problem of a fractional Laplacian. Comput. Math. with Appl. 74, 784–816 (2017)

    Article  MathSciNet  Google Scholar 

  2. Acosta, G., Borthagaray, J.P.: A fractional Laplace equation: Regularity of solutions and finite element approximations. SIAM J. Numer. Anal. 55, 472–495 (2017)

    Article  MathSciNet  Google Scholar 

  3. Andreu-Vaillo, F., Mazón, J.M., Rossi, J.D., Toledo-Melero, J. J.: Nonlocal Diffusion Problems, vol. 165. American Mathematical Society, Providence (2010)

    Book  Google Scholar 

  4. Barkai, E., Metzler, R., Klafter, J.: From continuous time random walks to the fractional Fokker-Planck equation. Phys. Rev. E. 61, 132–138 (2000)

    Article  MathSciNet  Google Scholar 

  5. Brockmann, D., Hufnagel, L., Geisel, T.: The scaling laws of human travel. Nature 439, 462–465 (2006)

    Article  Google Scholar 

  6. Candès, E.J., Wakin, M.B., Boyd, S.P.: Enhancing sparsity by reweighted l1 minimization. J. Fourier Anal. Appl. 14, 877–905 (2008)

    Article  MathSciNet  Google Scholar 

  7. Chartrand, R., Yin, W.: Iteratively reweighted algorithms for compressive sensing, pp 3869–3872 (2008)

  8. Demengel, F., Demengel, G., Erné, R.: Functional Spaces for the Theory of Elliptic Partial Differential Equations. Springer, London (2012)

    Book  Google Scholar 

  9. Du, Q., Gunzburger, M., Lehoucq, R.B., Zhou, K.: Analysis and approximation of nonlocal diffusion problems with volume constraints. SIAM Rev. 54, 667–686 (2012)

    Article  MathSciNet  Google Scholar 

  10. Du, Q., Gunzburger, M., Lehoucq, R.B., Zhou, K.: A nonlocal vector calculus, nonlocal volume-constrained problems, and nonlocal balance laws. Math. Models Methods Appl. Sci. 23, 493–540 (2013)

    Article  MathSciNet  Google Scholar 

  11. Gamerman, D., Lopes, H.F.: Markov Chain Monte Carlo: Stochastic simulation for Bayesian inference. Chapman and Hall/CRC, London (2006)

  12. Huang, Y., Oberman, A.: Numerical methods for the fractional Laplacian: A finite difference-quadrature approach. SIAM J. Numer. Anal. 52, 3056–3084 (2014)

    Article  MathSciNet  Google Scholar 

  13. Jia, J., Peng, J., Yang, J.: Harnack’s inequality for a space-time fractional diffusion equation and applications to an inverse source problem. J. Differ. Equ. 262, 4415–4450 (2017)

    Article  MathSciNet  Google Scholar 

  14. Jin, B., Rundell, W.: A tutorial on inverse problems for anomalous diffusion processes. Inv. Probl. 31, 035003 (2015)

    Article  MathSciNet  Google Scholar 

  15. Jin, B., Zou, J.: A Bayesian inference approach to the ill-posed Cauchy problem of steady-state heat conduction. Int. J. Numer. Methods Eng. 76, 521–544 (2008)

    Article  MathSciNet  Google Scholar 

  16. Jin, B., Zou, J.: Hierarchical Bayesian inference for ill-posed problems via variational method. J. Comput. Phys. 229, 7317–7343 (2010)

    Article  MathSciNet  Google Scholar 

  17. Jing, X., Jia, J., Peng, J.: Well-posedness for a nonlocal nonlinear diffusion equation and applications to inverse problems. Appl. Anal., 1–15 (2019)

  18. Kaipio, J., Somersalo, E.: Statistical and Computational Inverse Problems. Springer Science & Business Media, Berlin (2006)

    MATH  Google Scholar 

  19. Khaninezhad, M.M., Jafarpour, B.: Sparse randomized maximum likelihood (sprml) for subsurface flow model calibration and uncertainty quantification. Adv. Water Resour. 69, 23–37 (2014)

    Article  Google Scholar 

  20. Kullback, S., Leibler, R.A.: On information and sufficiency. Ann Math Stat 22, 79–86 (1951)

    Article  MathSciNet  Google Scholar 

  21. Li, C., zeng, F.: Numerical Methods for Fractional Calculus. Chapman & Hall/CRC, London (2015)

  22. Li, L., Jafarpour, B.: Effective solution of nonlinear subsurface flow inverse problems in sparse bases. Inv. Probl. 26, 105016 (2010)

    Article  MathSciNet  Google Scholar 

  23. Li, Y.S., Wei, T.: An inverse time-dependent source problem for a time-space fractional diffusion equation. Appl. Math. Comput. 336, 257–271 (2018)

    MathSciNet  MATH  Google Scholar 

  24. Li, Z., Imanuvilov, O.Y., Yamamoto, M.: Uniqueness in inverse boundary value problems for fractional diffusion equations. Inv. Probl. 32, 015004 (2016)

    Article  MathSciNet  Google Scholar 

  25. Lin, Y., Xu, C.: Finite difference/spectral approximations for the time-fractional diffusion equation. J. Comput. Phys. 225, 1533–1552 (2007)

    Article  MathSciNet  Google Scholar 

  26. Minden, V., Ying, L.: A simple solver for the fractional Laplacian in multiple dimensions. SIAM J. Sci. Comput. 42, A878–A900 (2020)

    Article  MathSciNet  Google Scholar 

  27. Podlubny, I.: Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications, vol. 198. Elsevier, Amsterdam (1998)

    Google Scholar 

  28. Vogel, C.R.: Computational Methods for Inverse Problems Society for Industrial and Applied Mathematics (2002)

  29. Song, X., Jiang, L., Zheng, G.-H.: Implicit sampling for hierarchical Bayesian inversion and applications in fractional multiscale diffusion models. J. Comput. Appl. Math. 375, 112826 (2020)

    Article  MathSciNet  Google Scholar 

  30. Song, X., Zheng, G.-H., Jiang, L.: Identification of the reaction coefficient in time fractional diffusion equations. J. Comput. Appl. Math. 345, 295–309 (2019)

    Article  MathSciNet  Google Scholar 

  31. Stuart, A.M.: Inverse problems: A Bayesian perspective. Acta Numerica 19, 451–559 (2010)

    Article  MathSciNet  Google Scholar 

  32. Sun, L., Wei, T.: Identification of the zeroth-order coefficient in a time fractional diffusion equation. Appl. Numer. Math. 111, 160–180 (2017)

    Article  MathSciNet  Google Scholar 

  33. Tatar, S., Tinaztepe, R., Ulusoy, S.: Simultaneous inversion for the exponents of the fractional time and space derivatives in the space-time fractional diffusion equation. Appl. Anal. 95, 1–23 (2014)

    Article  MathSciNet  Google Scholar 

  34. Tian, X., Du, Q.: Nonconforming discontinuous Galerkin methods for nonlocal variational problems. SIAM J. Numer. Anal. 53, 762–781 (2015)

    Article  MathSciNet  Google Scholar 

  35. Tsilifis, P., Bilionis, I., Katsounaros, I., Zabaras, N.: Computationally efficient variational approximations for Bayesian inverse problems. JVVUQ 1, 031004 (2016)

    Google Scholar 

  36. Webb, M.: Analysis and approximation of a fractional differential equation. Master’s thesis, University of Oxford (2012)

  37. Wei, T., Li, X., Li, Y.: An inverse time-dependent source problem for a time-fractional diffusion equation. Inv. Probl. 32, 085003 (2016)

    Article  MathSciNet  Google Scholar 

  38. Wei, T., Wang, J.-G.: Determination of robin coefficient in a fractional diffusion problem. Appl. Math. Model. 40, 7948–7961 (2016)

    Article  MathSciNet  Google Scholar 

  39. Yao, Z., Hu, Z., Li, J.: A TV-Gaussian prior for infinite-dimensional Bayesian inverse problems and its numerical implementations. Inv. Probl. 32, 075006 (2016)

    Article  MathSciNet  Google Scholar 

  40. Zeng, F., Li, C., Liu, F., Turner, I.: The use of finite difference/element approaches for solving the time-fractional subdiffusion equation. SIAM J. Sci. Comput. 35, A2976–A3000 (2013)

    Article  MathSciNet  Google Scholar 

  41. Zhang, Y.-X., Jia, J., Yan, L.: Bayesian approach to a nonlinear inverse problem for a time-space fractional diffusion equation. Inv. Probl. 34, 125002 (2018)

    Article  MathSciNet  Google Scholar 

  42. Zheng, G.-H., Ding, M.-H.: Uniqueness of the inverse reaction coefficient problems for nonlocal diffusion models arXiv:1812.00673 (2018)

  43. Zheng, G.-H., Ding, M.-H.: Identification of the degradation coefficient for an anomalous diffusion process in hydrology. Inv. Probl. (2019)

Download references

Funding

L. Jiang received support of NSFC 11871378 and the Science Challenge Project (No. TZ2018001), and G. Zheng received support of NSFC 11301168.

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Hunan University of Technology and Business, Changsha, 410205, China

    Xiaoyan Song

  2. School of Mathematics, Hunan University, Changsha, 410082, China

    Guang-Hui Zheng

  3. School of Mathematical Sciences, Tongji University, Shanghai, 200092, China

    Lijian Jiang

Authors
  1. Xiaoyan Song
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Guang-Hui Zheng
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Lijian Jiang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Lijian Jiang.

Additional information

Communicated by: Bangti Jin

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Song, X., Zheng, GH. & Jiang, L. Variational Bayesian inversion for the reaction coefficient in space-time nonlocal diffusion equations. Adv Comput Math 47, 31 (2021). https://doi.org/10.1007/s10444-021-09850-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10444-021-09850-1

Keywords

Mathematics Subject Classification (2010)

Search

Navigation