Stability of Traveling Wave Solutions of Nonlinear Dispersive Equations of NLS Type

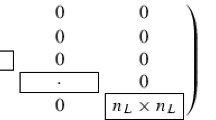
Abstract

We present a rigorous modulational stability theory for periodic traveling wave solutions to equations of nonlinear Schrödinger type. For Hamiltonian dispersive equations with a non-singular symplectic form and d conserved quantities (in addition to the Hamiltonian), one expects that generically ${{\mathcal {L}}}$, the linearization around a periodic traveling wave, will have a particular Jordan structure. The kernel $\ker ({\mathcal L})$ and the first generalized kernel $\ker ({\mathcal L}^2)/\ker ({{\mathcal {L}}})$ are expected to be d dimensional, with no higher generalized kernels. The breakup of this Jordan block under perturbations arising from a change in boundary conditions dictates the modulational stability or instability of the underlying periodic traveling wave. This general picture is worked out in detail for equations of nonlinear Schrödinger (NLS) type. We give explicit genericity conditions that guarantee that the Jordan form is the generic one: these take the form of non-vanishing determinants of certain matrices whose entries can be expressed in terms of a finite number of moments of the traveling wave solution. Assuming that these genericity conditions are met we give a normal form for the small eigenvalues that result from the break-up of the generalized kernel, in the form of the eigenvalues of a quadratic matrix pencil. We compare these results to direct numerical simulation for the cubic and quintic focusing and defocusing NLS equations subject to both longitudinal and transverse perturbations. The stability of traveling waves of the cubic NLS subject to longitudinal perturbations has been previously studied using the integrability and our results agree with those in the literature. All of the remaining cases are new.

Appendices

Appendix A: Constructing the Periodic Eigenfunctions

In this Appendix, we show how one can find the two periodic null vectors and two periodic generalized null vectors for ${\mathcal {L}}$. Given the four solutions to ${\mathcal {L}} \mathbf{u}=0$ from Equation (26) and the two additional quantities from Equation (27), we can write linear combinations

$$\begin{aligned} \mathbf{u}_0=&\sigma \left( \begin{array}{c}0\\ A\end{array}\right) ,&\mathbf{u}_1=&\gamma \left( \begin{array}{c}A_E\\ AS_E\end{array}\right) +\rho \left( \begin{array}{c}A_\kappa \\ AS_\kappa \end{array}\right) +\sigma \left( \begin{array}{c}A_\omega \\ AS_\omega \end{array}\right) ,\\ \mathbf{u}_2=&\sigma \left( \begin{array}{c}A_y\\ AS_y\end{array}\right) ,&\mathbf{u}_3=&\tau \left( \begin{array}{c}A_E\\ AS_E\end{array}\right) +\nu \left( \begin{array}{c}A_\kappa \\ AS_\kappa \end{array}\right) +\sigma \left( \begin{array}{c}0\\ yA/2\end{array}\right) . \end{aligned}$$

Then ${\mathcal {L}}{} \mathbf{u}_{0,2}=0$ and ${\mathcal {L}}\mathbf{u}_{1,3}=\mathbf{u}_{0,2}$. The null vectors $\mathbf{u}_{0,2}$ are already periodic, so we need to choose $\sigma ,\ \gamma ,\ \rho ,\ \tau ,\ \nu$ to enforce the boundary conditions $\mathbf{u}_j(T)=\mathbf{u}_j(0)$ on the generalized null vectors $\mathbf{u}_{1,3}$. Given that $A(0)=A(T)$ and $S(0)=S(T)-\eta$, we have

$$\begin{aligned} A_E(0)&= \left[ A(0)\right] _E=\left[ A(T)\right] _E=A_y(T)T_E+A_E(T)\\ \nonumber S_E(0)&= \left[ S(0)\right] _E=\left[ S(T)-\eta \right] _E=S_y(T)T_E+S_E(T)-\eta _E, \end{aligned}$$

and similarly for $A_\kappa$, $A_\omega$, $S_\kappa$, and $S_\omega$. Using these to equate $\mathbf{u}_1(0)=\mathbf{u}_1(T)$ and $\mathbf{u}_3(0)=\mathbf{u}_3(T)$ results in the following four equations:

$$\begin{aligned}&\gamma T_E+\rho T_\kappa +\sigma T_\omega =0, \\&\gamma \eta _E+\rho \eta _\kappa +\sigma \eta _\omega =0, \\&\tau T_E+\nu T_\kappa =0, \\&\tau \eta _E+\nu \eta _\kappa +\sigma T/2=0, \end{aligned}$$

which we can solve for $\gamma ,\ \rho ,\ \sigma ,\ \tau ,\ \nu$, finding

$$\begin{aligned}&\gamma = \{T,\eta \}_{\kappa ,\omega },&\rho = \{T,\eta \}_{\omega ,E},&\sigma = \{T,\eta \}_{E,\kappa },&\\&\tau = T T_\kappa /2,&\nu = -T T_E/2.&\end{aligned}$$

Appendix B: Evaluating Matrix Elements

In this Appendix, we show some details of how to compute the matrix elements from Equation (40).

We begin by computing the elements of $\mathbf{M}^{(2)}$, which are also the elements of the gram matrix

$$\begin{aligned}&\mathbf{v}_0 \mathbf{u}_0 = -\sigma \int _0^T A (\gamma A_E + \rho A_{\kappa } + \sigma A_{\omega }) \mathrm{d}y&\\&\mathbf{v}_2 \mathbf{u}_0 = -\sigma \int _0^T A (\tau A_E + \nu A_{\kappa }) \mathrm{d}y&\\&\mathbf{v}_2 \mathbf{u}_2 = \sigma \int _0^T A A_y (\tau S_E + \nu S_{\kappa } + \sigma \frac{y}{2}) - A S_y (\tau A_E + \nu A_{\kappa } ) \mathrm{d}y.&\end{aligned}$$

The first two are somewhat trivial, the third is only slightly more complicated and requires some integration by parts and the fact that $(\tau \partial _E + \nu \partial _\kappa ) \eta +\sigma T/2 = 0$. We see that

$$\begin{aligned}&\mathbf{v}_0 \mathbf{u}_0 = -\frac{\sigma }{2} (\gamma M_E + \rho M_\kappa + \sigma M_\omega ) = - \frac{\sigma }{2} \{\eta ,T,M\}_{\kappa ,E,\omega }&\\&\mathbf{v}_2 \mathbf{u}_0 = -\frac{\sigma }{2} (\tau M_E + \nu M_\kappa ) = -\frac{\sigma T}{4}\{T,M\}_{\kappa ,E}\\&\mathbf{v}_2 \mathbf{u}_2 = -\frac{\sigma }{2} (\tau \kappa T_E + \nu \kappa T_\kappa + \nu T + \sigma M/2) =-\frac{\sigma ^2M}{4}+\frac{\sigma T^2T_E}{4}. \end{aligned}$$

We also note that using $(\gamma \partial _E + \rho \partial _\kappa + \sigma \partial _\omega ) \eta = 0$, we also have

$$\begin{aligned} \mathbf{v}_0 \mathbf{u}_2&= \sigma \int _0^T A A_y (\gamma S_E + \rho S_{\kappa } + \sigma S_{\omega }) - A S_y (\gamma A_E + \rho A_{\kappa } + \sigma A_{\omega } ) \mathrm{d}y\\&= -\frac{\sigma }{2}(\gamma \kappa T_E+\rho \kappa T_\kappa +\rho T+\sigma \kappa T_\omega ) = -\frac{\sigma \rho T}{2}. \end{aligned}$$

It remains to compute the quantities for $\mathbf{M}^{(1)}$ and $\mathbf{M}^{(0)}$. For $\mathbf{M}^{(1)}$, because of the symmetry of ${\mathcal {L}}^{(1)}$, we need only to find the four quantities $\mathbf{v}_{0,2}{\mathcal {L}}^{(1)}{} \mathbf{u}_{0,2}$. One can show that ${\mathcal {L}}^{(1)}{} \mathbf{u}_0=2i\mathbf{u}_2$, so that $\mathbf{v}_0{\mathcal {L}}^{(1)}{} \mathbf{u}_0$ and $\mathbf{v}_2{\mathcal {L}}^{(1)}{} \mathbf{u}_0$ follow from $\mathbf{v}_j\mathbf{u}_k$. We have

$$\begin{aligned} \mathbf{v}_0{\mathcal {L}}^{(1)}{} \mathbf{u}_0&=-i\sigma \rho T,\\ \mathbf{v}_2{\mathcal {L}}^{(1)}{} \mathbf{u}_0&= -i\sigma (T\nu +\sigma M/2). \end{aligned}$$

Here we will compute $\mathbf{v}_0{\mathcal {L}}^{(1)}{} \mathbf{u}_2$, leaving computation of $\mathbf{v}_2{\mathcal {L}}^{(1)}{} \mathbf{u}_2$ as an exercise for the reader. The integral we seek to compute is

$$\begin{aligned} \mathbf{v}_0{\mathcal {L}}^{(1)}{} \mathbf{u}_2&=-2i\sigma \int _0^TA(\gamma S_E +\rho S_\kappa +\sigma S_\omega )(-A' S'-(A S')')\\&\quad +(\gamma A_E+\rho A_\kappa +\sigma A_\omega )(-A''+A (S')^2)\mathrm{d}y, \end{aligned}$$

which can be simplified using that $2A'S'+AS''=0$ and rearranged as

$$\begin{aligned} \mathbf{v}_0{\mathcal {L}}^{(1)}{} \mathbf{u}_2&=-2i\sigma \left[ \gamma \int _0^T -A''A_E +AA_E( S')^2\mathrm{d}y\right. \\&\quad \left. +\rho \int _0^T -A''A_\kappa +AA_\kappa ( S')^2\mathrm{d}y +\sigma \int _0^T -A''A_\omega +AA_\omega ( S')^2\mathrm{d}y\right] . \end{aligned}$$

Next we integrate some terms by parts, using that $AA'=(A^2)'/2$. This leads to

$$\begin{aligned} \mathbf{v}_0{\mathcal {L}}^{(1)}{} \mathbf{u}_2&=-2i\sigma \left[ \gamma \int _0^T (A')^2_E/2 +AA_E( S')^2\mathrm{d}y\right. \\&\quad \left. +\rho \int _0^T (A')^2_\kappa /2 +AA_\kappa ( S')^2\mathrm{d}y +\sigma \int _0^T (A')^2_\omega /2+AA_\omega ( S')^2\mathrm{d}y\right] . \end{aligned}$$

Now we use that $\int _0^T (A')^2\mathrm{d}y = K$ with it’s relevant derivatives to simplify:

$$\begin{aligned} \mathbf{v}_0{\mathcal {L}}^{(1)}{} \mathbf{u}_2&=-2i\sigma \left[ \gamma T/2 -\rho \eta /2-\sigma M/4\right. \\&\quad \left. +\gamma \int _0^T AA_E( S')^2\mathrm{d}y+\rho \int _0^T AA_\kappa ( S')^2\mathrm{d}y+\sigma \int _0^T AA_\omega ( S')^2\mathrm{d}y\right] . \end{aligned}$$

Finally, one can show that $AA_E( S')^2=\left( \frac{A^2( S')^2}{2}\right) _E-A^2 S' S'_E=\left( \frac{\kappa S'}{2}\right) _E-\kappa S'_E=-\kappa S'_E/2$. The same is true with $\omega$ derivatives, and similar is true with $\kappa$ derivatives. This leads to

$$\begin{aligned} \mathbf{v}_0{\mathcal {L}}^{(1)}{} \mathbf{u}_2&=-2i\sigma \left[ \frac{\gamma T}{2}-\frac{\rho \eta }{2}-\frac{\sigma M}{4}\right. \\&\quad \left. +\gamma \int _0^T -\kappa S'_E/2\mathrm{d}y+\rho \int _0^T -\kappa S'_\kappa /2+ S'/2\mathrm{d}y+\sigma \int _0^T -\kappa S'_\omega /2\mathrm{d}y\right] . \end{aligned}$$

Now, since $S(T)-S(0)=\eta$, we can complete the integration and simplify using $\gamma \eta _E+\rho \eta _\kappa +\sigma \eta _\omega =0$ to obtain

$$\begin{aligned} \mathbf{v}_0{\mathcal {L}}^{(1)}{} \mathbf{u}_2=-2i\sigma \left[ \frac{\gamma T}{2}-\frac{\sigma M}{4}\right] . \end{aligned}$$

In a similar way, one can find

$$\begin{aligned} \mathbf{v}_2{\mathcal {L}}^{(1)}{} \mathbf{u}_2 = -2i\sigma \left[ \frac{\tau T}{2}+\frac{\sigma \kappa T}{4}\right] . \end{aligned}$$

Now, to obtain the matrix elements of $\mathbf{M}^{(0)}$, we will need 8 more quantities: $\mathbf{v}_{1,3}{\mathcal {L}}^{(2)}{} \mathbf{u}_{0,2}$ and $\mathbf{v}_{1,3}{\mathcal {L}}^{(1)}{\mathcal {L}}^{-1}{\mathcal {L}}^{(1)}\mathbf{u}_{0,2}$. The first four are:

$$\begin{aligned} \mathbf{v}_1{\mathcal {L}}^{(2)}{} \mathbf{u}_0&=\sigma ^2 M,\\ \mathbf{v}_1{\mathcal {L}}^{(2)}{} \mathbf{u}_2&=\mathbf{v}_3{\mathcal {L}}^{(2)}{} \mathbf{u}_0 =\sigma ^2\kappa T,\\ \mathbf{v}_3{\mathcal {L}}^{(2)}{} \mathbf{u}_2&= \sigma ^2(2ET-\omega M-\zeta U), \end{aligned}$$

where $U=\int _0^TF(A^2)\mathrm{d}y$. Three of the latter four follow from $\mathbf{v}_{0,2}{\mathcal {L}}^{(1)}{} \mathbf{u}_{0,2}$ due to the fact that ${\mathcal {L}}^{(1)}{} \mathbf{u}_0=2i\mathbf{u}_2$ and $\mathbf{v}_1{\mathcal {L}}^{(1)}=2i\mathbf{v}_3$. Thus

$$\begin{aligned} \mathbf{v}_1{\mathcal {L}}^{(1)}{\mathcal {L}}^{-1}{\mathcal {L}}^{(1)}{} \mathbf{u}_0&=2i\mathbf{v}_2{\mathcal {L}}^{(1)}{} \mathbf{u}_0\\&=2\sigma \left( \nu T+\frac{\sigma M}{2}\right) \end{aligned}$$

and (also using symmetry)

$$\begin{aligned} \mathbf{v}_1{\mathcal {L}}^{(1)}{\mathcal {L}}^{-1}{\mathcal {L}}^{(1)}\mathbf{u}_2=\mathbf{v}_3{\mathcal {L}}^{(1)}{\mathcal {L}}^{-1}{\mathcal {L}}^{(1)}\mathbf{u}_0= 2\sigma \left( \tau T+\frac{\sigma \kappa T}{2}\right) . \end{aligned}$$

Finally, it remains to compute $\mathbf{v}_{3}{\mathcal {L}}^{(1)}{\mathcal {L}}^{-1}{\mathcal {L}}^{(1)}\mathbf{u}_{2}$. First, we note that we can write

$$\begin{aligned} {\mathcal {L}}^{(1)}{} \mathbf{u}_2&= -i\sigma \left( \begin{array}{c}0\\ 2A_{yy}-2A(S_y)^2\end{array}\right) \\&= 2i\omega \mathbf{u}_0+2i\zeta \mathbf{u}_4, \end{aligned}$$

using Equations (11), (12), (13). Then we can write

$$\begin{aligned} \mathbf{v}_3{\mathcal {L}}^{(1)}{\mathcal {L}}^{-1}{\mathcal {L}}^{(1)} \mathbf{u}_2 = 2i\omega \mathbf{v}_3{\mathcal {L}}^{(1)}{} \mathbf{u}_1 +2i\zeta \mathbf{v}_3{\mathcal {L}}^{(1)}{} \mathbf{u}_5. \end{aligned}$$

We already know the first two terms, and the third can be integrated in a similar way as

$$\begin{aligned} \mathbf{v}_3{\mathcal {L}}^{(1)}{} \mathbf{u}_5 = -2i\sigma \left[ \frac{\xi T}{2}-\frac{\sigma U}{4}\right] . \end{aligned}$$

Finally, we can combine and simplify to obtain

$$\begin{aligned} \mathbf{v}_{3}{\mathcal {L}}^{(1)}{\mathcal {L}}^{-1}{\mathcal {L}}^{(1)}\mathbf{u}_{2} =2\sigma \left( \frac{2\omega \gamma T}{2}-\frac{2\omega \sigma M}{4} +\zeta \xi T-\frac{\sigma \zeta U}{2}\right) . \end{aligned}$$

Putting all these quantities together, we obtain expressions for all the matrix elements.