Skip to main content
SpringerLink
Log in

The Weisfeiler–Leman Dimension of Chordal Bipartite Graphs Without Bipartite Claw

  • Original Paper
  • Published:
Graphs and Combinatorics Aims and scope Submit manuscript

Abstract

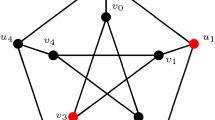
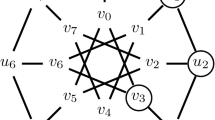
A graph X is said to be chordal bipartite if it is bipartite and contains no induced cycle of length at least 6. It is proved that if X does not contain bipartite claw as an induced subgraph, then the Weisfeiler–Leman dimension of X is at most 3. This implies that the Weisfeiler–Leman dimension of any bipartite permutation graph is at most 3. The proof is based on the theory of coherent configurations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Notes

  1. This closure is nothing else than the \(\alpha \)-extension of the coherent configuration \({{\,{\mathrm{WL}}\,}}(X)\), see [5, Definition 3.3.1].

References

  1. Arvind, V., Köbler, J., Rattan, G., Verbitsky, O.: Graph isomorphism, color refinement, and compactness. Comput. Complex. 26(3), 627–685 (2017)

    Article  MathSciNet  Google Scholar 

  2. Babai, L.: Graph isomorphism in quasipolynomial time. In: Proceedings of the 48th Annual ACM Symposium on Theory of Computing (STOC’16), pp. 684–697 (2016)

  3. Booth, K.S., Colbourn, C.J.: Problems polynomially equivalent to graph isomorphism, Technical Report CS-77-04, Comp. Sci. Dep., Univ. Waterloo (1979)

  4. Cai, J.-Y., Fürer, M., Immerman, N.: An optimal lower bound on the number of variables for graph identification. Combinatorica 12(4), 389–410 (1992)

    Article  MathSciNet  Google Scholar 

  5. Chen, G., Ponomarenko, I.: Coherent Configurations. Central China Normal University Press, Wuhan (2019)

    MATH  Google Scholar 

  6. Colbourn, C.J.: On testing isomorphism of permutation graphs. Networks 11, 13–21 (1981)

    Article  MathSciNet  Google Scholar 

  7. Dabrowski, K.K., Johnson, M., Paulusma, D.: Clique-width for hereditary graph classes. In: Lo, A., Mycroft, R., Perarnau, G., Treglown, A. (eds.) Surveys in Combinatorics 2019 (London Mathematical Society Lecture Note Series), pp. 1–56. Cambridge University Press, Cambridge (2019)

    Google Scholar 

  8. Durán, G., Grippo, L.N., Safe, M.D.: Structural results on circular-arc graphs and circle graphs: a survey and the main open problems. Discrete Appl. Math. 164(Part 2), 427–443 (2014)

    Article  MathSciNet  Google Scholar 

  9. Fuhlbrück, F., Köbler, J., Verbitsky, O.: Identiability of graphs with small color classes by the Weisfeiler–Leman algorithm. In: Proceedings of 37th International Symposium on Theoretical Aspects of Computer Science. Dagstühl Publishing, Germany, pp. 43:1–43:18 (2020)

  10. Gavrilyuk, A.L., Nedela, R., Ponomarenko, I.: The Weisfeiler–Leman dimension of distance-hereditary graphs, pp. 1–16. arXiv:2005.11766 [math.CO] (2020)

  11. Golumbic, M.C.: Algorithmic Graph Theory and Perfect Graphs. North-Holland Publishing Co., Amsterdam (2004)

    MATH  Google Scholar 

  12. Grohe, M.: Descriptive Complexity, Canonisation, and Definable Graph Structure Theory. Cambridge University Press, Cambridge (2017)

    Book  Google Scholar 

  13. Grohe, M., Kiefer, S.: A linear upper bound on the Weisfeiler–Leman dimension of graphs of bounded genus. In: 46th International Colloquium on Automata, Languages, and Programming (ICALP 2019), Article No. 117, pp. 117:1–117:15 (2019)

  14. Grohe, M., Neuen, D.: Canonisation and definability for graphs of bounded rank width. In: Proceedings of 2019 34th Annual ACM/IEEE Symposium on Logic in Computer Science (LICS), pp. 1–13 (2019)

  15. Kiefer, S., Schweitzer, P., Selman, E.: Graphs identified by logics with counting. In: Mathematical Foundations of Computer Science. Springer, Heidelberg, pp. 319–330 (2015)

  16. Kiefer, S., Ponomarenko, I., Schweitzer, P.: The Weisfeiler–Leman dimension of planar graphs is at most \(3\). J. ACM 66(6), Article 44 (2019)

    Article  MathSciNet  Google Scholar 

  17. Köbler, J., Kuhnert, S., Laubner, B., Verbitsky, O.: Interval graphs: canonical representations in logspace. SIAM J. Comput. 40(5), 1292–1315 (2011)

    Article  MathSciNet  Google Scholar 

  18. Koehler, E.: Graphs without asteroidal triples, Ph.D. Thesis, Berlin (1999)

  19. Uehara, R., Toda, S., Nagoya, T.: Graph isomorphism completeness for chordal bipartite graphs and strongly chordal graphs. Discrete Appl. Math. 145(3), 479–482 (2005)

    Article  MathSciNet  Google Scholar 

  20. Weisfeiler, B., Leman, A.: Reduction of a graph to a canonical form and an algebra which appears in the process. NTI 2(9), 12–16 (1968)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. St. Petersburg Department of the Steklov Mathematical Institute, St.Petersburg, Russia

    Ilia Ponomarenko

  2. Sobolev Institute of Mathematics, Novosibirsk, Russia

    Grigory Ryabov

  3. Novosibirsk State University, Novosibirsk, Russia

    Grigory Ryabov

Authors
  1. Ilia Ponomarenko
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Grigory Ryabov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Grigory Ryabov.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The work is supported by the Russian Foundation for Basic Research (Project 18-01-00752).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ponomarenko, I., Ryabov, G. The Weisfeiler–Leman Dimension of Chordal Bipartite Graphs Without Bipartite Claw. Graphs and Combinatorics 37, 1089–1102 (2021). https://doi.org/10.1007/s00373-021-02308-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00373-021-02308-7

Keywords

Search

Navigation