Abstract
We consider, within the framework of an improved \(5D\) Kaluza-Klein theory (KK\(\psi\)), the set of twenty most precise values ever published of Earth-based gravitational constant measurements. We take into account the possibility of a jump discontinuity into the geomagnetic potential in connection with the spin crossover of the iron (III) complex ions migrating from the Earth’s outer core into the deep Earth mantle as well as the spin crossover of the copper (III) complex ions migrating downwards within either the Earth’s crust or deep in the Earth’s mantle. Moreover, it turns out that the boundary conditions assigned to both internal, \(\phi\), and external, \(\psi\), scalar fields are sensitive to the aforementioned jump discontinuity too. Again, the fits to the experimental data are found in good agreement with the predictions of the linearized KK\(\psi\) theory. An estimate around the vacuum expectation values \(\psi=v\) and \(\phi=1\) of the second derivatives \({\partial^{2}f_{\textrm{EM}}}/{\partial\phi^{2}}(v,1)\), \({\partial^{2}f_{\textrm{EM}}}/{\partial\phi\partial\psi}(v,1)\) and \({\partial^{2}f_{\textrm{EM}}}/{\partial\psi^{2}}(v,1)\) of the coupling function, \(f_{\textrm{EM}}(\psi,\phi)\), of the electromagnetic field (EM) with \(\psi\) and \(\phi\), is provided for the first time, thereby enabling the determination of the product \(v\times f_{\textrm{EM}}(v,\phi)\) and \(v\times f_{\textrm{EM}}(\psi,1)\) in the second-order approximation from the experimental data.




Similar content being viewed by others
Notes
e.g., two simple pendulums with separation determined by a laser interferometer, a torsion balance-pendulum in the time-of-swing mode, a torsion balance pendulum using the angular-acceleration feedback, a torsion strip balance in the Cavendish mode, a torsion strip balance in electrostatic servo mode, a vertical atomic interferometer, a beam-balance (gravitational force of a large mercury mass on two copper cylinders with a modified commercial mass comparator).
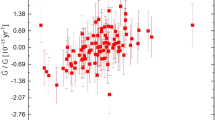
By comparing the gravitational constant values between the two groups that we have distinguished in our study.
Two equations can be inferred: \(\Delta V=0\) since \(\textrm{div}\textrm{curl}\vec{A}=0\) and \(\Delta\vec{A}=\vec{0}\) since \(\textrm{curl}\vec{\nabla}V=\vec{0}\) and on account of the Coulomb gauge \(\textrm{div}\vec{A}\)=0. Besides, let us notice that \(A^{2}+V^{2}=[{\mu_{0}M}/(4\pi r^{2})]^{2}\)
This particularity leads us to conjecture that there might be a hidden copper deposit sufficiently rich in copper (III) coordination compounds either in the Earth’s crust or deep in the Earth’s mantle at the longitude and latitude of the BIPM. There, the spin crossover of the copper (III) complex ion should occur, unlike the other laboratories mentioned in this study. Recently, large quantities of copper, lead and zinc have been unveiled within 200 kilometres of the transition between thick and thin lithosphere [31] and possibly in Earth’s deep mantle [32].
Let us notice that this force resembles the Poynting-Robertson light drag, \(\vec{F}={-}({P}/c^{2})\vec{v}\), where
$$P=\frac{1}{2}mc^{2}\left|\frac{dN}{dt}\right|=\frac{1}{2}|V_{0}|cI$$replaces the incident EM power. Also, as one can notice, this power \(P\) is akin to the Peltier heat per unit time generated at the junction of two conductors A and B whose Peltier coefficients would satisfy \(q(\Pi_{A}-\Pi_{B})=\frac{1}{2}mc^{2}\) when the current, \(I\), flows from A to B. By arranging one obtains
$$\vec{F}=-\frac{1}{2}\frac{|\dot{N}|}{N}\vec{p}={-}\frac{1}{2}\frac{mI}{|q|}\vec{v}={-}\frac{1}{2}|V_{0}|I\frac{\vec{v}}{c},$$where
$$\vec{p}=M\vec{v},\quad M=Nm,\quad\dot{N}=\frac{dN}{dt},\quad I=|q\dot{N}|.$$The work-energy theorem states that \(\Delta E_{c}=W\). Now, the change in the kinetic energy reads \(\Delta E_{c}=\frac{1}{2}Mv^{2}\), and the infinitesimal work
$$\delta W=\pm\frac{1}{2}\frac{V_{0}}{c}v^{2}dQ\quad\Rightarrow\quad W=\pm\frac{1}{2}\frac{QV_{0}}{c}v^{2}.$$Thus one obtains
$$\frac{1}{2}Mv^{2}=\pm\frac{1}{2}\frac{QV_{0}}{c}v^{2}\quad\Rightarrow\quad V_{0}=\pm\frac{Mc}{Q}=\pm\frac{mc}{q}.$$
REFERENCES
J. Luo and Z. K. Hu, Class. Quantum Grav. 17, 2351 (2000).
V. N. Melnikov, Arxiv prepint gr-qc/9903110.
J.P Mbelek and M. Lachièze-Rey, Arxiv prepint gr-qc/0012086.
J.P. Mbelek and M. Lachièze-Rey, Grav. Cosmol. 8, 331 (2002).
J.P. Mbelek, Grav. Cosmol. 25, 250 (2019).
E. Thébault et al., Earth planet and space 67, 79 (2015).
J.P. Mbelek, IJMPA 35, 2040027 (2020).
Z. K. Hu et al., Phys. Rev. D 71, 127505 (2005);
Z. K. Hu et al., Phys. Rev. D 71, 127505 (2005); J. Luo et al., Phys. Rev. D 59, 042001 (1999).
H. V. Parks and J. E. Faller, Phys. Rev. Letters 105, 110801 (2010);
H. V. Parks and J. E. Faller, Phys. Rev. Letters 105, 110801 (2010); Erratum: H. V. Parks and J. E. Faller, Phys. Rev. Letters 122, 199901 (2019).
Q. Li et al., Nature 560, 582 (2018).
T. J. Quinn, C. C. Speake, S. J. Richman, R. S. Davis, and A. A. Picard, Phys. Rev. Letters 87, 111101 (2001).
T. Quinn, C. Speake, H. Parks, and R. Davis, Phil. Trans. R. Soc. A 372, 20140032 (2014);
T. Quinn, C. Speake, H. Parks, and R. Davis, Phil. Trans. R. Soc. A 372, 20140032 (2014); T. Quinn, H. Parks, C. Speake, and R. Davis, Phys. Rev. Letters 111, 101102 (2013).
G. G. Luther and W. Towler, Phys. Rev. Letters 48, 121 (1982).
O. V. Karagioz and V. P. Izmailov, Measurement techniques 39, 979 (1996).
G. Rosi et al., Nature 510, 518 (2014).
J. Luo et al., Phys. Rev. Letters 102, 240801 (2009).
S. Schlamminger, E. Holzschuh and W. Kündig, Phys. Rev. Letters 89, 161102 (2002).
S. Schlamminger et al., Phys. Rev. D 74, 082001 (2006).
T. R. Armstrong and M. P. Fitzgerald, Phys. Rev. Letters 91, 201101 (2003).
S. Schlamminger, J. H. Gundlach, and R. D. Newman, Phys. Rev. D 91, 121101 (2015);
S. Schlamminger, J. H. Gundlach, and R. D. Newman, Phys. Rev. D 91, 121101 (2015); J. H. Gundlach and S. M. Merkowitz, Phys. Rev. Letters 85, 2869 (2000).
M. U. Sagitov et al., Dok. Acad. Nauk SSSR 245, 567 (1977).
U. Kleinevoss, Bestimmung der Newtonschen Gravitationskonstanten G, PhD thesis dissertation (Universität Wuppertal, Wuppertal, Germany, 2002).
C. H. Bagley and G. G. Luther, Phys. Rev. Letters 78, 3047 (1997).
R. Newman et al., Phil. Trans. R. Soc. A 372, 20140025 (2014).
V. V. Zelentsov, Russian Journal of Coordination Chemistry 29, 425 (2003).
Y. Ide et al., Dalton Trans. 46, 242 (2017).
S. Shankar et al., Nature Communications 9, 4750 (2018).
C. Krebs et al., Angewandte Chemie international Edition 38, 359 (1999).
B.S. Manhas et al., Synthesis and Reactivity in Inorganic and Metal-Organic Chemistry 29, 1009 (1999).
J. Meija et al., Pure and Applied Chemistry 88, 265 (2016).
M. J. Hoggard et al., Nature Geoscience 13, 504 (2020).
C. T. A. Lee et al., Science 336, 64 (2012).
P. R. Bevington and D. K. Robinson, Data Reduction and Error Analysis for the Physical Sciences (McGraw-Hill, New York, 2003), 3rd ed., pp. 204–208 and pp. 260–264.
S. S. Lobanov et al., arXiv: 1610.01048.
S. S. Lobanov et al., J. Geophys. Research: Solid Earth 122, 3565 (2017).
S. Layek et al., Phys. Rev. B 94, 125129 (2016).
M.-L. Boillot at al., New J. Chem. 26, 313 (2002).
X.-D. Che et al., Journal of Physical Chemistry Letters 8, 5587 (2017).
S. Speziale et al., PNAS 102, 17918 (2005).
Y. Aharonov and D. Bohm, Phys. Rev. 115, 485 (1959).
Author information
Authors and Affiliations
Corresponding author
Appendices
APPENDIX A
As one knows, the central ion of a complex ion can make a spin crossover (or spin transition/spin equilibrium) from a low spin state to a high spin state (or conversely) by absorbing (or emitting) a photon [34–36] or by migrating from a high-pressure area to a low-pressure area (or conversely). So, let us consider a distribution of identical monocinetic ions that constitute a current \(I\). We argue that these charged carriers, by making a pressure induced spin crossover at the Earth’s core-mantle boundary, will be subject to a drag force,
while creating in turn a jump discontinuity equal to \(\pm V_{0}\) in the geomagnetic potentialFootnote 5 As such, this implies an infinitesimal workFootnote 6 equal to
Hence the expression of the infinitesimal variation of the internal energy is
and consequently, the expression of the infinitesimal variation of the total energy
where \(E_{c}=\frac{1}{2}mv^{2}\), \(E_{c}^{\prime}=\frac{1}{2}mv^{\prime 2}\), \(dQ=qdN\), \(dQ^{\prime}=qdN^{\prime}\), \(Y_{i}\) is the intensive quantity associated to the extensive quantity \(X_{i}\), \(N\) and \(N^{\prime}\) denote the numbers of particles of mass \(m\) and electric charge \(q\) all identical up to the spin state, where the prime (respectively the absence of prime) stands for the population of those particles either in the high spin state (respectively. the low spin state) which undergoes a pressure-induced spin-crossover (see [36–39]) thereby increasing the number of population in the low spin state (respectively, the high spin state). Furthermore, \(dE_{c}=0\) and \(dE_{c}^{\prime}=0\), for ions in uniform motion. By neglecting the variation of the altitude, Eq. (A.4) reduces to
Moreover, for an isolated system in thermodynamic equilibrium, \(\mu^{\prime}=\mu\), \(N^{\prime}+N=\textrm{const}\), the total energy \(E_{\textrm{total}}\) is conserved, and all \(X_{i}\) (including the entropy) are assumed constant. Consequently, since \(E_{p}^{\prime}=E_{p}\) at the boundary where the spin crossover occurs, Eq. (A.5) reduces in turn to
Let us emphasize that Eq. (A.6) holds only if either the cations are subject to no drag force and no spin crossover, in which case \(v^{\prime}=v\) with respect to the terrestrial reference frame, or otherwise \(mc\pm qV_{0}=0\) during the spin crossover and \(v^{\prime}\neq v\) in accordance with the observed velocity gradient. This means that the spin transition of iron (III) cations from the high spin state to the low spin state (or converserly) in the deep mantle of the Earth above (or below) a certain pressure and temperature, will also involve a jump discontinuity of the geomagnetic potential of an amount, \(V_{0}\), such that
We argue that the above formula corresponds solely to a spin transition \(\Delta s=\pm 1\). For \(\Delta s\neq\pm 1\), it generalizes either to
or to
Peculiarly, when the spin crossover does not occur, \(\Delta V=V_{0}=0\) since \(\Delta s=0\). When \(n\) various species undergo the spin crossover at the same time at a point, according to the principle of superposition, Eq. (A.8) rewrites as
where \(\Delta s_{i}=s_{i}({\textrm{final}})-s_{i}({\textrm{initia}}l)\), hence \(\Delta s_{i}= s_{i}({\textrm{low}})-s_{i}({\textrm{high}})\) for a spin transition from high spin to low spin and \(\Delta s_{i}=s_{i}(h{\textrm{igh}})-s_{i}({\textrm{low}})\) for a spin transition from low to high spin.
APPENDIX B
Let us recall that the (pseudo)-scalar or vector electromagnetic potentials have no direct physical meaning in classical physics because of their arbitrary choice due to the gauge invariance. However, things change drastically in quantum mechanics, as can be seen in the Aharonov–Bohm effect [40]. Throughout, based on quantum-mechanical considerations not requiring any quantum gravity treatment still lacking hitherto, as a first attempt we provide a semiclassical justification of the relation between jump discontinuities in the geomagnetic potential and subsequently in the scalar fields \(\psi\) and \(\phi\) through Eqs. (8, (9). Indeed, the vector potential \(\vec{A}\) created in space by a magnetic dipole moment \(\vec{M}\) reads (see Section 3)
where \(\vec{E}=\dfrac{Nq\vec{r}}{4\pi\epsilon_{0}r^{3}}\) denotes the electric field created radially by a set of \(N\) identical central ions of electric charge \(q\) and mass \(m\) at a point defined by the position vector \(\vec{r}\) within a domain \((\Omega)\) of volume \(V_{\Omega}\). Now, the magnetic flux \(\Phi\) reads
where we have set \(\Theta=(\vec{M};\vec{r})\). Moreover, the pseudoscalar geomagnetic potential reads (see Section 3)
Now, the standard deviations
and
respectively, of \(V\) and \(\Phi\) reduce to
on account that both means \(V\) and \(\Phi\) are equal to zero, where we have set
So, by combining Eqs. (B.2) and (B.3), it follows
Analogously to the case of the Aharonov–Bohm effect, the standard deviation of the fluctuating magnetic flux associated to random jump discontinuities in the geomagnetic potential due to the spin crossover of identical central ions of mass \(m\), should be quantified through circular loops of radius \(l/(2\pi)=\hbar/(mc)\) (Compton wavelength of a particle of mass \(m\)). Thus,
and accordingly,
where \(k\) is an integer.
Rights and permissions
About this article
Cite this article
Mbelek, J.P., Ungem, L.B. Evidence for an Effective Gravitational Constant from the Laboratory Measurements. Gravit. Cosmol. 27, 54–62 (2021). https://doi.org/10.1134/S0202289321010138
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0202289321010138