Abstract
Five years ago a generalization of Kepler’s third law was formulated (Dmitrašinović and Šuvakov in Phys Lett A 379:1939–1945, 2015) for three-body orbits in Newtonian gravity with equal masses and vanishing angular momentum. In the meantime this linear relation between the scale-invariant period and the algebraic description of the orbit’s topology has been confirmed (Li and Liao in Sci China Phys Mech Astron 60(12):129511, 2017) in about 700 topologically distinct orbits with equal masses and vanishing angular momentum and theoretically explained (Dmitrašinović et al. in J Phys A Math Theor 51:315101, 2018) as a consequence of holomorphy of the action integral. More recently, Li et al. (Publ Astron Soc Jpn 70:64, 2018) presented 1349 unequal-mass periodic 3-body orbits with vanishing angular momentum. On that basis a “generalized Kepler’s third law” for unequal-mass orbits was proposed with a specific mass dependence of the “general” scale-invariant period. First, we show that Euler’s 3-body orbit does not satisfy this “generalized Kepler’s third law”. Then we discuss the general implications of scaling symmetry, and of the virial theorem in particular, on periods of 3-body orbits in Newtonian gravity, to show that in the absence of a universal \(S_3\) symmetry under permutations of three bodies in all periodic orbits there can be no truly universal Kepler’s third law for 3-body orbits. Orbits with definite permutation symmetries, however, may have similar, though not necessarily identical mass dependences of their periods, in the absence of angular momentum. All 1349 orbits in Li et al. (Publ Astron Soc Jpn 70:64, 2018) are symmetric under permutations of two equal-mass bodies, though not of the third one, even when the third body has a mass that is equal to the other two. That reduced \(S_2\) permutation symmetry allows for the possibility that this class of orbits has a common mass dependence. We have re-analyzed the mass dependence of these 1349 orbits subject to the normalization condition that all orbits must have the same sum of masses. The extracted mass-ratio dependencies of the scale-invariant period (normalized by the topology) for various families of orbits are generally different for different topological families of orbits. Thus there is no generalized Kepler’s third law for 3-body orbits with different masses either universally or in this subset of orbits.




Similar content being viewed by others
Notes
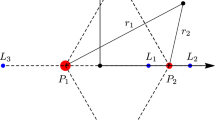
One may introduce a 3-body variable with the dimension of length that involves all three bodies, a.k.a. hyper-radius R, and use its maximum \(R_{max}\), or (some) average value \(\bar{R}\) instead of the semi-major axis in Kepler’s third law: \(T \sim R_{max, avrg}^{3/2}\).
Huggett and Jordan [18] say: “Topology has been referred to as rubber-sheet geometry” and ”Topology is geometry without distance or angle. The geometrical objects of study, not rigid but rather made of rubber or elastic, are especially stretchy.” Wikipedia: ”In mathematics, topology (from the Greek \(\tau {\omega }\pi \omega \sigma \), place, and \(\lambda {\omega } \gamma \omega \sigma \), study) is concerned with the properties of space that are preserved under continuous deformations, such as stretching, twisting, crumpling and bending, but not tearing or gluing.”
This in apparent agreement with the numerical 3-body data
Even in this limited class of orbits, such as the choreographic one, there is an ambiguity, with regard to the characteristic mass function \(M_N\): One can use any permutation-symmetric homogeneous function \(M_N(m_i)\) of three masses \(m_{i}, i=1,2,3\), of which there are (infinitely) many. For example, there are the following permutation-symmetric functions at one’s disposal: \(M = \sum _{i}m_{i}\), \(1/M_1 = \sum _{i}1/m_{i}\), \(\sqrt{M_2} = \sum _{i}\sqrt{m_{i}}\), \(1/\sqrt{M_3} = \sum _{i}\sqrt{1/m_{i}}\), \(M_4^2 = \sum _{i>j}m_{i}m_{j}\), \(1/M_5^2 = \sum _{i>j}1/(m_{i}m_{j})\), \(m_6 = \sum _{i>j} \sqrt{m_{i}m_{j}}\), \(1/M_7 = \sum _{i>j} 1/\sqrt{m_{i}m_{j}}\), etc..
As the period scales as \(T \rightarrow \sqrt{\frac{3}{\sum _i^3 m_i}} T\), i.e., the mass-scale-invariant period is \(\sqrt{\sum _i^3 m_i} T\), i.e., all orbits’ periods ought to be evaluated at the same value of the sum of masses: \(M = \sum _i^3 m_i = const. \). And the energy E, which by the virial theorem is one half of the potential energy averaged over one period, may be taken (following Lagrange solution’s example) as \(E = - \frac{1}{2} \sum _{i<j} m_i m_j\); thus the mass-scale-invariant energy is \(E (\sum _{i<j} m_i m_j)^{-1}\).
Liao [27] informed us that: “The reason why you can not find the linear curve may be because we gained the mean of scale-invariant average periods for different mass \(m_3\), then we fitted the mean of averages periods and \(m_3\). Indeed, the fitted line is an approximated formula. It agrees well with numerical results (the estimated error is about 1%) when \(m_3\) is larger than 1. Since we only studied the small mass \(m_3\) = 0.5 and 0.75, it can not give too much information for small \(m_3\). For small \(m_3\), the relation maybe more complicated. It needs further study.”.
If we accept the fitted formula, then we are obliged to conclude that the period vanishes at \(m_3/m = 0.2\), which is in manifest conflict with all the periodic general three-body orbits that are continuously connected with periodic orbits of the restricted 3-body problem, such as the Lagrange and Euler ones. Indeed, these two well-known solutions’ periods manifestly disobey the proposed “generalized Kepler’s third law for three-body orbits”.
Whereas the zero- and infinite-mass limits discussed above are (exactly) dual in the two-body case, i.e., one implies the other, that is not so in the general 3-body problem.
This disagrees with Liao’ private communication [27], which claims 19, whereas we found 20.
Of course, we need not have reanalyzed all these orbits just for the sake of that one conclusion, as the same conclusion could have been drawn from a single counter-example, such as the (well-known) Euler orbit.
References
Landau LD, Lifshitz EM (1976) Mechanics, 3rd edn. Butterworth-Heinemann, Oxford
Danby JMA (1992) Fundamentals of celestial mechanics. Willmann-Bell, Richmond
Broucke R, Boggs D (1975) Periodic orbits in the planar general three-body problem. Celest Mech 11:13
Broucke R (1975) On relative periodic solutions of the planar general three-body problem. Celest Mech 12:439
Hadjidemetriou JD (1975) The continuation of periodic orbits from the restricted to the general three-body problem. Celest Mech 12:155
Hadjidemetriou JD, Christides T (1975) Families of periodic orbits in the planar three-body problem. Celest Mech 12:175
Hadjidemetriou JD (1975) The stability of periodic orbits in the three-body problem. Celest Mech 12:255
Hénon M (1976) A family of periodic solutions of the planar three-body problem, and their stability. Celest Mech 13:267
Moore C (1993) Braids in classical gravity. Phys Rev Lett 70:3675
Janković MR, Dmitrašinović V (2016) Angular momentum and topological dependence of Kepler’s third law in the Broucke-Hadjidemetriou-Hénon family of periodic three-body orbits. Phys Rev Lett 116(5):064301
Šuvakov M, Dmitrašinović V (2013) Three classes of Newtonian three-body planar periodic orbits. Phys Rev Lett 110(11):114301
Li X, Liao S (2017) More than six hundred new families of Newtonian periodic planar collisionless three-body orbits. Sci China Phys Mech Astron 60(12):129511
Dmitrašinović V, Hudomal A, Shibayama M, Sugita A (2018) Linear Stability of periodic three-body orbits with zero angular momentum and topological dependence of Kepler’s third law: a numerical test. J Phys A Math Theor 51:315101
Li X, Liao S (2019) Collisionless periodic orbits in the free-fall three-body problem. New Astronomy 70:22–26. arXiv:1805.07980v1 (nlin)
Li XM, Jing YP, Liao SJ (2018) Over a thousand new periodic orbits of a planar three-body system with unequal masses. Publ Astron Soc Jpn 70:64
Montgomery R (1998) The N-body problem, the braid group, and action-minimizing periodic solutions. Nonlinearity 11:363–376
Tanikawa K, Mikkola S (2008) A trial symbolic dynamics of the planar three-body problem. In: Orlov VV, Rubinov AV (ed.) Proceedings of the resonances, stabilization, and stable chaos in hierarchical triple systems. St. Petersburg State University Press, St. Petersburg, pp. 26. (arXiv:0802.2465)
Huggett S, Jordan D (2009) A topological aperitif. Springer, London
Dmitrašinović V, Šuvakov M (2015) Topological dependence of Kepler’s third law for planar periodic three-body orbits with vanishing angular momentum. Phys Lett A 379:1939–1945
Hénon M (1977) Stability of interplay motions. Celest Mech 15:243
Hénon M (1974) Families of periodic orbits in the three-body problem. Celest Mech 10:375–388
Šuvakov M, Dmitrašinović V (2014) A guide to hunting periodic three-body orbits. Am J Phys 82:609–619
Orlov VV, Petrova AV, Martynova AI (2003) Classification of orbits in the plane isosceles three-body problem. Month Not R Astron Soc 333:495–500
Orlov VV, Petrova AV, Rubinov AV, Martynova AI (2004) Periodic orbits in the general three-body problem and the relationship between them. Astron Lett 30:349–356
Martynova AI, Orlov VV, Sokolov LL (2005) Analysis of the neighborhood of the 2: 1 resonance in the equal-mass three-body problem. Astron Lett 31(3):213–219
Sun B (2018) Kepler’s third law of n-body periodic orbits in a Newtonian gravitation field. Sci China Phys Mech Astron 61:054721. https://doi.org/10.1007/s11433-017-9154-0
Shijun L (2020) Private communication
Li X, Li X, Liao S (2020) One family of 13315 stable periodic orbits of the non-hierarchical unequal-mass triple system ArXiv:2007.10184
Acknowledgements
We thank Dr. Shijun Liao for clarifying communications and Dr. Igor Salom for good advice. This work was done in the summer of 2019 when B.R. was visiting Institute of Physics Belgrade as a summer intern, as part of summer activities approved by École Polytechnique.
Funding
This study was funded by the Serbian Ministry of Science and Technological Development (grants number OI 171037 and III 41011), and partially by IPB through grant by MESTD Republic of Serbia.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of Interest
The authors declare that they have no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Raonić, B., Dmitrašinović, V. On “generalized Kepler’s third law” and mass dependence of periods of three-body orbits. Meccanica 56, 1011–1024 (2021). https://doi.org/10.1007/s11012-021-01328-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11012-021-01328-0