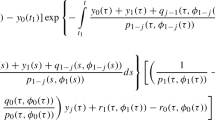Skip to main content
SpringerLink
Log in

Solitonic Combinations, Commuting Nonselfadjoint Operators, and Applications

  • Published:
Complex Analysis and Operator Theory Aims and scope Submit manuscript

Abstract

In this paper, we consider applications of the connection between the soliton theory and the commuting nonselfadjoint operator theory, established by Livšic and Avishai. An approach to the inverse scattering problem and to the wave equations is presented, based on the Livšic operator colligation theory (or vessel theory) in the case of commuting bounded nonselfadjoint operators in a Hilbert space, when one of the operators belongs to a larger class of nondissipative operators with asymptotics of the corresponding nondissipative curves. The generalized Gelfand–Levitan–Marchenko equation of the cases of different differential equations (the Korteweg–de Vries equation, the Schrödinger equation, the Sine–Gordon equation, the Davey–Stewartson equation) are derived. Relations between the wave equations of the input and the output of the generalized open systems, corresponding to the Schrödinger equation and the Korteweg–de Vries equation, are obtained. In these two cases, differential equations (the Sturm–Liouville equation and the 3-dimensional differential equation), satisfied by the components of the input and the output of the corresponding generalized open systems, are derived.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Alpay, D., Melnikov, A., Vinnikov, V.: Schur algorithm in the class SI of J-contractive functions intertwining solutions of linear differential equations. Integral Equ. Oper. Theory 74(3), 313–344 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  2. Borisova, G.S.: A new form of the triangular model of M.S. Livšic for a class of nondissipative operators. Comptes Rendus de l’Acadèmie bulgare des Sciences 53(10), 9–12 (2000)

  3. Borisova, G.S.: The operators \(A_{\gamma }=\gamma A+\overline{\gamma }A^*\) for a class of nondissipative operators \(A\) with a limit of the corresponding correlation function. Serdica Math. J. 29, 109–140 (2003)

    MathSciNet  Google Scholar 

  4. Borisova, G.S.: The connection between the Sturm-Liouville systems and the triangular model of couplings of dissipative and antidissipative operators. Comptes Rendus de l’Acadèmie bulgare des Sciences Tome 69(5), 563–572 (2016)

    MathSciNet  MATH  Google Scholar 

  5. Borisova, G.S.: Commuting nonselfdjoint operators, open systems, and wave equations. Comptes Rendus de l’Acadèmie bulgare des Sciences Tome 74(2), 157–165 (2021)

  6. Borisova, G.S., Kirchev, K.P.: Solitonic combinations and commuting nonselfadjoint operators. J. Math. Anal. Appl. 424, 21–48 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  7. Hristov, M.: On Bourgain algebras of backward shift invariant algebras and their subalgebras. Comptes Rendus de l’Acadèmie bulgare des Sciences 67(4), 449–458 (2014)

    MathSciNet  MATH  Google Scholar 

  8. Hristov, M.: Bourgain algebras of subalgebras of \(H^{\infty } (D)\) on the unit disk. Comptes Rendus de l’Acadèmie bulgare des Sciences 68(2), 141–149 (2015)

    MathSciNet  MATH  Google Scholar 

  9. Kirchev, K.P., Borisova, G.S.: Commuting nonselfadjoint operators and their characteristic operator-functions. Serdica Math. J. 23, 313–334 (1997)

    MathSciNet  MATH  Google Scholar 

  10. Kirchev, K.P., Borisova, G.S.: Nondissipative curves in Hilbert spaces having a limit of the corresponding correlation function. Integral Equ. Oper. Theory 40, 309–341 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  11. Kirchev, K., Borisova, G.: A triangular model of regular couplings of dissipative and anridissipative operators. Comptes rendus de l’Acadèmie bulgare des sciences 58(5), 481–486 (2005)

    MathSciNet  MATH  Google Scholar 

  12. Kirchev, K.P., Borisova, G.S.: Triangular models and asymptotics of continuous curves with bounded and unbounded semigroup generators. Serdica Math. J. 31, 95–174 (2005)

    MathSciNet  MATH  Google Scholar 

  13. Kirchev, K.P., Borisova, G.S.: Regular couplings of dissipative and anti-dissipative unbounded operators, asymptotics of the corresponding non-dissipative processes and the scattering theory. Integral Equ. Oper. Theory 57, 339–379 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  14. Livšic, M.S.: Operators, oscilations, waves (open systems). Transl. Math. Monogr. 34(5), (1972)

  15. Livšic, M.S.: Commuting operators and fields of systems, distributted in Euclidean space. Oper. Theory Adv. Appl. 4(5), (1982)

  16. Livšic, M.S.: System theory and wave dispersion. In: Proceedings of MTNS-83, Springer, New York (1984)

  17. Livšic, M.S.: Commuting nonselfadjoint operators and mapping of vector bundles on algebraic curves. Oper. Theory Adv. Appl. 19, 255–277 (1986). (Proceedings Workshop Amsterdam, June Y-7, Birkhauser (1985))

    MathSciNet  MATH  Google Scholar 

  18. Livšic, M.S.: Cayley–Hamilton theorem, vector bundles and divisors of commuting operators. Integral Equ. Oper. Theory 6(1), 250–273 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  19. Livšic, M.S.: Commuting nonselfadjoint operators and collective motions of systems. In: Commuting Nonselfadjoint Operators in Hilbert Space. Lecture Notes in Mathematics, vol 1272, 1–38. Springer, Berlin, Heidelberg (1987). https://doi.org/10.1007/BFb0078926

  20. Livšic, M.S.: What is a particle from the standpoint of system theory. Integral Equ. Oper. Theory 14, 552–563 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  21. Livšic, M.S., Kravitsky, N., Markus, A.S., Vinnikov, V.: Theory of Commuting Nonselfadjoint Operators. Kluwer Academic Publisher Group, Dordrecht (1995)

    Book  MATH  Google Scholar 

  22. Livšic, M.S., Avishai, Y.: A study of solitonic combinations based on the theory of commuting non-self-adjoint operators. Linear Algebra Appl. 122(123/124), 357–414 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  23. Livšic, M.S.: Vortices of 2D systems, operator theory, system theory and related topics. Oper. Theory Adv. Appl. 123, 7–41 (2001)

    MathSciNet  MATH  Google Scholar 

  24. Marchenko, V.A.: Nonlinear Equations and Operator Algebra. Naukova Dumka, Kiev (1986). (in Russian)

  25. Melnikov, A., Shusterman, R.: Solution of the Boussinesq equation using evolutionary vessels. Quantum Stud. Math. Found. 6(3), 335–351 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  26. Melnikov, A., Shusterman, R.: Evolution of nodes and their application to completely integrable PDEs. In: Linear Systems, Signal Processing and Hypercomplex Analysis, pp. 239–250. Birkháuser, Cham (2019)

  27. Melnikov, A.: Solution of the Korteweg–de Vries equation on the line with analytic initial potential. J. Math. Phys. 55(10), 101503 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  28. Melnikov, A.: Classification of KdV vessels with constant parameters and two dimensional outer space. Complex Anal. Oper. Theory 9(6), 1433–1450 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  29. Melnikov, A.: Construction of a Sturm–Liouville vessel using Gelfand–Levitan theory. On solution of the Korteweg–de Vries equation in the first quadrant. J. Math. Phys. 58, 051501 (2017). https://doi.org/10.1063/1.4980015

    Article  MathSciNet  MATH  Google Scholar 

  30. Melnikov, A.: On construction of solutions of evolutionary nonlinear Schrödinger equation. Int. J. Partial Differ. Equ. 1, Article ID 830413 (2015). http://dx.doi.org/10.1155/2014/830413

  31. Melnikov, A.: Inverse scattering of canonical systems and their evolution. Complex Anal. Oper. Theory 9, 793–819 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  32. Mysohata, S.: Theory of Partial Differential Equations, Moscow (1977). (in Russian)

  33. Scott, A.C., Chu, F.V.F., McLaughlin, D.W.: The soliton: a new concept in applied science. Proc. IEEE 63, 1443–1483 (1973)

    Article  MathSciNet  Google Scholar 

  34. Waksman, L.: Harmonic analysis of multi-parameter semigroups of contractions. In: Commuting Nonselfadjoint Operators in Hilbert Space. Lecture Notes in Mathematics, vol. 1272, pp. 39–114. Springer, Berlin, Heidelberg (1987). https://doi.org/10.1007/BFb0078927

  35. Zolotarev, V.A.: Time cones and a functional model on a Riemann surface. Math. USSR-Sb. 181, 965–995 (1990). ((English translation of Math. sb. 70, 399-429 (1991)))

    MathSciNet  Google Scholar 

Download references

Acknowledgements

I would like to express my thanks to the referee for his useful comments and advice.

Author information

Authors and Affiliations

  1. Faculty of Mathematics and Informatics, Konstantin Preslavsky University of Shumen, 9700, Shumen, Bulgaria

    Galina S. Borisova

Authors
  1. Galina S. Borisova
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Galina S. Borisova.

Additional information

Communicated by Gerald Teschl.

Dedicated to the memory of prof. Kiril P. Kirchev.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

This article is part of the topical collection “Spectral Theory and Operators in Mathematical Physics” edited by Jussi Behrndt, Fabrizio Colombo and Sergey Naboko.

Partially supported by the National Scientific Program “Information and Communication Technologies for a Single Digital Market in Science, Education and Security (ICTinSES)”, financed by the Ministry of Education and Science, and partially supported by Scientific Research Grant RD-08-42/2021 of University of Shumen. .

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Borisova, G.S. Solitonic Combinations, Commuting Nonselfadjoint Operators, and Applications. Complex Anal. Oper. Theory 15, 45 (2021). https://doi.org/10.1007/s11785-021-01086-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11785-021-01086-7

Keywords

Mathematics Subject Classification

Search

Navigation