Skip to main content
SpringerLink
Log in

Fractional Nonlocal Elasticity and Solutions for Straight Screw and Edge Dislocations

  • Published:
Physical Mesomechanics Aims and scope Submit manuscript

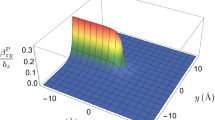
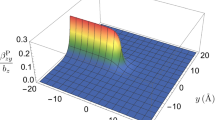
Abstract

Nonlocal elasticity assumes integral stress constitutive equation, takes into account interatomic long-range forces, reduces to the classical theory of elasticity in the long wave-length limit and to the atomic lattice theory in the short wave-length limit. In this paper, we propose the nonlocal elasticity theory with the kernel (the weight function in the integral stress constitutive equation) which is the Green function of the Cauchy problem for the fractional diffusion equation and is expressed in terms of the Mainardi function and Wright function being the generalizations of the exponential function. The solutions for the straight screw and edge dislocations in an infinite solid are obtained in the framework of the proposed theory.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1.
Fig. 2.

Similar content being viewed by others

REFERENCES

  1. Panin, V.E., Likhachev, V.A., and Grinyaev, Yu.V., Structural Levels of Deformation in Solids, Novosibirsk: Nauka, 1985.

  2. Eringen, A.C., Vistas on Nonlocal Continuum Physics, Int. J. Eng. Sci., 1992, vol. 30, no. 10, pp. 1551–1565.

  3. Eringen, A.C., Nonlocal Continuum Field Theories, New York: Springer, 2002.

  4. Panin, V.E. and Grinyaev, Yu.V., Physical Mesomechanics: A New Paradigm at the Interface of Solid State Physics and Solid Mechanics, Phys. Mesomech., 2003, vol. 6, no. 4, pp. 7–32.

  5. Panin, V.E., Egorushkin, V.E., and Panin, A.V., Physical Mesomechanics of a Deformed Solid as a Multilevel System. I. Physical Fundamentals of the Multilevel Approach, Phys. Mesomech., 2006, vol. 9, no. 3–4, pp. 9–20.

  6. Hirth, J.P. and Lothe, J., Theory of Dislocations, New York: McGraw-Hill, 1968.

  7. Landau, L.D. and Lifshitz, E.M., Theory of Elasticity, Oxford: Pergamon Press, 1974.

  8. Likhachev, V.A. and Khairov, R.Yu., Introduction to the Theory of Disclinations, Leningrad: Leningrad University Press, 1975.

  9. Teodosiu, C., Elastic Models of Crystal Defects, Berlin: Springer, 1982.

  10. Phillips, R., Crystal, Defects and Microstructures: Modeling Across Scales, Cambridge: Cambridge University Press, 2001.

  11. Balluffi, R.W., Introduction to Elasticity Theory for Crystal Defects, Singapore: World Scientific, 2016.

  12. Podstrigach, Ya.S., On a Nonlocal Theory of Solid Body Deformation, Sov. Appl. Mech., 1967, vol. 3, no. 2, pp. 44–46.

  13. Kröner, E., Elasticity Theory of Materials with Long Range Cohesive Forces, Int. J. Solids Struct., 1967, vol. 3, no. 5, pp. 731–742.

  14. Krumhansl, J.A., Some Considerations of the Relation between Solid State Physics and Generalized Continuum Mechanics, in Mechanics of Generalized Continua, Kröner, E., Ed., Berlin: Springer, 1968, pp. 298–311.

  15. Kunin, I.A., The Theory of Elastic Media with Microstructure and the Theory of Dislocations, in Mechanics of Generalized Continua, Kröner, E., Ed., Berlin: Springer, 1968, pp. 321–329.

  16. Edelen, D.G.B., Nonlocal Field Theories, in Continuum Physics. V. 4. Polar and Non-local Field Theories, Eringen, A.C., Ed., New York: Academic Press, 1976, pp. 75–204.

  17. Eringen, A.C., Linear Theory of Nonlocal Elasticity and Dispersion of Plane Waves, Int. J. Eng. Sci., 1972, vol. 10, no. 5, pp. 425–435.

  18. Eringen, A.C., Nonlocal Polar Field Theories, in Continuum Physics. V. 4. Polar and Nonlocal Field Theories, Eringen, A.C., Ed., New York: Academic Press, 1976, pp. 205–267.

  19. Kunin, I.A., Elastic Media with Microstructure. I. One-Dimensional Models, Berlin: Springer, 1982.

  20. Kunin, I.A., Elastic Media with Microstructure. II. Three-Dimensional Models, Berlin: Springer, 1983.

  21. Ghavanloo, E., Rafii-Tabar, H., and Fazelzadeh, S.A., Computational Continuum Mechanics of Nanoscopic Structures: Nonlocal Elasticity Approaches, Cham: Springer, 2019.

  22. Kovács, I. and Vörös, G., Lattice Defects in Nonlocal Elasticity, Physica B, 1979, vol. 96, no. 1, pp. 111–115.

  23. Povstenko, Y.Z., Point Defect in a Nonlocal Elastic Medium, J. Math. Sci., 2001, vol. 104, no. 5, pp. 1501–1505.

  24. Eringen, A.C., Edge Dislocation in Nonlocal Elasticity, Int. J. Eng. Sci., 1977, vol. 15, no. 3, pp. 177–183.

  25. Eringen, A.C., Screw Dislocation in Nonlocal Elasticity, J. Phys. D. Appl. Phys., 1977, vol. 10, no. 5, pp. 671–678.

  26. Eringen, A.C., On Differential Equations of Nonlocal Elasticity and Solutions of Screw Dislocation and Surface Waves, J. Appl. Phys., 1983, vol. 54, no. 9, pp. 4703–4710.

  27. Lazar, M. and Agiasofitou, E., Screw Dislocation in Nonlocal Anisotropic Elasticity, Int. J. Eng. Sci., 2011, vol. 49, no. 12, pp. 1404–1414.

  28. Povstenko, Y.Z., Straight Disclinations in Nonlocal Elasticity, Int. J. Eng. Sci., 1995, vol. 33, no. 4, pp. 575–582.

  29. Povstenko, Y.Z., Circular Dislocation Loops in Non-Local Elasticity, J. Phys. D. Appl. Phys., 1995, vol. 28, no. 1, pp. 105–111.

  30. Povstenko, Y.Z. and Matkovskii, O.A., Circular Disclination Loops in Nonlocal Elasticity, Int. J. Solids Struct., 2000, vol. 37, no. 44, pp. 6419–6432.

  31. Ari, N. and Eringen, A.C., Nonlocal Stress Field at Griffith Crack, Cryst. Lattice Deform. Amorph. Mater., 1983, vol. 10, pp. 33–38.

  32. Allegri, G. and Scarpa, F., On the Asymptotic Crack-Tip Stress Fields in Nonlocal Orthotropic Elasticity, Int. J. Solids Struct., 2014, vol. 51, no. 2, pp. 504–515.

  33. Nowinski, J.L., On the Three-Dimensional Boussinesq Problem for an Elastic Nonlocal Medium, Int. J. Eng. Sci., 1990, vol. 28, no. 12, pp. 1245–1251.

  34. Artan, R., Nonlocal Elastic Half Plane Loaded by a Concentrated Force, Int. J. Eng. Sci., 1996, vol. 34, no. 8, pp. 943–950.

  35. Povstenko, Y.Z., Axisymmetric Ring Loading in Nonlocal Elastic Space, Int. J. Eng. Sci., 2001, vol. 39, no. 3, pp. 285–302.

  36. Povstenko, Y.Z. and Kubik, I., Concentrated Ring Loading in a Nonlocal Elastic Medium, Int. J. Eng. Sci., 2005, vol. 43, no. 5–6, pp. 457–471.

  37. Podlubny, I., Fractional Differential Equations, San Diego: Academic Press, 1999.

  38. Magin, R.L., Fractional Calculus in Bioengineering, Redding: Begell House Publishers, 2006.

  39. Mainardi, F., Fractional Calculus and Waves in Linear Viscoelasticity: An Introduction to Mathematical Models, London: Imperial College Press, 2010.

  40. Tarasov, V.E., Fractional Dynamics: Applications of Fractional Calculus to Dynamics of Particles, Fields and Media, Berlin: Springer, 2010.

  41. Uchaikin, V.V., Fractional Derivatives for Physicists and Engineers, Berlin: Springer, 2013.

  42. Atanackovic, T.M., Pilipovic, S., Stankovic, B., and Zorica, D. Fractional Calculus with Applications in Mechanics: Vibrations and Diffusion Processes, Hoboken: John Wiley & Sons, 2014.

  43. Herrmann, R., Fractional Calculus: An Introduction for Physicists, Singapore: World Scientific, 2014.

  44. Povstenko, Y., Fractional Thermoelasticity, New York: Springer, 2015.

  45. Povstenko, Y., Linear Fractional Diffusion-Wave Equation for Scientists and Engineers, New York: Birkhäuser, 2015.

  46. Datsko, B. and Gafiychuk, V., Complex Spatio-Temporal Solutions in Fractional Reaction-Diffusion Systems near a Bifurcation Point, Fract. Calc. Appl. Anal., 2018, vol. 21, no. 1, pp. 237–253.

  47. Di Paola, M. and Zingales, M., Long-Range Cohesive Interactions of Non-Local Continuum Faced by Fractional Calculus, Int. J. Solids Struct., 2008, vol. 45, no. 21, pp. 5642–5659.

  48. Challamel, N., Zorica, D., Atanackovic, T.M., and Spasic, D.T., On the Fractional Generalization of Eringen’s Nonlocal Elasticity for Wave Propagation, C.R. Mecanique, 2013, vol. 341, no. 3, pp. 298–303.

  49. Carpinteri, A., Cornetti, P., and Sapora, A., Nonlocal Elasticity: An Approach Based on Fractional Calculus, Meccanica, 2014, vol. 49, no. 11, pp. 2551–2569.

  50. Tarasov, V.E., Toward Lattice Fractional Vector Calculus, J. Phys. A, 2014, vol. 47, no. 35, p. 355204.

  51. Tarasov, V.E., Lattice Fractional Calculus, Appl. Math. Comput., 2015, vol. 257, pp. 12–33.

  52. Tarasov, V.E., United Lattice Fractional Integro-Differentiation, Fract. Calc. Appl. Anal., 2016, vol. 19, no. 3, pp. 625–664.

  53. Tarasov, V.E., Fractional Nonlocal Continuum Mechanics and Microstructural Models, in Handbook of Nonlocal Continuum Mechanics for Materials and Structures, Voyiadjis, G.Z., Ed., Cham: Springer, 2019, pp. 839–849.

  54. Tarasov, V.E., Discrete Model of Dislocations in Fractional Nonlocal Elasticity, J. King Saud Univ. Sci., 2016., vol. 28, no. 1, pp. 33–36.

  55. Tarasov, V.E. and Aifantis, E.C., Non-Standard Extensions of Gradient Elasticity: Fractional Non-Locality, Memory and Fractality, Commun. Nonlinear Sci. Numer. Simulat., 2015, vol. 22, no. 1–3, pp. 197–227.

  56. Povstenko, Y., Generalized Theory of Diffusive Stresses Associated with the Time-Fractional Diffusion Equation and Nonlocal Constitutive Equations for the Stress Tensor, Comp. Math. Appl., 2019, vol. 78, no. 6, pp. 1819–1825.

  57. Sumelka, W., Thermoelasticity in the Framework of the Fractional Continuum Mechanics, J. Thermal Stresses, 2014, vol. 37, no. 6, pp. 678–706.

  58. Sumelka, W. and Blaszczyk, T., Fractional Continua for Linear Elasticity, Arch. Mech., 2014, vol. 66, no. 3, pp. 147–172.

  59. Sumelka, W., Fractional Calculus for Continuum Mechanics—Anisotropic Nonlocality, Bull. Polish Acad. Sci. Tech. Sci., 2016., vol. 64, no. 2, pp. 361–372.

  60. Romano, G. and Barretta, R., Nonlocal Elasticity in Nanobeams: The Stress-Driven Integral Model, Int. J. Eng. Sci., 2017, vol. 115, pp. 14–27.

  61. Apuzzo, A., Barretta, R., Faghidian, S.A., Luciano, R., and Marotti de Sciarra, F., Free Vibrations of Elastic Beams by Modified Nonlocal Strain Gradient Theory, Int. J. Eng. Sci., 2018, vol. 133, pp. 99–108.

  62. Lazar, M., Maugin, G.A., and Aifantis, E.C., On a Theory of Nonlocal Elasticity of Bi-Helmholtz Type and Some Applications, Int. J. Solids Struct., 2006, vol. 43, no. 6, pp. 1404–1421.

  63. Kilbas, A.A., Srivastava, H.M., and Trujillo, J.J., Theory and Applications of Fractional Differential Equations, Amsterdam: Elsevier, 2006.

  64. Sneddon, I., The Use of Integral Transforms, New York: McGraw-Hill, 1972.

  65. Erdélyi, A., Magnus, W., Oberhettinger, F., and Tricomi, F.G., Higher Transcendental Functions. V. 3, New York: McGraw-Hill, 1955.

  66. Prudnikov, A.P., Brychkov, Yu.A., and Marichev, O.I., Integrals and Series. V. 2: Special Functions, Amsterdam: Gordon & Breach Science Publishers, 1983.

Download references

Author information

Authors and Affiliations

  1. Faculty of Science and Technology, Jan Dlugosz University in Czestochowa, 42-200, Czestochowa, Poland

    Y. Povstenko

Authors
  1. Y. Povstenko
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Y. Povstenko.

Additional information

Russian Text © The Author(s), 2019, published in Fizicheskaya Mezomekhanika, 2020, Vol. 23, No. 2, pp. 35–44.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Povstenko, Y. Fractional Nonlocal Elasticity and Solutions for Straight Screw and Edge Dislocations. Phys Mesomech 23, 547–555 (2020). https://doi.org/10.1134/S1029959920060107

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1029959920060107

Keywords

Search

Navigation