Abstract—
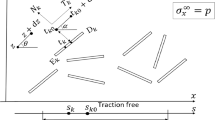
Problems of plane cracks of normal fracture (mathematical cuts) in the middle plane of a transversely isotropic elastic layer, the outer faces of which are under conditions of a sliding support, are considered. Isotropic planes are parallel or perpendicular to layer faces. Using the Fourier integral transform, the problems are reduced to integro-differential equations for crack opening, from which one can obtain the known equations of the corresponding problems for a transversely isotropic space and an isotropic layer by passing to the limit. A regular asymptotic method is applied for elliptical cracks; this method is effective for a relatively thick layer. It is shown the applicability domain of the method narrows with increasing anisotropy that is characterized by the roots of the characteristic equation (for an isotropic material, all roots are equal to unity). For strip-like cracks, closed solutions are obtained based on special approximations of the kernel symbols of integral equations, the relative errors of which decrease with increasing anisotropy. Calculations are made for known transversely isotropic materials.

Similar content being viewed by others
REFERENCES
H. Ding, W. Chen, and L. Zhang, Elasticity of Transversely Isotropic Materials (Springer, Dordrecht, 2006).
E. Pan and W. Chen, Static Green’s Functions in Anisotropic Media (Cambridge Univ. Press, New York, 2015).
W. K. Wilson, “Finite element methods for elastic bodies containing cracks,” in Mechanics of Fracture, Vol. 1: Methods of Analysis and Solutions of Crack Problems, Ed. by G. C. Sih (Kluwer, Leyden, 1973), pp. 484–515.
S. T. Raveendra and T. A. Cruse, “BEM analysis of problems of fracture mechanics,” in Developments in Boundary Element Methods–5. Industrial Applications of Boundary Element Methods, Ed. by P. K. Banerjee and R. B. Wilson (Elsevier, London, 1989), pp. 187–204.
E. I. Shifrin, Spatial Problems in Linear Fracture Mechanics (Fizmatlit, Moscow, 2002) [in Russian].
V. I. Fabrikant, “Non-traditional crack problem for transversely-isotropic body,” Europ. J. Mech. A: Solids 30, 902–912 (2011).
V. I. Fabrikant, “General flat crack located in the plane perpendicular to the planes of isotropy in transversely isotropic body,” Acta Mech. 226 (10), 3289–3306 (2015).
E. A. Artamonova and D. A. Pozharskii, “A strip cut in a transversely isotropic elastic solid,” J. Appl. Math. Mech. 77 (5), 551–558 (2013).
D. A. Pozharskii, “Periodic crack systems in a transversely isotropic solid,” Mech. Solids 54 (5), 709–716 (2019).
V. I. Fabrikant, “General flat crack arbitrarily located in the transversely-isotropic body,” Theor. Appl. Fract. Mech. 82, 69–76 (2016).
V. I. Fabrikant, “Contact and crack problems in generalized material and their relationships,” Int. J. Fract. 212 (1), 41–51 (2018).
V. M. Aleksandrov, “Longitudinal crack in an orthotropic elastic strip with free faces,” Mech. Solids 41 (1), 88–94 (2006).
V. M. Aleksandrov, “Two problems with mixed boundary conditions for an elastic orthotropic strip,” J. Appl. Math. Mech. 70 (1), 128–138 (2006).
R. V. Goldshtein and E. I. Shifrin, “On the possible crooking of a tensile crack in an anisotropic plane,” Mech. Solids 41 (6), 140–149 (2006).
A. V. Kaptsov and E. I. Shifrin, “Identification of multiple cracks in an anisotropic elastic plate by boundary data,” J. Phys. Conf. Ser. 1203 (1), 012029 (2019).
V. I. Fabrikant, Contact and Crack Problems in Linear Elasticity (Bentham, Sharjah, 2010).
V. M. Aleksandrov, B. I. Smetanin, and B. V. Sobol’, Thin Stress Concentrators in Elastic Bodies (Nauka, Moscow, 1993) [in Russian].
A. P. Prudnikov, Yu. A. Brychkov, and O. I. Marichev, Integrals and Series, Vol. 1: Elementary Functions (Gordon and Breach Sci. Publ., New York, 1986).
A. P. Prudnikov, Yu. A. Brychkov, and O. I. Marichev, Integral and Series, Vol. 2: Special Functions (Gordon and Breach Sci. Publ., New York, 1986).
R. V. Gol’dshtein and A. A. Spektor, “Variational method of investigation of three-dimensional mixed problems of a plane cut in an elastic medium in the presence of slip and adhesion of its surfaces,” J. Appl. Math. Mech. 47 (2), 232–239 (1983).
B. I. Smetanin and B. V. Sobol’, “Equilibrium of an elastic layer weakened by plane cracks,” J. Appl. Math. Mech. 48 (6), 757–764 (1984).
Funding
This work was supported by the Russian Foundation for Basic Research, project no. 18-01-00017.
Author information
Authors and Affiliations
Corresponding author
Additional information
Translated by I. Obrezanova
About this article
Cite this article
Artamonova, E.A., Pozharskii, D.A. Plane Cracks in a Transversely Isotropic Layer. Mech. Solids 55, 1406–1414 (2020). https://doi.org/10.3103/S002565442008004X
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.3103/S002565442008004X