An efficient second-order energy stable BDF scheme for the space fractional Cahn–Hilliard equation

Abstract

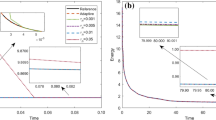
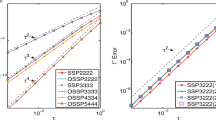
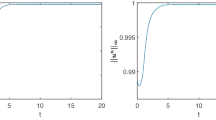
The space fractional Cahn–Hilliard phase-field model is more adequate and accurate in the description of the formation and phase change mechanism than the classical Cahn–Hilliard model. In this article, we propose a temporal second-order energy stable scheme for the space fractional Cahn–Hilliard model. The scheme is based on the second-order backward differentiation formula in time and a finite difference method in space. Energy stability and convergence of the scheme are analyzed, and the optimal convergence orders in time and space are illustrated numerically. Note that the coefficient matrix of the scheme is a $2 \times 2$ block matrix with a Toeplitz-like structure in each block. Combining the advantages of this special structure with a Krylov subspace method, a preconditioning technique is designed to solve the system efficiently. Numerical examples are reported to illustrate the performance of the preconditioned iteration.

Appendix

In this appendix, the convergence orders of (3.2) with lower spatial regularity are provided.

Example A

Consider Example 1 with the exact solution and source terms given by

$$\begin{aligned}&\phi (x,t) = \mu (x,t) = {\left\{ \begin{array}{ll} \exp (t) \left( 1 - x^2 \right) ^{1 + \alpha /2}, &{} (x,t) \in \Omega \times (0,T], \\ 0, &{} (x,t) \in \mathbb {R} {\setminus } \Omega \times (0,T], \end{array}\right. }\\ f(x,t)= & {} \exp (t) \left\{ \left( 1 - x^2 \right) ^{1 + \alpha /2} + \frac{2^{\alpha } \Gamma (\frac{\alpha + 1}{2}) \Gamma (2 + \alpha /2)}{\sqrt{\pi } \Gamma (2)} \left[ 1 - \left( \alpha + 1 \right) x^2 \right] \right\} \end{aligned}$$

and

$$\begin{aligned} \begin{aligned} \psi (x,t)&= \exp (t) \left\{ \left( 1 - x^2 \right) ^{1 + \alpha /2} - \frac{2^{\alpha } \Gamma (\frac{\alpha + 1}{2}) \Gamma (2 + \alpha /2)}{\sqrt{\pi } \Gamma (2)} \varepsilon ^2 \left[ 1 - \left( \alpha + 1 \right) x^2 \right] \right\} \\&\qquad - \exp (3 t) \left( 1 - x^2 \right) ^{3 + 3 \alpha /2} + \exp (t) \left( 1 - x^2 \right) ^{1 + \alpha /2}, \end{aligned} \end{aligned}$$

respectively.

The errors and the convergence orders are listed in Tables 5 and 6. They show that the convergence order of our scheme (3.2) is $\mathcal {O}(\tau ^2 + h^2)$. Comparing with the work [17], the convergence order of space is higher than $1 - \frac{\alpha }{2}$.

Table 5 Numerical errors and the time convergence orders for Example A with $\sigma = 1/16$ and $N = 2048$

Table 6 Numerical errors and the space convergence orders for Example A with $\sigma = 1/16$ and $M = 1024$

Table 7 Numerical errors and the convergence orders for Example B with $\sigma = 1/16$ and $M = N$

Example B

In this example, we consider SFCH (1.1) with a solution that has lower regularity than that of Example A. More precisely, consider Example 1 with the exact solution and source terms given by

$$\begin{aligned}&\phi (x,t) = \mu (x,t) = {\left\{ \begin{array}{ll} \exp (t) \left( 1 - x^2 \right) ^{\alpha /2}, &{} (x,t) \in \Omega \times (0,T], \\ 0, &{} (x,t) \in \mathbb {R} {\setminus } \Omega \times (0,T], \end{array}\right. }\\&f(x,t) = \exp (t) \left[ \left( 1 - x^2 \right) ^{\alpha /2} + \Gamma (\alpha + 1) \right] \end{aligned}$$

and

$$\begin{aligned} \begin{aligned} \psi (x,t)&= \exp (t) \left[ \left( 1 - x^2 \right) ^{\alpha /2} - \varepsilon ^2 \Gamma (\alpha + 1) \right] - \exp (3 t) \left( 1 - x^2 \right) ^{3 \alpha /2} \\&\quad +\, \exp (t) \left( 1 - x^2 \right) ^{\alpha /2}, \end{aligned} \end{aligned}$$

respectively.

The numerical errors and the observed convergence orders are listed in Table 7. It can be seen that for this lower regularity solution, the observed convergence order is almost $\alpha /2$.