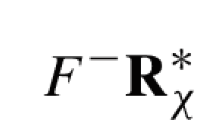Abstract
We establish an explicit Plancherel decomposition for \({{\,\mathrm{\mathrm {GL}}\,}}_n(F){\backslash } {{\,\mathrm{\mathrm {GL}}\,}}_n(E)\) where E/F is a quadratic extension of local fields of characteristic zero by making use of a local functional equation for Asai \(\gamma \)-factors. We also give two applications of this Plancherel formula: first to the global Ichino–Ikeda conjecture for unitary groups by completing a comparison between local relative characters that was left open by Zhang (J Am Math Soc 27:541–612, 2014) and secondly to the Hiraga–Ichino–Ikeda conjecture on formal degrees (J Am Math Soc 21(1):283–304, 2008) in the case of unitary groups.
Similar content being viewed by others
Notes
Strictly speaking, the general conjectures of [62] do not apply to \(Y_n\) since in loc. cit. the group acting G (which here is \(R_{E/F}{{\,\mathrm{\mathrm {GL}}\,}}_{n,E}\)) was assumed to be split over F. However, in light of another conjecture of Jacquet (see [59]) there is a natural way to extrapolate the conjecture of Sakellaridis–Venkatesh to the case at hand by considering the “L-group” of \(Y_n\) to be \({}^L Y_n={}^L U(n)\) and the morphism \({}^L Y_n\rightarrow {}^L G\) to be given by base-change (stable or unstable as before).
Let us emphasize that, although not transparent from the notation, the measure \(d\mu _G(\pi )\) depends on the choice of the additive character \(\psi '\): indeed, this measure is inversely proportional to the Haar measure on G(F) which, following the convention of Sect. 2.5, depends itself on \(\psi '\). Similarly, the density \(\mu _G(\pi )\) depends on \(\psi '\), a dependence which, in the case of \({{\,\mathrm{\mathrm {GL}}\,}}_n\), will be made more transparent by Proposition 2.132 below.
More precisely, the uniform boundedness principle implies that, for any sequence \(s_n\in {{\,\mathrm{\mathcal {H}}\,}}\) converging to 0, the sequence of tempered distributions \((s_nD_{s_n})_n\) is equicontinuous hence that, by continuity of \(s\mapsto \varphi _s\in {{\,\mathrm{\mathcal {S}}\,}}({{\,\mathrm{\mathcal {A}}\,}}_0)\), \(s_nD_{s_n}(\varphi _{s_n})-s_nD_{s_n}(\varphi _0)\) converges to 0 as \(n\rightarrow \infty \) and we can then apply Proposition 3.31 to the single Schwartz function \(\varphi _0\).
See footnote 3.
Note that in the linear case we need to consider distributions that are \(H_1(F)\times (H_2(F),\eta )\)-equivariant, and there is no such distribution supported on the trivial coset (because \(\eta \) is nontrivial on \(H_1(F)\cap H_2(F)\)).
where here “regular” means “with trivial stabilizer”.
References
Aizenbud, A., Gourevitch, D.: Schwartz functions on Nash manifolds. Int. Math. Res. Not. IMRN, (5), Art. ID rnm 155, 37 (2008)
Aizenbud, A., Lapid, E.: Distinguished representations in the Archimedean case, appendix D to “On representations distinguished by unitary groups” by B. Feigon, E. Lapid, O. Offen. Publications Mathématiques de L’IHÉS 115/1, 185–323 (2012)
Arthur, J.: Harmonic analysis of the Schwartz space on a reductive Lie group II. http://www.math.toronto.edu/arthur/pdf/20160115093856221.pdf
Arthur, J.: A Paley–Wiener theorem for real reductive groups. Acta Math. 150(1–2), 1–89 (1983)
Arthur, J.: The endoscopic classification of representations. Orthogonal and symplectic groups, American Mathematical Society Colloquium Publications, vol. 61. American Mathematical Society, Providence, RI, xviii+590 pp. (2013)
Baruch, E.M.: A proof of Kirillov’s conjecture. Ann. Math. (2) 158, 207–252 (2003)
Bernât, P., Dixmier, J.: Sur le dual d’un groupe de Lie. C. R. Acad. Sci. Paris 250, 1778–1779 (1960)
Bernstein, J.N.: All reductive \(p\)-adic groups are tame. Funct. Anal. Appl. 8, 91–93 (1974)
Bernstein, J.N.: \(P\)-invariant distributions on \(GL(N)\) and the classification of unitary representations of \(GL(N)\) (non-Archimedean case). In: Lie Group Representations, II. Lecture Notes in Mathematics, vol. 1041. Springer, Berlin, pp. 50–102 (1984)
Bernstein, J.N.: On the support of Plancherel measure. J. Geom. Phys. 5(4), 663–710 (1988)
Bernstein, J.N., Krötz, B.: Smooth Fréchet globalizations of Harish–Chandra modules. Israel J. Math. 199(1), 45–111 (2014)
Beuzart-Plessis, R.: A local trace formula for the Gan–Gross–Prasad conjecture for unitary groups: the Archimedean case, Astérisque No. 418, p. 308 (2020). ISBN:978-2-85629-919-7
Beuzart-Plessis, R.: Comparison of local spherical characters and the Ichino–Ikeda conjecture for unitary groups. arXiv:1602.06538, to appear in J. Inst. Math. Jussieu
Beuzart-Plessis, R.: Archimedean theory and \(\epsilon \)-factors for the Asai Rankin–Selberg integrals, to appear in Proceeding of Simons Symposium “Relative trace formulas”
Beuzart-Plessis, R.: A new proof of Jacquet–Rallis’s fundamental lemma. arXiv:1901.02653
Borel, A., Wallach, N.: Continuous Cohomology, Discrete Subgroups, and Representations of Reductive Groups, volume 67 of Mathematical Surveys and Monographs, 2nd edn. American Mathematical Society, Providence, RI (2000)
Bourbaki, N.: Éléments de mathématique. Fasc. XXXIII. Variétés différentielles et analytiques, Fascicule de résultats (Paragraphes 1 à 7). Actualités Scientifiques et Industrielles, No. 1333. Hermann, Paris (1967)
Casselman, W.: Canonical extensions of Harish–Chandra modules to representations of \(G\). Can. J. Math. 41(3), 385–438 (1989)
Casselman, W., Hecht, H., Miličić, D.: Bruhat filtrations and Whittaker vectors for real groups. In: The Mathematical Legacy of Harish–Chandra (Baltimore, MD, 1998). American Mathematical Society, Providence, pp. 151–190 (2000)
Chaudouard, P.-H.: On relative trace formulae: the case of Jacquet–Rallis. Acta Math. Vietnam. 44(2), 391–430 (2019)
Chaudouard, P.-H., Zydor, M.: Le transfert singulier pour la formule des traces de Jacquet–Rallis. arXiv:1611.09656, to appear in Compositio Math
Cowling, M., Haagerup, U., Howe R.: Almost \(L^2\) matrix coefficients. J. für die reine und angewandte Math. 387, 97–110 (1988)
Delorme, P.: Multipliers for the convolution algebra of left and right \(K\)-finite compactly supported smooth functions on a semisimple Lie group. Invent. Math. 75(1), 9–23 (1984)
Delorme, P.: Formule de Plancherel pour les fonctions de Whittaker sur un groupe réductif \(p\)-adique. Ann. Inst. Fourier (Grenoble) 63(1), 155–217 (2013)
Delorme, P.: Neighborhoods at infinity and the Plancherel formula for a reductive \(p\)-adic symmetric space. Math. Ann. 370(3–4), 1177–1229 (2018)
Dixmier, J.: \(C^*\)-algebras, Translated from the French by Francis Jellett. North-Holland Mathematical Library, vol. 15. North-Holland Publishing Co., Amsterdam-New York-Oxford, xiii+492 pp (1977). ISBN: 0-7204-0762-1
Dixmier, J., Malliavin, P.: Factorisations de fonctions et de vecteurs indéfiniment différentiables. Bull. Sci. Math. (2) 102(4), 307–330 (1978)
Filip, I.: A local relative trace formula for spherical varieties. PhD thesis Columbia university (2016)
Flicker, Y.Z.: Twisted tensors and Euler products. Bull. Soc. Math. France 116(3), 295–313 (1988)
Flicker, Y.Z.: On zeroes of the twisted tensor \(L\)-function. Math. Ann. 297, 199–219 (1993)
Gan, W.T., Gross, B. H., Prasad, D.: Symplectic local root numbers, central critical \(L\)-values and restriction problems in the representation theory of classical groups, in “Sur les conjectures de Gross et Prasad. I” Astérisque No. 346, 1–109 (2012)
Gelbart, S., Jacquet, H., Rogawski, J.: Generic representations for the unitary group in three variables. Israel J. Math. 126, 173–237 (2001)
Gelfand, I.M., Shilov, G.E.: Generalized Functions, vol. I. Academic Press, New York (1964)
Gross, B.H., Reeder, M.: Arithmetic invariants of discrete Langlands parameters. Duke Math. J. 154(3), 431–508 (2010)
Grothendieck, A.: Produits tensoriels topologiques et espaces nucléaires. Mem. Am. Math. Soc. 1955(16) (1955)
Grothendieck, A.: Sur certains espaces de fonctions holomorphes. I. J. Reine Angew. Math. 192, 35–64 (1953)
Harinck, P.: Base de la série la plus continue de fonctions généralisées sphériques sur \(G(\mathbb{C})/G(\mathbb{R})\). J. Funct. Anal. 153, 1–51 (1998)
Harish-Chandra: Discrete series for semisimple Lie groups. II. Explicit determination of the characters. Acta Math. 116, 1–111 (1966)
Harish-Chandra: Harmonic analysis on real reductive groups. III. The Maass-Selberg relations and the Plancherel formula. Ann. Math. 104, 117–201 (1976)
Neal Harris, R.: A refined Gross–Prasad conjecture for unitary groups. Int. Math. Res. Not. IMRN 2, 303–389 (2014)
Harris, M., Taylor, R.: The geometry and cohomology of some simple Shimura varieties. With an appendix by Vladimir G. Berkovich. Annals of Mathematics Studies, vol. 151. Princeton University Press, Princeton, NJ, viii+276 pp (2001). ISBN: 0-691-09090-4
Henniart, G.: Une preuve simple des conjectures de Langlands pour \(GL(n)\) sur un corps \(p\)-adique. Invent. Math. 139(2), 439–455 (2000)
Hiraga, K., Ichino, A., Ikeda, T.: Formal degrees and adjoint \(\gamma \)-factors. J. Am. Math. Soc. 21(1), 283–304 (2008)
Ichino, A., Lapid, E., Mao, Z.: On the formal degrees of square-integrable representations of odd special orthogonal and metaplectic groups. Duke Math. J. 166(7), 1301–1348 (2017)
Jacquet, H., Rallis, S.: On the Gross–Prasad conjecture for unitary groups. In: On Certain \(L\)-Functions. Clay Mathematics Proceedings, vol. 13. American Mathematical Society, Providence, RI, pp. 205–264 (2011)
Kable, A.C.: Asai \(L\)-function and Jacquet’s conjecture. Am. J. Math. 126, 789–820 (2004)
Kaletha, T., Minguez, A., Shin, S.W., White, P.-J.: Endoscopic classification of representations: inner forms of unitary groups, prepublication. arXiv:1409.3731 (2014)
Kemarsky, A.: Distinguished representations of \({{\rm GL}}_n(\mathbb{C})\). Israel J. Math. 207(1), 435–448 (2015)
Kottwitz, R.E.: Harmonic analysis on reductive \(p\)-adic groups and Lie algebras. In: Harmonic Analysis, the Trace Formula, and Shimura Varieties. Clay Mathematics Proceedings, vol. 4. American Mathematical Society, Providence, RI, pp. 393–522 (2005)
Langlands, R.P.: On the classification of irreducible representations of real algebraic groups. In: Representation Theory and Harmonic Analysis on Semisimple Lie Groups. Mathematical Surveys and Monographs, vol. 31. American Mathematical Society, Providence, RI, pp. 101–170 (1989)
Lapid, E., Mao, Z.: On the asymptotics of Whittaker functions. Represent. Theory 13, 63–81 (2009). https://doi.org/10.1090/S1088-4165-09-00343-4
Lapid, E., Mao, Z.: A conjecture on Whittaker–Fourier coefficients of cusp forms. J. Number Theory 146, 448–505 (2015)
Lapid, E., Mao, Z.: On a new functional equation for local integrals. Automorphic forms and related geometry: assessing the legacy of I. I. Piatetski-Shapiro. Contemporary Mathematics, vol. 614. American Mathematical Society, Providence, RI, pp. 261–294 (2014)
Li, W.-W.: Zeta Integrals, Schwartz Spaces and Local Functional Equations, to appear in Springer Lecture Notes in Mathematics. https://doi.org/10.1007/978-3-030-01288-5
Matringe, N.: Distinction and Asai \(L\)-functions for generic representations of general linear groups over \(p\)-adic fields. IMRN 1, 74–95 (2011)
Mok, C.P.: Endoscopic classification of representations of quasi-split unitary groups. Mem. Am. Math. Soc. 235, 1108 (2015)
Offen, O.: On local root numbers and distinction. J. Reine Angew. Math. 652, 165–205 (2011)
Ok, Y.: Distinction and gamma factors at \(\frac{1}{2}\): supercuspidal case. PhD Thesis, Columbia University (1997)
Prasad, D.: On a conjecture of Jacquet about distinguished representations of \(GL(n)\). Duke Math. J. 109, 67–78 (2001)
Rodier, F.: Modèles de Whittaker des représentations admissibles des groupes réductifs \(p\)-adiques quasi-déployés, manuscript. http://iml.univ-mrs.fr/~rodier/Whittaker.pdf (1975)
Sahi, S.: On Kirillov’s conjecture for Archimedean fields. Compos. Math. 72(1), 67–86 (1989)
Sakellaridis, Y., Venkatesh, A.: Periods and harmonic analysis on spherical varieties. Astérisque No. 396, viii+360 pp. (2017)
Scholze, P.: The local Langlands correspondence for \(GL_n\) over \(p\)-adic fields. Invent. Math. 192(3), 663–715 (2013)
Shahidi, F.: Fourier transforms of intertwining operators and Plancherel measures for \(GL(n)\). Am. J. Math. 106(1), 67–111 (1984)
Shahidi, F.: Local coefficients as Artin factors for real groups. Duke Math. J. 52(4), 973–1007 (1985)
Silberger, A.J., Zink, E.-W.: The formal degree of discrete series representations of central simple algebras over \(p\)-adic fields. Max-Planck-Institut für Mathematik (1996)
Smith, J.M.: Relative discrete series representations for two quotients of \(p\)-adic \(GL_n\). Can. J. Math. 70(6), 1339–1372 (2018)
Sun, B.: Bounding matrix coefficients for smooth vectors of tempered representations. Proc. AMS 137(1), 353–357 (2009)
Tadić, Marko: Geometry of dual spaces of reductive groups (non-Archimedean case). J. Anal. Math. 51, 139–181 (1988)
Tate, J.: Number theoretic background. In: Automorphic Forms, Representations, and \(L\)-Functions. Proceedings of Symposia Pure Mathematics, vol. 33. AMS, pp. 3–26 (1979)
Trèves, F.: Topological Vector Spaces, Distributions and Kernels. Academic Press, New York-London, xvi+624 pp. (1967)
Varadarajan, V.S.: Harmonic Analysis on Real Reductive Groups. Lecture Notes in Mathematics, vol. 576. Springer, Berlin-New York, v+521 pp. (1977)
Waldspurger, J.-L.: La formule de Plancherel pour les groupes \(p\)-adiques (d’après Harish-Chandra). J. Inst. Math. Jussieu 2(2), 235–333 (2003)
Waldspurger, J.-L.: Calcul d’une valeur d’un facteur \(\epsilon \) par une formule intégrale. In: Sur les conjectures de Gross et Prasad II. Astérisque No. 347, 1–102 (2012). ISBN: 978-2-85629-350-8
Wallach, N.R.: Real Reductive Groups I. Pure and Applied Mathematics, vol. 132. Academic Press, Inc., Boston, MA, xx+412 pp. (1988)
Wallach, N.R.: Real Reductive Groups II. Pure and Applied Mathematics, vol. 132-II. Academic Press, Inc., Boston, MA, xiv+454 pp. (1992). ISBN: 0-12-732961-7
Whittaker, E.T., Watson, G.N.: A Course of Modern Analysis. Cambridge University Press, London (1965)
Xue, H.: On the global Gan–Gross–Prasad conjecture for unitary groups: approximating smooth transfer of Jacquet-Rallis. J. Reine Angew. Math. (2015) to appear
Yun, Z.: The fundamental lemma of Jacquet and Rallis. With an appendix by Julia Gordon. Duke Math. J. 156(2), 167–227 (2011)
Zhang, W.: Fourier transform and the global Gan–Gross–Prasad conjecture for unitary groups. Ann. Math. (2) 180(3), 971–1049 (2014)
Zhang, W.: On arithmetic fundamental lemmas. Invent. Math. 188(1), 197–252 (2012)
Zhang, W.: Automorphic period and the central value of Rankin–Selberg \(L\)-function. J. Am. Math. Soc. 27, 541–612 (2014)
Zydor, M.: Les formules des traces relatives de Jacquet–Rallis grossières. J. Reine Angew. Math. 762, 195–259 (2020)
Acknowledgements
The results of this paper (in a slightly weaker form) have been announced by the author in his “Cours Peccot” in April-May 2017. I would like to thank the Collège de France for giving me the opportunity to give this course which has probably accelerated the present work and I apologize to anyone who was promised an earlier preprint.
I am grateful to Erez Lapid and Jean-Loup Waldspurger for helpful comments on a first version of this paper. I also thank the anonymous referee for his very accurate comments and suggestions for improvement.
The project leading to this publication has received funding from Excellence Initiative of Aix-Marseille University-A*MIDEX, a French “Investissements d’Avenir” programme.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
A Proof of Proposition 2.131
A Proof of Proposition 2.131
First, we recall the following elementary lemma (see [22, Proposition]).
Lemma A.01
(Sobolev lemma for compact groups) Let H be a Hilbert space, K be a compact group and C(K, H) be the space of continuous functions from K to H. Consider C(K, H) as a representation of K through the right regular action and for \(\rho \in \widehat{K}\) denote by \(C(K,H)[\rho ]\) its \(\rho \)-isotypic component. Then, for every \(\rho \in \widehat{K}\) and \(\varphi \in C(K,H)[\rho ]\) we have
Now we proceed to the proof of Proposition 2.131.
Let \(f\in {{\,\mathrm{\mathcal {S}}\,}}(G(F))\). The function \(\pi \in {{\,\mathrm{Temp}\,}}_{{{\,\mathrm{ind}\,}}}(G)\mapsto f_\pi \in {{\,\mathrm{\mathcal {C}}\,}}^w(G(F))\) is smooth by [12, Lemma 2.3.1(ii)] together with [3, §3] in the Archimedean case and [73, Proposition VII.1.3] in the p-adic case. Moreover, in the p-adic case the function \(\pi \mapsto f_\pi \) is compactly supported by [73, Théorème VIII.1.2]. It remains to check that f satisfies condition (2.9.1) in the Archimedean case and condition (2.9.2) in the p-adic case. We will concentrate on the Archimedean case, the p-adic case being similar and actually easier to handle. By definition of the topology on \({{\,\mathrm{\mathcal {C}}\,}}^w(G(F))\), it suffices to establish the following: for every Levi subgroup \(M\subset G\), \(D\in {{\,\mathrm{Sym}\,}}^\bullet ({{\,\mathrm{\mathcal {A}}\,}}_{M,\mathbb {C}}^*)\), \(u,v\in {{\,\mathrm{\mathcal {U}}\,}}({{\,\mathrm{\mathfrak {g}}\,}})\) and \(k\geqslant 0\) we have
for \(\tau \in \varPi _2(M)\) and \(g\in G(F)\) where \(\deg (D)\) stands for the degree of D and we have set \(\pi _\lambda =i_M^G(\tau _\lambda )\) and \(\pi =\pi _0\) for \(\lambda \in i{{\,\mathrm{\mathcal {A}}\,}}_M^*\).
As \(R(u)L(v)f_\pi =(R(u)L(v)f)_\pi \), up to replacing f by R(u)L(v)f we only need to establish (A.0.1) when \(u=v=1\). Assume proved the slightly weaker inequality (for any \(D\in {{\,\mathrm{Sym}\,}}^\bullet ({{\,\mathrm{\mathcal {A}}\,}}_{M,\mathbb {C}}^*)\))
Then, we will show that (A.0.1) holds for any \(k\geqslant 0\) (and \(u=v=1\)). We do this by induction on \(\deg (D)\). Let \(z\in {{\,\mathrm{\mathcal {Z}}\,}}({{\,\mathrm{\mathfrak {g}}\,}})\) be such that (2.6.2) is satisfied. Then we may as well assume that \(N(\pi )=\chi _{\pi }(z)\). Since \((z^kf)_\pi =\chi _\pi (z)^kf_\pi \) for every \(\pi \in {{\,\mathrm{Temp}\,}}_{{{\,\mathrm{ind}\,}}}(G)\) the result in degree 0 just follows from replacing f by \(z^k f\) in (A.0.2). In the general case the difference between
and
can be written as a finite sum
where \(D_i,D'_i\in {{\,\mathrm{Sym}\,}}^\bullet ({{\,\mathrm{\mathcal {A}}\,}}_{M,\mathbb {C}})\) for all \(1\leqslant i\leqslant n\) are of degree strictly less than D. Since the terms \(D_i(\lambda \mapsto \chi _{\pi _\lambda }(z)^k)_{\lambda =0}\) are all essentially bounded by a power of \(N(\pi )\), the above sum can be controlled by the induction hypothesis whereas (A.0.3) is controlled by (A.0.2) applied to \(z^k f\). This shows (A.0.1) assuming (A.0.2).
Fix \(D\in {{\,\mathrm{Sym}\,}}^\bullet ({{\,\mathrm{\mathcal {A}}\,}}_{M,\mathbb {C}}^*)\), a parabolic subgroup P with Levi component M, a maximal compact subgroup K of G(F) in good position relative to M and set \(K_M=K\cap M(F)\). Then, by restriction to K we can identify \(\pi _\lambda =i_P^G(\tau _\lambda )\) with \(\pi _K=i_{K_M}^K(\tau _{\mid K_M})\) as a K-representation for every \(\tau \in \varPi _2(M)\) and \(\lambda \in i{{\,\mathrm{\mathcal {A}}\,}}_M^*\). Choosing an invariant scalar product (., .) on \(\tau \), we endow \(\pi _K\) with the K-invariant scalar product
Finally, choose for any \(\rho \in \widehat{K}\) an orthonormal basis \({{\,\mathrm{\mathcal {B}}\,}}_{\tau }(\rho )\) of the \(\rho \)-isotypic component \(\pi _K[\rho ]\) of \(\pi _K\). Then, we have
for all \(\tau \in \varPi _2(M)\), \(\lambda \in i{{\,\mathrm{\mathcal {A}}\,}}_M^*\) and \(g\in G(F)\). Assume now that we can show the existence of \(r>0\) such that
for all \(\tau \in \varPi _2(M)\), \(g\in G(F)\), \(\rho \in \widehat{K}\) and \(e\in {{\,\mathrm{\mathcal {B}}\,}}_{\tau }(\rho )\). Let \(z_K\in {{\,\mathrm{\mathcal {Z}}\,}}(\mathfrak {k})\) be such that (2.6.2) is satisfied for \(G(F)=K\). Then we may as well assume that \(N(\rho )=\rho (z_K)\) for all \(\rho \in \widehat{K}\) and hence up to replacing f by \(L(z_K)^{k+r}f\) in (A.0.5) we obtain the same inequality with \(N(\rho )^r\) replaced by \(N(\rho )^{-k}\). Therefore, by (A.0.4), we would get for any \(k>0\) an inequality
As for k large enough the sum \(\sum _{\rho \in \widehat{K}} \frac{\dim (\pi _K[\rho ])}{N(\rho )^k}\) converges and is bounded independently of \(\pi \) (see e.g. [12, (2.2.2)]). For such a k the above inequality implies (A.0.2).
Thus, it only remains to establish (A.0.5). Actually, it suffices to show the existence of \(r>0\) such that
for all \(\tau \in \varPi _2(M)\), \(g\in G(F)\), \(\rho \in \widehat{K}\) and \(e\in {{\,\mathrm{\mathcal {B}}\,}}_{\tau }(\rho )\). Indeed, if this is the case we would get
for all \(\tau \in \varPi _2(M)\), \(g\in G(F)\), \(\rho \in \widehat{K}\) and \(e\in {{\,\mathrm{\mathcal {B}}\,}}_{\tau }(\rho )\), where the differentiation under the integral sign is justified by the absolute convergence of the resulting expression and in the last line we have used the well-known ‘doubling formula’ \(\int _K \varXi ^G(gk\gamma )dk=\varXi ^G(g)\varXi ^G(\gamma )\) (see [72, Proposition 16.(iii) p. 329]).
Fix a decomposition \(\gamma =m_P(\gamma )u_P(\gamma )k_P(\gamma )\) for every \(\gamma \in G(F)\) where \(m_P(\gamma )\in M(F)\), \(u_P(\gamma )\in U_P(F)\) and \(k_P(\gamma )\in K\) and set \(H_P(\gamma ):=H_M(m_P(\gamma ))\). Then to prove (A.0.6) we first note that
so that (once again differentiation under the integral is easily justified)
for all \(\tau \in \varPi _2(M)\), \(e\in \pi _K\) and \(g\in G(F)\) where when we write \(D(H_P(kg))\) we consider D as a polynomial function on \({{\,\mathrm{\mathcal {A}}\,}}_M\). Clearly \(|D(H_P(kg))|\ll \sigma (g)^{\deg (D)}\) for all \(g\in G(F)\) and \(k\in K\) and therefore
for all \(\tau \in \varPi _2(M)\), \(e\in \pi _K\) and \(g\in G(F)\). By [22, Theorem 2], we have
for all \(\tau \in \varPi _2(M)\), all \(v,v'\in \tau \) and all \(m\in M(F)\). Note that \(\dim (\tau (K_M)e(k))\leqslant \dim (\pi (K)e)\) for all \(\tau \in \varPi _2(M)\) and \(e\in \pi _K\) and that by a new application of [22, Theorem 2] \(\dim (\pi (K)e)\leqslant \dim (\rho )^2\) if \(e\in \pi _K[\rho ]\). Combining this with (A.0.7), we obtain
for all \(\tau \in \varPi _2(M)\), \(\rho \in \widehat{K}\), \(e\in \pi _K[\rho ]\) and \(g\in G(F)\) where in the last inequality we have used [72, Proposition 16(iv) p. 329] and Lemma A.01. Since \(\Vert e\Vert =1\) and there exists \(n\geqslant 1\) such that \(\dim (\rho )\leqslant N(\rho )^n\) ([75, p. 291]), this gives (A.0.6) and ends the proof of the Proposition 2.131. \(\square \)
Rights and permissions
About this article
Cite this article
Beuzart-Plessis, R. Plancherel formula for \({{\,\mathrm{\mathrm {GL}}\,}}_n(F){\backslash } {{\,\mathrm{\mathrm {GL}}\,}}_n(E)\) and applications to the Ichino–Ikeda and formal degree conjectures for unitary groups. Invent. math. 225, 159–297 (2021). https://doi.org/10.1007/s00222-021-01032-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00222-021-01032-6