The Role of Anisotropic Proton Pressure in the Generation of Geomagnetic Pulsations

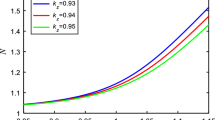
Abstract—The effect of hot anisotropic plasma on the development of cyclotron instability in the near-Earth space plasma at finite plasma pressure ${{\beta }_{ \bot }}$ is studied. It is shown that the finiteness of ${{\beta }_{ \bot }}$​ does not only modify the imaginary part of the dispersion equation which is associated with the amplification of waves but also changes the real part of this equation which describes wave propagation along the magnetic field lines. This leads to a change in the cyclotron instability increment and causes variations in the parameters of propagation of electromagnetic ion-cyclotron (EMIC) waves along a field line. The obtained results suggest that for the generation of EMIC waves in the near-Earth plasma, small values of perpendicular proton plasma pressure (${{\beta }_{ \bot }}$), large anisotropy values ($A$), and large values of ${{{{v}_{{\parallel h}}}} \mathord{\left/ {\vphantom {{{{v}_{{\parallel h}}}} {{{c}_{A}}}}} \right. \kern-0em} {{{c}_{A}}}}$ ratio are preferable.

Appendices

APPENDIX A

In the case of the Maxwellian distribution with different temperatures along and across the magnetic field ${{T}_{\parallel }}$ and ${{T}_{ \bot }}$, the squared refractive index ${{N}^{2}} = {{k_{z}^{2}{{c}^{2}}} \mathord{\left/ {\vphantom {{k_{z}^{2}{{c}^{2}}} {{{\omega }^{2}}}}} \right. \kern-0em} {{{\omega }^{2}}}}$ of transverse waves with circular polarization which is presented in (Shafranov, 1963, p. 77, formula (9.36)) has the following form (${{v}_{\parallel }} = \sqrt {{{2{{T}_{\parallel }}} \mathord{\left/ {\vphantom {{2{{T}_{\parallel }}} m}} \right. \kern-0em} m}}$):

$$\begin{gathered} {{N}^{2}} = \varepsilon \pm g = 1 - \sum {\frac{{\omega _{0}^{2}}}{\omega }} \left\{ {\frac{1}{{\omega \mp {{\omega }_{B}}}}Z\left( {\frac{{\omega \mp {{\omega }_{B}}}}{{{{k}_{z}}{{v}_{\parallel }}}}} \right)} \right. \\ \times \,\,\left[ {\frac{{{{T}_{ \bot }}}}{{{{T}_{\parallel }}}} \pm \frac{{{{\omega }_{B}}}}{\omega }} \right.\left. {\left. {\left( {1 - \frac{{{{T}_{ \bot }}}}{{{{T}_{\parallel }}}}} \right)} \right] + \frac{1}{\omega }\left( {1 - \frac{{{{T}_{ \bot }}}}{{{{T}_{\parallel }}}}} \right)} \right\}. \\ \end{gathered} $$

(A.1)

(There are two equations in formula A.1, one with the upper signs and the other with the lower signs; and each equation defines its own wave type. The upper sign corresponds to the electromagnetic ion cyclotron (EMIC) waves which are considered in this paper).

We consider plasma consisting of cold electrons and ions with small admixture of hot anisotropic protons:

$$\begin{gathered} {{n}_{{0e}}} = {{n}_{{0i}}},\,\,\,\omega _{{0e}}^{2} = \frac{{4\pi n{{{\kern 1pt} }_{0}}{{e}^{2}}}}{{{{m}_{e}}}},\, \\ \omega _{{0i}}^{2} = \frac{{4\pi n{{{\kern 1pt} }_{0}}{{e}^{2}}}}{{{{m}_{i}}}},\,\,\,\,\omega _{{0h}}^{2} = \frac{{4\pi n{{{\kern 1pt} }_{h}}{{e}^{2}}}}{{{{m}_{i}}}}. \\ \end{gathered} $$

According to (Shafranov, 1963. p. 132 (II.5)),

$$\left. \begin{gathered} Z(z) = X(z) - iY(z); \hfill \\ X(z) = 2z{{e}^{{ - {{z}^{2}}}}}\int\limits_0^x {{{e}^{{{{t}^{2}}}}}} dt; \hfill \\ Y(z) = \sqrt \pi z{{e}^{{ - {{z}^{2}}}}}. \hfill \\ \end{gathered} \right\}$$

(A.2)

Function has the following asymptotic decomposition (Shfranov, 1963, p. 74):

$$X(z) = 1 + \frac{1}{{2{{z}^{2}}}} + \frac{3}{{4{{z}^{4}}}} + ...,$$

(A.3)

hence

$$Z\left( {\frac{{\omega - {{\omega }_{B}}}}{{{{k}_{z}}{{v}_{\parallel }}}}} \right) = 1 + \frac{{k_{z}^{2}v_{\parallel }^{2}}}{{{{{(\omega - {{\omega }_{B}})}}^{2}}}} + ...$$

and

$$\begin{gathered} {{N}^{2}} = 1 - \sum {\frac{{\omega _{0}^{2}}}{\omega }} \left\{ {\frac{1}{{\omega - {{\omega }_{B}}}}Z\left( {\frac{{\omega - {{\omega }_{B}}}}{{{{k}_{z}}{{v}_{\parallel }}}}} \right)} \right. \\ \left. {\left. { \times \,\,\left[ {\frac{{{{T}_{ \bot }}}}{{{{T}_{\parallel }}}} + \frac{{{{\omega }_{B}}}}{\omega }} \right.\left( {1 - \frac{{{{T}_{ \bot }}}}{{{{T}_{\parallel }}}}} \right)} \right] + \frac{1}{\omega }\left( {1 - \frac{{{{T}_{ \bot }}}}{{{{T}_{\parallel }}}}} \right)} \right\}. \\ \end{gathered} $$

(A.4)

Denoting $\Omega = {{\omega }_{{Bi}}}$, ${{k}_{\parallel }} = {{k}_{z}}$, after summation over all particles (taking into account that $\omega \ll {{\omega }_{{Be}}}$), we have

$$\begin{gathered} \frac{{{{c}^{2}}k_{\parallel }^{2}}}{{{{\omega }^{2}}}} = 1 + \frac{{\omega _{{0i}}^{2}}}{{\Omega (\Omega - \omega )}} - \frac{{\omega _{{0h}}^{2}}}{\omega } \\ \times \,\,\left[ {Z\left( {\frac{{\omega - \Omega }}{{{{k}_{\parallel }}{{v}_{{\parallel h}}}}}} \right)\left( {\frac{A}{\omega } - \frac{1}{{\Omega - \omega }}} \right) - \frac{A}{\omega }} \right], \\ \frac{{{{c}^{2}}k_{\parallel }^{2}}}{{{{\omega }^{2}}}} + \frac{{\omega _{{0h}}^{2}}}{\omega }\frac{{k_{\parallel }^{2}v_{{\parallel h}}^{2}}}{{2{{{(\omega - \Omega )}}^{2}}}}\left( {\frac{A}{\omega } - \frac{1}{{\Omega - \omega }}} \right) \\ = 1 + \frac{{\omega _{{0i}}^{2}}}{{\Omega (\Omega - \omega )}} + \frac{{\omega _{{0h}}^{2}}}{{\omega (\Omega - \omega )}} \\ + \,\,i\sqrt \pi \frac{{\omega _{{0h}}^{2}}}{\omega }\frac{{\omega - \Omega }}{{{{k}_{\parallel }}{{v}_{{\parallel h}}}}}\exp \left[ { - {{{\left( {\frac{{\omega - \Omega }}{{{{k}_{\parallel }}{{v}_{{\parallel h}}}}}} \right)}}^{2}}} \right]\left( {\frac{A}{\omega } - \frac{1}{{\Omega - \omega }}} \right). \\ \end{gathered} $$

(A.5)

We transform this expression ($v_{{\parallel h}}^{2} = {{2{{T}_{\parallel }}} \mathord{\left/ {\vphantom {{2{{T}_{\parallel }}} m}} \right. \kern-0em} m}$):

$$\begin{gathered} \frac{{\omega _{{0h}}^{2}}}{\omega }\left[ {\frac{{k_{\parallel }^{2}v_{{\parallel h}}^{2}}}{{2{{{(\omega - \Omega )}}^{2}}}}} \right]\left( {\frac{A}{\omega } - \frac{1}{{\Omega - \omega }}} \right) = \frac{{4\pi {{n}_{h}}{{e}^{2}}}}{{m\omega }}\frac{{2{{T}_{ \bot }}B_{0}^{2}}}{{2{{T}_{ \bot }}B_{0}^{2}}} \\ \times \,\,\frac{{k_{\parallel }^{2}v_{{\parallel h}}^{2}}}{{2{{\Omega }^{2}}{{{\left( {\frac{\omega }{\Omega } - 1} \right)}}^{2}}}}\left( {\frac{A}{\omega } - \frac{1}{{\Omega - \omega }}} \right) \\ = \frac{{{{\beta }_{ \bot }}}}{{2m\omega }}\frac{{{{e}^{2}}B_{0}^{2}}}{{{{T}_{ \bot }}}}\frac{{{{m}^{2}}{{c}^{2}}k_{\parallel }^{2}v_{{\parallel h}}^{2}}}{{2{{e}^{2}}{{B}^{2}}{{{\left( {\frac{\omega }{\Omega } - 1} \right)}}^{2}}}}\left( {\frac{A}{\omega } - \frac{1}{{\Omega - \omega }}} \right) \\ = \frac{{{{c}^{2}}k_{\parallel }^{2}}}{{{{\omega }^{2}}}}\frac{{{{\beta }_{ \bot }}}}{2}\frac{{{{T}_{\parallel }}}}{{{{T}_{ \bot }}}}\frac{{\left[ {A(\Omega - \omega ) - \omega } \right]}}{{\Omega {{{\left( {1 - \frac{\omega }{\Omega }} \right)}}^{3}}}} \\ = \frac{{{{c}^{2}}k_{\parallel }^{2}}}{{{{\omega }^{2}}}}\frac{{{{\beta }_{ \bot }}}}{2}\frac{{\left[ {A\left( {1 - \frac{\omega }{\Omega }} \right) - \frac{\omega }{\Omega }} \right]}}{{(A + 1){{{\left( {1 - \frac{\omega }{\Omega }} \right)}}^{3}}}} \\ = \frac{{{{c}^{2}}k_{\parallel }^{2}}}{{{{\omega }^{2}}}}\frac{{{{\beta }_{ \bot }}}}{2}\frac{{\left[ {A(1 - x) - x} \right]}}{{(A + 1){{{(1 - x)}}^{3}}}} = \frac{{{{c}^{2}}k_{\parallel }^{2}}}{{{{\omega }^{2}}}}\frac{{{{\beta }_{ \bot }}}}{2}\frac{{(\tilde {A} - x)}}{{{{{(1 - x)}}^{3}}}}. \\ \end{gathered} $$

Here, $x = {\omega \mathord{\left/ {\vphantom {\omega \Omega }} \right. \kern-0em} \Omega }$ and $\tilde {A} = {{A{\kern 1pt} } \mathord{\left/ {\vphantom {{A{\kern 1pt} } {(A + 1)}}} \right. \kern-0em} {(A + 1)}}$.

Then, the dispersion equation takes on the following form:

$$\begin{gathered} \frac{{{{c}^{2}}k_{\parallel }^{2}}}{{{{\omega }^{2}}}}\left( {1 + \frac{{{{\beta }_{ \bot }}}}{2}\frac{{\left( {\tilde {A} - x} \right)}}{{{{{\left( {1 - x} \right)}}^{3}}}}} \right) = \frac{{\omega _{{0i}}^{2}}}{{\Omega (\Omega - \omega )}} \\ + \,\,\frac{{\omega _{{0h}}^{2}}}{\omega }\left[ {\left( {\frac{1}{{\Omega - \omega }}} \right)} \right] + i\sqrt \pi \frac{{\omega _{{0h}}^{2}}}{\omega }\frac{{\omega - \Omega }}{{k_{\parallel }^{2}v_{{\parallel h}}^{2}}} \\ \times \,\,\exp {{\left[ { - {{{\left( {\frac{{\omega - \Omega }}{{k_{\parallel }^{2}v_{{\parallel h}}^{2}}}} \right)}}^{2}}} \right]}^{{}}}\left( {\frac{A}{\omega } - \frac{1}{{\Omega - \omega }}} \right). \\ \end{gathered} $$

(A.6)

Next,

$$\begin{gathered} \frac{{\omega _{{0i}}^{2}{{\omega }^{2}}}}{{\Omega (\Omega - \omega )}} + {{\omega }^{2}}\frac{{\omega _{{0h}}^{2}}}{\omega }\left[ {\left( {\frac{1}{{\Omega - \omega }}} \right)} \right] \\ = \frac{{\omega _{{0i}}^{2}{{x}^{2}}}}{{(1 - x)}}\left( {1 + \frac{{{{n}_{h}}}}{{{{n}_{0}}}}\frac{1}{x}} \right) \approx \frac{{\omega _{{0i}}^{2}{{x}^{2}}}}{{(1 - x)}}. \\ \end{gathered} $$

(A.7)

In the last formula it is assumed that $\frac{{{{n}_{h}}}}{{{{n}_{0}}}}\frac{1}{x} \ll 1$ and $x = {\omega \mathord{\left/ {\vphantom {\omega \Omega }} \right. \kern-0em} \Omega }$.

Thus, the dispersion formula for the considered waves is

$$\begin{gathered} \frac{{{{c}^{2}}k_{\parallel }^{2}}}{{\omega _{{0i}}^{2}}}\left[ {1 + \frac{{{{\beta }_{ \bot }}}}{2}\frac{{\tilde {A} - x}}{{{{{(1 - x)}}^{3}}}}} \right] = \frac{{{{x}^{2}}}}{{(1 - x)}} \\ - \,\,i\sqrt \pi \omega \frac{{\omega _{{0h}}^{2}}}{{\omega _{{0i}}^{2}}}\frac{{\Omega - \omega }}{{{{k}_{\parallel }}{{v}_{{\parallel h}}}}}\exp \left[ { - {{{\left( {\frac{{\omega - \Omega }}{{{{k}_{\parallel }}{{v}_{{\parallel h}}}}}} \right)}}^{2}}} \right]\left( {\frac{A}{\omega } - \frac{1}{{\Omega - \omega }}} \right). \\ \end{gathered} $$

Taking into account that $F = 1 + \frac{{{{\beta }_{ \bot }}}}{2}\frac{{\tilde {A} - x}}{{{{{(1 - x)}}^{3}}}}$ and $\frac{{\omega _{{0h}}^{2}}}{{\omega _{{0i}}^{2}}} = \frac{{{{n}_{h}}}}{{{{n}_{0}}}}$, we obtain the above formula in the form

$$\begin{gathered} \frac{{{{c}^{2}}k_{\parallel }^{2}}}{{\omega _{{0i}}^{2}}}F = \frac{{{{x}^{2}}}}{{(1 - x)}} - i\sqrt \pi \frac{{{{n}_{h}}}}{{{{n}_{0}}}}\frac{1}{{{{k}_{\parallel }}{{v}_{{\parallel h}}}}} \\ \times \,\,\frac{{(A + 1)(\tilde {A} - x)}}{x}\exp \left[ { - {{{\left( {\frac{{\omega - \Omega }}{{{{k}_{\parallel }}{{v}_{{\parallel h}}}}}} \right)}}^{2}}} \right]. \\ \end{gathered} $$

(A.8)

With the allowance for $\frac{1}{{{{k}_{\parallel }}{{v}_{{\parallel h}}}}} = \frac{{{{{(1 - x)}}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{x}\frac{{{{c}_{A}}}}{{{{v}_{{\parallel h}}}}}\frac{1}{\Omega }{{F}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}$, formula (A.8) transforms into

$$\begin{gathered} \frac{{{{c}^{2}}k_{\parallel }^{2}}}{{\omega _{{0i}}^{2}}}F = \frac{{{{x}^{2}}}}{{(1 - x)}} - i\sqrt \pi \frac{{\omega _{{0h}}^{2}}}{{\omega _{{0i}}^{2}}}\frac{{{{{\left( {1 - x} \right)}}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{x} \\ \times \,\,\left( {\frac{{{{c}_{A}}}}{{{{v}_{{\parallel h}}}}}} \right){{F}^{{1{\text{/}}2}}}\left[ {A\left( {1 - x} \right) - x} \right]\exp \left[ { - {{{\left( {\frac{{\omega - \Omega }}{{{{k}_{\parallel }}{{v}_{{\parallel h}}}}}} \right)}}^{2}}} \right]. \\ \end{gathered} $$

(A.9)

This expression almost coincides with the dispersion equation (2).

Then, using denotations ${{c}^{2}}{{k_{\parallel }^{2}} \mathord{\left/ {\vphantom {{k_{\parallel }^{2}} {\omega _{{0i}}^{2}}}} \right. \kern-0em} {\omega _{{0i}}^{2}}} = {{k}^{2}}$ and $\tilde {A} = {A \mathord{\left/ {\vphantom {A {(A + 1)}}} \right. \kern-0em} {(A + 1)}}$, we obtain

$$\begin{gathered} {{k}^{2}}F = \frac{{{{x}^{2}}}}{{(1 - x)}} - i\sqrt \pi \frac{{{{n}_{h}}}}{{{{n}_{0}}}}\frac{{{{{(1 - x)}}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{x}\frac{{{{c}_{A}}}}{{{{v}_{{\parallel h}}}}} \\ \times \,\,{{F}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}(A + 1)(\tilde {A} - x)\exp \left[ { - \frac{{{{{(1 - x)}}^{3}}}}{{{{x}^{2}}}}{{{\left( {\frac{{{{c}_{A}}}}{{{{v}_{{\parallel h}}}}}} \right)}}^{2}}F} \right]. \\ \end{gathered} $$

(A.10)

Derivation of Increment

The full dispersion equation for the considered waves has the following form (Appendix A):

$$\begin{gathered} {{k}^{2}}F = \frac{{{{x}^{2}}}}{{(1 - x)}} - i\sqrt \pi \frac{{{{n}_{h}}}}{{{{n}_{0}}}}\frac{{{{{(1 - x)}}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{x}\frac{{{{c}_{A}}}}{{{{v}_{{\parallel h}}}}}{{F}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}(A + 1) \\ \times \,\,(\tilde {A} - x)\exp \left[ { - \frac{{{{{(1 - x)}}^{3}}}}{{{{x}^{2}}}}{{{\left( {\frac{{{{c}_{A}}}}{{{{v}_{{\parallel h}}}}}} \right)}}^{2}}F} \right], \\ \end{gathered} $$

(B.1)

where ${{c}_{A}} = {{{{B}_{0}}} \mathord{\left/ {\vphantom {{{{B}_{0}}} {\sqrt {4\pi {{n}_{0}}{{m}_{i}}} }}} \right. \kern-0em} {\sqrt {4\pi {{n}_{0}}{{m}_{i}}} }}$; ${{v}_{{\parallel h}}} = \sqrt {{{2{{T}_{\parallel }}} \mathord{\left/ {\vphantom {{2{{T}_{\parallel }}} {{{m}_{i}}}}} \right. \kern-0em} {{{m}_{i}}}}}$; $F = 1 + \frac{{{{\beta }_{ \bot }}}}{2}\frac{{\tilde {A} - x}}{{{{{(1 - x)}}^{3}}}}$; $\tilde {A} = \frac{A}{{A + 1}}$; $k = \frac{x}{{{{{(1 - x)}}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}\frac{1}{{{{F}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}$; $x = {\omega \mathord{\left/ {\vphantom {\omega \Omega }} \right. \kern-0em} \Omega },$ $\omega = {{\omega }_{r}} + i\gamma$.

We then use the standard formula for determining the increment $\gamma = - \frac{{\operatorname{Im} \varepsilon (\omega ,{{k}_{\parallel }})}}{{\partial \operatorname{Re} {{\varepsilon (\omega ,{{k}_{\parallel }})} \mathord{\left/ {\vphantom {{\varepsilon (\omega ,{{k}_{\parallel }})} {\partial \omega }}} \right. \kern-0em} {\partial \omega }}}}.$

The applicability of the formula is ensured by the smallness of ratio ${{{{n}_{h}}} \mathord{\left/ {\vphantom {{{{n}_{h}}} {{{n}_{0}}}}} \right. \kern-0em} {{{n}_{0}}}}$. From (B.1), we have

$$\begin{gathered} \frac{\partial }{{\partial \omega }}\operatorname{Re} \varepsilon (\omega ,{{k}_{\parallel }}) = \frac{1}{\Omega }\left( {\frac{{\partial {{\varepsilon }_{r}}}}{{\partial x}}} \right) = \frac{1}{\Omega }\left( {{{k}^{2}}\frac{{\partial F}}{{\partial x}} - \frac{\partial }{{\partial x}}\frac{{{{x}^{2}}}}{{1 - x}}} \right) \\ = \frac{1}{\Omega }\left( {\frac{{{{x}^{2}}}}{{1 - x}}\frac{{\partial F}}{{F\partial x}} - \frac{\partial }{{\partial x}}\frac{{{{x}^{2}}}}{{1 - x}}} \right) \\ = \frac{1}{\Omega }\left[ {\frac{{{{x}^{2}}}}{{1 - x}}\frac{{\partial \ln F}}{{\partial x}} - \frac{{x(2 - x)}}{{{{{(1 - x)}}^{2}}}}} \right] \\ = - \frac{1}{\Omega }\frac{{x(2 - x)}}{{{{{(1 - x)}}^{2}}}}\left[ {1 - \frac{{x(1 - x)}}{{(2 - x)}}\frac{{\partial \ln F}}{{\partial x}}} \right]. \\ \end{gathered} $$

(B.2)

$$\begin{gathered} \operatorname{Im} \varepsilon (\omega ,{{k}_{\parallel }}) = \sqrt \pi \frac{{{{n}_{h}}}}{{{{n}_{0}}}}\frac{{{{{(1 - x)}}^{{1/2}}}}}{x}\frac{{{{c}_{A}}}}{{{{v}_{{\parallel h}}}}}{{F}^{{1/2}}}(A + 1) \\ \times \,\,(\tilde {A} - x)\exp \left[ { - \frac{{{{{(1 - x)}}^{3}}}}{{{{x}^{2}}}}{{{\left( {\frac{{{{c}_{A}}}}{{{{v}_{{\parallel h}}}}}} \right)}}^{2}}F} \right]. \\ \end{gathered} $$

As a result, we obtain the expression for the normalized increment (formula (2) in the main text of this paper):

$$\begin{gathered} \frac{\gamma }{\Omega } = {{\pi }^{{1/2}}}\frac{{{{n}_{h}}}}{{{{n}_{0}}}}\frac{{{{c}_{A}}}}{{{{v}_{{\parallel h}}}}}\frac{{(A + 1)(\tilde {A} - x){{{(1 - x)}}^{{{5 \mathord{\left/ {\vphantom {5 2}} \right. \kern-0em} 2}}}}{{F}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}}}{{{{x}^{2}}(2 - x)\left[ {1 - \frac{{x(1 - x)}}{{(2 - x)}}\frac{{\partial \ln F}}{{\partial x}}} \right]}} \\ \times \,\,\exp \left[ { - \frac{{{{{(1 - x)}}^{3}}}}{{{{x}^{2}}}}{{{\left( {\frac{{{{c}_{A}}}}{{{{v}_{{\parallel h}}}}}} \right)}}^{2}}F} \right]. \\ \end{gathered} $$

(B.3)