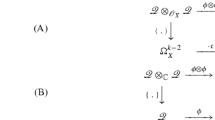Abstract
We study the graded geometric point of view of curvature and torsion of Q-manifolds (differential graded manifolds). In particular, we get a natural graded geometric definition of Courant algebroid curvature and torsion, which correctly restrict to Dirac structures. Depending on an auxiliary affine connection K, we introduce the K-curvature and K-torsion of a Courant algebroid connection. These are conventional tensors on the body. Finally, we compute their Ricci and scalar curvature.
Similar content being viewed by others
Notes
For a convenient track of the type of indices, we temporarily use, e.g., \(\Gamma _\mu {}^{s_\alpha }{}_{s_\beta }\) to denote the component contracting with \(s_\alpha \frac{\partial }{\partial s_\beta }\) in the definition of the covariant derivative, where \(\alpha \) can be \(\mu \) up and \(\mu \) down.
References
Liu, Z.-J., Weinstein, A., Xu, P.: Manin triples for Lie bialgebroids. J. Differ. Geom. 45(3), 547–574 (1997)
Gualtieri, M.: Branes on Poisson Varieties, The Many Facets of Geometry: A Tribute to Nigel Hitchin (2007)
Hohm, O., Zwiebach, B.: Towards an invariant geometry of double field theory. J. Math. Phys. 54, 032303 (2013)
Jurčo, B., Vysoký, J.: Courant algebroid connections and string effective actions. Noncommut. Geom. Phys. 4, ed. by: Y.Maeda, H. Moriyoshi, M. Kotani S. Watamura, World Scientific, pp. 211-265 211 (2017)
Garcia-Fernandez, M.: Torsion-free generalized connections and heterotic supergravity. Commun. Math. Phys. 332(1), 89 (2014)
Coimbra, A., Strickland-Constable, C., Waldram, D.: Supergravity as generalised geometry I: type II theories. JHEP 1111, 091 (2011)
Coimbra, A., Strickland-Constable, C., Waldram, D.: Supergravity as generalised geometry II: \(E_{d(d)} \times {\mathbb{R}}^+\) and M theory. JHEP 1403, 019 (2014)
Hull, C., Zwiebach, B.: Double field theory. JHEP 0909, 099 (2009)
Hull, C., Zwiebach, B.: The Gauge algebra of double field theory and Courant brackets. JHEP 0909, 090 (2009)
Hohm, O., Hull, C., Zwiebach, B.: Background independent action for double field theory. JHEP 1007, 016 (2010)
Roytenberg, D.: On the structure of graded symplectic supermanifolds and Courant algebroids. In: Voronov, T. (ed.) Quantization, Poisson Brackets and Beyond, Contemporary Mathematics, vol. 315. American Mathematical Society, Providence (2002)
Ševera, P.: Letters to Alan Weinstein about Courant algebroids, arXiv:1707.00265 [math.DG]
Vinogradov, A.M.: Unification of the Schouten and Nijenhuis brackets, cohomology, and superdifferential operators. Mat. Zametki 47(6), 138 (1990)
Bonelli, G., Zabzine, M.: From current algebras for p-branes to topological M-theory. JHEP 0509, 015 (2005)
Deser, A., Stasheff, J.: Even symplectic supermanifolds and double field theory. Commun. Math. Phys. 339(3), 1003 (2015)
Deser, A., Sämann, C.: Extended Riemannian geometry I: local double field theory. Ann. Henri Poincaré 19, 2297 (2018)
Cattaneo, A.S., Schaetz, F.: Introduction to supergeometry. Rev. Math. Phys. 23(06), 669–690 (2011)
Quillen, D.: Superconnections and the Chern character. Topology 24(1), 89–95 (1985)
Mehta, R.A.: Q-algebroids and their cohomology. J. Symplectic Geom. 7(3), 263–293 (2009)
Kotov, A., Strobl, T.: Characteristic classes associated to Q-bundles. Int. J. Geom. Methods Mod. Phys. 12(01), 1550006 (2014)
Grützmann, M., Strobl, T.: General Yang–Mills type gauge theories for \(p\)-form gauge fields: from physics-based ideas to a mathematical framework or From Bianchi identities to twisted Courant algebroids. Int. J. Geom. Methods Mod. Phys. 12, 1550009 (2014)
Roytenberg, D.: AKSZ-BV formalism and Courant algebroid-induced topological field theories. Lett. Math. Phys. 79, 143–159 (2007)
Vaintrob, A.Y.: Lie algebroids and homological vector fields. Russ. Math. Surv. 52, 428 (1997)
Abad, C., Crainic, M.: Representations up to homotopy of Lie algebroids. J. für die reine und angewandte Mathematik (Crelles J.) 663, 91–126 (2012)
Gualtieri, M.: Generalized complex geometry, arXiv preprint arXiv:math/0401221 (2004)
Ševera, P., Valach, F.: Ricci flow, Courant algebroids, and renormalization of Poisson–Lie T-duality. Lett. Math. Phys. 107(10), 1823 (2017)
Ševera, P.: Some title containing the words “homotopy” and “symplectic”, eg this one. arXiv preprint arXiv:math/0105080 (2001)
Acknowledgements
We are grateful to Christian Sämann for many discussions at an early stage of this research. P.A. and A.D. thank the University of Florence for hospitality. F.B. and A.D. thank the INFN sezione di Torino for hospitality. The research of A.D. was supported by OP RDE Project No. CZ.02.2.69/0.0/0.0/16_027/0008495, International Mobility of Researchers at Charles University, as well as by the COST action MP 1405 Quantum structure of spacetime and the Corfu Summer Institute 2019 at EISA. The work of P.A. is partially supported by INFN, CSN4, Iniziativa Specifica GSS, and by Università del Piemonte Orientale. P.A. is affiliated to INdAM, GNFM (Istituto Nazionale di Alta Matematica, Gruppo Nazionale di Fisica Matematica).
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Ruben Minasian.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Generalized Connection on \({{\mathbb {T}}}[1]M\) and Torsion Components
In this appendix, we collect our conventions on the component form of a generalized connection on \(E = {{\mathbb {T}}}[1]M\) which are used in the main text, in particular in Sect. 5 for the torsion. A generalized connection D is split into two parts, one along a vector field and another along a one form. More precisely, \(D = \nabla + V\) where
where \(\nabla ^{TT}\) and \(\nabla ^{T^*T^*}\) are the connections on TM and \(T^*M\) defined by the coefficientsFootnote 1\(\Gamma _\mu {}^\nu {}_\rho \equiv \Gamma _\mu {}^{s_\nu }{}_{s_\rho }\) and \(\tilde{\Gamma }_{\mu }{}_\nu {}^\rho \equiv \Gamma _{\mu }{}^{s^\nu }{}_{s^\rho }\), respectively, while \(\nabla ^{TT^*}\in \Gamma (T^*M\otimes TM^{\otimes 2})\) and \(\nabla ^{T^*T}\in \Gamma (T^*M{}^{\otimes 3})\) are defined by \(\Gamma _{\mu }{}^\nu {}^\rho \equiv \Gamma _{\mu }{}^{s_\nu }{}_{s^\rho }\) and \(\Gamma _{\mu }{}_{\nu }{}_\rho \equiv \Gamma _{\mu }{}^{s^\nu }{}_{s_\rho }\). Analogously, the component V decomposes as
so that we define the components of \(V^{T^*T}\) by \(V^{\mu }{}_{\nu }{}_\rho \equiv V^{\mu }{}^{s^\nu }{}_{s_\rho }\), respectively of \(V^{T^*T^*}\) by \(V^{\mu }{}_\nu {}^\rho \equiv V^{\mu }{}^{s^\nu }{}_{s^\rho }\). Similarly, for \(V^{TT}\) by \(\tilde{V}^{\mu }{}^\nu {}_\rho \equiv V^{\mu }{}^{s_\nu }{}_{s_\rho }\) and for \(V^{TT^*}\) by \(V^{\mu }{}^\nu {}^{\rho }\equiv V^{\mu }{}^{s_\nu }{}_{s^\rho }\).
With these conventions, the direct evaluation of the torsion \(T(Q_{{{\mathcal {E}}}}) = Q_{{{\mathcal {E}}}}(\tau _{{\mathcal {M}}})\) gives, as mentioned in chapter 5, three contributions
which we now give explicitly in components. They are
and
It is clear that the first term appearing in \(T(\Gamma )\) is the usual torsion of the affine connection \(\nabla ^{TT}\). As in the discussion of the (1, 1) component of the curvature, we can introduce an affine connection on TM in order to get a covariant \(\tilde{p}\) so that \(T^{(1,1)}(\Gamma ,V)-\tilde{p}_\mu s^\mu \in \Gamma (T^*M\otimes TM\otimes {{\mathbb {T}}}M)\). This gives the K-dependent form of the (1, 1) component of the torsion, as described and used in (36) of the main text.
Lagrangian Submanifolds of \(T^*[2]T[1]M\)
It is known that lagrangian submanifolds of \(T^*[2]T[1]M\) invariant under the homological vector field correspond to Dirac structures of the Courant algebroid associated to it (e.g., section 4 of [27]). In this appendix, we work out explicitly the details of the characterization of Dirac structures in this language which is particularly useful for the main text. Let \(L \subset {{\mathbb {T}}}M\) be a Dirac structure; we are going to describe the corresponding \(d_{{\mathcal {M}}}\)-invariant lagrangian submanifold \({{\mathcal {L}}}_L {\mathop {\hookrightarrow }\limits ^{\phi }} T^*[2]T[1]M\).
As a Lie algebroid, L is described by the NQ-manifold L[1] of degree 1. Locally, let us choose for the latter coordinates \((y^\mu ,\lambda ^A)\) for L[1] of degrees (0, 1) and for the Courant algebroid \((x^\mu , \psi ^\mu , b_\mu , p_\mu )\) as in the main text. Finally, let us denote by \(\omega = dx^\mu dp_\mu + d\psi ^\mu db_\mu \) the canonical symplectic structure of \(T^*[2]T[1]M\). We describe the embedding \(\phi \) by the following formulas:
where \(\rho ^\mu _A\) and \(\rho _{\mu A}\) denote the components of the maps \(\rho _{T^*}\) and \(\rho \) introduced in Sect. 4 and \(\phi _{\mu AB}\) to be determined. For a basis of vector fields, using (54) this means
\({{\mathcal {L}}}_L\) being lagrangian means \(\phi ^* \omega = 0\), which gives the following two conditions:
The first condition fixes \(\phi _{\mu AB}\) in the transformations (54), and the second one is satisfied thanks to the fact that L is lagrangian.
It is now a direct computation the check that \(d_{{\mathcal {M}}}\) restricts to \({{\mathcal {L}}}_L\), indeed \(\phi _*(d_{L[1]})=d_{{\mathcal {M}}}\) follows from involutivity of L.
Rights and permissions
About this article
Cite this article
Aschieri, P., Bonechi, F. & Deser, A. On Curvature and Torsion in Courant Algebroids. Ann. Henri Poincaré 22, 2475–2496 (2021). https://doi.org/10.1007/s00023-021-01024-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00023-021-01024-5