Stochastic model for drought analysis of the Colorado River Basin

Abstract

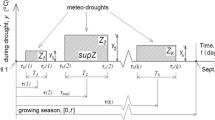
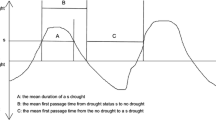
Extreme changes in weather across the globe are substantially responsible to incite calamities like drought. While we cannot control weather, it is a harsh reality that we cannot stop droughts. However, the consequent detriments can discerningly be reduced to a tolerable limit by developing long term rational planning. To attain this objective, probability models are imperative as they measure and analyze the variation in the random phenomena in an orderly way and help to collect useful information leading toward meaningful predictions. In this paper, an explicit distribution based on the convolution of stochastic variables is derived from the Bivariate Affine-Linear Exponential (BALE) distribution to model the interarrival time of drought. The proposed model follows the trend of the observed drought data of the Colorado Drainage Basin in the USA that provides the rationale for its reliable forecasting. The return periods are also estimated for the interarrival time of drought to obtain important inferences for future planning. Finally, some quantiles associated with this model are provided, which are useful to predict changes in the interarrival times of droughts.

Appendices

Appendix 1

Case	Non-drought duration (months)	Drought duration (months)
1	15	10
2	12	3
3	3	10
4	5	39
5	8	16
6	4	9
7	48	10
8	3	2
9	17	10
10	14	5
11	26	28
12	31	6
13	19	4
14	3	5
15	53	6
16	5	11
17	8	2
18	2	7
19	2	21
20	7	16
21	2	3
22	21	17
23	20	12
24	4	6
25	4	7
26	7	7
27	24	8
28	8	5
29	2	19
30	6	3
31	2	7
32	5	26
33	4	10
34	16	15
35	8	16
36	7	25
37	5	2
38	14	16
39	16	4
40	2	3
41	31	8
42	17	11
43	7	9
44	5	15
45	5	5
46	8	6
47	5	11
48	71	19
49	4	18
50	3	3
51	9	5
52	19	9
53	13	3
54	2	6
55	23	2
56	2	4
57	6	59
58	14	8
59	8	9
60	3	9
61	7	15
62	10	25
63	13	7
64	9	2
65	2	6
66	3	20
67	9	18

Appendix 2

Proof 1

Using the transformations $S = X + Y$ and $W = \frac{X}{X + Y}$ in (3), the joint density of S and W becomes.

$$f\left( {s,w} \right) = \left( {\beta s + \gamma ws^{2} } \right)\exp \left( { - sw - \beta s + \beta sw - \gamma s^{2} w + \gamma s^{2} w^{2} } \right).$$

(8)

Using (8), the pdf of S can be written as

$$f_{S} \left( s \right) = \int\limits_{0}^{1} {\left( {\beta s + \gamma s^{2} w} \right)\exp \left( { - sw - \beta s + \beta sw - \gamma s^{2} w + \gamma s^{2} w^{2} } \right)} \,\,\,dw,$$

Using Mathematica and simplifying, we get (4).

To derive the moments of S, we need the following lemma:

Lemma 1

Prudnikov et al. (1986) [vol. 1, Eq. (2.3.6.9), p-324]. If $a,b \in {\mathbb{R}},\,\,s > 0\,$ and $\,|\arg \,c| < \pi ,$

$$\int\limits_{0}^{\infty } {\frac{{x^{a - 1} }}{{\left( {c + x} \right)^{b} }}\exp \left( { - sx} \right)\,dx = \Gamma \left( a \right)\left( c \right)^{a - b} \Psi \left( {a,a + 1 - b;cs} \right)} ,$$

where $\Psi \left( . \right)$ is Kummer’s (confluent hypergeometric) function of second kind which is given by

$$\Psi \left( {x,y;z} \right) = \frac{1}{\Gamma \left( x \right)}\int\limits_{0}^{\infty } {\exp \left( { - zt} \right)t^{x - 1} \left( {1 + t} \right)^{y - x - 1} \,\,dt.}$$

Now the product moment of the distribution proposed in (1) is obtained as:

$$E\left( {X^{p} Y^{q} } \right) = \alpha \int\limits_{0}^{\infty } {\int\limits_{0}^{\infty } {x^{p} y^{q} \left( {\beta + \gamma x} \right)} } \exp \left( { - \alpha x - \beta y - \gamma xy} \right)\,\,dydx,\,\,\,\,\,\,\,p,q = 1,2,....$$

$$= \frac{{\alpha \,\Gamma \left( {q + 1} \right)}}{{\gamma^{q} }}\int\limits_{0}^{\infty } {\frac{{x^{{\left( {p + 1} \right) - 1}} \exp \left( { - \alpha x} \right)}}{{\left( {\frac{\beta }{\gamma } + x} \right)^{q} }}} \,\,\,\,\,\,\,dx.$$

Using Lemma 1 and further simplifying we arrive at

$$E\left( {X^{p} Y^{q} } \right) = \frac{{\alpha \,\beta^{p - q + 1} }}{{\gamma^{p + 1} }}\Gamma \left( {q + 1} \right)\Gamma \left( {p + 1} \right)\Psi \left( {p + 1,p - q + 2;\frac{\alpha \beta }{\gamma }} \right).$$

(9)

Putting $\alpha = 1,$ we get from (9)

$$E\left( {X^{p} Y^{q} } \right) = \frac{{\beta^{p - q + 1} }}{{\gamma^{p + 1} }}\Gamma \left( {q + 1} \right)\Gamma \left( {p + 1} \right)\Psi \left( {p + 1,p - q + 2;\frac{\beta }{\gamma }} \right),\,{\text{for}}\,p > - 1\,\,{\text{and}}\,\,q > - 1.$$

(10)

Proof 2

The result follows by writing $E\left( {S^{n} } \right) = E\left( {X + Y} \right)^{n} = \sum\limits_{k = 0}^{n} {\left( {\mathop {}\limits_{k}^{n} } \right)} E\left( {X^{k} Y^{n - k} } \right)$ and applying (10) to each expectation in the sum, we get the result stated in (5).