Introduction

This paper considers the place of mathematical activity in Initial Teacher Education (ITE) for elementary school preservice teachers. As a group of researchers working on ITE programmes in four English universities, we explore preservice teachers’ learning within the context of mathematical activities in a university-based ITE session, and as they plan and evaluate their subsequent teaching of mathematics. Due to the diverse elementary school curriculum demands and time constraints, opportunities for preservice elementary teachers to engage with mathematical activities themselves, and to reflect on them, can be limited. Our focus on prospective teachers doing mathematics for themselves has drawn us to aspects of the theory of enactivism, which posits that “all knowing is doing” (Maturana and Varela 1998, p. 26), and to Mason’s (2010) conceptualisation of teaching and learning as relating to awareness. These two perspectives are strongly related in that they both share the view that sensory and cognitive functioning has a biological basis; and that knowing is in our perceptions and actions, and in reflection upon our actions (Preciado‑Babb, Metz and Marcotte 2015).

Mason (2010) argues that the role of the mathematics teacher is to direct students’ shifts of attention, and that working on mathematics for themselves can engage teachers in retro-spection and pro-spection of their teaching. Referring to Diderot’s phrase ‘l’ esprit d’ escalier’, Mason (1994, p. 10) explains what he calls “spection” as “the retrospective thought on the stairs after an incident when you think of what you could have said or done”. We view Mason’s (2010) notion of spection as being closely linked to the enactivist notion of deliberate analysis (Varela 1999), defined as the way that expert teachers are able to act spontaneously and to analyse their actions retrospectively, as they reflect upon the reasons for their actions. Both notions underscore the importance of retrospective thought about an action or event for the awakening of awareness that can inform future action in teaching and in learning.

In this study, we combine deliberate analysis with spection. We employ the term deliberate retrospective analysis (or deliberate retro-spection) and adopt the position that deliberate retrospective analysis of one’s actions can be used as a means for novice teachers to learn and become expert (Brown and Coles 2011; Varela 1999). We therefore focus on exploring the expressed, explicit and articulated awarenesses that emerge when preservice teachers are encouraged to engage with deliberate retro-spection of their own learning, and how this subsequently may inform pro-spection of their teaching.

Previous research has explored the notion of deliberate analysis with a focus on teaching actions, such as task design and listening to students in the classroom (e.g. Brown and Coles 2012; Coles and Brown 2016; Davis 1997; Mason 2008; Preciado‑Babb, Metz and Marcotte 2015; Towers and Proulx 2013). Preciado-Babb, Metz and Marcotte (2015) bring together elements of enactivism with Mason’s notions of awareness, and apply these to the learning of practising teachers. Our study differs from this research in that our focus is on the action of preservice teachers “working on mathematics for themselves” (Mason 2010, p. 43).

We investigate what preservice teachers sensitise themselves to when they engage in deliberate, retrospective analysis of their own mathematical actions when working on generalisation in the familiar context of visual growing patterns, and when they attempt to construct a mathematical argument about the validity of a generalisation. We shall present preservice teachers’ articulated awarenesses about the learning and teaching of mathematics that emerged from our analysis of qualitative data from interviews, lesson plans and lesson evaluations.

Exploring the kinds of awarenesses that preservice teachers articulate within an environment that encourages deliberate retrospective analysis of their learning and implications for teaching is highly significant for understanding mechanisms that can enhance prospective teachers’ capabilities for critical pedagogical action.

We seek to address the following research question:

What are the kinds of expressed awarenesses that emerge when preservice teachers engage in a process of deliberate retro-spection of their own mathematical activity and pro-spection of their teaching relating to generalisation of visual patterns?

The paper contributes a new dimension to the discussion about the focus of novice elementary school teachers’ retrospective reflection by examining how deliberate retrospective analysis of doing mathematics, and not only of teaching actions, can develop awarenesses that underlie the growth of expertise in teaching mathematics.

Theoretical perspectives

Varela, Thompson and Rosch (1991) introduced enactivism as a modern continuation of the phenomenology of Merleau-Ponty (1962), articulating an all-encompassing conceptualisation of learning in biological organisms such as cells, species and societies. In mathematics education, researchers increasingly draw on enactivism as a theory of cognition that can be utilised to explore the learning of students and teachers. We draw particularly on the work of Brown and Coles (Brown and Coles 2011, 2012; Coles and Brown 2016) to explicate the theoretical perspective in which we position this study.

In enactivism, the role of the body is central to conceptualising human knowledge/knowing in meaning-making processes. This focus on embodied cognition counters enduring notions, influenced by a Cartesian view of mind–body, that thought and learning are brain-based, dislocated from emotion and identity. From an enactivist perspective, knowledge is not acquired but “depends on being in a world that is inseparable from the human body, language, and social history” (Varela, Thompson and Rosch 1991, p. 149). Hence, as Coles and Brown (2016) explain, knowing cannot be separated from the knower nor the context in which the knower acts. Thus, what we are able to become aware of depends on how we relate, through our senses, in a physical way with the world around us.

The concept of knowledge as action is key, and enactivists often refer to knowing rather than knowledge. Brown and Coles (2011) emphasise the equivalence of knowing and doing (Maturana and Varela 1998) and that doing is also knowing. Knowing and action are dependent on what individuals perceive. Varela, Thompson and Rosch (1991) foreground processes of categorisation in a sensory world as a component of knowing. Within enactivism, making distinctions is seen as individuals’ “basic mental function” (Coles and Brown 2016, p. 157) and learning is thereby a process of making ever-finer distinctions that allow us to act in our complex world (Brown and Coles 2012). Likewise, Mason (2002) views learning as becoming more sensitive to distinctions and developing awareness of connections amongst distinctions.

Mason (1998) extends the notion of awareness in the context of teacher learning, arguing that teaching involves the development of a complex of awarenesses. He proposes that “at the heart of the matter of learning from experience is the person’s attention” (Mason 2010, p. 35). For Mason (op. cit.), the purpose of teachers’ personal engagement in mathematics is to sensitise themselves to the struggles that their pupils experience. Knowing-about mathematics or theories of learning does not in itself guarantee knowing-to. Knowing-to is rather “a state of awareness, of preparedness to see in the moment” (Mason and Spence 1999, p. 151).This is the essence of Mason’s “spection”, which is “being awake in the moment, noticing and responding freshly and creatively in the instant, catching oneself before embarking on habitual behaviour” (1994, p.10). Thus, “spection” is retrospective thought after an incident that interrogates what could have been done, and the need to seek out habits and “make them available for re-questioning…. to maintain a conjecturing ‘as-if’ stance towards the whole process of learning and teaching” (Mason 1994, p. 10).

While Mason links “spection” with the process of reflection, he argues that mere “thinking back” is not sufficient. He notes:

While reflection continues to be an ill-defined and overly used term, I use it to refer to retrospective re-entering of salient moments from the recent past, and attempting to give accounts of these in descriptions which do not embellish, judge or justify … To prepare for future actions, I am prospective by mentally imagining myself in a typical situation in which I wish to work differently, and projectively imagining myself responding in the way I wish. (Mason 1994, p. 11).

On this basis, retro-spection and pro-spection are proposed as actions through which teachers can alert themselves to the struggles that students experience, “issues that may need probing and actions to take in order to promote responding freshly and more sensitively to situations that emerge” (Mason 2010, p. 43).

Mason proposes the use of “critical incidents” as a pedagogical tool that can trigger memories of one’s past experience which can then become the “objects for analysis and comparison with others” (Mason 1994, p. 9). Mason cautions that the descriptions of past events that one initially creates from re-entering salient moments of the past should be free from judgement statements before one begins to subject such simple descriptions to analysis. Simple, judgement-free descriptions of one’s past experience of an event take the form of “data” that one will then compare, analyse and interrogate in the search of patterns and different possibilities for future action.

We therefore argue that Mason’s (2010) notion of “spection” and his position about the fundamental role that analysis and comparison of past experiences plays in learning is closely related to the enactivist notion of deliberate analysis, which denotes the way that expert teachers are able to act and analyse their actions retrospectively, unearthing the reasons that underlie their actions (Varela 1999), enabling them to be open to alternative options for future action.

Discussing an enactivist approach to learning and teaching mathematics, Brown and Coles (2012) make a point similar to that made by Mason above, arguing that reflecting on its own is not sufficient for learning, if it does not include a level of challenge and tension. They therefore argue that rather than seeking a definition of what reflection is, it is more important to ask how individuals can undertake reflecting for learning. Brown and Coles (ibid) posit that the enactivist notion of “deliberate analysis” provides a useful approach to addressing this question. Deliberate analysis “steers a middle path between unreflective spontaneity of action (which is not open to analysis) and deliberateness (which characterises the way beginners are often in the position of needing to rationally decide each course of action)” (Brown and Coles 2011, p. 862). They propose that deliberate analysis involves reflecting upon a previous, spontaneous, action and considering why the action was taken. This post hoc analysis allows the reconstruction of “intelligent awareness” (Varela 1999, p. 32) underpinning the action. Therefore, taking the “middle path” involves “maintaining an on-going alertness to the detail of what we experience” (Brown and Coles 2012, p. 223).

In our work, we view Mason’s (1994) notion of “retrospective reflection” or “retro-spection” and the enactivist notion of “deliberate analysis”, as explained by Brown and Coles (2012), as notions that share the premise of the importance of retrospective thought to enable the emergence of awareness that can shape future action. We therefore bring together these notions and propose that engagement with “deliberate retrospective analysis”, operationalised in our paper as the retrospective re-entering of salient moments of mathematics activity and reflection upon one’s own “doing” of mathematics, followed by prospective imagining of teaching practice, can be productive for preservice teachers in their trajectory towards building expertise in mathematics teaching.

Research by both Mason (1998) and Brown and Coles (2012) has examined novice teachers’ retro-spection and deliberate analysis in relation to teaching events and teaching actions. In our research, we focus on preservice teachers’ “doing mathematics for themselves”. In our work the “event” or “action” is preservice teachers’ own mathematical activity, and we examine the awarenesses that emerge from their deliberate retro-spection of this activity, and how it connects with pro-spection of their own teaching.

We recognise the limitations of an empirical paper such as this in addressing the full complexity of the theoretical notions that we draw upon, but in this section we have presented key aspects of the ideas that we focus on in theoretically framing our research. We now proceed to review existing research on learning and teaching generalisation, in the context of visual growing pattern activities.

Review of literature on visual growing patterns

A common context for generalising in mathematics teaching and learning, sometimes referred to as “growing patterns”, is a sequence of geometric figures constructed from, for example, matchsticks, squares or dots. The representation of triangular numbers as rows of dots is a familiar example. Engagement with sensorimotor patterns offers opportunities for learners to perceive regularity. Mason et al (1985) describe three stages in generalising a pattern or relationship: seeing or recognising the pattern, articulating a description of it, and making a written recording. There is a growing body of research which explores the ways in which learners attempt to generalise, but relatively little research on how teachers become alert to learners’ struggles and how they learn to address them.

Literature on learners’ work on pattern generalisation identifies the value of “manipulating the figure itself to make counting easier; finding a local rule (recursion) which reflects one way to build the next term from previous ones; (and) spotting a pattern which leads to a direct formula” (Mason 1996, p. 75–76). This distinction between finding a local, recursive relationship and a direct, functional relationship (e.g. Ferrara and Sinclair 2016) is a theme running through the literature on pattern generalising, with learners finding the identification of functional relationships more difficult (MacGregor and Stacey 1993; Stacey and MacGregor 2001). Wilkie and Clarke (2016) found that directing learners to consider a figure much further in the sequence prompted some to move from a recursive to a functional generalisation.

Other literature identifies the significance of visualisation in pattern generalisation, focusing more closely on learners’ sensorimotor experience. Drawing on enactivism, Samson and Schäfer (2011) identify figural pattern generalisation as an embodied process. Ferrara and Sinclair (2016) found that the incorporation of tangible materials into classroom routines motivated shifts in pupils’ awareness of the relationship between the position number and the number of elements in the figure. The use of such materials, of colour to represent different components, and questioning about the pattern, position and unknown positions were also found to be helpful (Warren and Cooper 2008).

Seeing the structure of a geometric figure supports what Bills and Rowland (1999) refer to as “structural” generalisation. This is in contrast to “empirical” generalisation (op. cit.) in which learners look for an arithmetic relationship in their results (Bills and Rowland 1999), so that the resulting empirical generalisation is then “divorced from the structure of the pattern” (Küchemann 2010 p. 233). Hewitt (1992), referring to the matchstick square figure (that we discuss later, see Fig. 1), points to the way in which attending to how elements are placed or counted can support structural generalisation. In our work, we draw on research on learners’ responses to visual growing patterns, and recognise that teachers have an array of pedagogical choices to make. We are interested in the ways that preservice teacher education develops awareness of such choices.

Fig. 1
figure 1

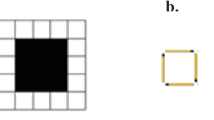
The matchstick problem as presented in Hershkowitz et al. (2001)

Full size image

Discussion of teachers’ and preservice teachers’ engagement with such patterns is to be found in the literature. Hershkowitz, Arcavi and Bruckheimer (2001), discussing the same figure as Hewitt (1992), analysed teachers’ work on the number of matchsticks needed to build an n x n square (Fig. 1).

The authors identify a range of “visually driven” solutions to the problem, which include decomposing the structure into substructures and units. The most popular strategy involved “counting” n matches in each row and noticing that that are n + 1 rows, and similarly n + 1 columns each with n matches, leading to 2n(n + 1) altogether. The authors report that, in spite of repeated visual prompts, many teachers persisted with an unsuccessful numerical (i.e. “empirical”) approach. They speculate that this may be because:

(a) their mind’s eye was not used to visual analysis, and/or (b) visual means were not highly regarded and not considered as a legitimate mathematical way to produce a general and formal solution. Some of the teachers were unaware of or unable to appreciate that a general solution and its justification can be produced entirely visually (Hershkowitz et al. 2001, p. 263).

There is much evidence that elementary teachers and preservice teachers find pattern generalisation an area of difficulty (Goulding, Rowland and Barber 2002; Wilkie 2014; Demonty, Vlassis and Fagnant 2018). Wilkie (2016) highlights the challenge and “importance of teachers developing their own ability to generalise patterns and to learn to understand the process by which students develop functional thinking through recursive and explicit generalisation” (p. 270). Yeşildere-Imre and Akkoç (2012) found that opportunities to observe the teaching of pattern generalisation enabled preservice teachers to recognise children’s difficulties and to use particular pedagogical strategies. However, like their school mentors and the teachers discussed in Hershkowitz et al. (2001), preservice teachers still preferred to find patterns in tables of numbers rather than to refer to visual representations. Vale, Pimentel and Barbosa (2018) argue for rich tasks, including visual growing patterns, to be part of teacher education, with their potential for sharing strategies and making connections between visual and non-visual forms of reasoning. They report research claiming that preservice teachers can be taught the act of “seeing” as a problem-solving strategy, and to formulate and identify elegant solutions to problems.

Methodology

In adopting an enactivist approach in this study we recognise that our beliefs and attitudes concerning mathematics teaching and learning influence the research process, and that we embody these beliefs in our methodological decisions (Brown 2015). We address the issue of reliability in enactivist research by giving detailed accounts of each aspect of our research process (Lozano 2015).

An important feature of enactivism is the engagement of multiple researchers (Reid and Mgombelo 2015). In our research team, we recognise that by working together, we bring multiple interpretations of our data. We collected pilot data of preservice teachers’ growing pattern activities in two of our universities, and of their reflections on taking part in the activities. Between each taught session we met as a group to discuss our interpretations of these data, recognising and respecting our differing backgrounds and perspectives (Reid 1996). During these sessions, our focus was on identifying and understanding what helped the preservice teachers to generalise, on the order of activities and how effective our data collection tools were. These decisions were refined to develop our methodological approach.

Research design and methods

Two universities were selected in which to focus our fieldwork: both of them provide one-year postgraduate elementary Initial Teacher Education (ITE) programmes. University One (U1) offered a mathematics specialist option within its ITE programme, and all eight preservice teachers taking this option agreed to participate in our study. University Two (U2) ran a generalist programme, including a mathematics component for all its ITE students, and seven of these preservice teachers opted to be involved in the study. One of the authors of this paper was a teacher educator on the U1 programme; another author was a U2 teacher educator.

In each of these programmes, a taught session on algebraic reasoning took place; this included a selection of growing pattern activities agreed by the two teacher educators. These are shown in Fig. 2a and b. Significantly, the function related to the “flowerbed” sequence is linear, whereas the “matchsticks” function is not. During these sessions, preservice teachers worked on the activities in pairs or small groups. They were encouraged to explore a range of ways that the problem could be “seen” and to share their experiences with the wider group. In university-based ITE programmes in England, the pedagogy linked to the mathematical content is typically addressed alongside consideration of the mathematical activity: during the algebraic reasoning session, the preservice teachers reflected on pedagogical approaches that they might draw on in their own teaching.

Fig. 2
figure 2

a Flowerbed growing pattern b Matchstick squares growing pattern

Full size image

After each class session, on the same day in most cases, the participants were interviewed individually by visiting members of the research team who had not been involved in the class. They asked introductory questions about the participant’s mathematics background, and their approaches to the growing pattern tasks. The participants brought jottings made in the session to inform their description of their approaches. Semi-structured interviews provided an opportunity for participants to revisit actions and to analyse retrospectively the mathematics that they enacted, illuminating key elements from their own perspectives.

The mathematics specialist preservice teachers at U1 had more time allocated to mathematics on their ITE programme. They were asked to prepare a lesson which involved reasoning about growing patterns, to teach to a group of elementary-aged pupils. To assist these preservice teachers’ planning of this lesson, the university teacher educator showed them some other possible pattern “starting points”.

Lesson plans and subsequent lesson evaluations were collected. A group session, where all the preservice teachers in attendance contributed to a discussion about their own lessons, was audio recorded with their permission.

Data analysis

We analysed data from interviews, lesson plans, lesson evaluations and the recorded follow-up university session over two phases. In the first phase, the project team worked in pairs focusing on the mathematical actions described by each preservice teacher in the interviews, and as illustrated in their jottings. We identified preservice teachers’ different approaches to generalising the visual patterns, and identified shifts in reasoning, with attention to recursive and functional relationships (Ferrara and Sinclair 2016). This analysis was presented at two conferences in the UK (Alderton et al. 2017; Rowland et al. 2018).

In the second phase, our analysis foregrounded Mason’s (2010) “Working on mathematics for themselves” as a key mechanism for developing teachers’ “spection”. In this paper, we present findings from data that were analysed across the dimensions of retro-spection and pro-spection relating to “Working on mathematics for themselves”.

We now describe three analysis Cycles.

Cycle 1 using the framework

Initially, we worked individually on the full set of data from each participant to identify all their comments, written and spoken, relating to deliberate retrospective analysis of their own mathematical action and their related pro-spection of teaching actions. In line with enactivist approaches to data analysis, we valued our multiple perspectives (Reid 1996), sharing our analysis in pairs before reviewing with the whole research team. The examples for each participant were categorised using a framework that we developed drawing on Mason (2010). Figure 3 exemplifies this process. In this case, Jacob’s retro-spection is on his work on the matchsticks pattern, followed by data from his pro-spection about a proposed lesson.

Fig. 3
figure 3

Framework of analysis informed by Mason (2010)

Full size image

The anticipated purpose and value of preservice teachers’ reconstruction that took place in the interview, lesson plan, evaluation and follow-up session, is that, as Mason (2010, p. 32) notes, “we do not usually learn from the experience alone … Just because I engage in mathematical activity, it does not follow that I am aware of the activity itself as a whole. As many teacher educators have found, some people are disposed to reflect on their experience, to pick out moments and ponder them, and others seem not to be so disposed”.

Cycle 2 identifying threads

Cycle 2 of our analysis involved identifying “threads” of retro-spection and pro-spection evident through all the data sources for each preservice teacher. We identified examples of preservice teachers talking retrospectively about a particular approach that had been helpful or challenging for them when working on the mathematics themselves, and talking prospectively about this approach in preparation for their teaching, either in the interview transcript or their lesson plan. Again, we worked in pairs, initially to identify these threads and specific extracts from the data sources, before discussing them across the whole project team. By way of illustration, we list here the threads identified to be running through the data of four of the participants (pseudonyms are used at all points in the paper) (Fig. 4).

Fig. 4
figure 4

Examples of threads

Full size image

Cycle 3 expressed awarenesses

In the final cycle, we conducted inductive thematic analysis (Boyatzis 1998) within the retro-spection and pro-spection threads of Cycle 2. This enabled us to identify similarities and differences between the threads and resulted in the grouping of threads under key themes of expressed awarenesses. For example, Annie’s (U1) Thread 1 (which focused on the importance of using colours and physical resources) and Alice’s (U2) Thread 1 (about working on maths for herself and the use of visuals/manipulatives), along with other threads, were grouped under a theme which we named “Awareness of the role of resources in helping them ‘see’ the pattern”. We identified four themes:

  1. 1.

    Awareness of “how you see” a pattern;

  2. 2.

    Awareness of possible difficulty to link what you “see” with a mathematical expression;

  3. 3.

    Awareness of the role of resources in helping them “see” the pattern; and.

  4. 4.

    Awareness of how others “see” the pattern.

In this paper, we present our findings concerning the first three of these themes, because our present focus is on preservice teachers’ expressed awareness emerging from deliberate retrospective analysis of their own mathematical activity. The fourth theme is the focus of our on-going collaborative research.

Expressed awarenesses in emerging threads of retro-spection and pro-spection

In this section, we present preservice teachers’ expressed awarenesses concerning the first three themes stated above.

Awareness of “how you see” a pattern

Deliberate retro-spection on preservice teachers’ own experience when working with generalisation tasks elicited an expressed awareness of the elements of the patterns that they noticed. This revealed interesting variation in the ways in which individuals saw the visual pattern, including awareness of productive and not-so-productive observations as they sought a general “rule”. Factors that shaped preservice teachers’ own observations and approaches to generalisation were subsequently noted in their expressed views about their subsequent approach to teaching generalisation.

Steve (U1) explained that he immediately focused on how the flowerbed pattern was developing and noticed the elements that were constant and those that were changing as the pattern grew.

The first way in which I saw this pattern developing was to look at it as though there was always going to be four in the top and bottom corners remaining the same … And so the variable within that must have been what was taking place on the …, well, on the edges of the square. … I quickly recognised that, oh it’s increasing by four each time, so if it’s dependent on, so it’s, if the variable’s four and I’ve got those four constants then it must be 4 multiplied by the case number added to the 4.

(Steve, U1, Interview, Flowerbed)

Steve reflected on the significance of noticing and identifying what is the “same and different” in each case of the pattern and the role that this has in reaching generalisations. He also noted that while it was easy to see the elements that remained constant in the flowerbed pattern, he found the matchstick pattern (Fig. 5) more challenging.

…I think to begin with I was attempting to do something on the outside, because you could see it, so I was aware that the perimeter was growing and the squares within it was increasing. … But then I was struggling to actually generate any sort of, or generalise further, and then I looked at…a little bit more, and I saw the horizontal and vertical.

(Steve, U1, Interview, Matchsticks)

Fig. 5
figure 5

Steve’s jottings when working with the matchstick pattern

Full size image

Steve went on to reflect on how he might include opportunities for children to work with growing patterns, noticing what is the “same and different” in order to generalise. His comments below underscore the importance of exploring and studying elements of visual patterns that change, and indicate an aspiration towards structural generalisation (Bills and Rowland 1999).

…being encouraged to look at it and see … how that formula’s been generated and where it’s actually coming from, rather than just a simple set of procedures that you’re expected to follow in order to generate…

(Steve, U1, Interview)

Like Steve, John, U1 referred to his notes on the Flowerbed problem (Fig. 6). He explained that he had also focused on the elements of the pattern that remained constant.

The first thing that I sort of was thinking about was the fact that they’ve all got four corners which stays the same. And then I thought about the dimension of the actual … square in the middle.

(John, U1, Interview)

Fig. 6
figure 6

John’s jottings when working with the flowerbed pattern

Full size image

John’s planned questioning (Fig. 7), suggested a focus on aspects that he found helpful (i.e. looking for what is changing and what stays the same) when working on the mathematical task himself, which he described in interview.

Fig. 7
figure 7

Extract from John’s lesson plan

Full size image

Describing his own approach to the matchstick pattern, Terry, U1 explained that he saw the structure of each case of the pattern as consisting of squares, which he shaded, and single matchsticks around the perimeter of each shape (Fig. 8).

I looked at what stayed the same and what was different each time. So for them, I first went, OK, so this one is going to be a square, so I just tick these…Yes, and then I did the same with the bottom. So then I had one square, two squares, plus four sticks [referring to case 2 of the pattern].

(Terry, U1, Interview, Matchsticks)

Fig. 8
figure 8

Terry’s jottings when working with the matchstick pattern

Full size image

Subsequently, Terry explained the importance that he attached to children being allowed time in the lesson to try different approaches and discuss these with others. His view is based on what he found helpful when working on the patterns himself.

I think giving children time to explore and really try and go as deep as they can in terms of a problem … because there’s so far that you can go with it, but I wouldn’t want to rush through something. Giving children plenty of opportunity to discuss, I think that’s quite important. … Because that’s what helped me.

(Terry, U1, Interview)

In school, Terry taught a lesson on generalisation with a group of Year 4 children (age 8–9). This was based on a sequence of “rocket” shapes, as shown below. We do not know how Terry came across this idea, but see Lawrence and Hennessy (2002) for a discussion about this growing pattern.

His prospective plan indicated an emphasis on allowing children time to explore their own different methods and to apply their preferred representation to work out a general rule (Fig. 9).

Fig. 9
figure 9

Extract from Terry’s lesson plan

Full size image

Elsa (U2) referred to her jottings (Fig. 10) and explained that she initially saw each case of the matchstick pattern as consisting of squares before focusing her attention on the rows and columns of matchsticks.

OK, there is four matchsticks and … they are divided in rows and columns, so here there’s one in a column and then they’re in rows. And then when it gets to this case [with reference to the 2 × 2 pattern], there’s two in rows and two and two, so six. And then in columns there’s six. And then you, the same thing for each case, and you realise that when you get, well you could try as many and there were always x number of columns, x number of rows, but the rows, there was always one more basically.

(Elsa, U2, Interview, Matchsticks)

Fig. 10
figure 10

Elsa’s jottings when working with the matchstick pattern

Full size image

In the above extract, Elsa described her approach to structural generalisation (Bills and Rowland 1999) focusing on how the visual structure of the pattern relates to the number of matchsticks that are needed to build each of the cases. Later, discussing the teaching approach that she would adopt, she highlighted the importance of prompting children to work on structure within the visual pattern and the relationships between numbers.

… I think it’s so important to do it like this because you get children to, first of all understand relations between, not just numbers but between mathematical ideas, rather than just focusing on the numbers…

(Elsa, U2, Interview)

Common themes emerging in preservice teachers’ retrospective account of their own reasoning and prospective approach to teaching were: a focus on identifying constant and variable elements in the visual pattern and thus making distinctions; the importance of connecting the visual representation with the resulting formula, in order to support their own and their students’ understanding of relations (in line with Hewitt 1992; Bills and Rowland 1999; Küchemann 2010); and the importance of allowing children to experiment with different approaches rather than imposing a particular way of working with patterns.

Awareness of difficulty translating “what you see” in a pattern into a mathematical expression of generalisation

We identified expressed awareness related to difficulties that preservice teachers experienced in their own learning followed by their anticipation of similar difficulties that are likely to arise for learners in their class.

Jacob (U1) referred back to his notes (Fig. 11), and explained that he looked at the number sequence that resulted from writing down the total number of matchsticks used for each case of the pattern, then counted the number of matchsticks that formed the inside of each square, and was able to notice that the number of internal matchsticks in each case was the same as the total number of matchsticks used to form the previous case of the pattern. For example, the number of internal matchsticks in Case 4 of the pattern is twenty-four which is the same as the total number of matchsticks used for Case 3. He noted this observation as being “really nice”, but he found it difficult to express this regularity in general terms.

I saw a perimeter and then I saw diagonals [diagonal pencil lines added by Jacob], like that, so here’s my perimeter and then that was made up of two … and one, two, three, four, one, two, three, four, one, two. And then I just thought, that’s interesting, that’s just the way I saw it. And I added them all up and figured out how many each one was, and noticed that on the inside, forget the perimeter … the number of matchsticks is equal to the whole of the last one. Which I just thought was really cool! But I carried on down that route, and found it really hard to kind of generalise from that point. I just noticed, oh that’s a really nice, you can get from the tenth term to the eleventh term, but you can’t find the tenth term by itself in that way.

(Jacob, U1, Interview)

Fig. 11
figure 11

Jacob’s jottings when working with the matchstick pattern in the session (annotation reads “Inside without perimeter = the total for the last one” and “Perimeter = 4n”)

Full size image

Jacob commented on the limitations of his initial observation and his recursive insight, noting that although it enabled him to know how the pattern grows from one term to the next term, it did not allow him to identify any term of the pattern without knowing the preceding term. Jacob was able to generalise the number of matchsticks needed for the perimeter of Case n as 4n. However, once again, he found it difficult to complete the general rule. When asked if he could generalise the perimeter, he responded:

4n, past that I think it was, looking at it in that way, as diagonals, …there might be a way, but I struggled to generalise. I suppose it would be 4n add something, but I don’t know what the something would be!

(Jacob, U1, Interview)

In his lesson on generalising patterns, Jacob planned to include the tables/chairs sequence (Fig. 12). The plan indicates his intention to draw the children’s attention to the 5th and 10th cases of the pattern. He anticipated that while 10, as a case number, is double 5, the children will eventually notice that this does not lead to double the number of chairs needed. It is interesting to note, as an aspect of his mathematical knowledge in teaching, that Jacob anticipated an error in reasoning that could have led the children to an erroneous conjecture—double the case number, double the number of chairs (e.g. Modestou and Gagatsis 2007).

Fig. 12
figure 12

Extracts from Jacob’s lesson plan

Full size image

Issues that Jacob raised when he retrospectively discussed his own difficulties with generalisation when working with a visual pattern were evident in his prospective anticipation of his own teaching. During the interview, he had reflected that sometimes you notice “nice” regularities in patterns, but moving from noticing to generalisation can be problematic: his subsequent lesson plan indicated his intention to tackle such points with the children in his class.

Hayley had worked with Jacob during the university-based generalisation session at U1, and she also expressed an awareness about the constraints associated with a recursive approach to generalising a pattern.

Jacob did adding on, but then he was like couldn’t do without knowing the previous term … so we didn’t know unless … like with 100, how do we generalise that? Because we’d have had to know 99. We were like, looked at different ways of doing it rather than doing the previous one.

(Hayley, U1, Interview, Matchsticks)

As part of her lesson plan, Hayley used a “growing crosses” pattern that had been introduced in the university session. Children are asked to consider the number of unit cubes in “cross” formations made from Cuisenaire rods (see, e.g. Rowland 1999, pp. 195ff). Hayley planned to encourage structural generalisation by asking children to identify what the 10th, 100th term and any term of the sequence would “look like” (Fig. 13).

Fig. 13
figure 13

Extract from Hayley’s lesson plan

Full size image

In subsequent retro-spection of her lesson in the follow-up university session, Hayley noted how one child saw a functional relationship which resulted in three other children then seeing it.

People saw this in different ways. So for the tenth one, they noticed that each side would have ten and then there’d be the one in the middle, and another girl saw it as you’d have the middle strip and then the two side strips, so you’d have two lots of ten, with the one in the middle, and another two lots of ten. So she saw it as a strip and sides…. It was 4 times 10, add the 1. And then we discussed then what the 100 would be. So they were like, 100 times 4 add 1, so it would be 401. And then we looked at, well will that work for any one? … so one was like, oh you could write it down as 4 times question mark add 1, and the question mark can be any number that you want it to be.

(Hayley, U1, Follow-up university session)

The extracts from Hayley’s retro-spection of her own learning experience when working with Jacob on pattern generalisation, and the expressed awareness of the possible limitations of a recursive approach were reflected in her subsequent planning for prompts and questioning that would encourage her own students to “see” and use, in their reasoning, structural and functional rather than recursive relationships.

An expressed awareness of a potential difficulty in translating what you see as changing in a visual pattern into a general rule was also identified in Fiona’s (U2) retro-spection of her own learning and views about teaching.

…because it was visual it did help me, but I didn’t quite work out how that would then relate to working out how many matchsticks there were because you know you might be adding on a certain amount but then how do you know how many are there to start with, if you’re thinking about the four, case 4 here being that section …

…we went straight for the numbers, and then she [teacher educator] was like, no, try and do it more visually. So we had to kind of take ourselves out of that and then into a visual way, and try and re-imagine it.

(Fiona, U2, Interview)

She explained how moving from an empirical approach to generalisation that seeks a functional relationship that “fits”, focusing on the numerical sequence, to working with a pattern visually, requires a shift of thinking. Her reflection on the affordances and difficulties associated with the different approaches to identifying a general rule after working with visual patterns herself, concluded with comments related to her prospective approach to teaching generalisation.

I think just doing, that will make it easier to teach algebra because I won’t be trying to teach them the n and the y and the numbers and the differences and stuff, if you go in straight with the visual, it might be more engaging, more accessible to children.

(Fiona, U2, Interview, Matchsticks)

Fiona expressed the view that a visual approach can make algebra more accessible and engaging and, in teaching, this could precede the introduction of the functional approach to generalisation.

As anticipated, the preservice teachers participating in this study expressed difficulty in moving from a recursive to a functional generalisation. This is well documented in the literature in relation to preservice teachers (Yeşildere Imre and Akkoç 2012; Wilkie 2014) and to children (MacGregor and Stacey 1993; Stacey and MacGregor 2001; Ferrara and Sinclair 2016). In our analysis, preservice teachers’ awareness of this difficulty, as expressed in deliberate retro-spection of their own mathematical action, is seen to underlie prospective, planned teaching actions, thus indicating preservice teachers’ sensitising to children’s potential difficulties in generalising visual patterns.

Awareness of the role that resources may play in helping learners to “see” the pattern

The preservice teachers’ awareness of the value that the individual’s actions and active construction of a pattern might have on learning was accompanied by critical awareness of the constraints that different kinds of resources might present for different learners, in enabling or hindering helpful visualisation of structure when working towards generalisation.

Hayley (U1) explained that the process of drawing the pattern made its rows-and-columns structure (Fig. 14) explicit to her and supported her efforts to derive a formula that represented the relationship between the different elements of the visual pattern.

I wanted to draw it out so I could count it as I was drawing it, and seeing the way I would draw it, see if the drawing would help me to see it. So I drew them out, and then … counted how many matchsticks there were. But when I was drawing it, I was drawing the rows, and then I was drawing the columns. So I saw numerically first, rather than anything. Because I was looking at the way I was drawing it while I was counting it.

… It’s the same with like building it myself, like I feel like when I do it myself, I can see it easier than just looking at that [figure] and being like—how am I going to do it now, kind of thing, if I actually draw them.

(Hayley, U1, Interview)

Fig. 14
figure 14

Hayley’s drawing of the matchstick pattern

Full size image

Hayley decided that making or drawing a pattern, as she did at the university session, would be a supportive strategy for children too when teaching her class.

Because it’s like seeing it, so them using it, or the cubes or the actual matchsticks we used, to actually have a go and see, well actually I’m adding on how they did, because if they put the matchsticks out in the rows, like I did when I was drawing, it might help them to see it and like, visualise it.

(Hayley, U1, Interview)

She subsequently planned for the pupils in her class to build and draw the pattern and provided them with squared paper and cubes during her lesson. Her planning included “Can they make the next one in the pattern? How are they making the pattern? How are they organising/counting?” (Fig. 15).

Fig. 15
figure 15

Extracts from Hayley’s assessment focus in her lesson plan and lesson evaluation

Full size image

However, when reflecting on her lesson as part of the follow-up university session, Hayley noted that there was a difference between making a pattern with materials and drawing a pattern that she was not aware of before, in terms of how supportive these two approaches and type of resource might be for children.

When they drew it, they found drawing it harder because they had to start from the beginning … to draw. But when they had the cubes, that’s when they noticed, oh you just add one more to each side.

(Hayley, U1, Follow-up university session)

In contrast to Hayley, Alice (U2) found that drawing the pattern herself “closed down” and restricted her thinking rather than enabling her to “see” the different possibilities in investigating the general rule. She therefore expressed the view that different kinds of resources might be more helpful and appropriate for work with certain patterns.

I started to draw it out myself, and then that, sort of, then closed down my thinking, to just think about one particular way of trying to find out the sort of, the algebraic reasoning that was going on, and then I just got stuck … it’s interesting to know that you could just use say one concrete resource and presume that when I’m teaching for my children, well you’ve got a concrete resource, that will naturally help you, but actually maybe it’s not the right one for that particular individual, maybe something else will then give that eureka moment to them, where it suddenly starts to work.

(Alice, U2, Interview)

While Hayley and Alice expressed different views about the usefulness of drawing when working with pattern generalisations tasks, both reached the conclusion that different types of resource might be helpful for different learners.

On the other hand, Andrew (U1) appeared to have a strong belief in the benefits of physically drawing and colouring the different parts of a pattern to reveal its structure.

I still struggle to see the corners and sides interpretation, just because I don’t, without physically having to colour it, I don’t think I could, be able to pull out the differences. …to share my answer I wrote it in a different colour, just to make sure I was doing it right.

(Andrew, U1, Interview, Matchsticks)

Andrew found several other ways of calculating the total number of matchsticks, based on seeing and drawing the structure in different ways (Fig. 16).

Fig. 16
figure 16

Andrew’s jottings while working with the matchstick pattern

Full size image

His preference for a visual approach to problem solving was also reflected in his own approach to teaching.

I incorporated that in my own teaching, so when we were looking at fractions, I insisted that everyone produced solutions visually and numerically.

(Andrew, U1, Interview)

Finally, Elsa (U2) expressed an explicit awareness of the value of concrete material in helping learners make connections between visual and symbolic representations of patterns. Elsa explained that the formula n(n + 1) (written in her notes, Fig. 17), related to the rows of matchsticks and that she was able to make the link between the visual and the symbolic, by using colour and concrete blocks of different colours to build the pattern.

I think I was seeing it as well, we had the, not the Cuisenaire, the, just the blocks, and they were different colours, and I was putting them …in different colours.

(Elsa, U2, Interview, Matchsticks)

Fig. 17
figure 17

Elsa’s jottings when working with the matchstick pattern in the session

Full size image

Elsa explained that concrete resources can reveal possible misconceptions, and support the teacher’s understanding of children’s possible misunderstandings and difficulties.

I think you could understand children’s misunderstandings better if you ask them to work out a problem like this, even if it’s Year 1. If you had a pattern of teddy bears and cars… and they didn’t recognise that pattern, you could kind of understand why, rather than asking them to solve an addition … with a wrong answer … but you don’t know what is wrong in their thinking.

(Elsa, U2, Interview)

These reflective comments on the role that concrete resources can play in helping learners to make links between visual and symbolic representations resonate with research evidence in relation to pedagogy and pattern spotting (Hewitt 1992; Warren and Cooper 2008; Wilkie and Clarke 2016). Our preservice teachers expressed an explicit sensitivity to this in relation to working on mathematics for themselves (Mason 2010) and deliberate retrospective analysis of their own mathematical activity.

Discussion

In this paper, we have explored the kinds of expressed awarenesses that emerge when preservice teachers are encouraged to engage in mathematical activity for themselves, and then in deliberate retrospective analysis of their own learning and pro-spection of their teaching. We have adopted a combined theoretical perspective influenced by the enactivist position that “all knowing is doing” (Maturana and Varela 1998, p. 26) and Mason’s (2010, p. 32) assertion that “we do not usually learn from experience alone”. Rather, “real learning integrates experience and includes making sense of it” (Mason 2010, p. 33) through a process of retrospective analysis, whereby one steps back from the activity itself to retrospectively reflect on it. On this theoretical basis, we designed opportunities for preservice teachers to do mathematics for themselves, focusing on the generalisation of visual patterns. Then, in subsequent individual interviews, we prompted preservice teachers’ deliberate retrospective analysis of their own mathematical activity.

We have presented three themes of expressed awarenesses relating to the teaching and learning of mathematical reasoning with a focus on growing, visual patterns: awareness of “how you see” a pattern, awareness of the difficulty translating “what you see” in a pattern into a mathematical expression of generalisation, and awareness of the role that resources may play in helping learners to “see” the pattern.

Awareness of “how you see” a pattern was strongly embedded in preservice teachers’ noticing of what changes and what remains the same in the visual patterns when working towards identifying the general rule. In deliberate retrospective analysis of this approach to pattern generalisation and pro-spection of their teaching, preservice teachers articulated the importance of making distinctions between different elements of the visual pattern in order to establish meaningful relations between visual and symbolic representations.

In analysing task design and examining student activity in the mathematics classroom, Coles and Brown (2016) describe the action of comparing and contrasting what stays the same and what is different, as the making and naming of distinctions about mathematical objects. This leads learners to ask questions, notice patterns and to generalise. From an enactivist perspective, distinguishing and categorising is one of the basic mental functions and a key to learning. Brown and Coles (2012) argue that learning comes about through adapting to feedback from the distinctions learners make, in an ongoing process of coordinations of actions with the environment.

The value of making distinctions appears as a key learning feature in the current study, in preservice teachers’ deliberate retrospective analysis of their own mathematics activity and their prospective approach to teaching. In the previously presented examples from our sample of preservice teachers, we drew attention to points of reflection such as the following from Steve:

… it became about trying to understand, well you know how that was changing.

and to John’s retrospective sharing of his thinking:

… the first thing that I sort of was thinking about was the fact that they’ve all got four corners which stays the same

and also to subsequent lesson plans where the focus of teacher questioning was planned to be on “What is happening?”, “What is changing and what is staying the same?”. These illustrate traced links between our participants’ awareness of the importance of comparing and noticing what is the same and what is different in their own mathematics activity, and their awareness of the value of encouraging children to notice what is the same and different in their prospective planning of their teaching.

We identified examples that illustrate how deliberate retrospective analysis and articulation of their own mathematics actions and struggles can sensitise preservice teachers to the difficulties that their students may face in the classroom. This kind of sensitivity was demonstrated in preservice teachers’ anticipation of potential difficulties that their students may face and their planning for pedagogical action. On this basis, we argue that teacher education environments that encourage a process of prompted retro-spection of the preservice teachers’ own learning, and pro-spection of teaching, can support and enhance their ability to respond rather than react. To respond, according to Mason (2010 p. 37), is “to make an intentional, conscious, considered choice of action”, which he considers to be rare, as “we usually react”. This notion relates to creating stepping stones to continued learning from the enactivist perspective, for the purpose of enabling preservice teachers to maintain an “on-going alertness” and become “aware of their own awareness” (Brown and Coles 2012, p. 223), thus taking the middle path between the extremes of spontaneous, unreflective action and rational calculation and deliberateness that can characterise the actions of novices (Varela 1999).

One example of this in the current study, is Hayley’s expressed view that actually drawing a pattern, as she did when working on the patterns herself (retro-spection of own learning), would be a supportive strategy for the children in her class too (pro-spection of teaching). However, evaluation of her own teaching actions as part of a follow-up university session indicated a subsequent layer of awareness and sensitivity to her choice of materials, given the contrast between what she had initially anticipated as appropriate pedagogical choice of action (based on her own learning), and her students’ learning experience in the classroom.

they found drawing it harder because they had to start from the beginning … to draw, but when they had the cubes, that’s when they noticed, oh you just add one more to each side. (Hayley, U1).

Brown and Coles (2011, p. 862) point out that deliberate analysis “allows experts to unpick, if necessary, the reasons an action was taken, and hence open themselves up to alternative possibilities in the future”. They emphasise that “enactivism implies a commitment to the view that beginners can learn in this way too” citing Varela (1999) who argues that beginners can use this sort of deliberate analysis to bypass deliberateness and become expert.

The example of Hayley, above, is an illustration of how post hoc deliberation can provoke the beginning of a process enabling a novice, in this case, to interrogate the reasons an action was taken, and thus open herself up to “alternative possibilities” for future action (Brown and Coles 2011, p. 862). Hayley appears to move from “deliberateness”, when requiring children to draw the patterns, to an awareness that this is too prescriptive and does not necessarily benefit all learners. Such a move, also observed in other threads of retro-spection and pro-spection, indicates a shift towards becoming more sensitive to how children learn to generalise, and is seen as a process that, fostered within a teacher education environment that seeks to trigger such kinds of awarenesses, can enable preservice teachers to make more critical pedagogical choices by acting upon those awarenesses in their teaching.

The notion of “seeing” a pattern emerges strongly in our data and connects the three themes of expressed awarenesses that we have described and exemplified. This is consistent with the enactivist notion of embodied cognition, whereby “perception consists of perceptually guided action” (Varela 1999, p. 4), as well as previous evidence that has supported the importance of visualisation in pattern generalisation (e.g. Vale et al. 2018). When working with the patterns, many of the participants chose to use drawing, shading, colouring and physical resources to support their “seeing” of elements of the structure. Preservice teachers’ verbal reports of their drawing actions provided an interesting insight into action as a visible aspect of their “embodied (enacted) understandings” (Davis 1995, p. 4), both in cases where the visual structure of the pattern was discerned leading to generalisation (e.g. “without physically having to colour it, I don’t think I could be able to pull out differences”, Andrew, U1), as well as in cases of difficulty engaging with the visual elements of the pattern (e.g. “I started to draw it out myself, and then that sort of then closed down my thinking”, Alice, U2).

When encouraged to engage in deliberate retrospective analysis of their approaches to generalisation, preservice teachers articulated their own approaches with phrases such as: “the first way in which I saw this pattern”, “how I was viewing it” (Steve, U1), “what I had started doing is seeing it as…” (Elsa, U2). The use of “seeing”, “viewing”, “looked”, reflects preservice teachers’ prompted attention to figural structure. Importantly, it also indicates that the visual approach to generalisation, encouraged by the learning environment, became an individually and personally experienced embodied process (Samson and Schäfer 2011) and a process that differed, in many cases, from the embodied experience of their peers. Examples such as: “people saw this in different ways” (Hayley, U1), highlight such expressed awareness. In pro-spection of their teaching approach, the preservice teachers expressed it as awareness of the importance to acknowledge different interpretations of visual patterns in their classrooms (as indicated, for example, in Terry’s lesson plan in Fig. 9) and to allow learners the opportunity to share explanations of their processes of generalisation (Samson and Schäfer 2011).

Conclusion

This study focused on preservice elementary teachers’ deliberate retrospective analysis on their own processes and experiences of doing mathematics for themselves during university-based taught sessions. We explored the kinds of awarenesses that they articulated and the connections they then made to their future teaching, thereby tracing threads between retro-spection of learning and pro-spection of teaching. Our mathematical focus on generalisation of visual growing patterns proved to be an area which many found challenging. Our findings support our contention that attempting to generalise visual growing patterns can enable preservice teachers to sensitise themselves to students’ struggles. We argue that learning experiences that trigger preservice teachers’ awarenesses and sensitivity to their own, individual, and possibly differing, embodied processes of pattern generalisation need to constitute an important component of teacher education programmes, in order to prepare teachers who will be sensitive and astute to the individually embodied processes of knowing manifested by the learners in their classrooms.

We acknowledge some limitations of the study. Firstly, addressing only one area of mathematics was a pragmatic decision to enable depth and focus. From previous studies we were confident that a study of generalisation from sequences of visual patterns would be productive. It will be interesting and valuable to examine other key mathematical content domains and processes that pose challenges for novice teachers, and offer the potential to identify emerging awarenesses. Secondly, enhancing our data collection to capture preservice teachers’ mathematical embodied actions, their use of drawing, use of concrete materials and language in situ during university-based sessions could stimulate rich retrospective discussions. This could be a focus of future research.

Our findings have important implications for Initial Teacher Education (ITE). We argue that the role of universities in teacher education is to support the development of critical engagement and analytical perspectives, drawing on theory in order to develop practice. ITE contexts that integrate the learning of mathematics simultaneously with a focus on pedagogy offer a rich experience and context for reflection and the development of awarenesses. This can be achieved by providing opportunities for preservice teachers to engage in mathematics activities that have the potential to instigate critical incidents which challenge their thinking, in an environment where there is time to experiment, reason and collaborate with support and guidance from mathematics teacher educators. Above all, we argue that such experiences for preservice teachers enable the development of a disposition of an “on-going alertness to the detail of what is experienced” (Brown and Coles 2012, p. 223). An enactivist perspective can enable teacher educators to foreground deliberate retrospective analysis, so as to activate preservice teachers’ ability and sensitivity to effectively analyse and adapt their own learning and practice. We contend that nurturing these kinds of awarenesses will foster the growth of expertise in teaching mathematics.