Skip to main content
SpringerLink
Log in

Fractional Modeling of Fin on non-Fourier Heat Conduction via Modern Fractional Differential Operators

  • Research Article-Mechanical Engineering
  • Published:
Arabian Journal for Science and Engineering Aims and scope Submit manuscript

Abstract

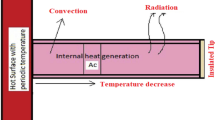
The enhancement of heat transfer for electronic kits and automobiles has become highly dependent on the finned heat exchangers; this is because fin provides high heat transfer rate and superior performance with a significant temperature reduction. In this manuscript, a fractional modeling of non-Fourier heat conduction problem of a fin is proposed within the periodic temperature boundary condition. The mathematical modeling is performed via classical theory of heat conduction that is directly proportional to temperature gradient through which hyperbolic heat conduction equation for a fin is generated. The hyperbolic heat conduction equation for a fin is fractionalized via modern approaches of fractional differentiations, namely Atangana–Baleanu and Caputo–Fabrizio differential operators. In order to have analyticity of hyperbolic heat conduction equation for a fin, we invoked the mathematical techniques of Laplace transform. The exact solutions of temperature distribution have been obtained in terms of Fox-H and Mittag–Leffler functions with the product of convolution. The solutions of temperature distribution have been classified into integer verses non-integer theories by making fractional parameters \( \alpha = \beta = 1 \) and \( \alpha \ne \beta \ne 1 \), respectively. Our results suggest that due to variability of different rheological parameters on temperature distribution, the cooling process is faster via fractional models in comparison to non-fractional model. Additionally, it is also observed that thermal wave propagates at a specific time results the reciprocal trend in temperature distribution.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Abbreviations

\( b \) :

Straight fin with uniform thickness

\( L \) :

Length

\( T_{0} \) :

Initial temperature

\( w \) :

Width

\( \omega \) :

Temperature oscillation frequency

\( A \) :

Input temperature amplitude

\( \alpha ,\beta \) :

Fractional parameters

\( T_{\text{b,m}} \) :

Mean base temperature

\( T_{\infty } \) :

Ambient temperature

\( T_{\text{b}} \left( {0,t} \right) \) :

Periodic base temperature

\( \tau \) :

Relaxation time

\( t \) :

Time

\( T\left( {y,t} \right) \) :

Temperature

\( k \) :

Thermal conductivity

\( \rho \) :

Density

\( C \) :

Specific heat capacity in a medium

\( \lambda_{1} \) :

Relaxation time

\( \lambda_{2} \) :

Relaxation time

\( \lambda_{3} \) :

Convective heat transfer

\( \theta \) :

Temperature distribution

\( \Omega \) :

Frequency of the temperature oscillation

\( A_{0} - A_{4} \) :

Letting parameters of Caputo–Fabrizio fractional operator

\( B_{0} - B_{4} \) :

Letting parameters of Atangana–Baleanu fractional operator

References

  1. Aziz, A.; Na, T.Y.: Periodic heat transfer in fins with variable thermal parameters. Int. J. Heat Mass Transf. 24, 1397–1404 (1981)

    Article  Google Scholar 

  2. Aziz, A.; Na, T.Y.: Perturbation analysis for periodic heat transfer in radiating fins. Wrmeund stoffübertragung 15(3), 245–253 (1981)

    Article  Google Scholar 

  3. Eslinger, R.G.; Chung, B.T.F.: Periodic heat transfer in radiating or convecting fins or fin arrays. Am. Inst. Aeronaut. Astronaut. J. 17(10), 1134–1140 (1979)

    Article  Google Scholar 

  4. Houghton, J.M.; Ingham, D.B.; Heggs, P.J.: The one dimensional analysis of oscillating heat transfer in a finned assembly. J. Heat Transf. 114, 546–552 (1992)

    Article  Google Scholar 

  5. Taler, D.: Transient inverse heat transfer problem in control of plate fin and tube heat exchangers. Arch. Thermodyn. 29(4), 185–194 (2008)

    Google Scholar 

  6. Ching-Yu, Y.: Estimation of the periodic thermal conditions on the non-Fourier fin problem. Int. J. Heat Mass Transf. 48, 3506–3515 (2005)

    Article  Google Scholar 

  7. Kundu, B.; Lee, K.S.: A non-Fourier analysis for transmitting heat in fins with internal heat generation. Int. J. Heat Mass Transf. 64, 1153–1162 (2013)

    Article  Google Scholar 

  8. Das, R.; Prasad, K.D.: Prediction of porosity and thermal diffusivity in a porous fin using differential evolution algorithm. Swarm Evol. Comput. 23, 27–39 (2015)

    Article  Google Scholar 

  9. Hatami, M.; Ganji, D.D.; Gorji-Bandpy, M.: Experimental and numerical analysis of the optimized finned tube heat exchanger for OM314 diesel exhaust recovery. Energy Conserv. Manag. 97, 26–41 (2015)

    Article  Google Scholar 

  10. Singh, S.; Kumar, D.; Rai, K.N.: Wavelet collocation solution of non-linear Fin problem with temperature dependent thermal conductivity and heat transfer coefficient. Int. J. Nonlinear Anal. Appl. 6(1), 105–118 (2015)

    MATH  Google Scholar 

  11. Singh, K.; Das, R.: Approximate analytical method for porous stepped fins with temperature-dependent heat transfer parameters. J. Thermophys. Heat Transf. 30(3), 661–672 (2016)

    Article  MathSciNet  Google Scholar 

  12. Jing, M.; Sun, Y.; Li, B.: Simulation of combined conductive, convective and radiative heat transfer in moving irregular porous fins by spectral element method. Int. J. Therm. Sci. 118, 475–487 (2017)

    Article  Google Scholar 

  13. Kashif, A.A.; Abdon, A.: Dual fractional modeling of rate type fluid through non-local differentiation. Numer. Methods Partial Differ. Equ. (2020). https://doi.org/10.1002/num.22633

    Article  Google Scholar 

  14. Khader, M.M.; Saad, K.M.: On the numerical evaluation for studying the fractional KdV, KdV–Burgers and Burgers equations. Eur. Phys. J. Plus 133(8), 3335 (2018)

    Article  Google Scholar 

  15. Kashif, A.A.; Ambreen, S.; Basma, S.; Abdon, A.: Application of statistical method on thermal resistance and conductance during magnetization of fractionalized free convection flow. Int. Commun. Heat Mass Transf. 119, 104971 (2020). https://doi.org/10.1016/j.icheatmasstransfer.2020.104971

    Article  Google Scholar 

  16. Koca, I.; Atangana, A.: Solutions of Cattaneo–Hristov model of elastic heat diffusion with Caputo–Fabrizio and Atangana–Baleanu fractional derivatives. Therm. Sci. (2017). https://doi.org/10.2298/TSCI160102102M

    Article  Google Scholar 

  17. Abro, K.A.; Mehwish, S.; Abdon, A.; Jose, F.G.A.: Thermophysical properties of Maxwell Nanofluids via fractional derivatives with regular kernel. J. Therm. Anal. Calorim. (2020). https://doi.org/10.1007/s10973-020-10287-9

    Article  Google Scholar 

  18. Abro, K.A.; Gomez-Aguilar, J.F.: A comparison of heat and mass transfer on a Walter’s-B fluid via Caputo–Fabrizio versus Atangana–Baleanu fractional derivatives using the Fox-H function. Eur. Phys. J. Plus 134, 101 (2019). https://doi.org/10.1140/epjp/i2019-12507-4

    Article  Google Scholar 

  19. Aziz, U.A.; Samia, R.; Samina, S.; Kashif, A.A.: Fractional modeling and synchronization of ferrofluid on free convection flow with magnetolysis. Eur. Phys. J. Plus 135, 841–855 (2020). https://doi.org/10.1140/epjp/s13360-020-00852-4

    Article  Google Scholar 

  20. Sheikh, N.A.; Ali, F.; Khan, I.; Gohar, M.; Saqib, M.: On the applications of nanofluids to enhance the performance of solar collectors: a comparative analysis of Atangana–Baleanu and Caputo–Fabrizio fractional models. Eur. Phys. J. Plus 132(12), 540 (2017)

    Article  Google Scholar 

  21. Kashif, A.A.: Role of fractal-fractional derivative on ferromagnetic fluid via fractal Laplace transform: a first problem via fractal–fractional differential operator. Eur. J. Mech. B Fluids 85, 76–81 (2021). https://doi.org/10.1016/j.euromechflu.2020.09.002

    Article  MathSciNet  Google Scholar 

  22. Owolabi, K.M.; Atangana, A.: Robustness of fractional difference schemes via the Caputo subdiffusion-reaction equations. Chaos Solitons Fractals 111, 119–127 (2018)

    Article  MathSciNet  Google Scholar 

  23. Qasim, A.; Samia, R.; Aziz, U.A.; Kashif, A.A.: Thermal investigation for electrified convection flow of Newtonian fluid subjected to damped thermal flux on a permeable medium. Phys. Scr. (2020). https://doi.org/10.1088/1402-4896/abbc2e

    Article  Google Scholar 

  24. Kashif, A.A.; Bhagwan, D.: A scientific report of non-singular techniques on microring resonators: an application to optical technology. Optik Int. J. Light Electron. Opt. 224, 165696 (2020). https://doi.org/10.1016/j.ijleo.2020.165696

    Article  Google Scholar 

  25. Gómez-Aguilar, J.F.; Atangana, A.; Morales-Delgado, V.F.: Electrical circuits RC, LC, and RL described by Atangana–Baleanu fractional derivatives. Int. J. Circ. Theory Appl. 45(11), 1514–1533 (2017)

    Article  Google Scholar 

  26. Abro, K.A.; Abdon, A.: Numerical and mathematical analysis of induction motor by means of AB–fractal–fractional differentiation actuated by drilling system. Numer. Methods Partial Differ. Equ. (2020). https://doi.org/10.1002/num.22618

    Article  Google Scholar 

  27. Abro, K.A.; Muzaffar, H.L.; Gomez-Aguilar, J.F.: Application of Atangana–Baleanu fractional derivative to carbon nanotubes based non-Newtonian nanofluid: applications in nanotechnology. J. Appl. Comput. Mech. 6(SI), 1260–1269 (2020). https://doi.org/10.22055/JACM.2020.33461.2229

    Article  Google Scholar 

  28. Kashif, A.A.; Atangana, A.: Porous effects on the fractional modeling of magnetohydrodynamic pulsatile flow: an analytic study via strong kernels. J. Therm. Anal. Calorim. (2020). https://doi.org/10.1007/s10973-020-10027-z

    Article  Google Scholar 

  29. Bhojraj, L.; Kashif, A.A.; Abdul, W.S.: Thermodynamical analysis of heat transfer of gravity-driven fluid flow via fractional treatment: an analytical study. J. Therm. Anal. Calorim. (2020). https://doi.org/10.1007/s10973-020-09429-w

    Article  Google Scholar 

  30. Abro, K.A.; Atangana, A.: A comparative study of convective fluid motion in rotating cavity via Atangana–Baleanu and Caputo–Fabrizio fractal–fractional differentiations. Eur. Phys. J. Plus 135, 226–242 (2020). https://doi.org/10.1140/epjp/s13360-020-00136-x

    Article  Google Scholar 

  31. Qureshi, S.; Yusuf, A.: Modeling chickenpox disease with fractional derivatives: from caputo to Atangana–Baleanu. Chaos Solitons Fractals 122, 111–118 (2019). https://doi.org/10.1016/j.chaos.2019.03.020

    Article  MathSciNet  MATH  Google Scholar 

  32. Kashif, A.A.; Ambreen, S.; Atangana, A.: Thermal stratification of rotational second-grade fluid through fractional differential operators. J. Therm. Anal. Calorim. (2020). https://doi.org/10.1007/s10973-020-09312-8

    Article  Google Scholar 

  33. Qureshi, S.; Abdon, A.: Mathematical analysis of dengue fever outbreak by novel fractional operators with field data. Physica A (2019). https://doi.org/10.1016/j.physa.2019.121127

    Article  MathSciNet  Google Scholar 

  34. Gómez-Aguilar, J.F.; Abro, K.A.; Olusola, K.; Ahmet, Y.: Chaos in a calcium oscillation model via Atangana–Baleanu operator with strong memory. Eur. Phys. J. Plus 134, 140 (2019). https://doi.org/10.1140/epjp/i2019-12550-1

    Article  Google Scholar 

  35. Abro, K.A.; Abdon, A.: Role of non-integer and integer order differentiations on the relaxation phenomena of viscoelastic fluid. Phys. Scr. 95, 035228 (2020). https://doi.org/10.1088/1402-4896/ab560c

    Article  Google Scholar 

  36. Kashif Ali Abro: Fractional characterization of fluid and synergistic effects of free convective flow in circular pipe through Hankel transform. Phys. Fluids 32, 123102 (2020). https://doi.org/10.1063/5.0029386

    Article  Google Scholar 

  37. Kashif, A.A.: A fractional and analytic investigation of thermo-diffusion process on free convection flow: an application to surface modification technology. Eur. Phys. J. Plus 135(1), 31–45 (2019). https://doi.org/10.1140/epjp/s13360-019-00046-7

    Article  Google Scholar 

  38. Singh, J.; Kumar, D.; Baleanu, D.: On the analysis of fractional diabetes model with exponential law. Adv. Differ. Equ. 2018(1), 1–13 (2018)

    Article  MathSciNet  Google Scholar 

  39. Kumar, D.; Singh, J.; Baleanu, D.: A new analysis of the Fornberg–Whitham equation pertaining to a fractional derivative with Mittag–Leffler-type kernel. Eur. Phys. J. Plus 133(2), 1–17 (2018)

    Article  Google Scholar 

  40. Caputo, M.; Fabrizio, M.A.: New definition of fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 1, 73–85 (2015)

    Google Scholar 

  41. Abro, K.A.; Abdon, A.: Mathematical analysis of memristor through fractal-fractional differential operators: a numerical study. Math. Methods Appl. Sci. (2020). https://doi.org/10.1002/mma.6378

    Article  MathSciNet  MATH  Google Scholar 

  42. Kashif, A.A.; Atangana, A.: Numerical study and chaotic analysis of meminductor and memcapacitor through fractal-fractional differential operator. Arab. J. Sci. Eng. (2020). https://doi.org/10.1007/s13369-020-04780-4

    Article  Google Scholar 

  43. Atangana, A.; Baleanu, D.: New fractional derivative with non local and non-singular kernel: theory and application to heat transfer model. Therm. Sci. 20, 763–769 (2016)

    Article  Google Scholar 

  44. Kashif, A.A.; Atangana, A.: A comparative analysis of electromechanical model of piezoelectric actuator through Caputo–Fabrizio and Atangana–Baleanu fractional derivatives. Math. Methods Appl. Sci. (2020). https://doi.org/10.1002/mma.6638

    Article  MathSciNet  Google Scholar 

  45. Abro, K.A.; Jose, F.G.A.: Role of Fourier sine transform on the dynamical model of tensioned carbon nanotubes with fractional operator. Math. Methods Appl. Sci. (2020). https://doi.org/10.1002/mma.6655

    Article  Google Scholar 

  46. Al-khafaji, O.R.; Alabbas, A.H.: Computational fluid dynamics modeling study for the thermal performance of the pin fins under different parameters. In: IOP Conference Series: Materials Science and Engineering, vol. 745, pp. 012070. https://doi.org/10.1088/1757-899x/745/1/012070

  47. Asıf, Y.; Hulya, D.; Kashif, A.A.; Dogan, K.: Role of Gilson-Pickering equation for the different types of soliton solutions: a nonlinear analysis. Eur. Phys. J. Plus 135, 657 (2020). https://doi.org/10.1140/epjp/s13360-020-00646-8

    Article  Google Scholar 

  48. Aziz, U.A.; Muhammad, T.; Abro, K.A.: Multiple soliton solutions with chiral nonlinear Schrödinger’s equation in (2 + 1)-dimensions. Eur. J. Mech. B. Fluids (2020). https://doi.org/10.1016/j.euromechflu.2020.07.014

    Article  Google Scholar 

  49. Aziz, U.A.; Mukarram, A.; Abro, K.A.: Electroosmotic slip flow of Oldroyd-B fluid between two plates with non-singular kernel. J. Comput. Appl. Math. 376, 112885–112899 (2020). https://doi.org/10.1016/j.cam.2020.112885

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The author Kashif Ali Abro is highly thankful and grateful to Mehran university of Engineering and Technology, Jamshoro, Pakistan, for generous support and facilities of this research work.

Author information

Authors and Affiliations

  1. Department of Basic Sciences and Related Studies, Mehran University of Engineering and Technology, Jamshoro, Pakistan

    Kashif Ali Abro

  2. Departamento de Ingeniería Electrónica, CONACyT-Tecnológico Nacional de México/CENIDET, Interior Internado Palmira S/N, Col. Palmira, C.P. 62490, Cuernavaca, Morelos, Mexico

    Jose Francisco Gomez-Aguilar

Authors
  1. Kashif Ali Abro
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jose Francisco Gomez-Aguilar
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Kashif Ali Abro.

Ethics declarations

Conflict of interest

The authors declare no conflict of interest.

Appendix

Appendix

$$ {\mathcal{L}}^{ - 1} \left( {\frac{1}{{\left( {s^{\alpha } - \beta } \right)}}} \right) = t^{\alpha - 1} {\mathbf{E}}_{\alpha ,\alpha } \left( { - \beta t^{\alpha } } \right), $$
(A1)
$$ {\mathcal{L}}^{ - 1} \left( {\frac{{s^{\alpha } }}{{s\left( {s^{\alpha } + \beta } \right)}}} \right) = {\mathbf{E}}_{\alpha } \left( { - \beta t^{\alpha } } \right), $$
(A2)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Abro, K.A., Gomez-Aguilar, J.F. Fractional Modeling of Fin on non-Fourier Heat Conduction via Modern Fractional Differential Operators. Arab J Sci Eng 46, 2901–2910 (2021). https://doi.org/10.1007/s13369-020-05243-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s13369-020-05243-6

Keywords

Search

Navigation