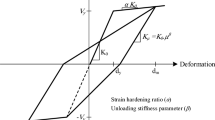
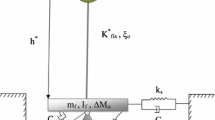
Abstract
This study aims to consider the effect of soil–structure interaction (SSI) on the ductility and hysteretic energy demands of superstructures and propose empirical equations for demand prediction in soil–structure systems. To this end, the FEMA 440 procedure was considered to develop nonlinear single-degree-of-freedom oscillators with a period range of 0.1–3.0 s, as the representative of superstructures. The elastic-perfectly plastic and a moderate pinching degrading hysteretic models were considered for the nonlinear response of the superstructure. The model of the nonlinear soil–foundation system was developed through the Winkler method. In this regard, the type of soil beneath the foundation was assumed as D category, according to the site classification in ASCE 7-10. A wide range of key parameters, including the strength reduction factors (2 ≤ Rμ ≤ 8), the foundation safety factor (3 ≤ SF ≤ 7), the foundation-to-structure height aspect ratio (1 ≤ h/b ≤ 5), and the foundation length-to-width ratio (3 ≤ Lf/Bf ≤ 20) was introduced into the analytical models to conduct parametric studies. Results show the considerable effect of SSI on reducing the ductility and hysteretic energy demands in superstructures with short fundamental periods. More demand reduction can be achieved by providing the lateral sliding of the foundation on the soil surface, especially for systems with a small aspect ratio. The pinching–degrading hysteretic behavior of the superstructure remarkably modifies the level of demands. Moreover, predictive models were proposed for estimating the ductility and hysteretic energy demands in flexible base systems. These models modify demands in the rigid base structures based on their physical and mechanical properties. The developed models consider the effects of structural hysteretic behavior as well as foundation flexibility. The efficiency of the proposed model was assessed on a multi-story frame. Finally, the required ductility capacity of the systems was determined through the Park–Ang damage index and by using the developed predictive models. Results show the efficiency of the empirical models to reasonably estimate the required ductility capacity.




























Similar content being viewed by others
References
Agency FEM (2003) NEHRP recommended provisions for seismic regulations for new buildings and other structures. Fema
Agency FEM (2005) Improvement of nonlinear static seismic analysis procedures. In FEMA 440. Applied Technology Council (ATC-55 Project)
Akbas B (2006) A neural network model to assess the hysteretic energy demand in steel moment resisting frames. Struct Eng Mech 23(2):177–193
Alhaddad MS, Wazira KM, Al-Salloum YA, Abbas H (2015) Ductility damage indices based on seismic performance of RC frames. Soil Dyn Earthq Eng 77:226–237. https://doi.org/10.1016/j.soildyn.2015.05.014
American Society of Civil Engineers (2017a) Seismic evaluation and retrofit of existing buildings. American Society of Civil Engineers, Reston
American Society of Civil Engineers (2017b) Minimum Design Loads and Associated Criteria for Buildings and Other Structures, ASCE/SEI 7-16. American Society of Civil Engineers (ASCE), Reston, VA
Anastasopoulos I, Kourkoulis R, Gelagoti F, Papadopoulos E (2012) Rocking response of SDOF systems on shallow improved sand: an experimental study. Soil Dyn Earthq Eng 40:15–33. https://doi.org/10.1016/j.soildyn.2012.04.006
Arroyo D, Ordaz M (2007a) On the estimation of hysteretic energy demands for SDOF systems. Earthq Eng Struct Dyn 36(15):2365–2382. https://doi.org/10.1002/eqe.736
Arroyo D, Ordaz M (2007b) Hysteretic energy demands for SDOF systems subjected to narrow band earthquake ground motions. Applications to the lake bed zone of Mexico City. J Earthq Eng 11(2):147–165. https://doi.org/10.1080/13632460601123131
ASCE, SEI7 (2010) Minimum design loads for buildings and other structures. ASCE, Reston
Avilés J, Pérez-Rocha Luis E (2005) Influence of foundation flexibility on Rμ and Cμ factors. J Struct Eng 131(2):221–230. https://doi.org/10.1061/(ASCE)0733-9445(2005)131:2(221)
Baéz JI, Miranda E (2000) Amplification factors to estimate inelastic displacement demands for the design of structures in the near field. In: Proceedings of the 12th world conference on earthquake engineering, Auckland, New Zealand
Bararnia M, Hassani N, Ganjavi B, Ghodrati Amiri G (2018) Estimation of inelastic displacement ratios for soil–structure systems with embedded foundation considering kinematic and inertial interaction effects. Eng Struct 159:252–264. https://doi.org/10.1016/j.engstruct.2018.01.002
Benazouz C, Moussa L, Ali Z (2012) Ductility and inelastic deformation demands of structures. Struct Eng Mech 42(5):631–644
Bertero VV, Mahin SA, Herrera RA (1978) Aseismic design implications of near-fault san fernando earthquake records. Earthq Eng Struct Dyn 6(1):31–42. https://doi.org/10.1002/eqe.4290060105
Bolisetti C, Whittaker AS, Coleman JL (2018) Linear and nonlinear soil–structure interaction analysis of buildings and safety-related nuclear structures. Soil Dyn Earthq Eng 107:218–233. https://doi.org/10.1016/j.soildyn.2018.01.026
Boulanger R (2000) The PySimple1, TzSimple1, and QzSimple1 material models, documentation for the OpenSees platform. http://opensees.berkeley.edu
Boulanger Ross W, Curras Christina J, Kutter Bruce L, Wilson Daniel W, Abghari A (1999) Seismic soil–pile–structure interaction experiments and analyses. J Geotech Geoenviron Eng 125(9):750–759. https://doi.org/10.1061/(ASCE)1090-0241(1999)125:9(750)
Centre B.a.H.R. (2014) BHRC; Iranian code of practice for seismic resistant design of buildings (Standard 2800)
Chikh B, Laouami N, Mebarki A, Leblouba M, Mehani Y, Kibboua A, Hadid M, Benouar D (2017) Seismic structural demands and inelastic deformation ratios: sensitivity analysis and simplified models. Earthq Struct 13(1):59–66
Chopra AK (1995) Dynamics of structures: theory and applications to earthquake engineering. Prentice Hall, Upper Saddle River
Chopra AK (2007) Earthquake dynamics of base-isolated buildings. In: Dynamics of structures: theory and applications to earthquake engineering, 3rd ed. Pearson Prentice Hall, Upper Saddle River
Chopra Anil K, Goel Rakesh K (2000) Evaluation of NSP to estimate seismic deformation: SDF systems. J Struct Eng 126(4):482–490. https://doi.org/10.1061/(ASCE)0733-9445(2000)126:4(482)
Chopra AK, Goel RK (1999) Capacity–demand–diagram methods based on inelastic design spectrum. Earthq Spectra 15(4):637–656. https://doi.org/10.1193/1.1586065
Code I.o.B.N. (2014) IBNC; Iranian National Building Code, Part 10-Steel Design
Fairhurst M, Bebamzadeh A, Ventura CE (2019) Effect of ground motion duration on reinforced concrete shear wall buildings. Earthq Spectra 35(1):311–331. https://doi.org/10.1193/101117EQS201M
Fajfar P, Vidic T (1994) Consistent inelastic design spectra: Hysteretic and input energy. Earthq Eng Struct Dyn 23(5):523–537. https://doi.org/10.1002/eqe.4290230505
Fatahi B, Tabatabaiefar SHR (2014) Fully nonlinear versus equivalent linear computation method for seismic analysis of midrise buildings on soft soils. Int J Geomech 14(4):04014016. https://doi.org/10.1061/(ASCE)GM.1943-5622.0000354
FEMA-440 (2005) Improvement of nonlinear static seismic analysis procedures. Applied Technology Council (ATC-55 Project)
Gajan S, Raychowdhury P, Hutchinson TC, Kutter BL, Stewart JP (2010) Application and validation of practical tools for nonlinear soil–foundation interaction analysis. Earthq Spectra 26(1):111–129. https://doi.org/10.1193/1.3263242
Ganjavi B, Hao H (2012a) A parametric study on the evaluation of ductility demand distribution in multi-degree-of-freedom systems considering soil–structure interaction effects. Eng Struct 43:88–104. https://doi.org/10.1016/j.engstruct.2012.05.006
Ganjavi B, Hao H (2012b) Effect of structural characteristics distribution on strength demand and ductility reduction factor of MDOF systems considering soil–structure interaction. Earthq Eng Eng Vibr 11(2):205–220. https://doi.org/10.1007/s11803-012-0111-7
Gazetas G (1991) Formulas and charts for impedances of surface and embedded foundations. J Geotech Eng 117(9):1363–1381. https://doi.org/10.1061/(ASCE)0733-9410(1991)117:9(1363)
Gazetas G, Anastasopoulos I, Adamidis O, Kontoroupi T (2013) Nonlinear rocking stiffness of foundations. Soil Dyn Earthq Eng 47:83–91. https://doi.org/10.1016/j.soildyn.2012.12.011
Ghandil M, Behnamfar F (2017) Ductility demands of MRF structures on soft soils considering soil–structure interaction. Soil Dyn Earthq Eng 92:203–214. https://doi.org/10.1016/j.soildyn.2016.09.051
Ghannad MA (1998) A study on the effect of soil–structure interaction on the dynamic properties of structures using simplified methods. 名古屋大学
Ghannad MA, Jafarieh AH (2014) Inelastic displacement ratios for soil–structure systems allowed to uplift. Earthq Eng Struct Dyn 43(9):1401–1421. https://doi.org/10.1002/eqe.2405
Goda K, Hong HP, Lee CS (2009) Probabilistic characteristics of seismic ductility demand of SDOF systems with Bouc–Wen hysteretic behavior. J Earthq Eng 13(5):600–622. https://doi.org/10.1080/13632460802645098
Gulkan P, Sozen MA (1974) Inelastic responses of reinforced concrete structure to earthquake motions, pp 604–610
Harden CW (2005) Numerical modeling of the nonlinear cyclic response of shallow foundations. Pacific Earthquake Engineering Research Center
Harden C, Hutchinson T, Moore M (2006) Investigation into the effects of foundation uplift on simplified seismic design procedures. Earthq Spectra 22(3):663–692. https://doi.org/10.1193/1.2217757
Hassani N, Bararnia M, Ghodrati Amiri G (2018) Effect of soil–structure interaction on inelastic displacement ratios of degrading structures. Soil Dyn Earthq Eng 104:75–87. https://doi.org/10.1016/j.soildyn.2017.10.004
Hatzigeorgiou GD (2010) Ductility demand spectra for multiple near- and far-fault earthquakes. Soil Dyn Earthq Eng 30(4):170–183. https://doi.org/10.1016/j.soildyn.2009.10.003
Homaei F (2020) Investigating the effects of inelastic soil–foundation interface response on the seismic demand of soil–structure systems. Can J Civ Eng. https://doi.org/10.1139/cjce-2020-0059
Homaei F, Yazdani M (2020) The probabilistic seismic assessment of aged concrete arch bridges: The role of soil–structure interaction. Structures 28:894–904. https://doi.org/10.1016/j.istruc.2020.09.038
Hou H, Qu B (2015) Duration effect of spectrally matched ground motions on seismic demands of elastic perfectly plastic SDOFS. Eng Struct 90:48–60. https://doi.org/10.1016/j.engstruct.2015.02.013
Iwan WD (1980) Estimating inelastic response spectra from elastic spectra. Earthquake Eng Struct Dynam 8(4):375–388. https://doi.org/10.1002/eqe.4290080407
Khashaee P (2005) Damage-based seismic design of structures. Earthq Spectra 21(2):371–387. https://doi.org/10.1193/1.1896366
Kim Y-S, Roesset Jose M (2004) Effect of nonlinear soil behavior on inelastic seismic response of a structure. Int J Geomech 4(2):104–114. https://doi.org/10.1061/(ASCE)1532-3641(2004)4:2(104)
Krawinkler H, Nassar AA (1992) Seismic design based on ductility and cumulative damage demands and capacities. In: Nonlinear seismic analysis and design of reinforced concrete buildings. CRC Press, Boca Raton, pp 31–48
Lee D-G, Song J-K, Yun C-B (1997) Estimation of system-level ductility demands for multi-story structures. Eng Struct 19(12):1025–1035. https://doi.org/10.1016/S0141-0296(97)00010-2
Li H, Yi T, Gu M, Huo L (2009) Evaluation of earthquake-induced structural damages by wavelet transform. Prog Nat Sci 19(4):461–470. https://doi.org/10.1016/j.pnsc.2008.09.002
Lignos Dimitrios G, Krawinkler H (2011) Deterioration modeling of steel components in support of collapse prediction of steel moment frames under earthquake loading. J Struct Eng 137(11):1291–1302. https://doi.org/10.1061/(ASCE)ST.1943-541X.0000376
Lou M, Wang H, Chen X, Zhai Y (2011) Structure–soil–structure interaction: literature review. Soil Dyn Earthq Eng 31(12):1724–1731. https://doi.org/10.1016/j.soildyn.2011.07.008
Maheshwari BK, Sarkar R (2011) Seismic behavior of soil–pile–structure interaction in liquefiable soils: parametric study. Int J Geomech 11(4):335–347. https://doi.org/10.1061/(ASCE)GM.1943-5622.0000087
Marano GC, Greco R (2006) Damage and ductility demand spectra assessment of hysteretic degrading systems subject to stochastic seismic loads. J Earthq Eng 10(05):615–640. https://doi.org/10.1142/S1363246906002785
Masaeli H, Khoshnoudian F, Musician S (2018) Incremental dynamic analysis of nonlinear rocking soil–structure systems. Soil Dyn Earthq Eng 104:236–249. https://doi.org/10.1016/j.soildyn.2017.09.013
Mashhadi S, Asadi A, Homaei F, Tajammolian H (2020) The performance-based seismic response of special steel MRF: Effects of pulse-like ground motion and foundation safety factor. Structures 28:127–140. https://doi.org/10.1016/j.istruc.2020.08.040
McKenna F, Fenves GL, Scott MH (2000) Open system for earthquake engineering simulation. In University of California, Berkeley
Miranda E (2000) Inelastic displacement ratios for structures on firm sites. J Struct Eng 126(10):1150–1159. https://doi.org/10.1061/(ASCE)0733-9445(2000)126:10(1150)
Miranda E (2001) Estimation of inelastic deformation demands of SDOF systems. J Struct Eng 127(9):1005–1012. https://doi.org/10.1061/(ASCE)0733-9445(2001)127:9(1005)
Molazadeh M, Saffari H (2018) The effects of ground motion duration and pinching–degrading behavior on seismic response of SDOF systems. Soil Dyn Earthq Eng 114:333–347. https://doi.org/10.1016/j.soildyn.2018.06.032
Nakhaei M, Ghannad MA (2006) The effect of soil–structure interaction on hysteretic energy demand of buildings. Struct Eng Mech 24(5):641–645
National, I.o.B., and Code (2014) IBNC; Iranian national building code, Part 7—foundation design
Neethu B, Das D, Garia S (2017) Effects of ground motion frequency content on performance of isolated bridges with SSI. Earthq Struct 13(4):353–363. https://doi.org/10.12989/eas.2017.13.4.353
Ning C-L, Cheng Y, Yu X-H (2019) A simplified approach to investigate the seismic ductility demand of shear-critical reinforced concrete columns based on experimental calibration. J Earthq Eng. https://doi.org/10.1080/13632469.2019.1605949
Palanci M, Senel SM (2019) Correlation of earthquake intensity measures and spectral displacement demands in building type structures. Soil Dyn Earthq Eng 121:306–326. https://doi.org/10.1016/j.soildyn.2019.03.023
Pan Y, Ventura Carlos E, Liam Finn WD (2018) Effects of ground motion duration on the seismic performance and collapse rate of light-frame wood houses. J Struct Eng 144(8):04018112. https://doi.org/10.1061/(ASCE)ST.1943-541X.0002104
Park YJ, Ang Alfredo HS (1985) Mechanistic seismic damage model for reinforced concrete. J Struct Eng 111(4):722–739. https://doi.org/10.1061/(ASCE)0733-9445(1985)111:4(722)
Park YJ, Ang Alfredo HS, Wen Yi K (1985) Seismic damage analysis of reinforced concrete buildings. J Struct Eng 111(4):740–757. https://doi.org/10.1061/(ASCE)0733-9445(1985)111:4(740)
Park YJ, Ang AHS, Wen YK (1987) Damage-limiting aseismic design of buildings. Earthq Spectra 3(1):1–26. https://doi.org/10.1193/1.1585416
Prasanth T, Ghosh S, Collins KR (2008) Estimation of hysteretic energy demand using concepts of modal pushover analysis. Earthq Eng Struct Dyn 37(6):975–990. https://doi.org/10.1002/eqe.802
Pu W, Wu M (2018) Ductility demands and residual displacements of pinching hysteretic timber structures subjected to seismic sequences. Soil Dyn Earthq Eng 114:392–403. https://doi.org/10.1016/j.soildyn.2018.07.037
Raychowdhury P, Hutchinson T (2008) Nonlinear material models for winkler-based shallow foundation response evaluation. In: GeoCongress 2008, New Orleans, Louisiana, United States, pp 686–693
Raychowdhury P, Hutchinson TC (2009) Performance evaluation of a nonlinear Winkler-based shallow foundation model using centrifuge test results. Earthq Eng Struct Dyn 38(5):679–698. https://doi.org/10.1002/eqe.902
Rosenblueth E, Herrera I (1964) On a kind of hysteretic damping. J Eng Mech Div 90(4):37–48
Ruiz-García J, Miranda E (2003) Inelastic displacement ratios for evaluation of existing structures. Earthq Eng Struct Dyn 32(8):1237–1258. https://doi.org/10.1002/eqe.271
Ruiz-García J, Miranda E (2004) Inelastic displacement ratios for design of structures on soft soils sites. J Struct Eng 130(12):2051–2061. https://doi.org/10.1061/(ASCE)0733-9445(2004)130:12(2051)
Samimifar M, Massumi A, Moghadam AS (2019) A new practical equivalent linear model for estimating seismic hysteretic energy demand of bilinear systems. Struct Eng Mech 70(3):289–301
Sen N, Gupta VK (2018) On estimation of seismic damage from ductility and hysteretic energy demands in equivalent oscillators using linear response. Eng Struct 172:663–686. https://doi.org/10.1016/j.engstruct.2018.04.096
Shakib H, Homaei F (2017) Probabilistic seismic performance assessment of the soil–structure interaction effect on seismic response of mid-rise setback steel buildings. Bull Earthq Eng 15(7):2827–2851. https://doi.org/10.1007/s10518-017-0087-9
Sharifi A, Banan M-R, Banan M-R (2012) A strain-consistent approach for determination of bounds of ductility damage index for different performance levels for seismic design of RC frame members. Eng Struct 37:143–151. https://doi.org/10.1016/j.engstruct.2011.12.025
Shin M, Kim B (2017) Effects of frequency contents of aftershock ground motions on reinforced concrete (RC) bridge columns. Soil Dyn Earthq Eng 97:48–59. https://doi.org/10.1016/j.soildyn.2017.02.012
Sivaselvan Mettupalayam V, Reinhorn Andrei M (2000) Hysteretic models for deteriorating inelastic structures. J Eng Mech 126(6):633–640. https://doi.org/10.1061/(ASCE)0733-9399(2000)126:6(633)
Smith-Pardo JP (2011) Performance-based framework for soil–structure systems using simplified rocking foundation models. Struct Eng Mech 40(6):763–782
Terzaghi K (1943) Theoretical soil mechanics. Wiley, New York
van de Lindt JW, Goh GH (2004) Effect of earthquake duration on structural reliability. Eng Struct 26(11):1585–1597. https://doi.org/10.1016/j.engstruct.2004.05.017
van de Lindt John W, Goh G (2004) Earthquake duration effect on structural reliability. J Struct Eng 130(5):821–826. https://doi.org/10.1061/(ASCE)0733-9445(2004)130:5(821)
Veletsos AS (1977) Dynamics of structure–foundation systems. In: Structural and geotechnical mechanics, pp 333–361
Wang C-H, Foliente Greg C, Sivaselvan Mettupalayam V, Reinhorn Andrei M (2001) Hysteretic models for deteriorating inelastic structures. J Eng Mech 127(11):1200–1202. https://doi.org/10.1061/(ASCE)0733-9399(2001)127:11(1200)
Wang F, Ke K, Zhang H, Yam MCH (2018) Constant-ductility-based energy factor demands of oscillators with modified Clough hysteretic model. Soil Dyn Earthq Eng 115:36–40. https://doi.org/10.1016/j.soildyn.2018.08.014
Yazdani A, Yazdannejad K (2019) Estimation of the seismic demand model for different damage levels. Eng Struct 194:183–195. https://doi.org/10.1016/j.engstruct.2019.05.071
Yi W-J, Zhang H-Y, Kunnath Sashi K (2007) Probabilistic constant-strength ductility demand spectra. J Struct Eng 133(4):567–575. https://doi.org/10.1061/(ASCE)0733-9445(2007)133:4(567)
Yi T-H, Li H-N, Sun H-M (2013) Multi-stage structural damage diagnosis method based on. Smart Struct Syst 12(3_4):345–361
Yue J, Qian J, Beskos DE (2019) Seismic damage performance levels for concrete encased steel columns using acoustic emission tests and finite element analysis. Eng Struct 189:471–483. https://doi.org/10.1016/j.engstruct.2019.03.077
Zampieri P, Zanini MA, Faleschini F (2016) Influence of damage on the seismic failure analysis of masonry arches. Constr Build Mater 119:343–355. https://doi.org/10.1016/j.conbuildmat.2016.05.024
Zhou Y, Song G, Tan P (2019) Hysteretic energy demand for self-centering SDOF systems. Soil Dyn Earthq Eng 125:105703. https://doi.org/10.1016/j.soildyn.2019.105703
Acknowledgements
The author would like to acknowledge the financial support of the Institute of Science and High Technology and Environmental Sciences, Graduate University of Advanced Technology, Kerman, Iran, under grant number of 98.314.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix
Appendix
This appendix deals with details for computing the predicted values of EN and μm from the proposed formulation. First, considering the studied structure in this research (see Sect. 6), in which, the fundamental natural vibration period of the structure is Tfix = 2.0 s.
-
1.
Based on Eqs. (27) and (28), the regression factors of a0, a1, a2, b0, b1, b2 for an EPP model is obtained as:
$$\left\{ \begin{aligned} a_{0} = \text{e}^{{\left( {0.9324\ln \left( {7.5} \right) - 0.0192} \right)}} = 6.42 \hfill \\ a_{1} = \text{e}^{{\left( {0.6101\left( {7.5} \right) - 4.3851} \right)}} = 1.21 \hfill \\ a_{2} = 2.037 \hfill \\ \end{aligned} \right.\,\,\,\quad \,\,\,\left\{ \begin{aligned} b_{0} = \text{e}^{{\left( {1.7064\ln \left( {7.5} \right) + 0.5631} \right)}} = 54.67 \hfill \\ b_{1} = \text{e}^{{\left( {2.0578\ln \left( {7.5} \right) - 2.9348} \right)}} = 3.36 \hfill \\ b_{2} = 2.6073 \hfill \\ \end{aligned} \right.$$(47) -
2.
Substituting the regression factors of a0, a1, a2, b0, b1, b2 into Eqs. (25) and (26):
$$\mu_{{m\,\left( {fix} \right)}} = 6.42 + \frac{1.21}{{\left( {2.0} \right)^{2.037} }} = 6.71\quad E_{{N\,\left( {fix} \right)}} = 54.67 + \frac{3.36}{{\left( {2.0} \right)^{2.6073} }} = 55.22$$(48)
For the SSI model, since the fundamental natural vibration period of the structure is Tfix = 2.0 s, the 2nd part of Eqs. (30) and (31) were used for computing the predicted values of EN(SSI) and μm(SSI). In this regard:
-
3.
Equations (32) and (33) are essential for computing the regression constants of m1, n1, m2, and n2 by considering the Pijs factors from Tables 4 and 5. For example, considering a non-sliding model of foundation, the regression factors of m1, n1, m2, and n2 are obtained as:
$$\begin{aligned} m_{1} & = 1.41 - 0.3185\left( {2.56} \right) + 0.2808\left( 3 \right) - 0.01144\left( {2.56} \right)^{2} + 0.06539\left( {2.56} \right)\left( 3 \right) \hfill \\ & \quad - 0.03667\left( 3 \right)^{2} = 1.534 \hfill \\ n_{1} & = 1.831 + 0.1117\left( {2.56} \right) - 0.08769\left( 3 \right) + 0.003761\left( {2.56} \right)^{2} - 0.02298\left( {2.56} \right)\left( 3 \right) \hfill \\ \quad + 0.01183\left( 3 \right)^{2} = 1.8085 \hfill \\ \end{aligned}$$(49)$$\begin{aligned} m_{2} &= 1.012 - 0.1098\left( {2.56} \right) + 0.03543\left( 3 \right) - 0.0112\left( {2.56} \right)^{2} + 0.02847\left( {2.56} \right)\left( 3 \right) \hfill \\ & \quad - 0.00757\left( 3 \right)^{2} = 0.9143 \hfill \\ n_{2} & = 0.9676 + 0.04591\left( {2.56} \right) - 0.00337\left( 3 \right) + 0.009683\left( {2.56} \right)^{2} - 0.01489\left( {2.56} \right)\left( 3 \right) \hfill \\ & \quad + 0.002444\left( 3 \right)^{2} = 1.046 \hfill \\ \end{aligned}$$(50) -
4.
Substituting the regression factors of m1, n1, m2, and n2 into the 2nd part of Eqs. (30) and (31), the predicted values of EN(SSI) and μm(SSI) for the SSI model is obtained as:
$$E_{{N\,\left( {SSI} \right)}} = 1.534\left( {7.5} \right)^{1.8085} = 58.66\,\quad \mu_{{m\,\left( {SSI} \right)}} = 0.9143\left( {7.5} \right)^{1.046} = 7.523$$(51)
Now assuming another structure with a sliding foundation (SLD) and Tfix = 1.5 s, Rµ = 4, h/b = 2, SF = 3, and a PD model; the following steps are used for predicting EN and μm:
-
1.
Computing the regression factors of a0, a1, a2, b0, b1, b2 from Eqs. (27) and (28) for a PD model:
$$\left\{ \begin{aligned} a_{0} = \text{e}^{{\left( {0.976\ln \left( 4 \right) - 0.0318} \right)}} = 3.748 \hfill \\ a_{1} = \text{e}^{{\left( {1.7336\ln \left( 4 \right) - 3.8084} \right)}} = 0.245 \hfill \\ a_{2} = 2.146 \hfill \\ \end{aligned} \right.\,\quad \,\,\left\{ \begin{aligned} b_{0} = \text{e}^{{\left( {1.6411\ln \left( 4 \right) + 0.3537} \right)}} = 13.856 \hfill \\ b_{1} = \text{e}^{{\left( {2.0493\ln \left( 4 \right) - 3.1421} \right)}} = 0.740 \hfill \\ b_{2} = 2.7761 \hfill \\ \end{aligned} \right.$$(52) -
2.
Computing EN(fix) and μm(fix) for the rigid-based structure by substituting the regression factors of a0, a1, a2, b0, b1, b2 into Eqs. (25) and (26):
$$\mu_{{m\,\left( {fix} \right)}} = 3.748 + \frac{0.245}{{\left( {1.5} \right)^{2.146} }} = 3.85\,\quad E_{{N\,\left( {fix} \right)}} = 13.856 + \frac{0.74}{{\left( {1.5} \right)^{2.7761} }} = 14.096\,$$(53)
For the SSI model, the 1st part of Eqs. (30) and (31) is used for computing the predicted values of EN(fix) and μm(fix). In this case:
-
3.
The regression constants ei, fi, gi, and hi are computed using the polynomial function of degree 3 in Eq. (38) through regression factors in Table 7 for EN and Table 9 for μm, which yields: e1 = -0.1234, f1 = 1.5592, g1 = -0.1052, h1 = 2.5189, e2 = -0.17988, f2 = 1.1796, g2 = 0.1684, h2 = 2.1. The computation details are presented for one of the coefficients (say e1):
$$\begin{aligned} e_{1} & = - 0.4968 + 1.47\left( {\frac{2}{4}} \right) + 0.2119\left( 4 \right) - 2.386\left( {\frac{2}{4}} \right)^{2} - 0.1799\left( 4 \right)\left( {\frac{2}{4}} \right) \\ & \quad - 0.03523\left( 4 \right)^{2} + 0.5958\left( {\frac{2}{4}} \right)^{3} \, + 0.1747\left( {\frac{2}{4}} \right)^{2} \left( 4 \right) - 0.01014\left( {\frac{2}{4}} \right)\left( 4 \right)^{2} \\ & \quad + 0.00223\left( 4 \right)^{3} = - 0.1234 \\ \end{aligned}$$(54) -
4.
Computing the regression factors of ci and di through Eqs. (36) and (37):
$$\begin{aligned} c_{1} & = \exp \left[ { - 0.1234\left( 3 \right) + 1.5592} \right] = 3.284 \\ d_{1} & = - \exp \left[ { - 0.1052\left( 3 \right) + 2.5189} \right] = - 9.055 \\ c_{2} & = \exp \left[ { - 0.17988\left( 3 \right) + 1.1796} \right] = 1.8964 \\ d_{2} & = - \exp \left[ { - 0.1684\left( 3 \right) + 2.10} \right] = - 4.93 \\ \end{aligned}$$(55) -
5.
Now substituting these factors into the 1st part of Eqs. (30) and (31) yields to:
$$\begin{aligned} c_{1} T_{fix} + d_{1} & = 3.284\left( {1.5} \right) - 9.055 = - 4.129 \\ c_{2} T_{fix} + d_{2} & = 1.8964\left( {1.5} \right) - 4.93 = - 2.085 \\ \end{aligned}$$(56)
On the other hand, from Fig. 15, the mean elastic acceleration response spectrum of the ground motion records at Tfix = 1.5 s is about 1.48. Thus, substituting into the left side of Eqs. (30) and (31):
Rights and permissions
About this article
Cite this article
Homaei, F. Estimation of the ductility and hysteretic energy demands for soil–structure systems. Bull Earthquake Eng 19, 1365–1413 (2021). https://doi.org/10.1007/s10518-020-01028-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10518-020-01028-2