No CrossRef data available.
Article contents
A NEW ALGORITHM FOR DECOMPOSING MODULAR TENSOR PRODUCTS
Published online by Cambridge University Press: 11 January 2021
Abstract
Let p be a prime and let
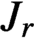$J_r$
denote a full
$r \times r$
Jordan block matrix with eigenvalue
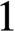$1$
over a field F of characteristic p. For positive integers r and s with
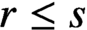$r \leq s$
, the Jordan canonical form of the
$r s \times r s$
matrix
$J_{r} \otimes J_{s}$
has the form
$J_{\lambda _1} \oplus J_{\lambda _2} \oplus \cdots \oplus J_{\lambda _{r}}$
. This decomposition determines a partition
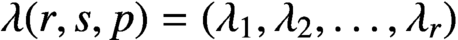$\lambda (r,s,p)=(\lambda _1,\lambda _2,\ldots , \lambda _{r})$
of
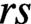$r s$
. Let
$n_1, \ldots , n_k$
be the multiplicities of the distinct parts of the partition and set
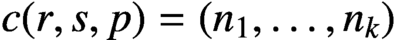$c(r,s,p)=(n_1,\ldots ,n_k)$
. Then
$c(r,s,p)$
is a composition of r. We present a new bottom-up algorithm for computing
$c(r,s,p)$
and
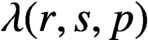$\lambda (r,s,p)$
directly from the base-p expansions for r and s.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © 2020 Australian Mathematical Publishing Association Inc.
References
Alperin, J. L., Local Representation Theory, Cambridge Studies in Advanced Mathematics, 11 (Cambridge University Press, Cambridge, 1986).CrossRefGoogle Scholar
Barry, M. J. J., ‘Decomposing tensor products and exterior and symmetric squares’, J. Group Theory 14 (2011), 59–82.CrossRefGoogle Scholar
Barry, M. J. J., ‘On a question of Glasby, Praeger and Xia’, Comm. Algebra 43 (2015), 4231–4246.CrossRefGoogle Scholar
Barry, M. J. J., ‘More on periodicity and duality associated with Jordan partitions’, Preprint, 2019, arXiv:1907.06519.Google Scholar
Glasby, S. P., Praeger, C. E. and Xia, B., ‘Decomposing modular tensor products: “Jordan partitions”, their parts and
$p$
-parts’, Israel J. Math. 209(1) (2015), 215–233.CrossRefGoogle Scholar
$p$
-parts’, Israel J. Math. 209(1) (2015), 215–233.CrossRefGoogle ScholarGlasby, S. P., Praeger, C. E. and Xia, B., ‘Decomposing modular tensor products, and periodicity of “Jordan partitions”’, J. Algebra 450 (2016), 570–587.CrossRefGoogle Scholar
Green, J. A., ‘The modular representation algebra of a finite group’, Illinois J. Math. 6 (1962), 607–619.CrossRefGoogle Scholar
Hou, X.-D., ‘Elementary divisors of tensors products and
$p$
-ranks of binomial matrices’, Linear Algebra Appl. 374 (2003), 255–274.CrossRefGoogle Scholar
$p$
-ranks of binomial matrices’, Linear Algebra Appl. 374 (2003), 255–274.CrossRefGoogle ScholarIima, K.-i. and Iwamatsu, R., ‘On the Jordan decomposition of tensored matrices of Jordan canonical forms’, Math. J. Okayama Univ. 51 (2009), 133–148.Google Scholar
Korhonen, M., ‘Jordan blocks of unipotent elements in some irreducible representations of classical groups in good characteristic’, Proc. Amer. Math. Soc. 147(10) (2019), 4205–4219.CrossRefGoogle Scholar
Lawther, R., ‘Jordan block sizes of unipotent elements in exceptional algebraic groups’, Comm. Algebra 23(11) (1995), 4125–4156.CrossRefGoogle Scholar
Lawther, R., ‘Correction to: “Jordan block sizes of unipotent elements in exceptional algebraic groups”, Comm. Algebra 23(11) (1995), 4125–4156’, Comm. Algebra 26(8) (1998), 2709.CrossRefGoogle Scholar
McFaul, J. D., ‘On the elementary divisors of the tensor product of two matrices’, Linear Algebra Appl. 33 (1980), 67–86.CrossRefGoogle Scholar
Norman, C. W., ‘On the Jordan form of the tensor product over fields of prime characteristic’, Linear Multilinear Algebra 38 (1995), 351–371.CrossRefGoogle Scholar
Ralley, T., ‘Decomposition of products of modular representations’, J. Lond. Math. Soc. 44 (1969), 480–484.CrossRefGoogle Scholar
Renaud, J.-C., ‘The decomposition of products in the modular representation ring of a cyclic group of prime power order’, J. Algebra 58 (1979), 1–11.CrossRefGoogle Scholar
Srinivasan, B., ‘The modular representation ring of a cyclic
$p$
-group’, Proc. Lond. Math. Soc. (3) 4 (1964), 677–688.CrossRefGoogle Scholar
$p$
-group’, Proc. Lond. Math. Soc. (3) 4 (1964), 677–688.CrossRefGoogle Scholar