Abstract
We establish global regularity and stability for the irrotational relativistic Euler equations with equation of state \(\bar{p}{}=K\bar{\rho }{}\), where \(0<K<1/3\), for small initial data in the expanding direction of FLRW spacetimes of the form \((\mathbb R\times \mathbb T^3,-d\bar{t}{}^2+\bar{t}{}^2\delta _{ij} dx^i dx^j\)). This provides the first case of non-dust fluid stabilization by spacetime expansion where the expansion rate is of power law type but non-accelerated. In particular, the time integral of the inverse scale factor diverges as \(t\rightarrow \infty \). The result implies that structure formation in cosmological evolution associated with the development of shocks in fluids necessarily requires a phase of deccelerating expansion of the Universe to occur in the case that the matter is massive.
Similar content being viewed by others
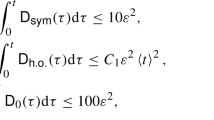
Notes
By introducing a change of time coordinate according to the formula \(\bar{t}{}= 1/t\), the metric (1.6) can be brought into the more recognizable form
$$\begin{aligned} \bar{g}{}= -d\bar{t}{}^2 +\bar{t}{}^2 \delta _{ij}dx^idx^j, \end{aligned}$$where now \((\bar{t}{},x^i)\in [1/T_0,\infty )\times \mathbb {T}{}^3\). We refer to such metrics as ‘Milne-like’ since the scale factor \(\bar{t}{}^2\) is the same as in Milne, even though the spatial geometry \((\mathbb {T}{}^3, \delta )\) is different from \((\mathbb {H}^3, g_{\mathbb {H}^3})\) or quotients thereof, appearing in the standard Milne spacetime.
The constant \(C(|||u|||_{k})\) implicitly depends on the various constants, e.g. \(\gamma _1\), \(\gamma _2\), \(\beta _1\), etc., that were introduced in the assumption in Sect. 4.1.
References
Adams, R.A., Fournier, J.: Sobolev Spaces, 2nd edn. Academic Press, Cambridge (2003)
Andersson, L., Fajman, D.: Nonlinear stability of the Milne model with matter. Commun. Math. Phys. 378, 261–298 (2020)
Andersson, L., Moncrief, V.: Einstein spaces as attractors for the Einstein flow. J. Differ. Geom. 89(1), 1–47 (2011)
Beyer, F., Oliynyk, T.A., Olvera-SantaMaría, J.A.: The Fuchsian approach to global existence for hyperbolic equations. Commun. Part. Diff. Eq. (accepted), preprint [arXiv:1907.04071]
Bildhauer, S., Buchert, T., Kasai, M.: Solutions in Newtonian cosmology: the Pancake theory with cosmological constant. Astron. Astrophys. 263, 23–29 (1992)
Christodoulou, D.: The Formation of Shocks in 3-Dimensional Fluids. EMS, Switzerland (2007)
Fajman, D., Wyatt, Z.: Attractors of the Einstein-Klein Gordon system. Comm. Partial Differential Equations (2020)
Friedman, A.: Partial Differential Equations. Krieger Publishing Company, Malabar, USA (1976)
Friedrich, H.: Sharp asymptotics for Einstein-\(\lambda \)-dust flows. Commun. Math. Phys. 350, 803–844 (2017)
Hadžić, M., Speck, J.: The global future stability of the FLRW solutions to the Dust-Einstein system with a positive cosmological constant. J. Hyper. Differ. Equ. 12, 87–188 (2015)
LeFloch, P.G., Wei, C.: The global nonlinear stability of self-gravitating irrotational Chaplygin fluids in a FRW geometry. Annales de l’Institut Henri Poincaré C, Analyse non linéaire. (2020)
Liu, C., Oliynyk, T.A.: Cosmological Newtonian limits on large spacetime scales. Commun. Math. Phys. 364, 1195–1304 (2018)
Liu, C., Oliynyk, T.A.: Newtonian limits of isolated cosmological systems on long time scales. Annales Henri Poincaré 19, 2157–2243 (2018)
Liu, C., Wei, C.: Future stability of the FLRW spacetime for a large class of perfect fluids. preprint [arXiv:1810.11788] (2019)
Lübbe, C., Kroon, J.A.V.: A conformal approach for the analysis of the non-linear stability of radiation cosmologies. Ann Phys 328, 1–25 (2013)
Oliynyk, T.A.: Future stability of the FLRW fluid solutions in the presence of a positive cosmological constant. Commun. Math. Phys. 346 (2016), 293–312; see the preprint [arXiv:1505.00857] for a corrected version
Oliynyk, T.A.: Future global stability for relativistic perfect fluids with linear equations of state \(p=K \rho \) where \(1/3\). preprint [arXiv:2002.12526] (2020)
Ringstöm, H.: Future stability of the Einstein-non-linear scalar field system. Invent. Math. 173, 123–208 (2008)
Ringström, H.: Power law inflation. Commun. Math. Phys. 290(1), 155–218 (2009)
Rodnianski, I., Speck, J.: The stability of the irrotational Euler–Einstein system with a positive cosmological constant. J. Eur. Math. Soc. 15, 2369–2462 (2013)
Speck, J.: The stabilizing effect of spacetime expansion on relativistic fluids with sharp results for the radiation equation of state. Arch. Rat. Mech. 210, 535–579 (2013)
Taylor, M.E.: Partial Differential Equations III: Nonlinear Equations. Springer, Berlin (1996)
Rendall, A.D., Brauer, U., Reula, O.: The cosmic no-hair theorem and the non-linear stability of homogeneous Newtonian cosmological models. Class. Quant. Grav 11, 2283–2296 (1994)
Wei, C.: Stabilizing effect of the power law inflation on isentropic relativistic fluids. J. Differ. Equ. 265, 3441–3463 (2018)
Acknowledgements
This work was partially supported by the Australian Research Council Grant DP170100630 and by the Swedish Research Council under Grant No. 2016-06596 while the authors were in residence at Institut Mittag-Leffler in Djursholm, Sweden during the winter semester of 2019 as part of the program General Relativity, Geometry and Analysis: beyond the first 100 years after Einstein. We are grateful to the Institute for its support and hospitality during our stay. The author T.A.O would also like to thank the Albert Einstein Institute for its support during a visit in November, 2019 where work on this article was carried out. The author Z.W was supported by The Maxwell Institute Graduate School in Analysis and its Applications, a Centre for Doctoral Training funded by the UK Engineering and Physical Sciences Research Council (grant EP/L016508/01), the Scottish Funding Council, Heriot-Watt University and the University of Edinburgh. D.F acknowledges support from the Austrian Science Fund (FWF) through the Project Geometric transport equations and the non-vacuum Einstein flow (P 29900-N27).
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by A. Ionescu
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Calculus Inequalities
Calculus Inequalities
In this appendix, we collect, for the convenience of the reader, a number of calculus inequalities that we employ. The proof of the following inequalities are well known and may be found, for example, in the books [1, 8] and [22].
Theorem A.1
(Hölder’s inequality) If \(0< p,q,r \le \infty \) satisfy \(1/p+1/q = 1/r\), then
for all \(u\in L^p(\mathbb {T}{}^{n})\) and \(v\in L^q(\mathbb {T}{}^{n})\).
Theorem A.2
(Sobolev’s inequality) Suppose \(1\le p < \infty \) and \(s\in \mathbb {Z}{}_{> n/p}\). Then
for all \(u\in W^{s,p}(\mathbb {T}{}^{n})\).
Theorem A.3
(Product and commutator estimates)
-
(i)
Suppose \(1\le p_1,p_2,q_1,q_2\le \infty \), \(s\in \mathbb {Z}{}_{\ge 1}\), \(|\alpha |=s\) and
$$\begin{aligned} \frac{1}{p_1}+\frac{1}{p_2} = \frac{1}{q_1} + \frac{1}{q_2} = \frac{1}{r}. \end{aligned}$$Then
$$\begin{aligned} \Vert D^\alpha (uv)\Vert _{L^r} \lesssim \Vert u\Vert _{W^{s,p_1}}\Vert v\Vert _{L^{q_1}} + \Vert u\Vert _{L^{p_2}}\Vert v\Vert _{W^{s,q_2}} \end{aligned}$$and
$$\begin{aligned} \Vert [D^\alpha ,u]v\Vert _{L^r} \lesssim \Vert D u\Vert _{L^{p_1}}\Vert v\Vert _{W^{s-1,q_1}} + \Vert D u\Vert _{ W^{s-1,p_2}}\Vert v\Vert _{L^{q_2}} \end{aligned}$$for all \(u,v \in C^\infty (\mathbb {T}{}^{n})\).
-
(ii)
Suppose \(s_1,s_2,s_3\in \mathbb {Z}{}_{\ge 0}\), \(\;s_1,s_2\ge s_3\), \(1\le p \le \infty \), and \(s_1+s_2-s_3 > n/p\). Then
$$\begin{aligned} \Vert uv\Vert _{W^{s_3,p}} \lesssim \Vert u\Vert _{W^{s_1,p}}\Vert v\Vert _{W^{s_2,p}} \end{aligned}$$for all \(u\in W^{s_1,p}(\mathbb {T}{}^{n})\) and \(v\in W^{s_2,p}(\mathbb {T}{}^{n})\).
Theorem A.4
(Moser’s estimates) Suppose \(1\le p \le \infty \), \(s\in \mathbb {Z}{}_{\ge 1}\), \(0\le k\le s\), \(|\alpha |=k\) and \(f\in C^s(U)\), where U is open and bounded in \(\mathbb {R}{}\) and contains 0, and \(f(0)=0\). Then
for all \(u \in C^0(\mathbb {T}{}^{n})\cap L^\infty (\mathbb {T}{}^{n})\cap W^{s,p}(\mathbb {T}{}^{n})\) with \(u(x) \in U\) for all \(x\in \mathbb {T}{}^{n}\).
Lemma A.5
(Ehrling’s lemma) Suppose \(1\le p < \infty \), \(s_0,s,s_1\in \mathbb {Z}{}_{\ge 0}\), and \(s_0< s < s_1\). Then for any \(\epsilon >0\) there exists a constant \(C=C(\epsilon ^{-1})\) such that
for all \(u\in W^{s_1,p}(\mathbb {T}{}^{n})\).
Rights and permissions
About this article
Cite this article
Fajman, D., Oliynyk, T.A. & Wyatt, Z. Stabilizing Relativistic Fluids on Spacetimes with Non-Accelerated Expansion. Commun. Math. Phys. 383, 401–426 (2021). https://doi.org/10.1007/s00220-020-03924-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00220-020-03924-9