Evolution of infection avoidance in populations affected by sexually transmitted infections

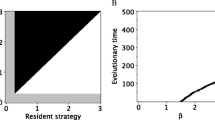
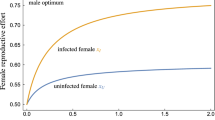
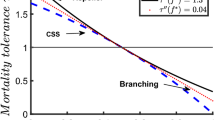
Abstract

Infectious diseases affect many populations and it should be natural to avoid contacts with infected individuals to prevent contagion. Recognizability of infected individuals is essential for infection avoidance. In the case of sexually transmitted infections, such avoidance is accomplished by choosing a healthy mating partner. We find that different mating strategies arise as a consequence of evolution of infection avoidance. Considering infection avoidance as host willingness to accept infection risk upon encountering a potential yet infected mating partner, we show that evolutionary bistability occurs: either no avoidance evolves or a degree of avoidance is attained at which the host population ends up disease-free. In the latter case, evolutionary suicide may even occur so that the host population goes extinct. Infection avoidance may also be driven by a degree of infection visibility and therefore thought of as a parasite trait. In that case, parasite crypticity (and hence no avoidance) evolves provided there is no cost on the degree of infection (non-)visibility. Considering a virulence-visibility trade-off leads to parasite crypticity, too, and no virulence. On the other hand, an intermediate degree of infection visibility becomes an evolutionary attractor if a transmissibility-visibility trade-off is adopted, or when the transmissibility-visibility trade-off and the virulence-visibility trade-off act simultaneously.

Appendices

Appendix 1

Development of model 1

Here we derive model (1) of the main text (see also Berec and Maxin, 2013; Theuer and Berec, 2018). Let $\mathcal {M}(N_F,N_M)$ denote the rate at which females of density $N_F$ and males of density $N_M$ mate; it is also called a mating function. Dynamics of female and male populations is then commonly modeled as (Caswell and Weeks, 1986; Lindström and Kokko, 1998; Kot, 2001; Rankin and Kokko, 2007)

$$\begin{aligned} \frac{\mathrm {d}N_F}{\mathrm {d}t} &= \frac{1}{2} b w \mathcal {M}(N_F,N_M) - (\mu _F + dN) N_F, \nonumber \\ \frac{\mathrm {d}N_M}{\mathrm {d}t} &= \frac{1}{2} b w \mathcal {M}(N_F,N_M) - (\mu _M + dN) N_M. \end{aligned}$$

(36)

The term $(bw/2)\mathcal {M}(N_F,N_M)$, common for both equations, stands for reproduction: w is the proportion of matings that result in reproduction, b is the number of offspring per reproductive event, and 1/2 stems from assuming a 1:1 sex ratio at birth. The second term in both equations stands for mortality, where $\mu _F$ and $\mu _M$ represent the per-capita background mortality rate of females and males, respectively. Total mortality is further modulated by a crowding factor d; $N=N_F+N_M$ is the total population density.

We now extend model 36 to account for a sexually transmitted infection (STI). To do this, we split female and male populations into two subclasses: susceptible individuals currently free of infection but prone to getting it (denoting by $S_F$ and $S_M$ the respective classes of susceptible individuals) and infected individuals which are infectious and may infect susceptible ones upon mating (denoting by $I_F$ and $I_M$ the respective classes of infected individuals). Females and males are assumed to mate randomly at a total rate $\mathcal {M}(S_F+I_F,S_M+I_M)$. Because $X/(S_F+I_F)$ is the proportion of females of type X among all females and $Y/(S_M+I_M)$ is the proportion of males of type Y among all males, the rate at which females of class X (where X is $S_F$ or $I_F$) mate with males of class Y (where Y is $S_M$ or $I_M$) is

$$\begin{aligned} \mathcal {M}(S_M+I_M,S_F+I_F)\,\frac{X}{S_F+I_F}\,\frac{Y}{S_M+I_M}. \end{aligned}$$

(37)

Upon mating between a susceptible female and an infected male, the STI is transmitted with probability $\xi _M$, hence the force of infection for the susceptible female is

$$\begin{aligned} \xi _M \mathcal {M}(S_M+I_M,S_F+I_F)\,\frac{1}{S_F+I_F}\,\frac{I_M}{S_M+I_M}. \end{aligned}$$

(38)

Similarly, upon mating between an infected female and a susceptible male, the infection is transmitted with probability $\xi _F$, hence the force of infection for the susceptible male is

$$\begin{aligned} \xi _F \mathcal {M}(S_M+I_M,S_F+I_F)\,\frac{1}{S_M+I_M}\,\frac{I_F}{S_F+I_F}. \end{aligned}$$

(39)

Finally, the STI is assumed to increase the per-capita mortality of infected females and males by $\alpha _F$ and $\alpha _M$, respectively, and to decrease the per-capita fertility of infected females and males by factors $\sigma _F$ and $\sigma _M$, respectively. With all this, we can write our sex-structured SI (susceptible-infected) model as is the total per-female per-male mating rate.

$$\begin{aligned} \frac{\mathrm {d}S_F}{\mathrm {d}t}= & \ {} \frac{bw}{2} \Lambda (S_F + (1-\sigma _F) I_F) (S_M + (1-\sigma _M) I_M) \nonumber \\&- \xi _M \Lambda S_F I_M - (\mu _F+dN) S_F, \nonumber \\ \frac{\mathrm {d}S_M}{\mathrm {d}t}= & \ {} \frac{bw}{2} \Lambda (S_F + (1-\sigma _F) I_F) (S_M + (1-\sigma _M) I_M) \nonumber \\&- \xi _F \Lambda S_M I_F - (\mu _M+dN) S_M, \nonumber \\ \frac{\mathrm {d}I_F}{\mathrm {d}t}= & \ {} \xi _M \Lambda S_F I_M - (\mu _F+dN) I_F - \alpha _F I_F, \nonumber \\ \frac{\mathrm {d}I_M}{\mathrm {d}t}= & \ {} \xi _F \Lambda S_M I_F - (\mu _M+dN) I_M - \alpha _M I_M, \end{aligned}$$

(40)

where

$$\begin{aligned} \Lambda = \frac{\mathcal {M}(S_M+I_M,S_F+I_F)}{(S_F+I_F)(S_M+I_M)} \end{aligned}$$

(41)

One of our main assumptions is that susceptible individuals are willing to mate with infected individuals with a probability $\psi$. Because of this, we modify model 40 and have

$$\begin{aligned} \frac{\mathrm {d}S_F}{\mathrm {d}t}= & \ {} \frac{bw}{2} \Lambda R - \psi \xi _M \Lambda S_F I_M - (\mu _F+dN) S_F, \nonumber \\ \frac{\mathrm {d}S_M}{\mathrm {d}t}= & \ {} \frac{bw}{2} \Lambda R - \psi \xi _F \Lambda S_M I_F - (\mu _M+dN) S_M, \nonumber \\ \frac{\mathrm {d}I_F}{\mathrm {d}t}= & \ {} \psi \xi _M \Lambda S_F I_M - (\mu _F+dN) I_F - \alpha _F I_F, \nonumber \\ \frac{\mathrm {d}I_M}{\mathrm {d}t}= & \ {} \psi \xi _F \Lambda S_M I_F - (\mu _M+dN) I_M - \alpha _M I_M, \end{aligned}$$

(42)

where

$$\begin{aligned} R= & \ {} S_F S_M + \psi [(1-\sigma _F) I_F S_M + (1-\sigma _M) I_M S_F] \nonumber \\&+ (1-\sigma _F) I_F (1-\sigma _M) I_M. \end{aligned}$$

(43)

We further assume, in line with most published epidemiological models, that female and male life histories are identical and hence that model parameters are sex-independent. Setting $\mu _F=\mu _M=\mu$, $\sigma _F=\sigma _M=\sigma$, $\xi _F=\xi _M=\xi$, and $\alpha _F=\alpha _M=\alpha$, it follows that $S_M = S_F = S/2$ where $S = S_M + S_F$ and $I_M = I_F = I/2$ where $I = I_M + I_F$ (provided that this also holds for the respective initial conditions). Summing equations for $S_F$ and $S_M$ and for $I_F$ and $I_M$ the sex-structured model 42 then reduces to the following unstructured SI model:

$$\begin{aligned} \frac{\mathrm {d}S}{\mathrm {d}t}= & \ {} b w \mathcal {M}\left( \frac{N}{2},\frac{N}{2}\right) \frac{S^2 + 2 \psi (1-\sigma ) IS + (1-\sigma )^2 I^2}{N^2} \nonumber \\&- 2 \psi \xi \mathcal {M}\left( \frac{N}{2},\frac{N}{2}\right) \frac{SI}{N^2} - (\mu +dN) S , \nonumber \\ \frac{\mathrm {d}I}{\mathrm {d}t}= & \ {} 2 \psi \xi \mathcal {M}\left( \frac{N}{2},\frac{N}{2}\right) \frac{SI}{N^2} - (\mu +dN) I - \alpha I . \end{aligned}$$

(44)

where $N=S+I$.

Next we assume, in line with many published sex-structured models, that the mating function $\mathcal {M}(N_F,N_M)$ is degree-one homogeneous, that is,

$$\begin{aligned} \mathcal {M}(ax,ay) = a \mathcal {M}(x,y) \end{aligned}$$

(45)

for any positive number x, y, a (Caswell and Weeks 1986; Miller and Inouye, 2011). We thus have $\mathcal {M}(N/2,N/2) = (N/2) \mathcal {M}(1,1)$ and model 44 becomes

$$\begin{aligned} \frac{\mathrm {d}S}{\mathrm {d}t}= & \ {} \frac{b w}{2} \mathcal {M}(1,1) \frac{S^2 + 2 \psi (1-\sigma ) IS + (1-\sigma )^2 I^2}{N} \nonumber \\&- \psi \xi \mathcal {M}(1,1)\frac{SI}{N} - (\mu +dN) S, \nonumber \\ \frac{\mathrm {d}I}{\mathrm {d}t}= & \ {} \psi \xi \mathcal {M}(1,1)\frac{SI}{N} - (\mu +dN) I - \alpha I. \end{aligned}$$

(46)

Finally, denoting $\beta = (bw/2)\mathcal {M}(1,1)$ and $\lambda = \xi \mathcal {M}(1,1)$, which are both compound parameters, we eventually get

$$\begin{aligned} \frac{\mathrm {d}S}{\mathrm {d}t}= & \ {} \beta \frac{S^2 + 2 \psi (1-\sigma ) IS + (1-\sigma )^2 I^2}{N} \nonumber \\&- \psi \lambda \frac{SI}{N} - (\mu +dN) S, \nonumber \\ \frac{\mathrm {d}I}{\mathrm {d}t}= & \ {} \psi \lambda \frac{SI}{N} - (\mu +dN) I - \alpha I. \end{aligned}$$

(47)

And this is exactly model 1 in the main text.

Appendix 2

Evolutionary singular point in case of evolution from host perspective

Non-sterilizing infections

With no sterilization ($\sigma =0$), the resident-only model 1 can be rewritten in terms of the total population density $N=S+I$ and the infection prevalence $i=I/N$ as

$$\begin{aligned} \frac{\mathrm {d}N}{\mathrm {d}t}= & \ {} N\left[ \beta \left( 1-2i\left( 1-\psi \right) \left( 1-i \right) \right) -\mu -dN-\alpha i \right] ,\nonumber \\ \frac{\mathrm {d}i}{\mathrm {d}t}= &\ {} i \left[ \left( 1 - i \right) \left( \lambda \, \psi -\alpha +2\beta i \left( 1-\psi \right) \right) -\beta \right] . \end{aligned}$$

(48)

Since the second equation in model 48 does not depend on N, the infection prevalence equilibria are $i^*_1=0$ and then $i^*_2$ and $i^*_3$ as solutions of equation

$$\begin{aligned} \left( 1 - i \right) \left( \lambda \, \psi -\alpha +2\beta i \left( 1-\psi \right) \right) -\beta = 0, \end{aligned}$$

(49)

satisfying $0 < i_{2,3}^* \le 1$. From the evolutionary perspective, we are only interested in the stable resident-only endemic equilibrium, which is

$$\begin{aligned} N^*_3= & \ {} \left[ \beta -\mu - 2 \beta i^*_3 \left( 1-\psi \right) \left( 1-i^*_3 \right) - \alpha i^*_3 \right] /d, \nonumber \\ i^*_3= & \ {} \frac{2\beta (1-\psi )-\lambda \psi +\alpha + \sqrt{\Delta } }{4\beta (1-\psi ) }, \end{aligned}$$

(50)

where $\Delta = (2\beta (1-\psi )-\lambda \psi +\alpha )^2 + 8\beta (1-\psi )(\lambda \psi -\alpha -\beta )$ (Theuer and Berec, 2018). Existence and stability conditions are provided as expressions 11 in the main text, where we denote $N^*_3$ as $N^*$ and $i^*_3$ as $i^*$.

For the evolutionary singular point $\psi ^*$ it holds that

$$\begin{aligned} i^*_3(\psi ^*) = 1+ \frac{\alpha }{\beta -\lambda \psi ^*}, \end{aligned}$$

(51)

which is derived in the main text as formula 9. Since $i^*_3$ is one of the solutions of equation 49, by plugging the formula 51 into the equation 49 we get an equation for the evolutionary singular point $\psi ^*$. The result is a quadratic equation in $\psi ^*$, with roots

$$\begin{aligned} \psi ^*_1= & \ {} \frac{\beta (\alpha +\beta )}{2\alpha \beta +\lambda (\beta -\alpha )}, \nonumber \\ \psi ^*_2= & \ {} \frac{\alpha +\beta }{\lambda }. \end{aligned}$$

(52)

Since both $i^*_3$ (for stable endemic equilibrium) and $i^*_2$ (for unstable equilibrium) are roots of 49, one of $\psi ^*_1$ and $\psi ^*_2$ corresponds to stable and one to unstable equilibrium. Plugging $\psi ^*_2$ into 51 we get $i^*_3(\psi ^*_2) = 0$, thus not an endemic equilibrium. Therefore, the evolutionary singular point $\psi ^*$, corresponding to stable endemic equilibrium, is $\psi ^*_1$.

Partially sterilizing infections

For partially sterilizing infections ($0<\sigma <1$) we follow similar calculations , which are however more complicated. The resident-only model 1 can be rewritten in terms of the total population density $N=S+I$ and the infection prevalence $i=I/N$ as

$$\begin{aligned} \frac{\mathrm {d}N}{\mathrm {d}t}= & \ {} N\left[ \beta \left( 1-2i X \right) -\mu -dN-\alpha i \right] ,\nonumber \\ \frac{\mathrm {d}i}{\mathrm {d}t}= & \ {} i \left[ \left( 1 - i \right) \left( \lambda \, \psi -\alpha \right) +2\beta i X -\beta \right] , \end{aligned}$$

(53)

where $X= \sigma -i \sigma ^2/2 +(1-\sigma )(1-\psi )(1-i)$. Again, since the second equation in model 53 does not depend on N, the infection prevalence equilibria are $i^*_1=0$ and then $i^*_2$ and $i^*_3$ as solutions of equation (again provided that $0 < i_{2,3}^* \le 1$)

$$\begin{aligned} \left( 1 - i \right) \left( \lambda \, \psi -\alpha \right) +2\beta i \left( \sigma -i \sigma ^2/2 +(1-\sigma )(1-\psi )(1-i)\right) -\beta = 0. \end{aligned}$$

(54)

Equation 9 also holds for the evolutionary singular point $\psi ^*$ in partially sterilizing infections. Plugging the formula 9 into 54, we get an equation for the evolutionary singular point $\psi ^*$. As for non-sterilizing infections, the result is a quadratic equation in $\psi ^*$, which now has roots

$$\begin{aligned} \psi ^*_1= & \ {} \frac{\beta (\alpha +\beta )(1-\sigma )^2}{2\alpha \beta (1-\sigma )+\lambda (\beta (1-\sigma )^2-\alpha )}, \nonumber \\ \psi ^*_2= & \ {} \frac{\alpha +\beta }{\lambda }. \end{aligned}$$

(55)

Plugging $\psi ^*_2$ into 9 we get $i^*(\psi ^*_2) = 0$, thus not an endemic equilibrium. Therefore the evolutionary singular point $\psi ^*$, corresponding to stable endemic equilibrium, is $\psi ^*_1$.