The Compact Nanoradian Precision Laser Inclinometer—an Innovative Instrument for the Angular Microseismic Isolation of the Interferometric Gravitational Antennas

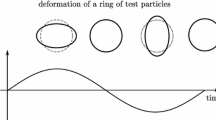
Abstract

The conceptual new design of the Precision Laser Inclinometer (PLI) is proposed and developed what resulted in creation of compact instrument for its possible use in a restricted vacuum volume of sensitive elements of Gravitational Wave Interferometric Antennas. It is shown that the use of a position sensitive method—the dividing plates method—for the detection of angular inclination of laser beams, allows to reduce the dimensions of the PLI to a cube of 11 × 11 × 15 cm³ or to a cylinder with a diameter of 15 cm and a height of 11 cm. The reduction of the dimensions results in a simultaneous increase of 1.9 times in the sensitivity of recording the angular inclinations of the earth’s surface.

Appendices

APPENDIX A

Figure 1 shows the displacement α of the laser beam on the dividing plate. One can determine the difference between the signals from the photodetectors Ph1 and Ph2 taking into account the reflection from the glass plate and the metal layer.

For the calculation, we introduce the following notation:

R_m—the coefficient of reflection from the metallic surface;

R_g—the coefficient of reflection from the glass surface;

T_g—the coefficient of transmission by the flat-parallel glass plate.

Considering that the optical plate has two reflective surfaces it can be shown that R_g and T_g are:

$${{R}_{g}} = 2R\left( {1 - R} \right),$$

(A.1)

$${{T}_{g}} = 1 - {{R}_{g}},$$

(A.2)

where R is the coefficient of reflection from one surface of a dividing glass plate.

One can determine the power of the laser beam, which arrives on the photodetectors Ph1, Ph2:

$$\begin{gathered} {{P}_{{{\text{Ph1}}}}} = {{P}_{m}} + {{P}_{g}} = {{R}_{m}}\left( {\frac{P}{2} - {{P}_{\alpha }}} \right) + {{R}_{g}}\left( {\frac{P}{2} + {{P}_{\alpha }}} \right) \\ = P\left[ {{{R}_{m}}\left( {\frac{1}{2} - \frac{{{{P}_{\alpha }}}}{P}} \right) + {{R}_{g}}\left( {\frac{1}{2} + \frac{{{{P}_{\alpha }}}}{P}} \right)} \right], \\ \end{gathered} $$

$$\begin{gathered} {{P}_{{{\text{Ph2}}}}} = \left( {\frac{P}{2} + {{P}_{\alpha }}} \right){{T}_{g}} = \left( {\frac{P}{2} + {{P}_{\alpha }}} \right)\left( {1 - {{R}_{g}}} \right) \\ = P\left[ {\left( {\frac{1}{2} + \frac{{{{P}_{\alpha }}}}{P}} \right) - {{R}_{g}}\left( {\frac{1}{2} + \frac{{{{P}_{\alpha }}}}{P}} \right)} \right]. \\ \end{gathered} $$

Thus, the power difference arriving on the photodetectors Ph1 and Ph2 is

$$\begin{gathered} \Delta P = {{P}_{{{\text{Ph2}}}}} - {{P}_{{{\text{Ph1}}}}} \\ = P\left[ {\left( {\frac{1}{2} + \frac{{{{P}_{\alpha }}}}{P}} \right) - {{R}_{m}}\left( {\frac{1}{2} - \frac{{{{P}_{\alpha }}}}{P}} \right) - 2{{R}_{g}}\left( {\frac{1}{2} + \frac{{{{P}_{\alpha }}}}{P}} \right)} \right]. \\ \end{gathered} $$

With a symmetric arrangement of the laser beam relative to the dividing line of the dividing plate (α = 0 and consequently ${{P}_{\alpha }} = 0$), the difference in power of the laser beams falling on the photoreceivers Ph1, Ph2 will be

$$\Delta {{P}_{0}} = {{P}_{{{\text{Ph2}}}}} - {{P}_{{{\text{Ph1}}}}} = P\left( {\frac{1}{2} - \frac{{{{R}_{m}}}}{2} - {{R}_{g}}} \right).$$

(A.3)

Taking into account the proportional dependence of the signal U on laser power P, we determine the difference signals $\Delta U$ and $\Delta {{U}_{{\alpha }}}$ from the photoreceivers Ph1 and Ph2.

Thus, the difference signal and $\Delta {{U}_{{\alpha }}}$ from the photoreceivers Ph1 and Ph2, corresponding to the offset α of the laser beam from the symmetric position of the laser beam relative to the dividing line, will be

$$\begin{gathered} \Delta {{U}_{\alpha }} = \Delta U - \Delta {{U}_{0}} = U\frac{{\Delta P}}{P} - U\frac{{\Delta {{P}_{0}}}}{P} \\ = U\frac{{{{P}_{\alpha }}}}{P}\left( {1 + {{R}_{m}} - 2{{R}_{g}}} \right) = K~U\frac{{{{P}_{\alpha }}}}{P}.~~ \\ \end{gathered} $$

(A.4)

As can be seen from the expression (A.4) $\Delta {{U}_{{\alpha }}}$ is proportional to the relative power $~\frac{{{{P}_{{\alpha }}}}}{P}$ of the laser radiation in gap corresponding to the displacement α.

When using

(a) the metallic reflecting layer of Al (${{R}_{m}} = 0.85$);

(b) glass with index of refraction n = 1.5;

(c) polarized laser irradiation of λ = 0.65 μm;

(d) plate inclination angle of 45° ($2{{R}_{g}} = 0.05$)

one gets finally $K = \left( {1 + {{R}_{m}} - 2{{R}_{g}}} \right) =$1.8.

APPENDIX B

To find the displacement signal $\frac{{\Delta {{U}_{{\alpha }}}}}{U}$ one is to determine the $\frac{{{{P}_{\alpha }}}}{P}$ ratio in function of the displacement α.

Figure 19 shows a single-mode laser beam spot, separated into two parts by a dividing plate.

Fig. 18.

The single-mode laser beam displacement on the dividing plate.

Fig. 19.

The dividing of the single-mode laser beam by the dividing plate.

We need to determine the power difference between the laser beams incident on the photoreceivers Ph1 and Ph2 (Fig. 18). The power of the laser beam P₁, transmitted by the plate and the reflected power P₂, are shown in Fig. 19.

Define the expression P₁ and P₂ for a single-mode laser beam

$$\begin{gathered} {{P}_{1}} = \frac{{2P}}{{\pi r_{0}^{2}}}{{P}_{y}}{{P}_{x}} = \frac{{2P}}{{\pi {{r}^{2}}}}\left( {\int\limits_{ - \infty }^\infty {\exp } \left( { - \frac{{2{{y}^{2}}}}{{r_{0}^{2}}}} \right)dy} \right) \\ \times \,\,\int\limits_{ - w}^a {\exp } \left( { - 2\frac{{{{x}^{2}}}}{{r_{0}^{2}}}} \right)dx = P\left\{ {{\text{erf}}\left( {\frac{{w\sqrt 2 }}{{{{r}_{0}}}}} \right) + {\text{erf}}\left( {\frac{{\alpha \sqrt 2 }}{{{{r}_{0}}}}} \right)} \right\}, \\ \end{gathered} $$

$$\begin{gathered} {{P}_{2}} = \frac{{2P}}{{\pi r_{0}^{2}}}{{P}_{y}}{{P}_{x}} = \frac{{2P}}{{\pi r_{0}^{2}}}\left( {\int\limits_{ - \infty }^\infty {\exp } \left( { - \frac{{2{{y}^{2}}}}{{r_{0}^{2}}}} \right)dy} \right) \\ \times \,\,\int\limits_\alpha ^w {\exp } \left( { - 2\frac{{{{x}^{2}}}}{{r_{0}^{2}}}} \right)dx = P\left\{ {{\text{erf}}\left( {\frac{{\alpha \sqrt 2 }}{{{{r}_{0}}}}} \right) - {\text{erf}}\left( {\frac{{w\sqrt 2 }}{{{{r}_{0}}}}} \right)} \right\}, \\ \end{gathered} $$

$$\begin{gathered} {{P}_{1}} - {{P}_{2}} = 2P\left\{ {{\text{erf}}\left( {\frac{{w\sqrt 2 }}{{{{r}_{0}}}}} \right) + {\text{erf}}\left( {\frac{{\alpha \sqrt 2 }}{{{{r}_{0}}}}} \right)} \right. \\ + {\text{erf}}\left( {\frac{{\alpha \sqrt 2 }}{{{{r}_{0}}}}} \right) - \left. {{\text{erf}}\left( {\frac{{w\sqrt 2 }}{{{{r}_{0}}}}} \right)} \right\} = 2\frac{{2P}}{{\pi r_{0}^{2}}}{\text{erf}}\left( {\frac{{\alpha \sqrt 2 }}{{{{r}_{0}}}}} \right), \\ \end{gathered} $$

$$\begin{gathered} {{P}_{1}} + {{P}_{2}} = P\left\{ {{\text{erf}}\left( {\frac{{w\sqrt 2 }}{r}} \right) + {\text{erf}}\left( {\frac{{\alpha \sqrt 2 }}{r}} \right)} \right. \\ \left. { - \,\,{\text{erf}}\left( {\frac{{\alpha \sqrt 2 }}{{{{r}_{0}}}}} \right) + {\text{erf}}\left( {\frac{{w\sqrt 2 }}{{{{r}_{0}}}}} \right)} \right\} = 2P{\text{erf}}\left( {\frac{{w\sqrt 2 }}{{{{r}_{0}}}}} \right), \\ \end{gathered} $$

$$\frac{{{{P}_{1}} - {{P}_{2}}}}{{{{P}_{1}} + {{P}_{2}}}} = \frac{{{{P}_{\alpha }}}}{P} = \frac{{{\text{erf}}\left( {\frac{{\alpha \sqrt 2 }}{{{{r}_{0}}}}} \right)}}{{{\text{erf}}\left( {\frac{{w\sqrt 2 }}{{{{r}_{0}}}}} \right)}}~,~~~$$

(B.1)

where r₀ is radius of the laser beam and w = 300 μm is—as accepted by us—the upper value of possible α displacement.

Thus, the final expression for the photoreceivers Ph1 and Ph2 difference signal from in the method of dividing plates has the form

$$\frac{{\Delta {{U}_{\alpha }}}}{U} = 1.8~\frac{{{\text{erf}}\left( {\frac{{\alpha \sqrt 2 }}{{{{r}_{0}}}}} \right)}}{{{\text{erf}}\left( {\frac{{w\sqrt 2 }}{{{{r}_{0}}}}} \right)}}.~~$$

(B.2)