Skip to main content
SpringerLink
Log in

Dynamics of nonlocal thick nano-bars

  • Original Article
  • Published:
Engineering with Computers Aims and scope Submit manuscript

Abstract

The thick bar model, accounting for the lateral deformation, shear stiffness, and lateral inertia effect, is the most comprehensive structural theory to study the axial deformation of carbon nanotubes. Physically motivated definition of the axial force field and associated higher order boundary conditions are determined applying a consistent variational framework. The effects of long-range interactions are suitably realized in the framework of the nonlocal integral elasticity. The integral convolutions of the nonlocal constitutive law are determined and suitably resorted with the equivalent nonlocal differential model equipped with non-standard boundary conditions. Preceding contributions on the elastodynamic analysis of the elastic thick bar are, therefore, amended by properly taking into account the higher order and non-standard boundary conditions. The established size-dependent thick bar model is demonstrated to be exempt from the inherent drawbacks of the nonlocal differential formulation and leads to well-posed elastodynamic problems. The wave desperation response and free vibrational behavior of elastic thick bars with kinematic constraints of nano-mechanics interest are rigorously investigated by making recourse to a viable solution approach. New numerical benchmarks are detected for the elastodynamic response of nonlocal thick nano-bars. A consistent approach for nanoscopic study of the field quantities in the nonlocal mechanics is proposed that is capable of properly confirming the smaller-is-softer phenomenon.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Ansari R, Hasrati E, Torabi J (2020) Effect of external pressure on the vibration analysis of higher order shear deformable FG-CNTRC spherical panels. Eng Comput. https://doi.org/10.1007/s00366-020-01138-0

    Article  Google Scholar 

  2. Civalek Ö, Avcar M (2020) Free vibration and buckling analyses of CNT reinforced laminated non-rectangular plates by discrete singular convolution method. Eng Comput. https://doi.org/10.1007/s00366-020-01168-8

    Article  Google Scholar 

  3. Zare Y, Rhee KY (2020) Effect of interfacial/interphase conductivity on the electrical conductivity of polymer carbon nanotubes nanocomposites. Eng Comput. https://doi.org/10.1007/s00366-020-01062-3

    Article  Google Scholar 

  4. Marami G, Adib Nazari S, Faghidian SA, Vakili-Tahami F, Etemadi S (2016) Improving the mechanical behavior of the adhesively bonded joints using RGO additive. Int J Adhes Adhes 70:277–286. https://doi.org/10.1016/j.ijadhadh.2016.07.014

    Article  Google Scholar 

  5. Jena SK, Chakraverty S, Malikan M (2020a) Application of shifted Chebyshev polynomial-based Rayleigh-Ritz method and Navier’s technique for vibration analysis of a functionally graded porous beam embedded in Kerr foundation. Eng Comput. https://doi.org/10.1007/s00366-020-01018-7

    Article  Google Scholar 

  6. Jena SK, Chakraverty S, Malikan M (2020b) Implementation of non-probabilistic methods for stability analysis of nonlocal beam with structural uncertainties. Eng Comput. https://doi.org/10.1007/s00366-020-00987-z

    Article  Google Scholar 

  7. Dilena M, Fedele Dell’Oste M, Fernández-Sáez J, Morassi A, Zaera R (2020) Hearing distributed mass in nanobeam resonators. Int J Solids Struct 193–194:568–592. https://doi.org/10.1016/j.ijsolstr.2020.02.025

    Article  Google Scholar 

  8. Roudbari MA, Ansari R (2020) Single-walled boron nitride nanotube as nano-sensor. Continuum Mech Thermodyn 32:729–748. https://doi.org/10.1007/s00161-018-0719-6

    Article  MathSciNet  Google Scholar 

  9. Li L, Lin R, Ng TY (2020a) A fractional nonlocal time-space viscoelasticity theory and its applications in structural dynamics. Appl Math Model 84:116–136. https://doi.org/10.1016/j.apm.2020.03.048

    Article  MathSciNet  MATH  Google Scholar 

  10. Gholipour A, Ghayesh MH, Hussain S (2020) A continuum viscoelastic model of Timoshenko NSGT nano-beams. Eng Comput. https://doi.org/10.1007/s00366-020-01017-8

    Article  Google Scholar 

  11. Torabi J, Ansari R, Zabihi A, Hosseini K (2020) Dynamic and pull-in instability analyses of functionally graded nanoplates via nonlocal strain gradient theory. Mech Based Des Struct Mach. https://doi.org/10.1080/15397734.2020.1721298

    Article  Google Scholar 

  12. Pinnola FP, Faghidian SA, Barretta R, Marotti de Sciarra F (2020) Variationally consistent dynamics of nonlocal gradient elastic beams. Int J Eng Sci 149:103220. https://doi.org/10.1016/j.ijengsci.2020.103220

    Article  MathSciNet  MATH  Google Scholar 

  13. Barretta R, Faghidian SA, Marotti de Sciarra F, Penna R, Pinnola FP (2020) On torsion of nonlocal Lam strain gradient FG elastic beams. Compos Struct 233:111550. https://doi.org/10.1016/j.compstruct.2019.111550

    Article  Google Scholar 

  14. Jena SK, Chakraverty S, Malikan M, Tornabene F (2020) Effects of surface energy and surface residual stresses on vibro-thermal analysis of chiral, zigzag, and armchair types of SWCNTs using refined beam theory. Mech Based Des Struct Mach. https://doi.org/10.1080/15397734.2020.1754239

    Article  Google Scholar 

  15. Jena SK, Chakraverty S, Malikan M (2019) Implementation of Haar wavelet, higher order Haar wavelet, and differential quadrature methods on buckling response of strain gradient nonlocal beam embedded in an elastic medium. Eng Comput. https://doi.org/10.1007/s00366-019-00883-1

    Article  Google Scholar 

  16. Serrano O, Zaera R, Fernández-Sáez J, Ruzzene M (2019) Generalized continuum model for the analysis of nonlinear vibrations of taut strings with microstructure. Int J Solids Struct 164:157–167. https://doi.org/10.1016/j.ijsolstr.2019.01.014

    Article  Google Scholar 

  17. Eringen AC (2002) Nonlocal continuum field theories. Springer, New York

    MATH  Google Scholar 

  18. Romano G, Diaco M (2020) On formulation of nonlocal elasticity problems. Meccanica. https://doi.org/10.1007/s11012-020-01183-5

    Article  Google Scholar 

  19. Fuschi P, Pisano AA, Polizzotto C (2019) Size effects of small-scale beams in bending addressed with a strain-difference based nonlocal elasticity theory. Int J Mech Sci 151:661–671. https://doi.org/10.1016/j.ijmecsci.2018.12.024

    Article  Google Scholar 

  20. Pisano AA, Fuschi P, Polizzotto C (2020) A strain-difference based nonlocal elasticity theory for small-scale shear-deformable beams with parametric warping. Int J Multiscale Comput Eng 18(1):83–102. https://doi.org/10.1615/IntJMultCompEng.2019030885

    Article  Google Scholar 

  21. Zhu XW, Li L (2019) A well-posed Euler–Bernoulli beam model incorporating nonlocality and surface energy effect. Appl Math Mech 40:1561–1588. https://doi.org/10.1007/s10483-019-2541-5

    Article  MathSciNet  MATH  Google Scholar 

  22. Li L, Lin R, Ng TY (2020b) Contribution of nonlocality to surface elasticity. Int J Eng Sci 152:103311. https://doi.org/10.1016/j.ijengsci.2020.103311

    Article  MathSciNet  MATH  Google Scholar 

  23. Faghidian SA (2020a) Higher-order nonlocal gradient elasticity: a consistent variational theory. Int J Eng Sci 154:103337. https://doi.org/10.1016/j.ijengsci.2020.103337

    Article  MathSciNet  MATH  Google Scholar 

  24. Faghidian SA (2020b) Two-phase local/nonlocal gradient mechanics of elastic torsion. Math Methods Appl Sci. https://doi.org/10.1002/mma.6877

    Article  Google Scholar 

  25. Faghidian SA (2020c) Higher-order mixture nonlocal gradient theory of wave propagation. Math Methods Appl Sci. https://doi.org/10.1002/mma.6885

    Article  MATH  Google Scholar 

  26. Ebrahimi F, Barati MR, Civalek Ö (2020) Application of Chebyshev–Ritz method for static stability and vibration analysis of nonlocal microstructure-dependent nano-structures. Eng Comput 36:953–964. https://doi.org/10.1007/s00366-019-00742-z

    Article  Google Scholar 

  27. Sedighi HM, Malikan M (2020) Stress-driven nonlocal elasticity for nonlinear vibration characteristics of carbon/boron-nitride hetero-nanotube subject to magneto-thermal environment. Phys Scr 95:055218. https://doi.org/10.1088/1402-4896/ab7a38

    Article  Google Scholar 

  28. Ansari R, Torabi J, Norouzzadeh A (2020) An integral nonlocal model for the free vibration analysis of Mindlin nanoplates using the VDQ method. Eur Phys J Plus 135:206. https://doi.org/10.1140/epjp/s13360-019-00018-x

    Article  Google Scholar 

  29. Fazlali M, Faghidian SA, Asghari M, Shodja HM (2020) Nonlinear flexure of Timoshenko–Ehrenfest nano-beams via nonlocal integral elasticity. Eur Phys J Plus 135:638. https://doi.org/10.1140/epjp/s13360-020-00661-9

    Article  Google Scholar 

  30. Ouakad HM, Valipour A, Żur KK, Sedighi HM, Reddy JN (2020) On the nonlinear vibration and static deflection problems of actuated hybrid nanotubes based on the stress-driven nonlocal integral elasticity. Mech Mater 148:103532. https://doi.org/10.1016/j.mechmat.2020.103532

    Article  Google Scholar 

  31. Civalek Ö, Uzun B, Yaylı MÖ, Akgöz B (2020) Size-dependent transverse and longitudinal vibrations of embedded carbon and silica carbide nanotubes by nonlocal finite element method. Eur Phys J Plus 135:381. https://doi.org/10.1140/epjp/s13360-020-00385-w

    Article  Google Scholar 

  32. Sedighi HM, Ouakad HM, Dimitri R, Tornabene F (2020) Stress-driven nonlocal elasticity for the instability analysis of fluid-conveying C-BN hybrid-nanotube in a magneto-thermal environment. Phys Scr 95:065204. https://doi.org/10.1088/1402-4896/ab793f

    Article  Google Scholar 

  33. Jena SK, Chakraverty S, Malikan M (2020c) Vibration and buckling characteristics of nonlocal beam placed in a magnetic field embedded in Winkler–Pasternak elastic foundation using a new refined beam theory: an analytical approach. Eur Phys J Plus 135:164. https://doi.org/10.1140/epjp/s13360-020-00176-3

    Article  Google Scholar 

  34. Hache F, Challamel N, Elishakoff I (2019a) Asymptotic derivation of nonlocal beam models from two-dimensional nonlocal elasticity. Math Mech Solids 24:2425–2443. https://doi.org/10.1177/1081286518756947

    Article  MathSciNet  MATH  Google Scholar 

  35. Hache F, Challamel N, Elishakoff I (2019b) Asymptotic derivation of nonlocal plate models from three-dimensional stress gradient elasticity. Continuum Mech Thermodyn 31:47–70. https://doi.org/10.1007/s00161-018-0622-1

    Article  MathSciNet  MATH  Google Scholar 

  36. Jena SK, Chakraverty S (2019) Dynamic behavior of an electromagnetic nanobeam using the Haar wavelet method and the higher-order Haar wavelet method. Eur Phys J Plus 134:538. https://doi.org/10.1140/epjp/i2019-12874-8

    Article  Google Scholar 

  37. Challamel N, Aydogdu M, Elishakoff I (2018) Statics and dynamics of nanorods embedded in an elastic medium: nonlocal elasticity and lattice formulations. Eur J Mech A Solids 67:254–271. https://doi.org/10.1016/j.euromechsol.2017.09.009

    Article  MathSciNet  MATH  Google Scholar 

  38. Hache F, Challamel N, Elishakoff I (2018) Lattice and continualized models for the buckling study of nonlocal rectangular thick plates including shear effects. Int J Mech Sci 145:221–230. https://doi.org/10.1016/j.ijmecsci.2018.04.058

    Article  Google Scholar 

  39. Civalek Ö, Numanoğlu HM (2020) Nonlocal finite element analysis for axial vibration of embedded love-bishop nanorods. Int J Mech Sci 188:105939. https://doi.org/10.1016/j.ijmecsci.2020.105939

    Article  Google Scholar 

  40. Barretta R, Faghidian SA, Marotti de Sciarra F (2020) A consistent variational formulation of Bishop nonlocal rods. Continuum Mech Thermodyn 32:1311–1323. https://doi.org/10.1007/s00161-019-00843-6

    Article  MathSciNet  Google Scholar 

  41. Yaylı MÖ (2020) Axial vibration analysis of a Rayleigh nanorod with deformable boundaries. Microsyst Technol 26:2661–2671. https://doi.org/10.1007/s00542-020-04808-7

    Article  Google Scholar 

  42. Uzun B, Kafkas U, Yaylı MÖ (2020) Axial dynamic analysis of a Bishop nanorod with arbitrary boundary conditions. ZAMM Z Angew Math Mech. https://doi.org/10.1002/zamm.202000039

    Article  MathSciNet  Google Scholar 

  43. Ecsedi I, Baksa A (2017) Free axial vibration of nanorods with elastic medium interaction based on nonlocal elasticity and Rayleigh model. Mech Res Commun 86:1–4. https://doi.org/10.1016/j.mechrescom.2017.10.003

    Article  Google Scholar 

  44. Güven U (2014) A generalized nonlocal elasticity solution for the propagation of longitudinal stress waves in bars. Eur J Mech A Solids 45:75–79. https://doi.org/10.1016/j.euromechsol.2013.11.014

    Article  MathSciNet  MATH  Google Scholar 

  45. Barretta R, Faghidian SA, Marotti de Sciarra F (2019) Aifantis versus Lam strain gradient models of Bishop elastic rods. Acta Mech 230:2799–2812. https://doi.org/10.1007/s00707-019-02431-w

    Article  MathSciNet  MATH  Google Scholar 

  46. Li X-F, Shen Z-B, Lee KY (2017) Axial wave propagation and vibration of nonlocal nanorods with radial deformation and inertia. ZAMM Z Angew Math Mech 97:602–616. https://doi.org/10.1002/zamm.201500186

    Article  MathSciNet  Google Scholar 

  47. Yosida K (1978) Functional analysis. Springer, New York

    Book  Google Scholar 

  48. Polyanin A, Manzhirov A (2008) Handbook of integral equations. CRC Press, New York

    Book  Google Scholar 

  49. Zhu X, Li L (2017a) Longitudinal and torsional vibrations of size-dependent rods via nonlocal integral elasticity. Int J Mech Sci 133:639–650. https://doi.org/10.1016/j.ijmecsci.2017.09.030

    Article  Google Scholar 

  50. Zhu X, Li L (2017b) On longitudinal dynamics of nanorods. Int J Eng Sci 120:129–145. https://doi.org/10.1016/j.ijengsci.2017.08.003

    Article  Google Scholar 

  51. Apuzzo A, Barretta R, Fabbrocino F, Faghidian SA, Luciano R, de Sciarra MF (2019) Axial and torsional free vibrations of elastic nano–beams by stress–driven two–phase elasticity. J Appl Comput Mech 5:402–413. https://doi.org/10.22055/jacm.2018.26552.1338

    Article  Google Scholar 

  52. Faghidian SA (2014) A smoothed inverse eigenstrain method for reconstruction of the regularized residual fields. Int J Solids Struct 51:4427–4434. https://doi.org/10.1016/j.ijsolstr.2014.09.012

    Article  Google Scholar 

  53. Faghidian SA (2015) Inverse determination of the regularized residual stress and eigenstrain fields due to surface peening. J Strain Anal Eng Des 50:84–91. https://doi.org/10.1177/0309324714558326

    Article  Google Scholar 

  54. Xiao W, Li L, Wang M (2017) Propagation of in-plane wave in viscoelastic monolayer graphene via nonlocal strain gradient theory. Appl Phys A 123:388. https://doi.org/10.1007/s00339-017-1007-1

    Article  Google Scholar 

  55. De Domenico D, Askes H, Aifantis EC (2018) Capturing wave dispersion in heterogeneous and microstructured materials through a three-length-scale gradient elasticity formulation. J Mech Behav Mater 27:20182002. https://doi.org/10.1515/jmbm-2018-2002

    Article  Google Scholar 

  56. De Domenico D, Askes H (2018) Nano-scale wave dispersion beyond the First Brillouin Zone simulated with inertia gradient continua. J Appl Phys 124:205107. https://doi.org/10.1063/1.5045838

    Article  Google Scholar 

  57. De Domenico D, Askes H, Aifantis EC (2019) Gradient elasticity and dispersive wave propagation: model motivation and length scale identification procedures in concrete and composite laminates. Int J Solids Struct 158:176–190. https://doi.org/10.1016/j.ijsolstr.2018.09.007

    Article  Google Scholar 

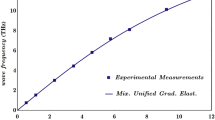
  58. Maultzsch J, Reich S, Thomsen C, Requardt H, Ordejón P (2004) Phonon dispersion in graphite. Phys Rev Lett 92:075501. https://doi.org/10.1103/PhysRevLett.92.075501

    Article  Google Scholar 

  59. Mohr M, Maultzsch J, Dobardžić E, Reich S, Milošević I, Damnjanović M, Bosak A, Krisch M, Thomsen C (2007) Phonon dispersion of graphite by inelastic X-ray scattering. Phys Rev B 76:035439. https://doi.org/10.1103/PhysRevB.76.035439

    Article  Google Scholar 

  60. Caprio MA (2005) LevelScheme: a level scheme drawing and scientific figure preparation system for mathematica. Comput Phys Commun 171:107–118. https://doi.org/10.1016/j.cpc.2005.04.010

    Article  MATH  Google Scholar 

Download references

Acknowledgements

Hamid Mohammad-Sedighi is grateful to the Research Council of Shahid Chamran University of Ahvaz for its financial support (Grant No. SCU.EM99.98).

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Science and Research Branch, Islamic Azad University, Tehran, Iran

    S. Ali Faghidian

  2. Mechanical Engineering Department, Faculty of Engineering, Shahid Chamran University of Ahvaz, Ahvaz, Iran

    Hamid Mohammad-Sedighi

  3. Drilling Center of Excellence and Research Center, Shahid Chamran University of Ahvaz, Ahvaz, Iran

    Hamid Mohammad-Sedighi

Authors
  1. S. Ali Faghidian
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hamid Mohammad-Sedighi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. Ali Faghidian.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Faghidian, S.A., Mohammad-Sedighi, H. Dynamics of nonlocal thick nano-bars. Engineering with Computers 38, 2487–2496 (2022). https://doi.org/10.1007/s00366-020-01216-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00366-020-01216-3

Keywords

Search

Navigation