Skip to main content
SpringerLink
Log in

Nonlinear responses and bifurcations of a rotor-bearing system supported by squeeze-film damper with retainer spring subjected to base excitations

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

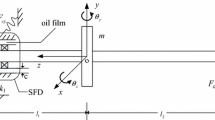
A high-speed rotating rotor system mounted on a moving vehicle is inevitably subjected to parametric excitations and exciting forces induced by base motions. Dynamic characteristics of a rotor-bearing system supported by squeeze-film damper with retainer spring subjected to unbalance and support motions are investigated. Using Lagrange’s principle, equations of motion for rotor system relative to a moving support are derived. Under base excitations, steady-state and transient responses are analyzed by frequency–amplitude curve, waveform, orbit, frequency spectrum, and Poincaré map. Changing with rotating speed or base harmonic frequency, journal motions are analyzed by bifurcation diagram. The results indicate that under base axial rotation, increasing base angular velocity, first two critical speeds decrease but resonant amplitudes increase slightly. The journal whirls around the static eccentricity with noncircular orbit. Under base lateral rotation, critical speeds, and resonant amplitudes remain essentially unchanged, but orbit’s deviation is related to base angular velocity. Excited by base harmonic translation, the integral multiples of fundamental frequency \(k{\varOmega }\left( {k = 1,2} \right)\), base harmonic frequency \({\varOmega}^{z}\), and combined frequencies \(k{\varOmega } \pm j{\varOmega }^{z} { }\left( {k,j = 1,2} \right)\) are stimulated, changing the motions from periodic to quasiperiodic. Overall, it provides a flexible approach with good expandability to predict dynamic characteristics of squeeze-film damped rotor system under base motions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26
Fig. 27
Fig. 28
Fig. 29
Fig. 30
Fig. 31

Similar content being viewed by others

References

  1. Barrett, L.E., Gunter, E.J.: Steady State and Transient Analysis of a Squeeze Film Damper Bearing for Rotor Stability. National Aeronautics and Space Administration, Washington, DC (1975)

    Google Scholar 

  2. Holmes, R., Dogan, M.: Investigation of a rotor bearing assembly incorporating a squeeze-film damper bearing. J. Mech. Eng. Sci. 24(3), 129–137 (1982). https://doi.org/10.1243/JMES_JOUR_1982_024_026_02

    Article  Google Scholar 

  3. Holmes, R., Dogan, M.: The performance of a sealed squeeze-film bearing in a flexible support structure. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 199(1), 1–9 (1985). https://doi.org/10.1243/PIME_PROC_1985_199_084_02

    Article  Google Scholar 

  4. Holmes, R., Sykes, J.E.H.: The vibration of an aero-engine rotor incorporating two squeeze-film dampers. Proc. Inst. Mech Eng. Part G J. Aerospace Eng. 210(7), 39–51 (1996). https://doi.org/10.1243/PIME_PROC_1996_210_343_02

    Article  Google Scholar 

  5. Vance, J.M.: Rotordynamics of Turbomachinery. Wiley, New York (1988)

    Google Scholar 

  6. Mclean, L.J., Hahn, E.J.: Unbalance behavior of squeeze film damped multi-mass flexible rotor bearing systems. J. Lubr. Technol. 105(1), 22–28 (1983). https://doi.org/10.1115/1.3261094

    Article  Google Scholar 

  7. Mclean, L.J., Hahn, E.J.: Stability of squeeze film damped multi-mass flexible rotor bearing systems. J. Tribol. 107(3), 402–409 (1985). https://doi.org/10.1115/1.3261094

    Article  Google Scholar 

  8. Zhao, J.Y., Linnett, I.W., Mclean, L.J.: Stability and bifurcation of unbalanced response of a squeeze film damped flexible rotor. J. Tribol. 116(2), 361–368 (1994a). https://doi.org/10.1115/1.2927236

    Article  Google Scholar 

  9. Zhao, J.Y., Linnett, I.W., Mclean, L.J.: Subharmonic and quasi-periodic motions of an eccentric squeeze film damper-mounted rigid rotor. J. Vib. Acoust. Trans. ASME 116(3), 357–363 (1994b). https://doi.org/10.1115/1.2930436

    Article  Google Scholar 

  10. Bonello, P., Brennan, M.J., Holmes, R.: Non-linear modelling of rotor dynamic systems with squeeze film dampers—an efficient integrated approach. J. Sound Vib. 249(4), 743–773 (2002). https://doi.org/10.1006/jsvi.2001.3911

    Article  Google Scholar 

  11. Bonello, P., Hai, P.M.: A receptance harmonic balance technique for the computation of the vibration of a whole aero-engine model with nonlinear bearings. J. Sound Vib. 324(1–2), 221–242 (2009). https://doi.org/10.1016/j.jsv.2009.01.039

    Article  Google Scholar 

  12. Inayat-Hussain, J.I.: Bifurcations in the response of a flexible rotor in squeeze-film dampers with retainer springs. Chaos Solitons Fract. 39(2), 519–532 (2009). https://doi.org/10.1016/j.chaos.2007.01.086

    Article  Google Scholar 

  13. Chu, F.L., Holmes, R.: The effect of squeeze film damper parameters on the unbalance response and stability of a flexible rotor. J. Eng. Gas Turbines Power 120(1), 140–148 (1996). https://doi.org/10.1115/1.2818065

    Article  Google Scholar 

  14. Chu, F.L., Holmes, R.: Efficient computation on nonlinear responses of a rotating assembly incorporating the squeeze-film damper. Comput. Methods Appl. Mech. Eng. 164(3), 363–373 (1998). https://doi.org/10.1016/S0045-7825(98)00097-8

    Article  MATH  Google Scholar 

  15. Chu, F.L., Holmes, R.: The damping capacity of the squeeze film damper in suppressing vibration of a rotating assembly. Tribol. Int. 33(2), 81–97 (2000). https://doi.org/10.1016/S0301-679X(00)00030-X

    Article  Google Scholar 

  16. Wang, S.J., Liao, M.F., Li, W.: Vibration characteristics of squeeze film damper during maneuver flight. Int. J. Turbo Jet-Eng. 32(2), 193–197 (2015). https://doi.org/10.1515/tjj-2014-0020

    Article  Google Scholar 

  17. Shoyama, T.: Nonlinear vibration of saturated water journal bearing and bifurcation analysis. J. Vib. Acoust. Trans. ASMEe 141(2), 021016 (2019). https://doi.org/10.1115/1.4042041

    Article  Google Scholar 

  18. Soni, A.H., Srinivasan, V.: Seismic analysis of a gyroscopic mechanical system. J. Vib. Acoust. Stress Reliab. Design Trans. ASME 105(4), 449–455 (1983). https://doi.org/10.1115/1.3269127

    Article  Google Scholar 

  19. Srinivasan, V., Soni, A.H.: Seismic analysis of a rotor-bearing system. Earthq. Eng. Struct. Dyn. 12(3), 287–311 (1984). https://doi.org/10.1002/eqe.4290120302

    Article  Google Scholar 

  20. Samali, B., Kim, K.B., Yang, J.N.: Random vibration of rotating machines under earthquake excitations. J. Eng. Mech ASCE 112(6), 550–565 (1986). https://doi.org/10.1061/(asce)0733-9399(1986)112:6(550)

    Article  Google Scholar 

  21. Kim, K.B., Yang, J.N., Lin, Y.K.: Stochastic response of flexible rotor-bearing systemto seismic excitations. Probab. Eng. Mech. 1(3), 122–130 (1986). https://doi.org/10.1016/0266-8920(86)90021-4

    Article  Google Scholar 

  22. Suarez, L.E., Singh, M.P., Rohanimanesh, M.S.: Seismic response of rotating machines. Earthq. Eng. Struct. Dyn. 21(1), 21–36 (1992). https://doi.org/10.1002/eqe.4290210102

    Article  Google Scholar 

  23. Lund, J.W.: Modal response of a flexible rotor in fluid-film bearings. J. Eng. Ind. Trans. ASME 96(2), 525–533 (1974). https://doi.org/10.1115/1.3438360

    Article  Google Scholar 

  24. Koike, H., Ishihara, K.: Impact response of rotor-bearing system to an arbitrary excitation of pedestals (1st report: comparison of linear analysis with experiment). Bull. JSME 26(220), 1783–1790 (1983). https://doi.org/10.1299/jsme1958.26.1783

    Article  Google Scholar 

  25. Koike, H., Ishihara, K.: Impact response of rotor-bearing system to an arbitrary excitation of pedestals (2nd report: experiments on rotor vibration due to various types of excitation). Bull. JSME 27(233), 2506–2513 (1984). https://doi.org/10.1299/jsme1958.27.2506

    Article  Google Scholar 

  26. Sakata, M., Kimura, K., Okamoto, S., Oikawa, K.: Vibration analysis of a high speed and light weight rotor system subjected to a pitching or turning motion, I: a rigid rotor system on flexible suspensions. J. Sound Vib. 184(5), 871–885 (1995). https://doi.org/10.1006/jsvi.1995.0350

    Article  MATH  Google Scholar 

  27. Okamoto, S., Sakata, M., Kimura, K., Ohnabe, H.: Vibration analysis of a high speed and light weight rotor system subjected to a pitching or turning motion, II: a flexible rotor system on flexible suspensions. J. Sound Vib. 184(5), 887–906 (1995). https://doi.org/10.1006/jsvi.1995.0350

    Article  MATH  Google Scholar 

  28. Ge, Z.M., Chen, H.H.: Bifurcations and chaos in a rate gyro with harmonic excitation. J. Sound Vib. 194(1), 107–117 (1996). https://doi.org/10.1006/jsvi.1996.0348

    Article  Google Scholar 

  29. Lee, A.S., Kim, B.O., Kim, Y.C.: A finite element transient response analysis method of a rotor-bearing system to base shock excitations using the state-space Newmark scheme and comparisons with experiments. J. Sound Vib. 297(3–5), 595–615 (2006). https://doi.org/10.1016/j.jsv.2006.04.028

    Article  Google Scholar 

  30. Lee, A.S., Kim, B.O.: A FE transient response analysis model of a flexible rotor-bearing system with mount system to base shock excitation. In: Proceedings of the ASME TURBO EXPO 2007: Power for Land, Sea, and Air, Montreal, Canada. American Society of Mechanical Engineers, pp. 903–912 (2007). https://doi.org/10.1115/GT2007-27677

  31. Bachelet, L., Driot, N., Ferraris, G., Poirion, F.: Dynamical behavior of a rotor under rotational random base excitation. In: Proceedings of the ASME 2007 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Las Vegas, 2007. American Society of Mechanical Engineers, pp. 1251–1260 (2007). https://doi.org/10.1115/DETC2007-34799

  32. Duchemin, M., Berlioz, A., Ferraris, G.: Dynamic behavior and stability of a rotor under base excitation. J. Vib. Acoust. Trans. ASME 128(5), 576–585 (2006). https://doi.org/10.1115/1.2202159

    Article  Google Scholar 

  33. Driot, N., Lamarque, C.H., Berlioz, A.: Theoretical and experimental analysis of a base-excited rotor. J. Comput. Nonlinear Dyn. 1(3), 257–263 (2006). https://doi.org/10.1115/1.2209648

    Article  Google Scholar 

  34. El-Saeidy, F.A., Sticher, F.: Dynamics of a rigid rotor linear/nonlinear bearings system subject to rotating unbalance and base excitations. J. Vib. Control 16(3), 403–438 (2010). https://doi.org/10.1177/1077546309103565

    Article  MATH  Google Scholar 

  35. Das, A.S., Nighil, M.C., Dutt, J.K., Irretier, H.: Vibration control and stability analysis of rotor-shaft system with electromagnetic exciters. Mech. Mach. Theory 43(10), 1295–1316 (2008). https://doi.org/10.1016/j.mechmachtheory.2007.10.007

    Article  MATH  Google Scholar 

  36. Das, A.S., Dutt, J.K., Ray, K.: Active vibration control of unbalanced flexible rotor-shaft systems parametrically excited due to base motion. Appl. Math. Model. 34(9), 2353–2369 (2010). https://doi.org/10.1016/j.apm.2009.11.002

    Article  MathSciNet  MATH  Google Scholar 

  37. Das, A.S., Dutt, J.K., Ray, K.: Active control of coupled flexural-torsional vibration in a flexible rotor-bearing system using electromagnetic actuator. Int. J. Non-Linear Mech. 46(9), 1093–1109 (2011). https://doi.org/10.1016/j.ijnonlinmec.2011.03.005

    Article  Google Scholar 

  38. Soni, T., Das, A.S., Dutt, J.K.: Active vibration control of ship mounted flexible rotor-shaft-bearing system during seakeeping. J. Sound Vib. 467, 25 (2020). https://doi.org/10.1016/j.jsv.2019.115046

    Article  Google Scholar 

  39. Sun, K.K., Qiu, J.B., Karimi, H.R., Gao, H.J.: A novel finite-time control for nonstrict feedback saturated nonlinear systems with tracking error constraint. IEEE Trans. Syst. Man Cybern. Syst. (2019). https://doi.org/10.1109/TSMC.2019.2958072

    Article  Google Scholar 

  40. Sun, K.K., Liu, L., Qiu, J.B., Feng, G.: Fuzzy adaptive finite-time fault-tolerant control for strict-feedback nonlinear systems. IEEE Trans. Fuzzy Syst (2020). https://doi.org/10.1109/TFUZZ.2020.2965890

    Article  Google Scholar 

  41. Sun, K.K., Qiu, J.B., Karimi, H.R., Fu, Y.L.: Event-triggered robust fuzzy adaptive finite-time control of nonlinear systems with prescribed performance. IEEE Trans. Fuzzy Syst. (2020). https://doi.org/10.1109/TFUZZ.2020.2979129

    Article  Google Scholar 

  42. Han, Q.K., Chu, F.L.: Parametric instability of flexible rotor-bearing system under time-periodic base angular motions. Appl. Math. Model. 39(15), 4511–4522 (2015). https://doi.org/10.1016/j.apm.2014.10.064

    Article  MathSciNet  MATH  Google Scholar 

  43. Chen, L.Q., Wang, J.J., Han, Q.K., Chu, F.L.: Nonlinear dynamic modeling of a simple flexible rotor system subjected to time-variable base motions. J. Sound Vib. 404, 58–83 (2017). https://doi.org/10.1016/j.jsv.2017.05.032

    Article  Google Scholar 

  44. Yi, Y.W., Qiu, Z.Y., Han, Q.K.: The effect of time-periodic base angular motions upon dynamic response of asymmetric rotor systems. Adv. Mech. Eng. 10(3), 12 (2018). https://doi.org/10.1177/1687814018767172

    Article  Google Scholar 

  45. Dakel, M., Baguet, S., Dufour, R.: Steady-state dynamic behavior of an on-board rotor under combined base motions. J. Vib. Control 20(15), 2254–2287 (2014a). https://doi.org/10.1177/1077546313483791

    Article  Google Scholar 

  46. Dakel, M.Z., Baguet, S., Dufour, R.: Bifurcation analysis of a non-linear on-board rotor-bearing system. In: Proceedings of the ASME 2014 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Buffalo, 2014. American Society of Mechanical Engineers, pp. 795–805. https://doi.org/10.1115/DETC2014-34352

  47. Dakel, M., Baguet, S., Dufour, R.: Nonlinear dynamics of a support-excited flexible rotor with hydrodynamic journal bearings. J. Sound Vib. 333(10), 2774–2799 (2014b). https://doi.org/10.1016/j.jsv.2013.12.021

    Article  Google Scholar 

  48. Briend, Y., Dakel, M., Chatelet, E., Andrianoely, M.A., Dufour, R., Baudin, S.: Effect of multi-frequency parametric excitations on the dynamics of on-board rotor-bearing systems. Mech. Mach. Theory 145, 1–31 (2020). https://doi.org/10.1016/j.mechmachtheory.2019.103660

    Article  Google Scholar 

  49. Wang, R., Guo, X.L., Wang, Y.F.: Nonlinear analysis of rotor system supported by oil lubricated bearings subjected to base movements. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 230(4), 543–558 (2016). https://doi.org/10.1177/0954406215578704

    Article  Google Scholar 

  50. Liu, Z.X., Liu, Z.S., Li, Y., Zhang, G.H.: Dynamics response of an on-board rotor supported on modified oil-film force considering base motion. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 232(2), 245–259 (2018). https://doi.org/10.1177/0954406216682052

    Article  Google Scholar 

  51. Greenhill, L.M., Nelson, H.D.: Iterative determination of squeeze film damper eccentricity for flexible rotor systems. J. Mech. Des. 104(2), 334–338 (1982). https://doi.org/10.1115/1.3256348

    Article  Google Scholar 

  52. Chen, X., Liao, M.F.: Transient characteristics of a dual-rotor system with intershaft bearing subjected to mass unbalance and base motions during start-up. In: Proceeding of the ASME Turbo Expo 2018: Turbomachinery Technical Conference and Exposition, Oslo, Norway, 2018. American Society of Mechanical Engineers, https://doi.org/10.1115/GT2018-75227

Download references

Acknowledgements

The authors are grateful for the support from Aerospace Propulsion Institute and Advanced Technology Institute at SUSTech. This research is technically supported by Center for Computational Science and Engineering at Southern University of Science and Technology.

Author information

Authors and Affiliations

  1. Department of Mechanics and Aerospace Engineering, Southern University of Science and Technology, Shenzhen, 518055, China

    Xi Chen, Xiaohua Gan & Guangming Ren

Authors
  1. Xi Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xiaohua Gan
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Guangming Ren
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xi Chen.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix 1

Generalized inertia matrices for disk element:

$$ \varvec{M}_{{{\text{t}},{\text{d}}}} = \left[ {\begin{array}{*{20}c} {m_{{\mathrm{d}}} } \\ 0 \\ 0 \\ 0 \\ \end{array} \begin{array}{*{20}c} 0 \\ {m_{{\mathrm{d}}} } \\ 0 \\ 0 \\ \end{array} \begin{array}{*{20}c} 0 \\ 0 \\ 0 \\ 0 \\ \end{array} \begin{array}{*{20}c} 0 \\ 0 \\ 0 \\ 0 \\ \end{array} } \right], {{\varvec{M}}}_{\mathrm{r},\mathrm{d}}=\left[\begin{array}{c}0\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{d}}\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{d}}\end{array}\right];$$

Generalized gyroscopic matrix and its component for disk element:

$${{\varvec{G}}}_{\mathrm{d}}=\left[\begin{array}{c}0\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{p}}\end{array} \begin{array}{c}0\\ 0\\ {-I}_{\mathrm{d}}^{\mathrm{p}}\\ 0\end{array}\right], \quad {{\varvec{H}}}_{\mathrm{d}}=\left[\begin{array}{c}0\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{p}}\end{array} \begin{array}{c}0\\ 0\\ 0\\ 0\end{array}\right]$$

where \({{\varvec{G}}}_{\mathrm{d}}={{\varvec{H}}}_{\mathrm{d}}-{{\varvec{H}}}_{\mathrm{d}}^{\mathrm{T}}\);

Parametric damping matrix, i.e., Coriolis effect matrix, induced by base motions for disk element:

$$ ~\varvec{C}_{{{\text{b}}x,{\text{d}}}} = \left[ {\begin{array}{*{20}c} 0 \\ {2m_{{\mathrm{d}}} } \\ 0 \\ 0 \\ \end{array} ~~\begin{array}{*{20}c} { - 2m_{{\mathrm{d}}} } \\ 0 \\ 0 \\ 0 \\ \end{array} ~~\begin{array}{*{20}c} 0 \\ 0 \\ 0 \\ { - \left( {I_{{\mathrm{d}}}^{{\text{p}}} - 2I_{{\mathrm{d}}}^{{\text{d}}} } \right)} \\ \end{array} ~~\begin{array}{*{20}c} 0 \\ 0 \\ {\left( {I_{{\mathrm{d}}}^{{\text{p}}} - 2I_{{\mathrm{d}}}^{{\text{d}}} } \right)} \\ 0 \\ \end{array} } \right] $$

Parametric stiffness matrix induced by base motions for disk element:

$$\begin{aligned}& \varvec{K}_{{{\text{a}},{\text{d}}}} = \left[ {\begin{array}{*{20}c} 0 \\ {m_{{\mathrm{d}}} } \\ 0 \\ 0 \\ \end{array} ~~\begin{array}{*{20}c} { - m_{{\mathrm{d}}} } \\ 0 \\ 0 \\ 0 \\ \end{array} ~~\begin{array}{*{20}c} 0 \\ 0 \\ 0 \\ {I_{{\mathrm{d}}}^{{\text{d}}} } \\ \end{array} ~~\begin{array}{*{20}c} 0 \\ 0 \\ { - I_{{\mathrm{d}}}^{{\text{d}}} } \\ 0 \\ \end{array} } \right] , \quad {{\varvec{K}}}_{\mathrm{r},\mathrm{d}}=\left[\begin{array}{c}0\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{p}}\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{p}}\end{array}\right] \\ &{{\varvec{K}}}_{\mathrm{b}x,\mathrm{d}}=\left[\begin{array}{c}{m}_{\mathrm{d}}\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ {m}_{\mathrm{d}}\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{d}}-{I}_{\mathrm{d}}^{\mathrm{p}}\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{d}}-{I}_{\mathrm{d}}^{\mathrm{p}}\end{array}\right],\end{aligned}$$
$$\begin{aligned} & \varvec{K}_{{{\text{b}}y,{\text{d}}}} = \left[ {\begin{array}{*{20}c} 0 \\ 0 \\ 0 \\ 0 \\ \end{array} ~~\begin{array}{*{20}c} 0 \\ {m_{{\mathrm{d}}} } \\ 0 \\ 0 \\ \end{array} ~~\begin{array}{*{20}c} 0 \\ 0 \\ 0 \\ 0 \\ \end{array} ~~\begin{array}{*{20}c} 0 \\ 0 \\ 0 \\ {I_{{\mathrm{d}}}^{{\text{p}}} - I_{{\mathrm{d}}}^{{\text{d}}} } \\ \end{array} } \right] , \quad {{\varvec{K}}}_{\mathrm{b}z,\mathrm{d}}=\left[\begin{array}{c}{m}_{\mathrm{d}}\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{p}}-{I}_{\mathrm{d}}^{\mathrm{d}}\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ 0\end{array}\right]\\ &{{\varvec{K}}}_{\mathrm{b}yz,\mathrm{d}}=\left[\begin{array}{c}0\\ {m}_{\mathrm{d}}\\ 0\\ 0\end{array} \begin{array}{c}{m}_{\mathrm{d}}\\ 0\\ 0\\ 0\end{array} \begin{array}{c}0\\ 0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{p}}-{I}_{\mathrm{d}}^{\mathrm{d}}\end{array} \begin{array}{c}0\\ 0\\ {I}_{\mathrm{d}}^{\mathrm{p}}-{I}_{\mathrm{d}}^{\mathrm{d}}\\ 0\end{array}\right]\end{aligned};$$

External force vectors caused by base motions for disk element:

$$ \varvec{S}_{{V,{\text{d}}}} = \left\{ {\begin{array}{*{20}c} {m_{{\mathrm{d}}} } & \quad 0 & \quad 0 & \quad 0 \\ \end{array} } \right\}^{{\text{T}}} , \quad {{\varvec{S}}}_{W,\mathrm{d}}={\left\{\begin{array}{cccc}0& \quad {m}_{\mathrm{d}}& \quad 0& \quad 0\end{array}\right\}}^{\mathrm{T}}$$
$$ \varvec{S}_{{{\rm B},{\text{d}}}} = \left\{ {\begin{array}{*{20}c} 0 & \quad 0 & \quad {I_{{\mathrm{d}}}^{{\text{p}}} } & \quad 0 \\ \end{array} } \right\}^{{\text{T}}} , \quad {{\varvec{S}}}_{\Gamma ,\mathrm{d}}={\left\{\begin{array}{cccc}0& \quad 0& \quad 0& \quad {I}_{\mathrm{d}}^{\mathrm{p}}\end{array}\right\}}^{\mathrm{T}}$$
$$ \varvec{S}_{{V\Gamma ,{\text{d}}}} = \left\{ {\begin{array}{*{20}c} {m_{{\mathrm{d}}} x_{{\mathrm{d}}} } & \quad 0 & \quad 0 & \quad {I_{{\mathrm{d}}}^{{\text{d}}} } \\ \end{array} } \right\}^{{\text{T}}} , \quad {{\varvec{S}}}_{W{\rm B},\mathrm{d}}={\left\{\begin{array}{cccc}0& \quad {m}_{\mathrm{d}}{x}_{\mathrm{d}}& \quad -{I}_{\mathrm{d}}^{\mathrm{d}}& \quad 0\end{array}\right\}}^{\mathrm{T}}$$

Force coefficients caused by base motions for disk element:

$$ f_{V} = \ddot{y}_{{\mathrm{b}}} + \omega _{{{\text{b}}z}} \dot{x}_{{\mathrm{b}}} - \omega _{{{\text{b}}x}} \dot{z}_{{\mathrm{b}}}, \quad {f}_{W}={\ddot{z}}_{\mathrm{b}}+{\omega }_{\mathrm{b}x}{\dot{y}}_{\mathrm{b}}-{\omega }_{\mathrm{b}y}{\dot{x}}_{\mathrm{b}}$$

Appendix 2

Translational shape function matrix:

$${{\varvec{N}}}_{\mathrm{t}}=\left[\begin{array}{c}{\theta }_{1}\\ 0\end{array} \begin{array}{l}0\\ {\theta }_{1}\end{array} \begin{array}{l}0\\ -{\theta }_{2}\end{array} \begin{array}{l}{\theta }_{2}\\ 0\end{array} \begin{array}{l}{\theta }_{3}\\ 0\end{array} \begin{array}{l}0\\ {\theta }_{3}\end{array} \begin{array}{l}0\\ -{\theta }_{4}\end{array} \begin{array}{l}{\theta }_{4}\\ 0\end{array}\right], \quad \left\{\begin{array}{l}{\theta }_{1}=\left[1-3{\upsilon }^{2}+2{\upsilon }^{3}+\varPhi \left(1-\upsilon \right)\right]/\left(1+\varPhi \right)\\ {\theta }_{2}=\left[\left(\upsilon -2{\upsilon }^{2}+{\upsilon }^{3}\right)l+\varPhi l\left(\upsilon -{\upsilon }^{2}\right)/2\right]/\left(1+\varPhi \right)\\ {\theta }_{3}=\left[3{\upsilon }^{2}-2{\upsilon }^{3}+\varPhi \upsilon \right]/\left(1+\varPhi \right)\\ {\theta }_{4}=\left[\left(-{\upsilon }^{2}+{\upsilon }^{3}\right)l-\varPhi l\left(\upsilon -{\upsilon }^{2}\right)/2\right]/\left(1+\varPhi \right)\end{array}\right.;$$

Rotational shape function matrix:

$$ {\varvec{N}}_{{\mathrm{r}}} = \left[ {\begin{array}{*{20}c} 0 \\ {\varphi_{1} } \\ \end{array} \begin{array}{*{20}c} { - \varphi_{1} } \\ 0 \\ \end{array} \begin{array}{*{20}c} {\varphi_{2} } \\ 0 \\ \end{array} \begin{array}{*{20}c} 0 \\ {\varphi_{2} } \\ \end{array} \begin{array}{*{20}c} 0 \\ {\varphi_{3} } \\ \end{array} \begin{array}{*{20}c} { - \varphi_{3} } \\ 0 \\ \end{array} \begin{array}{*{20}c} {\varphi_{4} } \\ 0 \\ \end{array} \begin{array}{*{20}c} 0 \\ {\varphi_{4} } \\ \end{array} } \right],\quad \left\{ {\begin{array}{*{20}c} { \varphi_{1} = \left( {6\upsilon^{2} - 6\upsilon } \right)/\left[ {l\left( {1 + \varPhi } \right)} \right]} \\ {\varphi_{2} = \left[ {\left( {1 - 4\upsilon + 3\upsilon^{2} } \right) + {\varPhi }\left( {1 - \upsilon } \right)} \right]/\left[ {\left( {1 + \varPhi } \right)} \right]} \\ { \varphi_{3} = \left( { - 6\upsilon^{2} + 6\upsilon } \right)/\left[ {l\left( {1 + \varPhi } \right)} \right]} \\ { \varphi_{4} = \left( {3\upsilon^{2} - 2\upsilon + {\varPhi }\upsilon } \right)/\left[ {\left( {1 + \varPhi } \right)} \right]} \\ \end{array} } \right.; $$

Shear shape function matrix:

$$ {\varvec{B}}_{{\mathrm{s}}} = \left[ {\begin{array}{*{20}c} {\zeta_{1} } \\ 0 \\ \end{array} \begin{array}{*{20}c} 0 \\ {\zeta_{1} } \\ \end{array} \begin{array}{*{20}c} 0 \\ { - \zeta_{2} } \\ \end{array} \begin{array}{*{20}c} {\zeta_{2} } \\ 0 \\ \end{array} \begin{array}{*{20}c} {\zeta_{3} } \\ 0 \\ \end{array} \begin{array}{*{20}c} 0 \\ {\zeta_{3} } \\ \end{array} \begin{array}{*{20}c} 0 \\ { - \zeta_{4} } \\ \end{array} \begin{array}{*{20}c} {\zeta_{4} } \\ 0 \\ \end{array} } \right],\quad \left\{ {\begin{array}{*{20}c} { \zeta_{1} = - \varPhi /\left[ {l\left( {1 + \varPhi } \right)} \right]} \\ { \zeta_{2} = - \varPhi /\left[ {2\left( {1 + \varPhi } \right)} \right]} \\ { \zeta_{3} = \varPhi /\left[ {l\left( {1 + \varPhi } \right)} \right]} \\ { \zeta_{4} = - \varPhi /\left[ {2\left( {1 + \varPhi } \right)} \right]} \\ \end{array} } \right.; $$

Generalized inertia matrices for shaft element:

$$ {\varvec{M}}_{{{\text{t}},{\text{sh}}}}^{{\text{e}}} = \rho A \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s){\varvec{N}}_{{\mathrm{t}}} (s){\text{d}}s,\quad {\varvec{M}}_{{{\text{r}},{\text{sh}}}}^{{\text{e}}} = \rho I_{{{\text{sh}}}}^{{\text{d}}} \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s){\varvec{N}}_{{\mathrm{r}}} (s){\text{d}}s; $$

Generalized gyroscopic matrix and its component for shaft element:

$$ {\varvec{G}}_{{{\text{sh}}}}^{{\text{e}}} = \rho I_{{{\text{sh}}}}^{{\text{p}}} \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 0 & \quad { - 1} \\ 1 & \quad 0 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{r}}} (s){\text{d}}s,\quad {\varvec{H}}_{{{\text{sh}}}}^{{\text{e}}} = \rho I_{{{\text{sh}}}}^{{\text{p}}} \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 0 & \quad 0 \\ 1 & \quad 0 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{r}}} (s){\text{d}}s; $$

Parametric damping matrix, i.e., Coriolis effect matrix, induced by base motions for shaft element:

\({\varvec{C}}_{{{\text{b}}x,{\text{sh}}}}^{{\text{e}}} = 2\rho A \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 0 & \quad { - 1} \\ 1 & \quad 0 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{t}}} (s){\text{d}}s - \rho \left( {I_{{{\text{sh}}}}^{{\text{p}}} - 2I_{{{\text{sh}}}}^{{\text{d}}} } \right) \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 0 & \quad { - 1} \\ 1 & \quad 0 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{r}}} (s){\text{d}}s\);

Parametric stiffness matrix induced by base motions for shaft element:

$$ \begin{aligned} & {\varvec{K}}_{{{\text{a}},{\text{sh}}}}^{{\text{e}}} = \rho A \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 0 & \quad { - 1} \\ 1 & \quad 0 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{t}}} (s){\text{d}}s + \rho I_{{{\text{sh}}}}^{{\text{d}}} \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 0 & \quad { - 1} \\ 1 & \quad 0 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{r}}} (s){\text{d}}s, \\ & {\varvec{K}}_{{{\text{r}},{\text{sh}}}}^{{\text{e}}} = \rho I_{{{\text{sh}}}}^{{\text{p}}} \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left[ {{\varvec{N}}_{{\mathrm{r}}} (s)} \right]{\text{d}}s, \\ & {\varvec{K}}_{{{\text{b}}x,{\text{sh}}}}^{{\text{e}}} = \rho A \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s){\varvec{N}}_{{\mathrm{t}}} (s){\text{d}}s - \rho \left( {I_{{{\text{sh}}}}^{{\text{p}}} - I_{{{\text{sh}}}}^{{\text{d}}} } \right) \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s){\varvec{N}}_{{\mathrm{r}}} (s){\text{d}}s, \\ & {\varvec{K}}_{{{\text{b}}y,{\text{sh}}}}^{{\text{e}}} = \rho A \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 0 & \quad 0 \\ 0 & \quad 1 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{t}}} (s){\text{d}}s + \rho \left( {I_{{{\text{sh}}}}^{{\text{p}}} - I_{{{\text{sh}}}}^{{\text{d}}} } \right) \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 0 & \quad 0 \\ 0 & \quad 1 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{r}}} (s){\text{d}}s, \\ & \quad {\varvec{K}}_{{{\text{b}}z,{\text{sh}}}}^{{\text{e}}} = \rho A \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 1 & \quad 0 \\ 0 & \quad 0 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{t}}} (s){\text{d}}s + \rho \left( {I_{{{\text{sh}}}}^{{\text{p}}} - I_{{{\text{sh}}}}^{{\text{d}}} } \right) \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 1 & \quad 0 \\ 0 & \quad 0 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{r}}} (s){\text{d}}s, \\ & {\varvec{K}}_{{{\text{b}}yz,{\text{sh}}}}^{{\text{e}}} = \rho A \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 0 & \quad 1 \\ 1 & \quad 0 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{t}}} (s){\text{d}}s + \rho \left( {I_{{{\text{sh}}}}^{{\text{p}}} - I_{{{\text{sh}}}}^{{\text{d}}} } \right) \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left[ {\begin{array}{*{20}c} 0 & \quad 1 \\ 1 & \quad 0 \\ \end{array} } \right]{\varvec{N}}_{{\mathrm{r}}} (s){\text{d}}s; \\ \end{aligned} $$

Bending and shear stiffness matrix for shaft element:

$$ \left[ {{\varvec{K}}_{{\mathrm{B}}} } \right]_{{{\text{sh}}}}^{{\text{e}}} = EI_{{{\text{sh}}}}^{{\text{d}}} \int \limits_{0}^{l} \left[ {{\varvec{N}}_{{\mathrm{r}}}^{\prime} (s)} \right]^{{\text{T}}} {\varvec{N}}_{{\mathrm{r}}}^{\prime} (s){\text{d}}s + \kappa GA \int \limits_{0}^{l} {\varvec{B}}_{{\mathrm{s}}}^{{\text{T}}} (s){\varvec{B}}_{{\mathrm{s}}} (s){\text{d}}s; $$

External force vectors caused by base motions for shaft element:

$$ \begin{aligned} & {\varvec{S}}_{{V,{\text{sh}}}}^{{\text{e}}} = \rho A \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s)\left\{ {\begin{array}{*{20}c} 1 \\ 0 \\ \end{array} } \right\}{\text{d}}s,\quad {\varvec{S}}_{{W,{\text{sh}}}}^{{\text{e}}} = \rho A \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s)\left\{ {\begin{array}{*{20}c} 0 \\ 1 \\ \end{array} } \right\}{\text{ds}}, \\ & {\varvec{S}}_{{{\rm B},{\text{sh}}}}^{{\text{e}}} = \rho I_{{{\text{sh}}}}^{{\text{p}}} \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left\{ {\begin{array}{*{20}c} 1 \\ 0 \\ \end{array} } \right\}{\text{d}}s,\quad {\varvec{S}}_{{\Gamma ,{\text{sh}}}}^{{\text{e}}} = \rho I_{{{\text{sh}}}}^{{\text{p}}} \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left\{ {\begin{array}{*{20}c} 0 \\ 1 \\ \end{array} } \right\}{\text{d}}s, \\ & {\varvec{S}}_{{V\Gamma ,{\text{sh}}}}^{{\text{e}}} = \rho A \int \limits_{0}^{l} x{\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s)\left\{ {\begin{array}{*{20}c} 1 \\ 0 \\ \end{array} } \right\}{\text{d}}s + \rho I_{{{\text{sh}}}}^{{\text{d}}} \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left\{ {\begin{array}{*{20}c} 0 \\ 1 \\ \end{array} } \right\}{\text{d}}s, \\ & {\varvec{S}}_{{W{\rm B},{\text{sh}}}}^{{\text{e}}} = \rho A \int \limits_{0}^{l} x{\varvec{N}}_{{\mathrm{t}}}^{{\text{T}}} (s)\left\{ {\begin{array}{*{20}c} 0 \\ 1 \\ \end{array} } \right\}{\text{d}}s - \rho I_{{{\text{sh}}}}^{{\text{d}}} \int \limits_{0}^{l} {\varvec{N}}_{{\mathrm{r}}}^{{\text{T}}} (s)\left\{ {\begin{array}{*{20}c} 1 \\ 0 \\ \end{array} } \right\}{\text{d}}s \\ \end{aligned} $$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chen, X., Gan, X. & Ren, G. Nonlinear responses and bifurcations of a rotor-bearing system supported by squeeze-film damper with retainer spring subjected to base excitations. Nonlinear Dyn 102, 2143–2177 (2020). https://doi.org/10.1007/s11071-020-06052-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-020-06052-0

Keywords

Search

Navigation