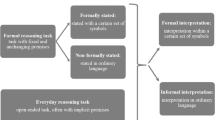
Abstract
In current philosophy of information, different authors have been supporting the veridicality thesis (VT). According to this thesis, an epistemically-oriented concept of information must have truth as one of its necessary conditions. Two challenges can be raised against VT. First, some philosophers object that veridicalists erroneously ignore the informativeness of false messages. Secondly, it is not clear whether VT can adequately explain the information considered in hypothetical reasoning. In this sense, logical diagrams offer an interesting case of analysis: by manipulating a logical diagram we can verify that a certain conclusion follows from a set of premises, but it cannot help us to determine the actual truth-value of a given set of propositions. Focusing on the latter challenge, in this paper I claim that logical diagrams set out potential counterexamples to VT and, consequently, pose a real challenge to this thesis. First, a veridicalist analysis of logical diagrams requires the assumption of metatheoretical properties which are not satisfied by some logical systems (and, consequently, are not satisfied by some systems of logical diagrams). So, VT does not fit well as a general framework for a theory of logical diagrammatic information. Secondly, based on semantic inferentialism, one can propose a normative interpretation of the inferential content of logical diagrams not exposed to the problems faced by VT. Moreover, there are several reasons to believe that veridicalism cannot accommodate such a normative interpretation. In other words, normativism represents a real (though still underexplored) alternative to veridicality. Due to these reasons, I conclude that, until further research, we should adopt a more parsimonious standpoint and say that logical diagrams provide inferential information simply.









Similar content being viewed by others
Availability of Data and Materials
Not applicable.
Code Availability
Not applicable.
Notes
There is the possibility (still non-explored, as far as I know) that the false information problem as formulated above is just a particular topic within a larger class of problems. Let us assume that ‘message x is true’ means ‘message x was in fact produced by our informational source u.’ Then, we have four possibilities: (i) x is true and p is true; (ii) x is false and p is false; (iii) x is false and p is true; (iv) x is true and p is false. Now, (i) is just veridical information as described by VT and (ii) is false information in the sense highlighted by Fetzer (2004). (iii) is a case analyzed by Dretske (1981, pp. 96–ff.) in which u does not produce x, but the receiver of the message believes otherwise and, irrespective of this, the content p of x is in fact true. This informational scenario is closely tied to Gettier (1963) cases and we have good reasons to believe that it is not epistemically informational. Finally, case (iv) is trickier: it seems to be informational, but it doesn’t portray a homogeneous set of cases. For example, (iv) collects both conventions as well as fictional facts (bear in mind all the information that we have on Sherlock Holmes’ biography). These are, arguably, different kinds of information capable of grounding distinct kinds of knowledge. So, the problem of the informativeness of (iv) is obscure in the sense that it wrongly collapses disparate questions. I want to thank an anonymous referee for drawing my attention to this issue.
One could object that logical diagrams are not a source of information of any kind since they do not display causal connections. However, even though these diagrammatic systems do not furnish causal information, they still play some informational role: they signalize a logical relation between premises and conclusion. In fact, information does not need to be causal, as Dretske (1981, pp. 26–ff.) famously stressed: there are cases in which we have information without causality, and vice versa. Logical diagrams put forward another example of this situation.
Notwithstanding, one could inquire whether the hypothetical information generated by logical diagrams might include probabilistic elements such as those taken into account in Scarantino and Piccinini’s probabilistic veridicalism. Now, although logical diagrams in general provide information on a strongly deterministic concept of inference, on the other hand, it is possible to improve these representational systems in order to depict some kind of probabilistic reasoning. For more details, see Radakovic and McDougall (2012) and Chow and Ruskey (2003).
Additionally, perhaps mathematical figures, diagrammatic representations which also seem to offer an informational support for hypothetical reasoning, could render further counterexamples to VT. For instance, the proofs by reductio in Euclid’s Elements (e.g., Euclid and Heath 1956, Book I, Proposition 6) are cases of diagrammatically informed hypothetical reasoning. Nevertheless, the question here is much more subtle. First, despite the important works on the rigorous use of diagrams in Euclidean geometry (Manders 2008), to mention a key example, it is still controversial whether figures can really play a justificatory role in mathematical proofs. Secondly, there is an open dispute between representationalist and antirepresentationalist stances on the semantics of mathematical figures. Thus, whereas Mander’s emphasizes that Euclidean reductio proofs highlight the supposed non-subrogative character of geometrical diagrams (Rabouin 2018, pp. 4773–ff.), on the other hand, Mortensen (2019) holds that, in the proof of contradiction of impossible figures (such as the Escher cube), the diagram seems to depict an impossible geometrical object. Consequently, the antirepresentationalist view suggested by Euclidean reductio proofs is not immediately generalizable for all uses of geometrical figures.
e.g., it is well-known that an unrestricted version of the deduction theorem does not hold in normal modal logics. However, some of these logics accept visual representation. For an example, see Braüner (1998).
Of course, Putnam is not an inferentialist, but the Twin Earth argument might reveal the sense in which, in line with inferentialism, a competent speaker can ignore the meaning of ‘water’. Indeed, inferentialist semantic theories generally characterize the inferentiality of a sentence not just in logical but also in material terms. In any case, in the context of an analysis of logical diagrams, we do not need to concern ourselves with material inferences, but only with the logical inferentiality of propositions.
One could raise the following question: is the sentential version of an argument as informative as its diagrammatic depiction? To properly answer this question, one should compare logical diagrams, logical symbolic systems and natural language. According to an influential tradition initiated by Leibniz (Casanave 2012), logical symbols play an ecthetic role similar to logical diagrams, that is, in accordance with this view, symbolic formulae enable us to visualize certain structural properties of its subject matter very much like diagrams and, consequently, are also a source of visual information. On the other hand, this feature seems to be entirely absent in natural language sentences. This is not a problem for semantic inferentialism. For the inferentialist, to understand a sentence is to understand its inferential network, but this does not mean that, by reading a natural language sentence we are informed (in an epistemically-oriented sense) about its set of inferential relations: a speaker can be linguistically competent even without knowing all the inferential facts about the sentences of her language.
My argument here against veridicalism displays some close connections with a recent paper by Field (2015). In this work, Field argues that it is preferable to elucidate inferential validity in normative rather than in truth-preserving terms, when it comes to defining a common ground for different logical systems and their respective conceptions of validity: ‘to regard an inference or argument as valid is (in large part anyway) to accept a constraint on belief: one that prohibits fully believing its premises without fully believing its conclusion’ (Field 2015, p. 42). That is, for him, to say that a set of premises \(\varGamma\) implies p is, more fundamentally, to say that the following is a valid norm of reasoning: If you assume \(\varGamma\), please assume p. (This is a simplification. To be precise, this norm is equivalent to Field’s analysis of inferential validity as a normative constraint on full belief, but he also introduces more sophisticated variations to capture the case of partial beliefs (Field 2015, pp. 45–ff.). We can disregard these complexities here). This is a quite strong normative conception of inference. As we will see in a moment, the inferentialist approach to semantics on which the present work relies gives place to a more modest conception. In any case, note that, from Field’s perspective, even though inferential validity may be connected to truth (and, more particularly, to truth-preservation), it is simply not definable in alethic terms.
To be clear on this point, note that inferentialism as well as a truth-conditional conception of meaning are both equally capable to provide an account of logic and semantics leading to the same results. One does not lose explanatory power by replacing the discourse on truth-conditions by an inferentialist understanding of the issue. The point is rather a foundational one: in the construction of a semantic theory, should we regard truth as a primitive semantic concept, or would it be better to examine alternatives such as inferentiality? For a complete survey of the philosophical reasons to embrace inferentialism, see Brandom (1998, Chap. 2). In this paper, I present an additional advantage of inferentialism towards truth-conditional semantics: inferentialism allows us to better understand the epistemic usefulness of logical diagrams. If the propositional content of a sentence is, more essentially, its inferential network, then, by depicting the inferentiality of a sentence, logical diagrams furnish conceptual elucidation.
One could, perhaps, suggest to split up the information produced by Venn diagrams into two parts, namely, a dynamic part that varies between informational contexts and a static one which remains fixed between different readings of the diagram. In fact, some authors have been very interested in the phenomenon of the informativeness of ambiguous sources. A particularly important proposal is info-metrics by Golan (2014, 2018), a theoretical framework for determining the output information of ambiguous (or, rather, incomplete, imperfect, noisy) messages. In this sense, arguably, by applying this theory one could elucidate the nature of the rigid (hard core, context independent) part of the inferential information delivered by Venn diagrams.
Of course, we can formalize the pragmatic norms governing diagrammatic practice into a set of explicitly stated transformation rules, as, for instance, Shin (1994) has done. Even so, one does not need to do it in order to competently use Venn diagrams. In this respect, there is no parallel between Venn diagrams and logical symbolic systems. In the latter, information extraction must always be justified by some set of written rules.
References
Barwise, J., & Etchemendy, J. (1990). Visual information and valid reasoning. Visualization in mathematics (pp. 9–24). Washington: Mathematical Association of America.
Brandom, R. (1998). Making it explicit: Reasoning, representing, and discursive commitment. Cambridge: Harvard University Press.
Brandom, R. (2008). Appendix to chapter V. Between saying and doing: Towards an analytic Pragmatism. Oxford: Oxford University Press.
Braüner, T. (1998). Peircean graphs for the modal logic S5. In International conference on conceptual structures (pp. 255–269). New York: Springer.
Casanave, A. L. (2012). Symbolic knowledge from Leibniz to Husserl. London: College Publications.
Chow, S., & Ruskey, F. (2003). Drawing area-proportional Venn and Euler diagrams. In International symposium on graph drawing (pp. 466–477). New York: Springer.
Dodig-Crnkovic, G. (2005). System modeling and information semantics. In Proceedings of the fifth promote IT conference. Lund: New Universities and University Colleges in Sweden.
Dretske, F. (1981). Knowledge and the flow of information. Stanford: CSLI Publications.
Esquisabel, O. (2012). Representing and abstracting: An analysis of Leibniz’s concept of symbolic knowledge. In A. L. Casanave (Ed.), Symbolic knowledge from Leibniz to Husserl (pp. 1–49). London: College Publications.
Euclid, & Heath T. (1956). The thirteen books of Euclid’s elements. North Chelmsfor: Courier Corporation.
Fetzer, J. (2004). Information: Does it have to be true? Minds and Machines, 14(2), 223–229.
Field, H. (2015). What is logical validity? Foundations of logical consequence (pp. 33–70). Oxford: Oxford University Press.
Floridi, L. (2004). Outline of a theory of strongly semantic information. Minds and Machines,14(2), 197–221.
Floridi, L. (2005). Is semantic information meaningful data? Philosophy and Phenomenological Research,70(2), 351–370.
Floridi, L. (2007). In defence of the veridical nature of semantic information. European Journal of Analytic Philosophy,3(1), 31–41.
Floridi, L. (2011). Semantic information and the correctness theory of truth. Erkenntnis,74(2), 147–175.
Frege, G. (1948). Sense and reference. The Philosophical Review,57(3), 209–230.
Gettier, E. (1963). Is knowledge justified true belief? Analysis,23(6), 121–123.
Giardino, V. (2013). A practice-based approach to diagrams. Visual reasoning with diagrams (pp. 135–151). New York: Springer.
Golan, A. (2014). Information dynamics. Minds and Machines,24(1), 19–36.
Golan, A. (2018). Foundations of info-metrics: Modeling, inference, and imperfect information. Oxford: Oxford University Press.
Goodman, N. (1976). Languages of art: An approach to a theory of symbols. Cambridge: Hackett publishing.
Keynes, J. N. (1906). Studies and exercises in formal logic: Including a generalisation of logical processes in their application to complex inferences. New York: Macmillan & Company.
Lance, M. (2001). The logical structure of linguistic commitment III: Brandomian scorekeeping and incompatibility. Journal of Philosophical Logic,30(5), 439–464.
Macbeth, D. (2009). Meaning, use, and diagrams. Etica & Politica,11(1), 369–384.
Mahmoodian, E. S. & Vatan, F. (1987). Generalization of Venn diagrams. In Eighteenth annual Iranian mathematics conference (pp. 154–161). Birjand: University of Birjand.
Manders, K. (2008). Diagram-based geometric practice. The philosophy of mathematical practice (pp. 65–79). Oxford: Oxford University Press.
Mortensen, C. (2019). Euclid’s geometry: The case of contradiction. Journal of Modern Greek. Special Issue ‘Greek Journeys and Philosophical Reflections’, 71.
Peregrin, J. (2010). Logic as based on incompatibility. The Logica yearbook (pp. 158–167). London: College Publications.
Peregrin, J. (2014). Inferentialism: Why rules matter. New York: Springer.
Rabouin, D. (2018). Logic of imagination. Echoes of Cartesian epistemology in contemporary philosophy of mathematics and beyond. Synthese,195(11), 4751–4783.
Radakovic, N., & McDougall, D. (2012). Using dynamic geometry software for teaching conditional probability with area-proportional Venn diagrams. International Journal of Mathematical Education in Science and Technology,43(7), 949–953.
Sautter, F. T. (2020). Quadraginta duo. Cognitio-Estudos,17(1), 118–127.
Scarantino, A., & Piccinini, G. (2010). Information without truth. Metaphilosophy,41(3), 313–330.
Sequoiah-Grayson, S. (2007). The metaphilosophy of information. Minds and Machines,17(3), 331–344.
Shimojima, A. (1999). The graphic-linguistic distinction exploring alternatives. Artificial Intelligence Review,13(4), 313–335.
Shin, S.-J. (1994). The logical status of diagrams. Cambridge: Cambridge University Press.
Shin, S.-J., Lemon, O., & Mumma, J. (2018). Diagrams. The Stanford encyclopedia of philosophy. Metaphysics Research Lab: Stanford University.
Tarski, A. (1956). The concept of truth in formalized languages. Logic, semantics, metamathematics (2nd ed., pp. 152–278). Oxford: Oxford University Press.
Venn, J. (1881). Symbolic logic. New York: B. Franklin.
Acknowledgements
I want to thank Frank Thomas Sautter, Tamires Dalmagro, Giorgio Venturi and all the members of the research group ‘Arbitrariness and Genericity’ (Fapesp/ Brazil) for suggestions on preliminary versions of this work.
Funding
The author is supported by a postdoc scholarship from Coordenação de Aperfeiçoamento de Pessoal de Nível Superior (CAPES/Brazil: project # 88887.475401/2020-00).
Author information
Authors and Affiliations
Contributions
Not applicable.
Corresponding author
Ethics declarations
Conflicts of Interest
The author declares no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Mendonça, B.R. Information and Diagrammatic Reasoning: An Inferentialist Reading. Minds & Machines 31, 99–120 (2021). https://doi.org/10.1007/s11023-020-09547-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11023-020-09547-2