Nonlinear radion interactions

Abstract

We consider the Randall–Sundrum model with two branes in which the fields of the Standard Model are localized on the brane with negative tension and the gravitational field and an additional stabilizing scalar Goldberg–Wise field propagate in the space between the branes. We construct a Lagrangian for scalar fluctuations of the gravitational and scalar fields against the background solution. We obtain an effective four-dimensional Lagrangian describing nonlinear self-coupling terms of the scalar radion field in a polynomial approximation up to the fourth order and also nonlinear terms of the coupling of the radion and Standard Model fields. We estimate possible values of the self-coupling constant.

A. Calculation of the coefficients of the polynomial expansion of the effective four-dimensional action of the radion field

We introduce the dimensionless quantities

$$\xi\equiv\frac{\mu}{ \tilde{k} },\qquad\sigma\equiv\frac{u}{ \tilde{k} },\qquad \rho\equiv\frac{ \varphi _0^2}{2M^3}. $$

(45)

We note that $\tilde{k}$ has the order of 1 to 10 TeV. Therefore, if the radion mass has the order of hundreds of GeV, then $\xi\sim0.1$ to 0.01 is a small parameter. In computing the four-dimensional Planck mass above, we assumed that $uL\ll1$. Because $\tilde{k} L\sim35$, $u/ \tilde{k}$ is also a small parameter.

It follows from the definition of $z$ and the background solution $\varphi$ that for $A(y)$ and $\varphi$, we have the relations

$$e^A=\xi^{-1}z,\qquad \varphi =e^{-uL}\xi^{\sigma} \varphi _0z^{-\sigma}. $$

(46)

It is easy to see that in accordance with (13),

$$\chi=B\frac{1}{\sqrt{8M^3}\xi^2}z^{1-\sigma}J_{\alpha}(z),\qquad \Phi=B\frac{3e^{uL}}{2\sqrt{\rho}\sigma\xi^{2+\sigma}} z[(\alpha+1+\sigma)J_{\alpha}(z)-zJ_{\alpha -1}(z)]. $$

(47)

The integral with an arbitrary function $f$ integrable at zero can be represented approximately as

$$\int_0^Lfe^{-2A}\,dy=\frac{\xi^2}{ \tilde{k} }\int_{\xi e^{- \tilde{k} L}}^{\xi}fz^{-3}\,dz \approx\frac{\xi^2}{ \tilde{k} }\int_0^{\xi}fz^{-3}\,dz. $$

(48)

We now calculate the normalizing factor $B$. We use the fact that $\alpha_0=1/2$:

$$\alpha_0\equiv\int_0^L\{6M^3\chi^2+\Phi^2\}e^{-2A}\,dy=\frac{1}{2}. $$

(49)

Integral (48) can be evaluated analytically and has the value

$$B^2\frac{9e^{2Lu}\xi^{2(\alpha-1-\sigma)}}{8 \tilde{k} \rho\sigma^2}f,$$

where

$$\begin{aligned} \, f={}&\frac{1}{4^{\alpha}\alpha^3\Gamma(\alpha)^2} \biggl\{\frac{\alpha\rho\sigma^2e^{-2Lu}}{3(\alpha-\sigma)} {}_2F_3\biggl(\alpha+\frac{1}{2},\alpha-\sigma; \alpha+1,2\alpha+1,\alpha-\sigma+1;-\xi^2\biggr)+{} \nonumber \\ &{}+(\alpha+\sigma+1)^2{}_2F_3\biggl(\alpha,\alpha+\frac{1}{2}; \alpha+1,\alpha+1,2\alpha+1;-\xi^2\biggr)-{} \nonumber \\ &{}-4\alpha(\alpha+\sigma+1){}_2F_3\biggl(\alpha,\alpha+\frac{1}{2}; 2\alpha,\alpha+1,\alpha+1;-\xi^2\biggr)\biggr\}+{} \nonumber \\ &{}+[(4\alpha+\xi^2)J_{\alpha}(\xi)^2-2(\alpha+1)\xi J_{\alpha}(\xi)J_{\alpha+1}(\xi)+\xi^2J_{\alpha+1}(\xi)^2]\xi^{-2\alpha}. \end{aligned}$$

(50)

We thus obtain

$$B=\frac{2\sqrt{\rho \tilde{k} }\sigma e^{-uL}}{3\xi^{\alpha-1-\sigma}\sqrt{f}} $$

(51)

for the normalizing coefficient $B$. Up to $\xi^2$, we have

$$\begin{aligned} \, \frac{1}{\sqrt{f}}={}&\frac{2^{\alpha}\sqrt{2\alpha}\Gamma(\alpha +1)} {\sqrt{(\alpha-1-\sigma)^2+\alpha\rho\sigma^2 e^{-2uL}/(3(\alpha-\sigma))}}\times{} \nonumber \\ &{}\times\biggl\{1+ \frac{\alpha\bigl(3(\alpha-1-\sigma)(\alpha+1-\sigma)^2+ (\alpha+1)e^{-2uL}\rho\sigma^2\bigr)} {12(\alpha+1)^2(\alpha+1-\sigma)\bigl((\alpha-1-\sigma)^2+ \alpha\rho\sigma^2e^{-2uL}/(3(\alpha-\sigma))\bigr)}\xi^2\biggr\}, \end{aligned}$$

(52)

$$\begin{aligned} \, B={}&\frac{2^{\alpha+1}\xi^{-(\alpha-1-\sigma)}\sqrt{ \tilde{k} }\Gamma(\alpha+1)} {\sqrt{3}\sqrt{1/(\alpha-\sigma)+3(\alpha-1-\sigma)^2 e^{2uL}/\alpha\rho\sigma^2}}\times{} \nonumber \\ &{}\times\biggl\{1+\frac{\xi^2}{4(\alpha+1)} \frac{1/(\alpha+1-\sigma)+3(\alpha-1-\sigma)(\alpha+1-\sigma) e^{2uL}/(\alpha+1)\rho\sigma^2} {1/(\alpha-\sigma)+3(\alpha-1-\sigma)^2e^{2uL}/\alpha\rho\sigma^2}\biggr\}. \end{aligned}$$

(53)

We obtain the expression for the coupling constant of the radion and matter fields

$$\begin{aligned} \, \Lambda={}&\sqrt{\frac{6M^3}{ \tilde{k} }}\frac{\sqrt{1/(\alpha-\sigma)+ 3(\alpha-1-\sigma)^2e^{2uL}/\alpha\rho\sigma^2}}{2(\alpha+1)}\times{} \nonumber \\ &{}\times\biggl\{1+ \frac{3(\alpha-1-\sigma)(\alpha+1-\sigma)^2e^{2uL}+(\alpha+1)\rho\sigma^2} {(\alpha+1)(\alpha+1-\sigma)\bigl(1/(\alpha-\sigma)+3(\alpha-1-\sigma)^2 e^{2uL}/\alpha\rho\sigma^2\bigr)\rho\sigma^2}\xi^2\biggr\}. \end{aligned}$$

(54)

Taking into account that

$$\alpha=1+\sigma+\frac{1}{6}\rho\sigma^2+O(\sigma^3)$$

up to ${\sigma}^2$, we can write $\Lambda$ in the same approximation as

$$\begin{aligned} \, \Lambda={}&\sqrt{\frac{3M^3}{8 \tilde{k} }}\biggl\{1- \frac{1}{2}\sigma+\frac{1}{24}[6-(4-e^{2uL})\rho]\sigma^2- \biggl(\frac{1}{16}(e^{2uL}-1)-\frac{1}{32}(3e^{2uL}-2)\sigma+{} \nonumber \\ &{}+\frac{1}{384}[18(2e^{2uL}-1)+ (8-5e^{2uL}-e^{4uL})\rho]\sigma^2\biggr)\xi^2\biggr\}. \end{aligned}$$

(55)

To calculate the coefficients $\alpha_1$, $\alpha_2$, $\beta_3$, and $\beta_3$, we expand the integrands in power series in $z$. Each power series thus obtained is a polynomial in $z$ and $\xi$, and all its terms are hence proportional to factors of the form $\xi^pz^q$. We omit all terms with $p+q+1>2$ to have only terms of the form $\xi^{p+q+1}$ of a degree less than two after integration over $z$. After integration, we expand in power series in $\sigma$ up to the second order inclusively and normalize to $\Lambda$ or ${\Lambda}^2$ in accordance with formula (43). As a result, up to $O(\sigma^3)$ and $O(\xi^3)$, we obtain the expressions for the normalized coefficients of the effective radion Lagrangian:

$$\begin{aligned} \, \bar{\alpha}_1={}&-2+\frac{1}{12}(2e^{2uL}-1)\rho\sigma^2- \frac{1}{4}\biggl(1-\sigma+\frac{3}{4}\biggl[1-\frac{1}{18} (4e^{2Lu}-1)\rho\biggr]\sigma^2\biggr)e^{2uL}\xi^2, \\ \bar{\alpha}_2={}&\biggl(1-\frac{1}{18}(3e^{2uL}-1)\rho\sigma^2\biggr)+ \biggl(\frac{5}{16}-\frac{17}{64}\sigma+\frac{45}{256} \biggl[1-\frac{4}{405}(27e^{2Lu}-4)\rho\biggr]\sigma^2\biggr)e^{2uL}\xi^2, \\ \bar{\beta}_3={}&\frac{3}{2}+e^{2uL}+\frac{1}{12}(e^{2Lu}-1)\rho\sigma+ \frac{1}{48}(3+2e^{2Lu}-4e^{4Lu})\rho\sigma^2+{} \\ &{}+\biggl\{-\frac{e^{2Lu}-1}{12\sigma}+ \frac{1}{144}(-6+e^{2Lu}+18e^{4Lu})-{} \\ &{}-\frac{1}{864}\bigl(2(-9+28e^{2Lu}+54e^{4Lu})- 15(e^{2Lu}-1)e^{2Lu}\rho\bigr)\sigma+{} \\ &{}+\frac{1}{10368}\bigl(2(-54+355e^{2Lu}+486e^{4Lu})+ 3(12+67e^{2Lu}-44e^{4Lu}-72e^{6Lu})\rho\bigr)\sigma^2\biggr\}\xi^2, \\ \bar{\beta}_4={}&\biggl\{18+3(1-e^{-2Lu})\rho\sigma- \frac{1}{24}[36e^{2Lu}+38e^{-2Lu}-(3-6e^{-2Lu}+3e^{-4Lu})\rho] \rho\sigma^2\biggr\}\frac{1}{\xi^2}+{} \\ &{}+\frac{1}{12}(-31+11e^{2Lu})+\biggl[-\frac{185}{36}e^{2Lu}- \frac{5}{24}+\biggl(\frac{19}{72} e^{2Lu}-\frac{5}{36}-\frac{1}{8}e^{-2Lu}\biggr)\rho\biggr]\sigma+{} \\ &{}+\biggl[\frac{1433}{432}e^{2Lu}+\frac{5}{48}-\frac{1}{72}\biggl(19e^{4Lu}+ \frac{193}{6}e^{2Lu}+\frac{169}{12}+\frac{49}{4}e^{-2Lu}\biggr)\rho+{} \\ &{}+\frac{1}{192}\bigl(3e^{2Lu}-5+e^{-2Lu}+ e^{-4Lu}\bigr)\rho^2\biggr]\sigma^2+{} \\ &{}+\biggl\{\frac{15e^{2Lu}}{4\rho\sigma^2}+ \frac{77760e^{2Lu}}{27648\rho\sigma}+\frac{3456e^{2Lu}}{27648\sigma}- \frac{630e^{2Lu}}{384\rho}+\frac{-382e^{2Lu}+29e^{4Lu}-939}{384}+{} \\ &{}+\biggl[\frac{225e^{2Lu}}{256\rho}+\frac{-692e^{4Lu}+ 372e^{2Lu}+2169}{1152}+ \frac{459e^{-2Lu}+41e^{2Lu}-83e^{4Lu}-345}{2304}\rho\biggr]\sigma+{} \\ &{}+\biggl[-\frac{465e^{2Lu}}{1024\rho}+ \frac{13100e^{4Lu}+3165e^{2Lu}-17577}{13824}+{} \\ &{}+\frac{534e^{6Lu}+3688e^{4Lu}+1817e^{2Lu}+6954-4329e^{-2Lu}}{27648}\rho-{} \\ &{}-\frac{(e^{2Lu}-1)^2(3+2e^{-2Lu}+51e^{-4Lu})}{6144}\rho^2\biggr] \sigma^2\biggr\}\xi^2. \end{aligned} $$

(56)

We note that in all expressions written in this appendix, we do not expand exponentials of the form $e^{nLu}$ ($n=-4,-2,\dots,6$) in power series, because although $Lu$ is considered a small value, the value of $e^{nLu}$ even for $Lu=0.05$ deviates from the linear approximation $1+6Lu$ by a quantity of the order of $Lu$. Expansion up to $(Lu)^2$ then increases the bulkiness of the obtained expressions but adds no new information.

We also note that for the coefficient $\bar{\beta}_4$, the leading term in the expansion in $\xi$ is positive and equal to $18/\xi^2$. Consequently, $\beta_4$ is equal to $18 \tilde{k} ^2/\Lambda^2$ in the leading order. Until now, we assumed that the values of $\tilde{k}$ and $\Lambda$ range from several units to several tens of TeV. If we also assume that the obtained effective theory must be perturbative, then $\beta_4$ must be less than some maximum value $\beta_{4\max}$, which imposes an additional constraint on the relation between the parameters $\tilde{k}$ and $\Lambda$: $\tilde{k} <\Lambda\sqrt{\beta_{4\max}/18}$.

B. Nonlinear transformation of the radion field

Effective Lagrangian (43) of the radion field has a nonstandard kinetic term. We can bring it to the usual form by a suitable nonlinear transformation. Namely, we introduce a new scalar field $\varphi$ satisfying the condition

$$P(r)\eta^{\mu\nu}r_{,\mu}r_{,\nu}= \frac{1}{2}\eta^{\mu\nu} \varphi _{,\mu} \varphi _{,\nu}. $$

(57)

For $P(r)=1/2+\alpha_1r+\alpha_2r^2$, we obtain

$$\begin{aligned} \, \varphi =\int_0^r\sqrt{\frac{1}{2}+\alpha_1r+\alpha_2r^2}\,dr={}& \frac{(\alpha_1+2\alpha_2r)\sqrt{2(1+2\alpha_1r+2\alpha_2r^2)}- \alpha_1\sqrt{2}}{8\alpha_2}-{} \nonumber \\ &{}-\frac{\alpha_1^2-2\alpha_2}{8\alpha_2^{3/2}}\log\biggl( \frac{\alpha_1+2\alpha_2r+\sqrt{2\alpha_2(1+2\alpha_1r+2\alpha_2r^2)}} {\alpha_1+\sqrt{2\alpha_2}}\,\biggr). \end{aligned}$$

(58)

The inverse expression (i.e., the explicit functional dependence of $r$ on $\varphi$) cannot be represented in an analytic form. Nevertheless, up to the third order in $\varphi$, we can write

$$r= \varphi -\frac{\alpha_1}{2} \varphi ^2+ \frac{1}{3}(2\alpha_1^2-\alpha_2) \varphi ^3+\ldots. $$

(59)

For the potential $U(r)$ in the fourth order in $\varphi$, we thus obtain

$$\begin{aligned} \, U(r)&=\mu^2\biggl\{\frac{1}{2}r^2+\frac{\bar{\beta}_3}{\Lambda}r^3+ \frac{\bar{\beta}_4}{\Lambda^2}r^4\biggr\}= \nonumber \\ &=\mu^2\biggl\{\frac{ \varphi ^2}{2}+\frac{1}{\Lambda} \biggl(\bar{\beta}_3-\frac{\bar{\alpha}_1}{2}\biggr) \varphi ^3+ \frac{1}{\Lambda^2}\biggl(\bar{\beta}_4- \frac{3\bar{\alpha}_1\bar{\beta}_3}{2}+ \frac{19\bar{\alpha}_1^2-8\bar{\alpha}_2}{24}\biggr) \varphi ^4\biggr\}. \end{aligned}$$

(60)

Substituting expressions for $\bar{\alpha}$ and $\bar{\beta}$ in the last formula, we obtain

$$U(r)=\mu^2\biggl\{\frac{1}{2} \varphi ^2+\frac{1}{\Lambda} \tilde{\beta} _3 \varphi ^3+ \frac{1}{\Lambda^2} \tilde{\beta} _4 \varphi ^4\biggr\}+ \frac{ \tilde{k} ^2}{\Lambda^2} \tilde{\beta} '_4 \varphi ^4. $$

(61)

Here, we decompose the coefficient of $r^4$ into two terms: the first of the order of $\mu^2$ with the dimensionless parameter $\tilde{\beta}$ and the second of the order of $\tilde{k} ^2$ with the dimensionless parameter $\tilde{\beta} '$. Up to $\xi^0$ (because $\xi$ is a small parameter), we obtain

$$\begin{aligned} \, \tilde{\beta} _3={}&\frac{5}{2}+e^{2Lu}+\frac{1}{12}(e^{2Lu}-1)\rho\sigma- \frac{1}{48}(2e^{2Lu}+4e^{4Lu}-5)\rho\sigma^2, \\ \tilde{\beta} _4={}&\frac{47}{12}e^{2Lu}+\frac{19}{4}-\biggl[\frac{185}{36}e^{2Lu}+ \frac{5}{24}+\biggl(\frac{37}{72}e^{2Lu}-\frac{7}{18}- \frac{1}{8}e^{-2Lu}\biggr)\rho\biggr]\sigma+{} \\ &{}+\biggl[\frac{5}{48}+\frac{1433}{432}e^{2Lu}+ \biggl(-\frac{55}{72}e^{4Lu}-\frac{451}{432}e^{2Lu}+ \frac{367}{864}-\frac{49}{288}e^{-2Lu}\biggr)\rho+{} \\ &{}+\biggl(\frac{1}{64}e^{2Lu}-\frac{5}{192}+\frac{1}{192}e^{-2Lu}+ \frac{1}{192}e^{-4Lu}\biggr)\rho^2\biggr]\sigma^2, \\ \tilde{\beta} '_4={}&18+3\biggl(1-e^{-2Lu}\biggr)\rho\sigma- \frac{1}{24}\biggl(36e^{6Lu}+38e^{2Lu}- 3\biggl(e^{4Lu}-2e^{2Lu}+1\biggr)\rho\biggr)e^{-4Lu}\rho\sigma^2. \end{aligned} $$

(62)

Because $\sigma$ is also a small parameter, neglecting it, we obtain

$$U(r)=\mu^2\biggl\{\frac{1}{2} \varphi ^2+\frac{1}{\Lambda} \biggl(\frac{5}{2}+e^{2Lu}\biggr) \varphi ^3+ \frac{1}{\Lambda^2}\biggl(\frac{47}{12}e^{2Lu}+ \frac{19}{4}\biggr) \varphi ^4\biggr\}+\frac{18 \tilde{k} ^2}{\Lambda^2} \varphi ^4. $$

(63)

We note that the factor $\tilde{k} ^2/\Lambda^2$ with $\varphi ^4$ outside the braces is larger than the analogous factor $\mu^2/\Lambda^2$ inside the braces by two to four orders (by a factor of $\xi^{-2}$). Taking relation (25) between the Planck mass and the fundamental energy scale into account, we obtain

$$\frac{18 \tilde{k} ^2}{\Lambda^2}\approx\frac{18}{\Lambda^2L^2} \log^2\biggl(\sqrt{\frac{3}{32}}\frac{M_{ \mathrm{Pl} }}{\Lambda}\biggr). $$

(64)

We obtain

$$\frac{18 \tilde{k} ^2}{\Lambda^2}\approx\frac{18}{\Lambda^2L^2}\log^2\biggl( \frac{7.44\cdot10^{14}}{(\Lambda/\text{TeV})}\biggr)\sim3.4\cdot10^3 $$

(65)

for $\Lambda\sim10$ TeV and $L\sim\Lambda^{-1}=0.1$ TeV$^{-1}$ and

$$\frac{18 \tilde{k} ^2}{\Lambda^2}\sim8.3\cdot10^{-2} $$

(66)

for $\Lambda\sim20$ TeV and $L\sim\Lambda^{-1}=10$ TeV$^{-1}$.

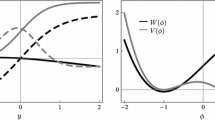
The self-coupling parameter $\lambda_3\equiv(\mu^2/\Lambda) \tilde{\beta} _3$ at $\varphi ^3$ has the dimension of mass, and the parameter $\lambda_4\equiv (\mu^2/\Lambda^2) \tilde{\beta} _4+( \tilde{k} ^2/\Lambda^2) \tilde{\beta} '_4\approx ( \tilde{k} ^2/\Lambda^2) \tilde{\beta} '_4$ at $\varphi ^4$ is dimensional. Therefore, a direct comparison of these quantities is not well defined. Nevertheless, the second parameter value is much greater than the first in a certain sense. In processes with four external radion lines in the low-energy limit, the contribution of the tree diagram with two third-order vertices is proportional to $\lambda_3^2/\mu^2$ and the contribution of the diagram with one fourth-order vertex is proportional to $\lambda_4$, i.e., $( \tilde{k} ^2/\mu^2)( \tilde{\beta} '_4/ \tilde{\beta} _3^2)$ times greater. The large value (compared with $\lambda_3^2/\mu^2$) is also guaranteed because the radion is at the true minimum in the effective four-dimensional model obtained after reduction. Indeed, the written fourth-order potential could have several minimums if the equation

$$\frac{dU}{d \varphi }=\{\mu^2+3\lambda_3 \varphi +4\lambda_4 \varphi ^2\} \varphi =0 $$

(67)

had more than one solution. For this, the discriminant before the quadratic equation in the braces must be positive, but this is not so:

$$D=9\lambda_3^2-16\lambda_4\mu^2=16\mu^2\lambda_4 \biggl(\frac{9}{16}\frac{\mu^2}{ \tilde{k} ^2}\frac{ \tilde{\beta} _3^2}{ \tilde{\beta} '_4}-1\biggr)<0. $$

(68)