Periodic TASEP with general initial conditions

Abstract

We consider the one-dimensional totally asymmetric simple exclusion process with an arbitrary initial condition in a spatially periodic domain, and obtain explicit formulas for the multi-point distributions in the space-time plane. The formulas are given in terms of an integral involving a Fredholm determinant. We then evaluate the large-time, large-period limit in the relaxation time scale, which is the scale such that the system size starts to affect the height fluctuations. The limit is obtained assuming certain conditions on the initial condition, which we show that the step, flat, and step-flat initial conditions satisfy. Hence, we obtain the limit theorem for these three initial conditions in the periodic model, extending the previous work on the step initial condition. We also consider uniform random and uniform-step random initial conditions.

Probabilistic argument for the step-flat case when \(L_s=O(L)\)

In the step-flat initial condition, the parameters satisfy $L=dN+L_s$ for $0<L_s<N$. We evaluated the limit when $L_s=O(\sqrt{L})$ in Sect. 7. In this section, we discuss the case when $L_s=O(L)$ and provide a probabilistic argument that the large time limit should be same as the step initial condition. It should be possible to make this argument rigorous but we content to discuss heuristically since this is not a main part of this paper.

We discuss in terms of the periodic directed last passage percolation (DLPP) model which is well-known to be related to the TASEP. Assume that each lattice point $(i,j)\in {\mathbb {Z}}^2$ is assigned an exponential random variable w(i, j) with parameter 1. These w(i, j)’s are all independent except for the following periodicity condition

$$\begin{aligned} w(i,j) = w(i+L-N,j-N),\quad i,j\in {\mathbb {Z}}. \end{aligned}$$

Let $\varLambda$ be a lattice path; $\varLambda$ consists of connected unit horizontal or vertical line segments with vertices in ${\mathbb {Z}}^2$, and $\varLambda$ does not intersect any line $y-x=$constant twice. We define the last passage time from $\varLambda$ to a lattice point $\mathrm{p}$ as

$$\begin{aligned} {\mathcal {L}}_\varLambda (\mathrm{p}):= \max _{\varPi :\varLambda \rightarrow \mathrm{p}} \sum _{(i,j)\in \varPi } w(i,j), \end{aligned}$$

where the maximum is over all possible up/right lattice path starting from any lattice point in $\varLambda$ and ending at $\mathrm{p}$. We assume that ${\mathcal {L}}_\varLambda (\mathrm{p})=-\infty$ if no such path exists. Similarly, one can define ${\mathcal {L}}_{\mathrm{q}}(\mathrm{p})$ if the lattice path is restricted to start from a given point $\mathrm{q}$.

Now we consider the step-flat and step initial conditions of the ${{\,\mathrm{PTASEP}\,}}$. These two initial conditions in the language of periodic DLPP, correspond to the lattice paths

$$\begin{aligned} \begin{aligned} \varLambda _{\mathrm {sf}} =&\left( \{(i,j): -(d-1)j \le i \le -(d-1)(j-1), 1\le i\le N\}\right. \\&\left. \cup \{(i,0):0\le i\le L_s\}\right) + (N,-L+N){\mathbb {Z}}\end{aligned} \end{aligned}$$

and

$$\begin{aligned} \varLambda _{\mathrm{step}}=\left( \{(0, j): 0\le j\le N\} \cup \{(i,0): 0\le i\le L-N\}\right) + (N,-L+N){\mathbb {Z}}, \end{aligned}$$

respectively. From the well-known connection between the TASEP and the DLPP, the convergence of the ${{\,\mathrm{PTASEP}\,}}$ with the step-flat initial condition to the step flat initial condition in the large L limit with $L_s=O(L)$ is translated into the following question on the periodic DLPP: Our goal is to show that when (1) $\mathrm{p}$ is far enough, more precisely,

$$\begin{aligned} \mathrm{{dist}}\,\left( \mathrm{p},\varLambda _{\mathrm {sf}}\right) = O(L^{3/2}), \end{aligned}$$

which corresponds to the relaxation time scale in PTASEP, and (2) $L_s= O(L)$, then

$$\begin{aligned} {\mathcal {L}}_{\varLambda _{\mathrm {sf}}} (\mathrm{p}) = {\mathcal {L}}_{\varLambda _{\mathrm{step}}} (\mathrm{p}) +o(L^{1/2}) \text { as }L\rightarrow \infty . \end{aligned}$$

(A.1)

It is known that in the relaxation time scale, both ${\mathcal {L}}_{\varLambda _{\mathrm {sf}}} (\mathrm{p})$ and ${\mathcal {L}}_{\varLambda _{\mathrm{step}}} (\mathrm{p})$ have $O(\sqrt{L})$ fluctuations. The above estimate implies ${\mathcal {L}}_{\varLambda _{\mathrm {sf}}} (\mathrm{p})$ and ${\mathcal {L}}_{\varLambda _{\mathrm{step}}} (\mathrm{p})$ have the same limiting fluctuation. In other words, if $L_s=O(L)$, these two initial conditions yield to the same limiting fluctuations in the relaxation time scale.

Fig. 12

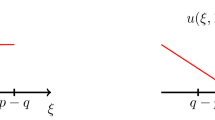
Illustration of periodic DLPP from $\varLambda _{\mathrm {sf}}$ to $\mathrm{p}$. In the figure on the left, the stair-shape path is $\varLambda _{\mathrm{step}}$, the dotted line is $\varLambda _{\mathrm{flat}}$ with particle density $\rho$, and $\varLambda _{\mathrm {sf}}$ lies between between $\varLambda _{\mathrm{step}}$ and $\varLambda _{\mathrm{flat}}$; It is the path between the white region above $\varLambda _{\mathrm{flat}}$ and the black region below $\varLambda _{\mathrm{step}}$. The figure on the right is a detailed view of the maximal path

See Fig. 12 for an illustration of $\varLambda _{\mathrm{step}}$ and $\varLambda _{\mathrm {sf}}$. From the definition, $\varLambda _{\mathrm {sf}}$ lies on the lower left side of $\varLambda _{\mathrm{step}}$. Hence, the last passage time from $\varLambda _{\mathrm {sf}}$ is larger than or equal to the time from $\varLambda _{\mathrm{step}}$. This implies that

$$\begin{aligned} {\mathcal {L}}_{\varLambda _{\mathrm {sf}}} (\mathrm{p}) \ge {\mathcal {L}}_{\varLambda _{\mathrm{step}}} (\mathrm{p}) . \end{aligned}$$

Thus, (A.1) follows is we show that

$$\begin{aligned} {\mathcal {L}}_{\varLambda _{\mathrm {sf}}} (\mathrm{p}) \le {\mathcal {L}}_{\varLambda _{\mathrm{step}}} (\mathrm{p}) +o(L^{1/2}) \text { as }L\rightarrow \infty . \end{aligned}$$

(A.2)

Let

$$\begin{aligned} \varLambda _{\mathrm{flat}}:=\{(x,y)\in {\mathbb {R}}^2: y=(1-\rho ^{-1}) x\}. \end{aligned}$$

We remark that $\varLambda _{\mathrm{flat}}$ is not necessary a lattice path. See Fig. 12 for $\varLambda _{\mathrm{flat}}$.

The inequality (A.2) heuristically follows from the slow decorrelation of (periodic) directed last passage percolation, which was discussed in [17]. We point out that although this paper was for the DLPP, the same argument extends to the periodic DLPP. The slow decorrelation implies, if the starting point $\mathrm{q}\in \varLambda _{\mathrm {sf}}$ is not on $\varLambda _{\mathrm{step}}$, say $\mathrm{q}$ is between two corners A and B as shown in Fig. 12, then

$$\begin{aligned} {\mathcal {L}}_{\mathrm{q}} (\mathrm{p}) = -c\cdot \mathrm{{dist}}\,(\mathrm{q},{{\bar{\mathrm{q}}}}) + {\mathcal {L}}_{\bar{\mathrm{q}}} (\mathrm{p}) +o((\mathrm{{dist}}\,(\mathrm{p},\mathrm{q}))^{1/3})=-c\cdot \mathrm{{dist}}\,(\mathrm{q},{{\bar{\mathrm{q}}}}) + {\mathcal {L}}_{\bar{\mathrm{q}}} (\mathrm{p}) +o(L^{1/2}), \end{aligned}$$

(A.3)

where $c>0$ is some constant independent of L, and ${\bar{\mathrm{q}}}$ is the intersection of the line $\mathrm{pq}$ and $\varLambda _{\mathrm{flat}}$.

Note that

$$\begin{aligned} {\mathcal {L}}_{\bar{\mathrm{q}}} (\mathrm{p})\le {\mathcal {L}}_{\varLambda _{\mathrm{flat}}}(\mathrm{p})={\mathcal {L}}_{\varLambda _{\mathrm{step}}}(\mathrm{p})+O(L^{1/2}), \end{aligned}$$

where the last equality follows from Theorem 6.4 (the one point distribution case) and the case we proved for step and flat initial conditions (Theorem 7.1)³. We assume that ${{\bar{\mathrm{q}}}}$ is within an O(L) interval where the maximum path from $\varLambda _{\mathrm{flat}}$ to $\mathrm{p}$ is obtained. Otherwise, ${\mathcal {L}}_{\bar{\mathrm{q}}} (\mathrm{p})<{\mathcal {L}}_{\varLambda _{\mathrm{step}}}(\mathrm{p})$ for far enough ${{\bar{\mathrm{q}}}}$. And ${\mathcal {L}}_{\mathrm{q}} (\mathrm{p})<{\mathcal {L}}_{\bar{\mathrm{q}}} (\mathrm{p}) +o(L^{1/2})<{\mathcal {L}}_{\varLambda _{\mathrm{step}}} (\mathrm{p}) +o(L^{1/2})$ holds trivially. This assumption means that ${\mathcal {L}}_{\bar{\mathrm{q}}} (\mathrm{p})$ and ${\mathcal {L}}_{B} (\mathrm{p})$ have the same deterministic order terms and they only differ from the fluctuation terms, which is of $O(L^{1/2})$.

We let C be the other intersection point of the line $B\mathrm{q}$ with $\varLambda _{\mathrm{step}}$. It also lies on $\varLambda _{\mathrm {sf}}$. By the definition, $\mathrm{{dist}}\,(A,C)=L_s$ has the same order as $\mathrm{{dist}}\,(A,B)$. Hence $\mathrm{{dist}}\,(\mathrm{q},{{\bar{\mathrm{q}}}})$ has the same order as $\mathrm{{dist}}\,(B,{{\bar{\mathrm{q}}}})$.

Now we consider two situations. If $\mathrm{{dist}}\,(\mathrm{q},{\bar{\mathrm{q}}})\ll O(L)$, then $\mathrm{{dist}}\,(B,{{\bar{\mathrm{q}}}})\ll O(L)$. In this case, ${\mathcal {L}}_{{\bar{\mathrm{q}}}}(\mathrm{p})$ is asymptotically identical to ${\mathcal {L}}_{B}(\mathrm{p})$ since the two points B and ${{\bar{\mathrm{q}}}}$ are closer than the correlation length O(L). More precisely, we have

$$\begin{aligned} {\mathcal {L}}_{{\bar{\mathrm{q}}}}(\mathrm{p})={\mathcal {L}}_{B}(\mathrm{p})+o(L^{1/2})\le {\mathcal {L}}_{\varLambda _{\mathrm{step}}}(\mathrm{p})+o(L^{1/2}). \end{aligned}$$

Together with (A.3) we obtain

$$\begin{aligned} {\mathcal {L}}_{\mathrm{q}} (\mathrm{p})\le {\mathcal {L}}_{\varLambda _{\mathrm{step}}}(\mathrm{p})+o(L^{1/2}). \end{aligned}$$

(A.4)

The second situation is that $\mathrm{{dist}}\,(\mathrm{q},{{\bar{\mathrm{q}}}})\gg O(L^{1/2})$. In this case, we use the trivial estimate

$$\begin{aligned} {\mathcal {L}}_{{\bar{\mathrm{q}}}}(\mathrm{p}) \le {\mathcal {L}}_{B}(\mathrm{p})+O(L^{1/2})\le {\mathcal {L}}_{\varLambda _{\mathrm{step}}}(\mathrm{p})+O(L^{1/2}), \end{aligned}$$

while the term $O(L^{1/2})$ in this estimate is always dominated by $-c\cdot \mathrm{{dist}}\,(\mathrm{q},{{\bar{\mathrm{q}}}})$ in (A.3). Hence we still have (A.4).

Note that $\mathrm{q}$ is an arbitrary point on $\varLambda _{\mathrm {sf}}$, the above estimates imply (A.2).