No CrossRef data available.
Article contents
On a rationality problem for fields of cross-ratios II
Published online by Cambridge University Press: 04 September 2020
Abstract
Let k be a field,
 $x_1, \dots , x_n$
be independent variables and let
$x_1, \dots , x_n$
be independent variables and let
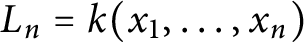 $L_n = k(x_1, \dots , x_n)$
. The symmetric group
$L_n = k(x_1, \dots , x_n)$
. The symmetric group
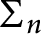 $\operatorname {\Sigma }_n$
acts on
$\operatorname {\Sigma }_n$
acts on
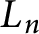 $L_n$
by permuting the variables, and the projective linear group
$L_n$
by permuting the variables, and the projective linear group
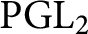 $\operatorname {PGL}_2$
acts by
$\operatorname {PGL}_2$
acts by

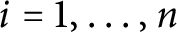 $i = 1, \dots , n$
. The fixed field
$i = 1, \dots , n$
. The fixed field
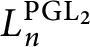 $L_n^{\operatorname {PGL}_2}$
is called “the field of cross-ratios”. Given a subgroup
$L_n^{\operatorname {PGL}_2}$
is called “the field of cross-ratios”. Given a subgroup
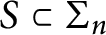 $S \subset \operatorname {\Sigma }_n$
, H. Tsunogai asked whether
$S \subset \operatorname {\Sigma }_n$
, H. Tsunogai asked whether
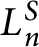 $L_n^S$
rational over
$L_n^S$
rational over
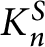 $K_n^S$
. When
$K_n^S$
. When
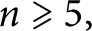 $n \geqslant 5,$
the second author has shown that
$n \geqslant 5,$
the second author has shown that
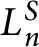 $L_n^S$
is rational over
$L_n^S$
is rational over
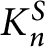 $K_n^S$
if and only if S has an orbit of odd order in
$K_n^S$
if and only if S has an orbit of odd order in
 $\{ 1, \dots , n \}$
. In this paper, we answer Tsunogai’s question for
$\{ 1, \dots , n \}$
. In this paper, we answer Tsunogai’s question for
 $n \leqslant 4$
.
$n \leqslant 4$
.
MSC classification
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2020
Footnotes
Tran-Trung Nghiem was supported by a Fondation Hadamard Scholarship. Zinovy Reichstein was partially supported by National Sciences and Engineering Research Council of Canada Discovery grant 253424-2017.





