Skip to main content
SpringerLink
Log in

Numerical solution of variable order fractional differential equations by using shifted Legendre cardinal functions and Ritz method

  • Original Article
  • Published:
Engineering with Computers Aims and scope Submit manuscript

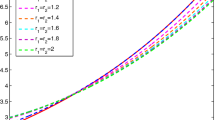
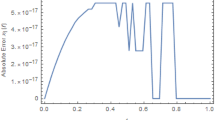
Abstract

In this paper, we present a new numerical method for solving variable order fractional differential equations, which is based on shifted Legendre cardinal functions. First, we obtain the pseudo-operational matrix of the variable order fractional derivative by applying the properties mentioned in the Caputo derivative of fractional variable order. Then, using Ritz method, the pseudo-operational matrix and collocation method, the problem is reduced to a system of algebraic equations that is solved by Newton’s iterative method. Illustrative examples are included to demonstrate the efficiency and accuracy of the proposed method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

References

  1. Benson DA, Meerschaert MM, Revielle J (2013) Fractional calculus in hydrologicmodeling: a numerical perspective. Adv Water Resour 51:479–497

    Article  Google Scholar 

  2. Popovic JK, Spasic DT, Tosic J, Kolarovic JL, Malti R, Mitic IM, Pilipovic S, Atanackovic TM (2015) Fractional model for pharmacokinetics of high dose methotrexate in children with acute lymphoblastic leukaemia. Commun Nonlinear Sci Numer Simul 22:451–471

    Article  MathSciNet  Google Scholar 

  3. Sierociuk D, Dzielinski A, Sarwas G, Petras I, Podlubny I, Skovranek T (2013) Modelling heat transfer in heterogeneous madia using fractional calculus. Phil Trans R Soc A 371:20120146. https://doi.org/10.1098/rsta.2012.0146

    Article  MATH  Google Scholar 

  4. Larsson S, Racheva M, Saedpanah F (2015) Discontinuous Galerkin method for an integro-differential equation modeling dynamic fractional order viscoelasticity. Comput Method Appl Mech Eng 283:196–209

    Article  MathSciNet  Google Scholar 

  5. Jiang Y, Wang X, Wang Y (2012) On a stochastic heat equation with first order fractional noises and applications to finance. J Math Anal Appl 396:656–669

    Article  MathSciNet  Google Scholar 

  6. Bohannan G (2008) Analog fractional order controller in temperature and motor control applications. J Vib Contr 14:1487–1498

    Article  MathSciNet  Google Scholar 

  7. Doha EH, Bhrawy AH, Ezz-Eldien SSA (2011) Chebyshev spectral method based on operational matrix for initial and boundary value problems of fractional order. Comput Math Appl 62:2364–2373

    Article  MathSciNet  Google Scholar 

  8. Saeedi H (2011) A CAS wavelet method for solving nonlinear Fredholm integro-differential equation of fractional order. Commun Nonlinear Sci Numer Simul 16:1154–1163

    Article  MathSciNet  Google Scholar 

  9. Zhang Y (2009) A finite difference method for fractional partial differential equation. Appl Math Comput 215:524–529

    MathSciNet  MATH  Google Scholar 

  10. Abbaszadeh M, Dehghan M (2020) A finite-difference procedure to solve weakly singular integro partial differential equation with space–time fractional derivatives. Eng Comput. https://doi.org/10.1007/s00366-020-00936-w

    Article  Google Scholar 

  11. Dehghan M, Abbaszadeh M (2017) A finite element method for the numerical solution of Rayleigh-Stokes problem for a heated generalized second grade fluid with fractional derivatives. Eng Comput 33(3):587–605

    Article  Google Scholar 

  12. Ghoreishi F, Yazdani S (2011) An extension of the spectral Tau method for numerical solution of multi-order fractional differential equations with convergence analysis. Comput Math Appl 61:30–43

    Article  MathSciNet  Google Scholar 

  13. Rahimkhani P, Ordokhani Y, Babolian E (2016) Fractional-order Bernoulli wavelets and their applications. Appl Math Model 40(17):8087–8107

    Article  MathSciNet  Google Scholar 

  14. Jafari H, Yousefi SA, Firoozjaee MA, Momani S, Khalique CM (2011) Application of Legendre wavelets for solving fractional differential equations. Comput Math Appl 62(3):1038–1045

    Article  MathSciNet  Google Scholar 

  15. Sabermahani S, Ordokhani Y, Yousefi SA (2019) Fractional-order fibonacci-hybrid functions approach for solving fractional delay differential equations. Eng Comput. https://doi.org/10.1007/s00366-019-00730-3

    Article  MATH  Google Scholar 

  16. Jafari H, Seifi S (2009) Homotopy analysis method for solving linear and nonlinear fractional diffusion-wave equation. Commun Nonlinear Sci Numer Simul 14:2006–2012

    Article  MathSciNet  Google Scholar 

  17. Gupta S, Kumar D, Singh J (2015) Numerical study for systems of fractional differential equations via Laplace transform. J Egypt Math Soc 23(2):256–262

    Article  MathSciNet  Google Scholar 

  18. Abbaszadeh M, Dehghan M (2019) Meshless upwind local radial basis function-finite difference technique to simulate the time fractional distributed-order advection-diffusion equation. Eng Comput 20:1–17. https://doi.org/10.1007/s00366-019-00861-7

    Article  Google Scholar 

  19. Nemati S, Lima P, Sedaghat S (2018) An effective numerical method for solving fractional pantograph differential equations using modification of hat functions. Appl Numer Math 131:174–189

    Article  MathSciNet  Google Scholar 

  20. Pedro HTC, Kobayashi MH, Pereira JMC, Coimbra CFM (2008) Variable order modeling of diffusive-convective effects on the oscillatory flow past a sphere. J Vib Control 14:1569–1672

    Article  MathSciNet  Google Scholar 

  21. Soon CM, Coimbra CFM, Kobayashi MH (2005) The variable viscoelasticity oscillator. Ann Phys 14(6):378–389

    Article  Google Scholar 

  22. Obembe AD, Hossain ME, Abu-Khamsin SA (2017) Variable-order derivative time fractional diffusion model for heterogeneous porous media. J Petrol Sci Eng 152:391–405

    Article  Google Scholar 

  23. Ingman D, Suzdalnitsky J, Zeifman M (2000) Constitutive dynamic-order model for nonlinear contact phenomena. J Appl Mech 67(2):383–390

    Article  Google Scholar 

  24. Sun HG, Chen YQ, Chen W (2011) Random-order fractional differential equation models. Signal Process 91:525–30

    Article  Google Scholar 

  25. Kilbas AA, Srivastava HM (2006) In: Trujillo JJ (ed) Theory and applications of fractional differential equations. Elsevier, Amsterdam

    MATH  Google Scholar 

  26. Lin R, Liu F, Anh V, Turner I (2009) Stability and convergence of a new explicit finite-difference approximation for the variable-order nonlinear fractional diffusion equation. Appl Math Comput 212(2):435–445

    MathSciNet  MATH  Google Scholar 

  27. Bhrawy AH, Zaky MA (2016) Numerical simulation for two-dimensional variable-order fractional nonlinear cable equation Nonlinear. Dyn 80(1):101–116

    MathSciNet  MATH  Google Scholar 

  28. Sabermahani S, Ordokhani Y, Lima PM (2020) A novel lagrange operational matrix and Tau-collocation method for solving variable-order fractional differential equations. Iran J Sci Technol Trans Sci 44:127–135. https://doi.org/10.1007/s40995-019-00797-z

    Article  MathSciNet  Google Scholar 

  29. Chen Y, Liu L, Liu D, Boutat D (2018) Numerical study of a class of variable order nonlinear fractional differential equation in terms of Bernstein polynomials. Ain Shams Eng J 9(4):1235–1241

    Article  Google Scholar 

  30. Chena YM, Wei YQ, Liu DY, Yua H (2015) Numerical solution for a class of nonlinear variable order fractional differential equations with Legendre wavelets. Appl Math Lett 46:83–88

    Article  MathSciNet  Google Scholar 

  31. Li X, Wu B (2017) A new reproducing kernel method for variable order fractional boundary value problems for functional differential equations. J Comput Appl Math 311:387–393

    Article  MathSciNet  Google Scholar 

  32. Heydari MH, Avazzadeh Z (2018) An operational matrix method for solving variable- order fractional biharmonic equation. Comput Appl Math 37(4):4397–4411

    Article  MathSciNet  Google Scholar 

  33. Dehestani H, Ordokhani Y, Razzaghi M (2020) Pseudo-operational matrix method for the solution of variable-order fractional partial integro-diferential equations. Eng Comput. https://doi.org/10.1007/s00366-019-00912-z

    Article  MATH  Google Scholar 

  34. Almeida R, Tavares D, Torres D (2019) The variable-order fractional calculus of variations. Applied sciences and technology. Springer, Cham

    Book  Google Scholar 

  35. Funaro D (1992) Approximation polynomial, of differential equations. Springer, New York

    Book  Google Scholar 

  36. Christoffel EB (1858) Ueber die Gaussche Quadratur und eine Verallgemeinerung derselben. J Reine Angew Math 55:61–82

    MathSciNet  Google Scholar 

  37. El-Sayed AA, Agarwal P (2019) Numerical solution of multiterm variable order fractional differential equations via shifted Legendre polynomials. Math Methods Appl Sci 42(11):3978–3991

    Article  MathSciNet  Google Scholar 

  38. Moghaddam BP, Machado JA, Behforooz H (2017) An integro quadratic spline approach for a class of variable-order fractional initial value problems. Chaos Solitons Fractals 102:354–360

    Article  MathSciNet  Google Scholar 

  39. Mokhtary P, Ghoreishi F (2014) Convergence analysis of spectral tau method for fractional Riccati differential equations. Bull Iran Math Soc 40(5):1275–1290

    MathSciNet  MATH  Google Scholar 

  40. Doha EH, Abdelkawy MA, Amin A, Baleanu D (2019) Approximate solutions for solving nonlinear variable-order fractional Riccati differential equations. Nonlinear Anal Model Control 24:176–188

    Article  MathSciNet  Google Scholar 

  41. Akgul A, Inc M, Baleanu D (2017) On solutions of variable-order fractional differential equations. Int J Optim Control Theor Appl 7(1):112–116

    Article  MathSciNet  Google Scholar 

  42. Zaky M, Doha EH, Taha TM, Baleanu D (2018) New recursive approximations for variable-order fractional operators with applications. Math Model Anal 23:227–239

    Article  MathSciNet  Google Scholar 

  43. Bhrawyi AH, Zaky MA, Abdel-Aty M (2017) A fast and precise numerical algorithm for a class of variable-order fractional differential equations. Proc Romanian Acad Ser Math Phys Tech Sci Inf Sci 18(1):17–24

    MathSciNet  Google Scholar 

Download references

Acknowledgements

We would like to thank the referees for their valuable comments and helpful suggestions to improve the earlier version of this paper.

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Mathematical Sciences, Alzahra University, Tehran, Iran

    Faezeh Sadat Yousefi & Yadollah Ordokhani

  2. Department of Mathematics, Faculty of Mathematical Sciences, Shahid Beheshti University, Tehran, Iran

    Sohrabali Yousefi

Authors
  1. Faezeh Sadat Yousefi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yadollah Ordokhani
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Sohrabali Yousefi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yadollah Ordokhani.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yousefi, F.S., Ordokhani, Y. & Yousefi, S. Numerical solution of variable order fractional differential equations by using shifted Legendre cardinal functions and Ritz method. Engineering with Computers 38, 1977–1984 (2022). https://doi.org/10.1007/s00366-020-01192-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00366-020-01192-8

Keywords

Search

Navigation