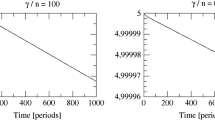
Abstract
The nutations of Mars are about to be estimated to a few milliarcseconds accuracy with the radioscience experiments onboard InSight and ExoMars 2022. The contribution to the nutations due to the liquid core and tidal deformations will be detected, allowing to constrain the interior of Mars. To avoid introducing systematic errors in the determination of the core properties, an accurate precession and nutation model for a rigidly behaving Mars is needed. Here, we develop such a model with adequate accuracy based on the Torque approach and compare it to previous models. We include in the model the forcings by the Sun, Phobos, Deimos, and the other planets of the solar system. We also include the geodetic precession and nutations. We use semi-analytical developments for the solar and planetary torques, and analytical solutions for the effect of Phobos and Deimos and for the geodetic precession and nutations. With a truncation criterion of 0.025 milliarcseconds in prograde and/or retrograde amplitude, we identify 43 nutation terms. The uncertainty on our solution mainly derives from the observational uncertainty on the current determination of the precession rate of Mars. Uncertainties related to our modeling choices are negligible in comparison. Given the current determination of the precession rate (\(7608.3\pm 2.1\) mas/yr, Konopliv et al. in Icarus 274:253–260, 2016. https://doi.org/10.1016/j.icarus.2016.02.052), our model predicts a dynamical flattening \(H_{{D}}=0.00538017\pm 0.00000148\) and a normalized polar moment of inertia \(C/\mathrm{MR}^2=0.36367\pm 0.00010\) for Mars.
Similar content being viewed by others
Notes
The arguments \(2\omega -2h\) of Borderies (h is the same angle as the angle \(\theta \) defined above), \(2\varLambda \) of Hilton, and \(2\varPhi _0\) of Groten correspond by definition to the argument q of RK79. Note that a confusion between the numerical values of \(\omega -\theta \) and \(\omega \) in Groten et al. (1996) (he wrongly uses \(q=-147^{\circ }\) instead of \(q\cong 142^{\circ }\)) renders his series incorrect.
Hilton (1991) also attempted to take into account the changes in eccentricity with time. He wrongly assumed that the orbit perturbations change the J2000 mean eccentricity (\(e_0\)), leading to incorrect changes in the precession rate and mean values of the nutation amplitudes. The eccentricity is defined as \(e=\sqrt{h^2+k^2}\) with h and k given in Bretagnon (1982) under the form \(\sum _n h_n\) and \(\sum _n k_n\), see Eq. (57) of Hilton (1991). When we follow his procedure to extract the J2000 mean eccentricity, we find \(e_0=0.0936037\), whereas \(e_0=0.0934006\), see Simon et al. (1994). As a consequence, the term in \(\sin M\) in his Table 1 with an amplitude of \(-\,634.4\) mas and which is proportional to \(e_0\) is wrongly changed by 1.4 mas, see his Table 2. What is wrong in Hilton’s procedure? First, he constructs the eccentricity as \(e=\sum _n \sqrt{h_n^2+k_n^2}=\sum _n e_n\) instead of \(\sqrt{(\sum _n h_n)^2+(\sum _n k_n)^2}\). Second, he expands each \(e_n\) about \(T=0\) and keeps the zeroth-order terms, which makes no sense for relatively short period terms (between 400 and 6000 days for the principal terms of h and k). The correct value of \(e_0\) can be obtained by expanding \(\sqrt{(\sum _n h_n)^2+(\sum _n k_n)^2}\) about \(\sqrt{h_0^2+k_0^2}\) with \(h_0\) and \(k_0\) the constant parts of h and k.
For the gravitational action of the Sun, the factor \(1/r^3\) in their disturbing potential \(U_i\) (their Eq. 7), with r the distance of the Sun to Mars, is developed as a function of the eccentricity and mean longitude of Mars, that is to say as if Mars was evolving on an osculating orbit. The factor \(P_2^0(\sin \delta )\) is expressed as a function of spherical coordinates which are converted from the rectangular coordinates of Mars in the VSOP87.
And therefore implicitly for all the secular variations of the mean orbital elements, not just for the secular variations in eccentricity or inclination.
Under the “Keplerian assumption,” the modulations in nutation amplitude can be obtained by extracting the long-period arguments (the orbital elements other than the mean anomaly, see Sect. 3.4.2) from the sine and cosine developments through a linearization around their epoch values.
The solution is obtained after repeated trigonometric manipulations of a large amount of terms. When the computation speed and random-access memory are too limited, as was the case 20 years ago, approximations which alter the accuracy of the solution must be done during the computation. See details of the computation procedure of the recomputed RMAN99 solution in Sect. 4.
The value 0.00536 reported in RMAN99 is in fact a wrong rounding of the value actually used, Fabian Roosbeek, personal communication.
Contrary to what is stated in RMAN99, his nutation series are referred to the mean orbit and equinox of epoch J2000 and not of date, as he sets \(t=0\) in his Eq. (3) (Roosbeek, personal communication).
The nutation series of BS99 are in fact referred to the non-inertial mean orbital plane of date. They introduce a complementary term in their Hamiltonian to account for the motion of the reference frame with respect to the mean orbital plane of epoch J2000, which results in complementary secular variations of the longitude and obliquity of Mars BF (see their Sect. 5). Neglecting those complementary variations therefore approximately amounts to consider the mean orbital plane of epoch J2000 as the reference plane, so that comparison between the different nutation theories is possible, and done in Table 1 and Fig. 2.
To the best of our knowledge, Groten et al. (1996) are the only one to find an effect of the triaxiality on the scaling factor \(H_{{D}}\), and therefore on the precession rate and the main nutation terms. However, it has been shown that triaxiality does not change the main precession/nutation behavior of Mars (e.g., Newman 2013), but induces only small semi-diurnal nutations (e.g., BS99), as we find in this paper.
Note that they give the values of the integration constants in obliquity and longitude, but referred to the 1980 mean orbit. With their values for the angles J and N, orienting the 1980 orbit with respect to ICRF, we retrieve the observed values for \(\alpha _0\) and \(\delta _0\), from which we will obtain again the integration constant in obliquity and longitude, but this time referred to the J2000 mean orbit (\(\varepsilon _0=25^\circ .191819740\) and \(\theta _0=35^\circ .497525780\)).
In Ward’s model, nutations are expressed with respect to the orbit of the date (see also the complementary term in the Hamiltonian of BS99 to account for the motion of the orbit of date, resulting in additional secular variations of the longitude and obliquity of Mars BF), and not to the orbit of a chosen epoch.
The direct torque by the planets leads to a secular variation in obliquity \({\dot{E}}_{{Pl}}\), see Eq. 48b. This is why we do not necessarily assume here that \({\dot{E}}=0\).
The mean equinox BF is defined in Sect. 3.3, after Eqs. (16a, 16b). The transformation in Eq. 38 does not go to the mean equinox BF strictly speaking, but to a mean BF whose x-axis is in the direction of the node of the mean BF of date over the mean orbit of date (and not of epoch). Additional rotations can be introduced to account for this, but without noticeable effect on the solution.
Consider Eqs. (41) and (45) of Roosbeek and Dehant (1998), and the relation \(N_{fH}=N_{rh}-m\), to get \(N_{rH}=m H_{{D}}/(1+H_{{D}})\simeq m H_{{D}}\).
Roosbeek (1999) took \(t=0\) in his Eq. (3) (Roosbeek, personal communication). Therefore, the transformation from the IF goes to the J2000 BF and not the BF of date.
References
Archinal, B.A., Acton, C.H., A’Hearn, M.F., Conrad, A., Consolmagno, G.J., Duxbury, T., et al.: Report of the IAU Working Group on cartographic coordinates and rotational elements: 2015. Celest. Mech. Dyn. Astron. 130, 22 (2018). https://doi.org/10.1007/s10569-017-9805-5
Borderies, N.: Theory of Mars rotation in Euler angles. Astron. Astrophys. 82, 129–141 (1980)
Borderies, N., Balmino, G., Castel, L., Moynot, B.: Study of Mars dynamics from lander tracking data analysis. Moon Planets 22, 191–200 (1980). https://doi.org/10.1007/BF00898430
Bouquillon, S., Souchay, J.: Precise modeling of the precession-nutation of Mars. Astron. Astrophys. 345, 282–297 (1999)
Bretagnon, P.: Theorie du mouvement de l’ensemble des planetes (VSOP82). Astron. Astrophys. 114, 278 (1982)
Bretagnon, P., Francou, G.: Planetary theories in rectangular and spherical variables—VSOP 87 solutions. Astron. Astrophys. 202, 309–315 (1988)
Chapront-Touze, M.: Orbits of the Martian satellites from ESAPHO and ESADE theories. Astron. Astrophys. 240, 159–172 (1990)
Davies, M.E., Abalakin, V.K., Bursa, M., Hunt, G.E., Lieske, J.H., Morando, B., et al.: Report of the IAU/IAG/COSPAR working group on cartographic coordinates and rotational elements of the planets and satellites—1988. Celes. Mech. Dyn. Astron. 46, 187–204 (1989). https://doi.org/10.1007/BF00053048
Defraigne, P., Dehant, V., Pâquet, P.: Link between the retrograde-prograde nutations and nutations in obliquity and longitude. Celest. Mech. Dyn. Astron. 62(4), 363–376 (1995). https://doi.org/10.1007/BF00692286
Defraigne, P., de Viron, O., Dehant, V., Van Hoolst, T., Hourdin, F.: Mars rotation variations induced by atmosphere and ice caps. J. Geophys. Res. 105(E10), 24563–24570 (2000). https://doi.org/10.1029/1999JE001227
Dehant, V., Mathews, P.M.: Precession, Nutation and Wobble of the Earth. Cambridge University Press, Cambridge (2015)
Dehant, V., Defraigne, P., Van Hoolst, T.: Computation of Mars’ transfer functions for nutations, tides and surface loading. Phys. Earth Planet. Inter. 117(1–4), 385–395 (2000). https://doi.org/10.1016/S0031-9201(99)00108-9
Dehant, V., de Viron, O., Karatekin, O., van Hoolst, T.: Excitation of Mars polar motion. Astron. Astrophys. 446(1), 345–355 (2006). https://doi.org/10.1051/0004-6361:20053825
Dehant, V., Le Maistre, S., Baland, R.M., Bergeot, N., Karatekin, Ö., Péters, M.J., et al.: The radio-science LaRa instrument onboard ExoMars 2020: to investigate the rotation and interior of Mars. Planet. Space Sci. (2020). https://doi.org/10.1016/j.pss.2019.104776
Fienga, A., Laskar, J., Kuchynka, P., Manche, H., Desvignes, G., Gastineau, M., et al.: The INPOP10a planetary ephemeris and its applications in fundamental physics. Celest. Mech. Dyn. Astron. 111(3), 363–385 (2011). https://doi.org/10.1007/s10569-011-9377-8
Folkner, W.M., Kahn, R.D., Preston, R.A., Yoder, C.F., Standish, E.M., Williams, J.G., et al.: Mars dynamics from Earth-based tracking of the Mars Pathfinder lander. J. Geophys. Res. 102(E2), 4057–4064 (1997a). https://doi.org/10.1029/96JE02125
Folkner, W.M., Yoder, C.F., Yuan, D.N., Standish, E.M., Preston, R.A.: Interior structure and seasonal mass redistribution of Mars from radio tracking of Mars pathfinder. Science 278, 1749 (1997b). https://doi.org/10.1126/science.278.5344.1749
Folkner, W.M., Dehant, V., Le Maistre, S., Yseboodt, M., Rivoldini, A., Van Hoolst, T., et al.: The rotation and interior structure experiment on the InSight mission to Mars. Space Sci. Rev. 214, 100 (2018). https://doi.org/10.1007/s11214-018-0530-5
Fukushima, T.: Geodesic nutation. Astron. Astrophys. 244(1), L11 (1991)
Fukushima, T.: A new precession formula. Astron. J. 126(1), 494–534 (2003). https://doi.org/10.1086/375641
Groten, E., Molodenski, S.M., Zharkov, V.N.: On the theory of Mars’ forced nutation. Astron. J. 111, 1388 (1996). https://doi.org/10.1086/117885
Hilton, J.L.: The motion of Mars’ pole. I—rigid body precession and nutation. Astron. J. 102, 1510–1527 (1991). https://doi.org/10.1086/115977
Hilton, J.L.: The motion of Mars’ pole. II. The effect of an elastic mantle and a liquid core. Aastron. J. 103, 619 (1992). https://doi.org/10.1086/116090
Jacobson, R.A.: The orbits and masses of the Martian satellites and the libration of Phobos. Astron. J. 139(2), 668–679 (2010). https://doi.org/10.1088/0004-6256/139/2/668
Jacobson, R.A., Lainey, V.: Martian satellite orbits and ephemerides. Planet. Space Sci. 102, 35–44 (2014). https://doi.org/10.1016/j.pss.2013.06.003
Jacobson, R.A., Konopliv, A.S., Park, R.S., Folkner, W.M.: The rotational elements of Mars and its satellites. Planet. Space Sci. 152, 107–115 (2018). https://doi.org/10.1016/j.pss.2017.12.020
Khan, A., Liebske, C., Rozel, A., Rivoldini, A., Nimmo, F., Connolly, J.A.D., et al.: A geophysical perspective on the bulk composition of Mars. J. Geophys. Res. (Planets) 123(2), 575–611 (2018). https://doi.org/10.1002/2017JE005371
Konopliv, A.S., Yoder, C.F., Standish, E.M., Yuan, D.-N., Sjogren, W.L.: A global solution for the Mars static and seasonal gravity, Mars orientation, Phobos and Deimos masses, and Mars ephemeris. Icarus 182, 23–50 (2006). https://doi.org/10.1016/j.icarus.2005.12.025
Konopliv, A.S., Park, R.S., Folkner, W.M.: An improved JPL Mars gravity field and orientation from Mars orbiter and lander tracking data. Icarus 274, 253–260 (2016). https://doi.org/10.1016/j.icarus.2016.02.052
Konopliv, A.S., Asmar, S.W., Folkner, W.M., Karatekin, Ö., Nunes, D.C., Smrekar, S.E., et al.: Mars high resolution gravity fields from MRO, Mars seasonal gravity, and other dynamical parameters. Icarus 211(1), 401–428 (2011). https://doi.org/10.1016/j.icarus.2010.10.004
Kuchynka, P., Folkner, W.M., Konopliv, A.S., Parker, T.J., Park, R.S., Le Maistre, S., et al.: New constraints on Mars rotation determined from radiometric tracking of the Opportunity Mars Exploration Rover. Icarus 229, 340–347 (2014). https://doi.org/10.1016/j.icarus.2013.11.015
Laskar, J., Robutel, P.: Stability of the planetary three-body problem. I. Expansion of the planetary Hamiltonian. Celest. Mech. Dyn. Astron. 62(3), 193–217 (1995). https://doi.org/10.1007/BF00692088
Le Maistre, S.: The Rotation of Mars and Phobos from Earth-based Radio-tracking Observations of a Lander. PhD thesis. Université Catholique de Louvain (2013)
Le Maistre, S., Rosenblatt, P., Rivoldini, A., Dehant, V., Marty, J.-C., Karatekin, O.: Lander radio science experiment with a direct link between Mars and the Earth. Planet. Space Sci. 68, 105–122 (2012). https://doi.org/10.1016/j.pss.2011.12.020
Le Maistre, S., Dehant, V., Marty, J.-C.: Mars nutation estimates from radio-tracking of landed missions prior InSight and ExoMars 2020. In: European Planetary Science Congress, 12: EPSC2018-1238 (2018)
Le Maistre, S., Péters, M.J., Marty, J.-C., Dehant, V.: On the impact of the operational and technical characteristics of the LaRa experiment on the determination of Mars’ nutation. Planet. Space Sci. 180, 104766 (2020). https://doi.org/10.1016/j.pss.2019.104766
Lowrie, W.: A Student’s Guide to Geophysical Equations. Cambridge University Press, Cambridge (2011)
Lyttleton, R.A., Cain, D.L., Liu, A.S.: Nutation of Mars. Technical report (1979)
Mathews, P.M., Buffett, B.A., Herring, T.A., Shapiro, I.I.: Forced nutations of the earth: influence of inner core dynamics. I—Theory. II—numerical results and comparisons. III—very long interferometry data analysis. J. Geophys. Res. 96, 8219–8257 (1991). https://doi.org/10.1029/90JB01955
McCarthy, D.D.: IERS Conventions (1996). IERS Technical Note 21, pp. 1–95 (1996)
Moisson, X., Bretagnon, P.: Analytical planetary solution VSOP2000. Celest. Mech. Dyn. Astron. 80, 205–213 (2001)
Murray, C.D., Dermott, S.F.: Solar System Dynamics. Cambridge University Press, Cambridge (1999)
Newman, W.I.: Rotational kinematics and torques for triaxial bodies. Icarus 223(1), 615–618 (2013). https://doi.org/10.1016/j.icarus.2012.12.023
Péters, M.J., Le Maistre, S., Yseboodt, M., Marty, J.C., Rivoldini, A., Van Hoolst, T., et al.: LaRa after RISE: expected improvement in the rotation and interior models. Planet. Space Sci. (2020). https://doi.org/10.1016/j.pss.2019.104745
Reasenberg, R.D., King, R.W.: The rotation of Mars. J. Geophys. Res. 84, 6231–6240 (1979). https://doi.org/10.1029/JB084iB11p06231
Rivoldini, A., Van Hoolst, T., Verhoeven, O., Mocquet, A., Dehant, V.: Geodesy constraints on the interior structure and composition of Mars. Icarus 213, 451–472 (2011). https://doi.org/10.1016/j.icarus.2011.03.024
Roosbeek, F.: Analytical developments of rigid Mars nutation and tide generating potential series. Celest. Mech. Dyn. Astron. 75, 287–300 (1999)
Roosbeek, F., Dehant, V.: RDAN97: An analytical development of rigid Earth nutation series using the torque approach. Celest. Mech. Dyn. Astron. 70(4), 215–253 (1998). https://doi.org/10.1023/A:1008350710849
Sasao, T., Okubo, S., Saito, M.: A simple theory on dynamical effects of stratified fluid core upon nutational motion of the Earth. In Duncombe, R.L. (ed.) Nutation and the Earth’s Rotation, volume 78 of IAU Symposium, p. 165 (1980)
Simon, J.L., Bretagnon, P., Chapront, J., Chapront-Touze, M., Francou, G., Laskar, J.: Numerical expressions for precession formulae and mean elements for the Moon and the planets. Astron. Astrophys. 282, 663–683 (1994)
Simon, J.-L., Francou, G., Fienga, A., Manche, H.: New analytical planetary theories VSOP2013 and TOP2013. Astron. Astrophys. 557, A49 (2013). https://doi.org/10.1051/0004-6361/201321843
Sinclair, A.T.: The motions of the satellites of Mars. Mon. Not. R. Astron. Soc. 155, 249 (1972). https://doi.org/10.1093/mnras/155.3.249
Standish Jr., E.M.: Orientation of the JPL Ephemerides, DE 200/LE 200, to the dynamical equinox of J 2000. Astron. Astrophys. 114, 297–302 (1982)
Standish Jr., E.M., Newhall, X., Williams, J., Folkner, W.: JPL Interoffice Memorandum 314, 10 (1995)
Van den Acker, E., Van Hoolst, T., de Viron, O., Defraigne, P., Forget, F., Hourdin, F., et al.: Influence of the seasonal winds and the CO\(_{2}\) mass exchange between atmosphere and polar caps on Mars’ rotation. J. Geophys. Res. (Planets) 107(E7), 5055 (2002). https://doi.org/10.1029/2000JE001539
Ward, W.R.: Climatic variations on Mars: 1. Astronomical theory of insolation. J. Geophys. Res. 79(24), 3375 (1974). https://doi.org/10.1029/JC079i024p03375
Woolard, E.W.: Theory of the rotation of the earth around its center of mass. In: Astronomical Papers Prepared for the Use of the American Ephemeris and Nautical Almanac, vol. 15, p. 1 (1953)
Yoder, C.F., Konopliv, A.S., Yuan, D.N., Standish, E.M., Folkner, W.M.: Fluid core size of Mars from detection of the solar tide. Science 300, 299–303 (2003). https://doi.org/10.1126/science.1079645
Acknowledgements
We thank Fabian Roosbeek and Georges Carpentier for helping us to understand the computational procedure of RMAN99. We thank Michael Efroimsky and an anonymous reviewer for their constructive comments and suggestions that have helped to improve our paper. The research leading to these results has received funding from the Belgian PRODEX program managed by the European Space Agency in collaboration with the Belgian Federal Science Policy Office.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Baland, RM., Yseboodt, M., Le Maistre, S. et al. The precession and nutations of a rigid Mars. Celest Mech Dyn Astr 132, 47 (2020). https://doi.org/10.1007/s10569-020-09986-0
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10569-020-09986-0