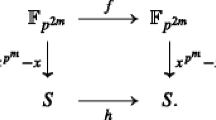Skip to main content
SpringerLink
Log in

Rédei permutations with cycles of the same length

  • Published:
Designs, Codes and Cryptography Aims and scope Submit manuscript

Abstract

Let \({\mathbb {F}}_{q}\) be a finite field of odd characteristic. We study Rédei functions that induce permutations over \(\mathbb {P}^1({\mathbb {F}}_{q})\) whose cycle decomposition contains only cycles of length 1 and j, for an integer \(j\ge 2\). When j is 4 or a prime number, we give necessary and sufficient conditions for a Rédei permutation of this type to exist over \(\mathbb {P}^1({\mathbb {F}}_{q})\), characterize Rédei permutations consisting of 1- and j-cycles, and determine their total number. We also present explicit formulas for Rédei involutions based on the number of fixed points, and procedures to construct Rédei permutations with a prescribed number of fixed points and j-cycles for \(j \in \{3,4,5\}\).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Ahmad S.: Cycle structure of automorphisms of finite cyclic groups. J. Comb. Theory 6(4), 370–374 (1969). https://doi.org/10.1016/S0021-9800(69)80032-3.

    Article  MathSciNet  MATH  Google Scholar 

  2. Barbero S., Cerruti U., Murru N.: Solving the Pell equation via Rédei rational functions. Fibonacci Quart. 48(4), 348–357 (2010).

    MathSciNet  MATH  Google Scholar 

  3. Bellini E., Murru N.: An efficient and secure RSA-like cryptosystem exploiting Rédei rational functions over conics. Finite Fields Appl. 39, 179–194 (2016). https://doi.org/10.1016/j.ffa.2016.01.011.

    Article  MathSciNet  MATH  Google Scholar 

  4. Castro F.N., Corrada-Bravo C., Pacheco-Tallaj N., Rubio I.: Explicit formulas for monomial involutions over finite fields. Adv. Math. Commun. 11(2), 301–306 (2017). https://doi.org/10.3934/amc.2017022.

    Article  MathSciNet  MATH  Google Scholar 

  5. Çeşmelioğlu A., Meidl W., Topuzoğlu A.: On the cycle structure of permutation polynomials. Finite Fields Appl. 14(3), 593–614 (2008). https://doi.org/10.1016/j.ffa.2007.08.003.

    Article  MathSciNet  MATH  Google Scholar 

  6. Charpin P., Mesnager S., Sarkar S.: Dickson polynomials that are involutions. In: Contemporary developments in finite fields and applications, pp. 22–47. World Science Publication, Hackensack (2016).

  7. Charpin P., Mesnager S., Sarkar S.: Involutions over the Galois field \(\mathbb{F}_{2^n}\). IEEE Trans. Inf. Theory 62(4), 2266–2276 (2016). https://doi.org/10.1109/TIT.2016.2526022.

    Article  MATH  Google Scholar 

  8. Chubb K., Panario D., Wang Q.: Fixed points of rational functions satisfying the Carlitz property. Appl. Algebra Eng. Commun. Comput. 30(5), 417–439 (2019). https://doi.org/10.1007/s00200-019-00382-2.

    Article  MathSciNet  MATH  Google Scholar 

  9. Fu S., Feng X., Lin D., Wang Q.: A recursive construction of permutation polynomials over \(\mathbb{F}_{q^2}\) with odd characteristic related to Rédei functions. Des. Codes Cryptogr. 87(7), 1481–1498 (2019). https://doi.org/10.1007/s10623-018-0548-4.

    Article  MathSciNet  MATH  Google Scholar 

  10. Gutierrez J., Winterhof A.: Exponential sums of nonlinear congruential pseudorandom number generators with Rédei functions. Finite Fields Appl. 14(2), 410–416 (2008). https://doi.org/10.1016/j.ffa.2007.03.004.

    Article  MathSciNet  MATH  Google Scholar 

  11. Kameswari P.A., Kumari R.C.: Cryptosystem with redei rational functions via pellconics. Int. J. Comput. Appl. 54(15), 1–6 (2012).

    Google Scholar 

  12. Lidl R., Mullen G.L.: Cycle structure of Dickson permutation polynomials. Math. J. Okayama Univ. 33, 1–11 (1991).

    MathSciNet  MATH  Google Scholar 

  13. Lidl R., Mullen G.L.: Unsolved Problems: When Does a Polynomial over a Finite Field Permute the Elements of the Field? II. Am. Math. Mon. 100(1), 71–74 (1993). https://doi.org/10.2307/2324822.

    Article  MathSciNet  MATH  Google Scholar 

  14. Mullen G.L. (ed.): Handbook of finite fields. Discrete Mathematics and Its Applications (Boca Raton). CRC Press, Boca Raton (2013). https://doi.org/10.1201/b15006.

  15. Murru N., Saettone F.M.: A novel RSA-like cryptosystem based on a generalization of the Rédei rational functions. In: Number-theoretic methods in cryptology. Lecture Notes in Computer Science, vol. 10737, pp. 91–103. Springer, Cham (2018).

  16. Niu T., Li K., Qu L., Wang Q.: New constructions of involutions over finite fields. Cryptogr. Commun. 12(2), 165–185 (2020). https://doi.org/10.1007/s12095-019-00386-2.

    Article  MathSciNet  MATH  Google Scholar 

  17. Niven I., Zuckerman H.S., Montgomery H.L.: An Introduction to the Theory of Numbers, 5th edn. Wiley, New York (1991).

    MATH  Google Scholar 

  18. Nöbauer R.: Cryptanalysis of the Rédei-scheme. Contributions to General Algebra, pp. 255–264. 3 (Vienna, 1984). Hölder-Pichler-Tempsky, Vienna (1985).

  19. Panario D., Reis L.: The functional graph of linear maps over finite fields and applications. Des. Codes Cryptogr. 87(2–3), 437–453 (2019). https://doi.org/10.1007/s10623-018-0547-5.

    Article  MathSciNet  MATH  Google Scholar 

  20. Qureshi C., Panario D.: Rédei actions on finite fields and multiplication map in cyclic group. SIAM J. Discret. Math. 29(3), 1486–1503 (2015). https://doi.org/10.1137/140993338.

    Article  MATH  Google Scholar 

  21. Rubio I.M., Corrada-Bravo C.J.: Cyclic decomposition of permutations of finite fields obtained using monomials. In: Finite fields and Applications. Lecture Notes in Computer Science, vol. 2948, pp. 254–261. Springer, Berlin (2004). https://doi.org/10.1007/978-3-540-24633-6-19.

  22. Rubio I.M., Mullen G.L., Corrada C., Castro F.N.: Dickson permutation polynomials that decompose in cycles of the same length. In: Finite Fields and Applications. Contemporary Mathematics, vol. 461, pp. 229–239. American Mathematical Society, Providence (2008). https://doi.org/10.1090/conm/461/08996.

  23. Sakzad A., Panario D., Sadeghi M., Eshghi N.: Self-inverse interleavers based on permutation functions for turbo codes. In: 2010 48th Annual Allerton Conference on Communication, Control, and Computing (Allerton), pp. 22–28 (2010).

  24. Sakzad A., Sadeghi M.R., Panario D.: Cycle structure of permutation functions over finite fields and their applications. Adv. Math. Commun. 6(3), 347–361 (2012).

    Article  MathSciNet  Google Scholar 

  25. Shanks D.: Five number-theoretic algorithms. In: Proceedings of the Second Manitoba Conference on Numerical Mathematics (Univ. Manitoba, Winnipeg, Man., 1972), pp. 51–70. Congressus Numerantium, No. VII (1973).

  26. Youssef A.M., Tavares S.E., Heys H.M.: A new class of substitution-permutation networks. Proc. 3rd Annu. Select. Areas Cryptogr. (SAC) pp. 132–147 (1996).

  27. Zheng D., Yuan M., Li N., Hu L., Zeng X.: Constructions of involutions over finite fields. IEEE Trans. Inf. Theory 65(12), 7876–7883 (2019). https://doi.org/10.1109/TIT.2019.2919511.

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Departamento de Matemática Pura e Aplicada, Universidade Federal do Rio Grande do Sul, Avenida Bento Gonçalves, 9500, Porto Alegre, RS, 91509-900, Brazil

    Juliane Capaverde & Virgínia M. Rodrigues

  2. Department of Mathematics, New York City College of Technology, The City University of New York, 300 Jay Street, Brooklyn, NY, 11201, USA

    Ariane M. Masuda

Authors
  1. Juliane Capaverde
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ariane M. Masuda
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Virgínia M. Rodrigues
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Juliane Capaverde.

Additional information

Communicated by P. Charpin.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Ariane M. Masuda received support for this project provided by a PSC-CUNY Grant, jointly funded by The Professional Staff Congress and The City University of New York.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Capaverde, J., Masuda, A.M. & Rodrigues, V.M. Rédei permutations with cycles of the same length. Des. Codes Cryptogr. 88, 2561–2579 (2020). https://doi.org/10.1007/s10623-020-00801-3

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10623-020-00801-3

Keywords

Search

Navigation