Skip to main content
SpringerLink
Log in

Analysis of the Stability of a Planetary System on Cosmogonic Time Scales

  • Published:
Astronomy Letters Aims and scope Submit manuscript

Abstract

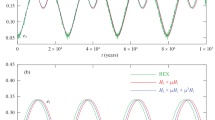
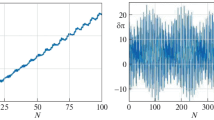
We consider the dynamical evolution of planetary systems whose structure is nearly circular and coplanar. The analysis is performed by the Hori–Deprit averaging method within the theory of the first order in planetary masses. A convenient set of canonical elements and a rarely employed variety of astrocentric coordinates are used to derive the equations of motion. Owing to the use of the chosen system of canonical elements, the expansions of the right-hand sides of the averaged equations contain a relatively small number of terms. Compared to other widespread coordinate systems, the astrocentric coordinates used by us allow a more convenient representation of the disturbing function to be obtained and do not require its expansion into a series in powers of a small parameter. On time scales \({\sim}10^{5}{-}10^{7}\) years we have studied the long-term evolution of the planetary systems HD 12661, \(\upsilon\) Andromedae, and some model systems by numerical integration of the averaged equations. Possible secular resonances have been revealed in the systems considered.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

Notes

  1. Recall that a particular solution of a system of ordinary differential equations is called Lagrange-stable if it remains definite and uniformly bounded at all \(t\geqslant t_{0}\), where \(t_{0}\) is the initial time. The Lagrangian triangular solutions in the general three-body problem can serve as an example of a Lagrange-stable system. A precise definition of the Lagrange stability can be found in the textbooks by Grebenikov (1976) and Demidovich (1967).

  2. Strictly speaking, small denominators appear even in the first order, see the first paragraph of Section 5.

  3. By the two-planet Sun–Jupiter–Saturn system Kuznetsov and Kholshevnikov (2006) mean a model two-planet system in which two planets with the masses and orbital parameters of Jupiter and Saturn revolve around a solar-mass star.

REFERENCES

  1. R. V. Baluev, Celest. Mech. Dyn. Astron. 111, 235 (2011).

    Article  ADS  Google Scholar 

  2. N. N. Bogolyubov and Yu. A. Mitropol’skii, Asymptotic Methods in the Theory of Nonlinear Oscillations (Gordon and Breach, London, 1961; Nauka, Moscow, 1974).

  3. V. V. Chazov, Solar System. Res. 47, 99 (2013).

    Article  ADS  Google Scholar 

  4. B. Cordani, Celest. Mech. Dyn. Astron. 89, 165 (2004).

    Article  ADS  MathSciNet  Google Scholar 

  5. B. P. Demidovich, Lectures on the Mathematical Theory of Stability (Nauka, Moscow, 1967) [in Russian].

    MATH  Google Scholar 

  6. G. E. O. Giacaglia, Perturbation Methods in Non-linear Systems (Springer, New York, 1972).

    Book  Google Scholar 

  7. E. A. Grebenikov, in Reference Book on Celestial Mechanics and Astrodynamics Ed. by G. N. Duboshin (Nauka, Moscow, 1976), p. 788 [in Russian].

    Google Scholar 

  8. E. A. Grebenikov, Averaging Method in Applied Problems (Nauka, Moscow, 1986) [in Russian].

    Google Scholar 

  9. E. Hairer, S. Nörsett, and G. Wanner, Solving Ordinary Differential Equations I: Nonstiff Problems (Springer, Berlin, Heidelberg, 1993).

    MATH  Google Scholar 

  10. K. V. Kholshevnikov, Asymptotic Methods of Celestial Mechanics (LGU, Leningrad, 1985) [in Russian].

    MATH  Google Scholar 

  11. K. V. Kholshevnikov and E. D. Kuznetsov, Celest. Mech. Dynam. Astron. 109, 201 (2011).

    Article  ADS  Google Scholar 

  12. K. V. Kholshevnikov, A. V. Greb, and E. D. Kuznetsov, Solar System. Res. 35, 243 (2001).

    Article  ADS  Google Scholar 

  13. K. V. Kholshevnikov, A. V. Greb, and E. D. Kuznetsov, Solar System. Res. 36, 68 (2002).

    Article  ADS  Google Scholar 

  14. O. M. Kochetova, V. B. Kuznetsov, Yu. D. Medvedev, Yu. A. Chernetenko, and V. A. Shor, Ephemerides of Minor Planets for 2020 (IAA RAS, St. Petersburg, 2019).

    Google Scholar 

  15. G. A. Krasinskiy, in Minor Planets, Collection of Articles, Ed. by N. S. Samoilova-Yakhontova (Nauka, Moscow, 1973), p. 81 [in Russian].

    Google Scholar 

  16. E. D. Kuznetsov and K. V. Kholshevnikov, Solar System. Res. 40, 239 (2006).

    Article  ADS  Google Scholar 

  17. E. D. Kuznetsov and K. V. Kholshevnikov, Solar System. Res. 43, 220 (2009).

    Article  ADS  Google Scholar 

  18. J. Laskar, Astron. Astrophys. 287, L9 (1994).

    ADS  Google Scholar 

  19. J. Laskar and P. Robutel, Celest. Mech. Dynam. Astron. 62, 193 (1995).

    Article  ADS  Google Scholar 

  20. M. H. Lee and S. J. Peale, Astrophys. J. 592, 1201 (2003).

    Article  ADS  Google Scholar 

  21. A.-S. Libert and N. Delsate, Mon. Not. R. Astron. Soc. 422, 2725 (2012).

    Article  ADS  Google Scholar 

  22. A.-S. Libert and M. Sansottera, Celest. Mech. Dynam. Astron. 117, 149 (2013).

    Article  ADS  Google Scholar 

  23. A. P. Markeev, Libration Points in Celestial Mechanics and Cosmodynamics (Nauka, Moscow, 1978) [in Russian].

    Google Scholar 

  24. D. V. Mikryukov, Astron. Lett. 42, 555 (2016).

    Article  ADS  Google Scholar 

  25. D. V. Mikryukov, Astron. Lett. 44, 337 (2018).

    Article  ADS  Google Scholar 

  26. D. V. Mikryukov and K. V. Kholshevnikov, Astron. Lett. 42, 268 (2016).

    Article  ADS  Google Scholar 

  27. Yu. A. Mitropol’skii, Averaging Method in Nonlinear Mechanics (Naukova Dumka, Kiev, 1971) [in Russian].

    Google Scholar 

  28. N. N. Moiseev, Asymptotic Methods of Nonlinear Mechanics (Nauka, Moscow, 1969) [in Russian].

    MATH  Google Scholar 

  29. A. Morbidelli, Modern Celestial Mechanics: Aspects of Solar System Dynamics (Taylor Francis, London, 2002).

    MATH  Google Scholar 

  30. C. Murray and S. Dermott, Solar System Dynamics (Cambridge Univ. Press, Cambridge, 2000).

    Book  Google Scholar 

  31. A. H. Nayfeh, Perturbation Methods (Wiley, New York, Chichester etc., 2000).

  32. A. S. Perminov and E. D. Kuznetsov, Solar System. Res. 49, 430 (2015).

    Article  ADS  Google Scholar 

  33. H. Poincaré, Leçons de Mécanique Céleste, tome I (Gauthier-Villars, Paris, 1905).

    MATH  Google Scholar 

  34. P. Robutel, L. Niederman, and A. Pousse, Comp. Appl. Math. 35, 675 (2016).

    Article  Google Scholar 

  35. A. Rodríguez and T. Gallardo, Astrophys. J. 628, 1006 (2005).

    Article  ADS  Google Scholar 

  36. M. Sansottera and A.-S. Libert, Celest. Mech. Dynam. Astron. 131, 38 (2019).

    Article  ADS  Google Scholar 

  37. I. I. Shevchenko, The Lidov-Kozai Effect—Applications in Exoplanet Research and Dynamical Astronomy (Springer, Switzerland, 2017).

    Book  Google Scholar 

  38. M. A. Vashkov’yak, S. N. Vashkov’yak, and N. V. Emel’yanov, Solar Syst. Res. 49, 191 (2015).

    Article  ADS  Google Scholar 

  39. V. M. Volosov and B. I. Morgunov, Averaging Method in the Theory of Nonlinear Oscillatory Systems (MGU, Moscow, 1971) [in Russian].

    MATH  Google Scholar 

  40. A. Wintner, The Analytical Foundations of Celestial Mechanics (Princeton Univ. Press, Princeton, 1941).

    MATH  Google Scholar 

Download references

ACKNOWLEDGMENTS

I thank K.V. Kholshevnikov for supervising the work and V.S. Shaidulin for his help in preparing the figures. I also express my gratitude to the anonymous referee for the fruitful comments and useful suggestions on the manuscript. All our computations were performed using the equipment of the Computing Center in the scientific park of the St. Petersburg State University. This work was financially supported by the Russian Science Foundation (project no. 19-72-10023).

Author information

Authors and Affiliations

  1. St. Petersburg State University, Universitetskii pr. 28, Petrodvoretz, St. Petersburg, 198504, Russia

    D. V. Mikryukov

Authors
  1. D. V. Mikryukov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to D. V. Mikryukov.

Additional information

Translated by V. Astakhov

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Mikryukov, D.V. Analysis of the Stability of a Planetary System on Cosmogonic Time Scales. Astron. Lett. 46, 344–358 (2020). https://doi.org/10.1134/S1063773720050059

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1063773720050059

Keywords:

Search

Navigation