Trigonometric Rosen–Morse Potential as a Quark–Antiquark Interaction Potential for Meson Properties in the Non-relativistic Quark Model Using EAIM

Abstract

Trigonometric Rosen–Morse potential is suggested as a quark–antiquark interaction potential for studying thermodynamic properties and masses of heavy and heavy–light mesons. For this purpose, the N-radial Schrödinger equation is analytically solved using an exact-analytical iteration method. The energy eigenvalues and corresponding wave functions are obtained in the N-space. The present results are applied in calculating the mass of mesons such as charmonium c ${\bar{\hbox {c}}}$, bottomonium b ${\bar{\hbox {b}}}$, b ${\bar{\hbox {c}}},$ and c ${\bar{\hbox {s}}}$ mesons and thermodynamic properties such as the mean internal energy, the specific heat, the free energy, and the entropy. The effect of dimensional number is studied on the meson properties. The present results are improved in comparison with other recent works and are in good agreement in comparison with experimental data. Thus, the present potential provides satisfied results in comparison with other works and experimental data.

Appendix A

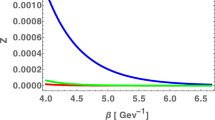
In this appendix, thermodynamics properties of the Trigonometric Rosen–Morse potential are studied, the partition function is given $\hbox {Z}=\mathop \sum \nolimits _{n=0}^\infty e^{-\beta E}$, where $\upbeta = \frac{1}{K T}$, K is the Boltzmann constant as in Ref. [1]

1.1 Partition function

$$\begin{aligned} \hbox {Z}\left( {\upbeta } \right) = \mathop \sum \limits _{n=0}^\infty e^{-\beta E_{nl}}, \end{aligned}$$

(A1)

by substituting Eq. (28), we obtain

$$\begin{aligned} \hbox {Z}\left( {\upbeta } \right) =\frac{e^{-\beta \left( \hbox {C}_{1}+\hbox {C}_{2}-\hbox {C}_{3} \right) }}{\left( 1-e^{-\beta \hbox {C}_{1}} \right) }, \end{aligned}$$

(A2)

where,

$$\begin{aligned} \hbox {C}_{1} \quad =\frac{1}{\mu }\sqrt{\frac{a(a+1)}{15d^{4}}},\quad \hbox {C}_{2}=\frac{l^{'}}{2\mu }\sqrt{\frac{a(a+1)}{15d^{4}} },\quad {\hbox { C}}_{3}= \frac{5}{6\mu }\frac{b^{2}}{a(a+1)d^{2}},\quad l^{'}= \sqrt{{8 \upmu \hbox {C+4}\left( \hbox {L+}\frac{N\hbox {-2}}{\hbox {2}} \right) ^{\mathrm{2}}}}.\nonumber \\ \end{aligned}$$

(A3)

1.2 Mean energy U

$$\begin{aligned} \hbox {U}\left( {\upbeta } \right)= & {} -\,\frac{\hbox {d}}{{\hbox {d}}\upbeta }\hbox {LnZ}\left( {\upbeta } \right) , \end{aligned}$$

(A4)

$$\begin{aligned} U\left( \beta \right)= & {} -\,e^{\beta \left( C_{1}+C_{2}-C_{3} \right) }\left( 1-e^{-\beta C_{1}} \right) \left( -\frac{e^{-\beta C_{1}-\beta \left( C_{1}+C_{2}-C_{3} \right) }C_{1}}{\left( 1-e^{-\beta C_{1}} \right) ^{2}}+\frac{e^{-\beta \left( C_{1}+C_{2}-C_{3} \right) }\left( -C_{1}-C_{2}+C_{3} \right) }{1-e^{-\beta C_{1}}} \right) .\nonumber \\ \end{aligned}$$

(A5)

1.3 Specific heat C

$$\begin{aligned} C(\beta )= & {} \frac{dU}{dT} = -\, \hbox {K} \beta ^{2} \frac{dU}{d\beta }, \end{aligned}$$

(A6)

$$\begin{aligned} C\left( \beta \right)= & {} -\,K\beta ^{2}\left( -\,e^{-\beta C_{1}+\beta \left( C_{1}+C_{2}-C_{3} \right) }C_{1}\left( -\frac{e^{-\beta C_{1}-\beta \left( C_{1}+C_{2}-C_{3} \right) }C_{1}}{\left( 1-e^{-\beta C_{1}} \right) ^{2}}\right. \right. \nonumber \\&\left. \left. +\,\frac{e^{-\beta \left( C_{1}+C_{2}-C_{3} \right) }\left( -C_{1}-C_{2}+C_{3} \right) }{1-e^{-\beta C_{1}}}\right) \right. \nonumber \\&\left. -\,e^{\beta \left( C_{1}+C_{2}-C_{3} \right) }(1-e^{-\beta C_{1}})(C_{1}+C_{2}-C_{3})\left( -\frac{e^{-\beta C_{1}-\beta \left( C_{1}+C_{2}-C_{3} \right) }C_{1}}{\left( 1-e^{-\beta C_{1}} \right) ^{2}}\right. \right. \nonumber \\&\left. +\,\frac{e^{-\beta \left( C_{1}+C_{2}-C_{3} \right) }\left( -C_{1}-C_{2}+C_{3} \right) }{1-e^{-\beta C_{1}}}\right) \nonumber \\&-\,e^{\beta \left( C_{1}+C_{2}-C_{3} \right) }(1-e^{-\beta C_{1}})\left( \frac{2e^{-2\beta C_{1}-\beta \left( C_{1}+C_{2}-C_{3} \right) }C_{1}^{2}}{\left( 1-e^{-\beta C_{1}} \right) ^{3}}\right. \nonumber \\&-\,\frac{e^{-\beta C_{1}-\beta \left( C_{1}+C_{2}-C_{3} \right) }C_{1}\left( -2C_{1}-C_{2}+C_{3} \right) }{\left( 1-e^{-\beta C_{1}} \right) ^{2}}-\frac{e^{-\beta C_{1}-\beta \left( C_{1}+C_{2}-C_{3} \right) }C_{1}\left( -C_{1}-C_{2}+C_{3} \right) }{\left( 1-e^{-\beta C_{1}} \right) ^{2}}\nonumber \\&\left. \left. +\,\frac{e^{-\beta \left( C_{1}+C_{2}-C_{3} \right) }\left( -C_{1}-C_{2}+C_{3} \right) ^{2}}{1-e^{-\beta C_{1}}}\right) \right) , \end{aligned}$$

(A7)

1.4 Free energy

$$\begin{aligned} \hbox {F}(\upbeta )= & {} -\,\hbox {KT }\,\hbox {LnZ}(\upbeta ), \nonumber \\ \hbox {F}\left( {\upbeta } \right)= & {} -\,\frac{\hbox {Log}\left[ \frac{e^{-\beta (C_{1}+C_{2}-C_{3})}}{(1-e^{-\beta C_{1}})}\right] }{\beta } \end{aligned}$$

(A8)

1.5 Entropy

$$\begin{aligned} \hbox {S}(\upbeta )= & {} \hbox {K}\,\hbox {ln}\,\hbox {Z}(\upbeta ) -\hbox {K}\,{ \upbeta } \frac{\partial }{\partial {\upbeta }} \hbox {ln}{\hbox {Z}(\upbeta )}, \nonumber \\ \hbox {S}\left( {\upbeta }\right)= & {} \hbox {K}\,\hbox {Log}\left[ \frac{\hbox {e}^{{-\upbeta }\left( \hbox {C}_{\mathrm{1}}+\hbox {C}_{\mathrm{2}}-\hbox {C}_{\mathrm{3}} \right) }}{\hbox {1}-\hbox {e}^{{-\upbeta }\hbox {C}_{\mathrm{1}}}}\right] \nonumber \\&-\,\hbox {e}^{{\upbeta }\left( \hbox {C}_{\mathrm{1}}+\hbox {C}_{\mathrm{2}}-\hbox {C}_{\mathrm{3}} \right) }\hbox {(1}-\hbox {e}^{{-\upbeta }\hbox {C}_{\mathrm{1}}})\hbox {K}\upbeta \nonumber \\&\left( \,-\frac{\hbox {e}^{{-\upbeta }\hbox {C}_{\mathrm{1}}{-\upbeta }\left( \hbox {C}_{\mathrm{1}}+\hbox {C}_{\mathrm{2}}-\hbox {C}_{\mathrm{3}} \right) }\hbox {C}_{\mathrm{1}}}{\left( \hbox {1}-\hbox {e}^{{-\upbeta }\hbox {C}_{\mathrm{1}}} \right) ^{\mathrm{2}}}+\frac{\hbox {e}^{{-\upbeta }\left( \hbox {C}_{\mathrm{1}}+\hbox {C}_{\mathrm{2}}-\hbox {C}_{\mathrm{3}} \right) }\left( -\,\hbox {C}_{\mathrm{1}}-\hbox {C}_{\mathrm{2}}+\hbox {C}_{\mathrm{3}} \right) }{\hbox {1}-\hbox {e}^{{-\upbeta }\hbox {C}_{\mathrm{1}}}}\right) . \end{aligned}$$

(A9)