Skip to main content
SpringerLink
Log in

Hybrid lattice metamaterials with auxiliary resonators made of functionally graded materials

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

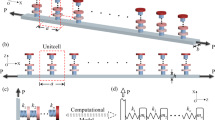
This paper aims to enhance and tune wave-propagation characteristics of periodic architected structures by adding auxiliary resonators made of functionally graded materials (FGMs). For this purpose, cantilever FGM beams are added to periodic metamaterials with square and hexagonal topologies, and the effects of the material distribution of the added resonators on their wave-attenuation performance are analyzed. More specifically, a combination of locally resonant and Bragg-type bandgaps is formed as a result of adding FGM resonators, while the conventional structures have no bandgap in the considered region. The studied low-frequency region is of high importance, and the appearance of wide bandgaps there opens horizons for new structural and acoustic applications. Further, these bandgaps depend on the material parameters of the resonators, and their location and width are changed, systematically as functions of the elastic modulus ratio, density ratio, and non-negative power-law exponent of the resonators. For the numerical analysis, a finite element formulation is developed for an FGM beam, and the wave propagation is studied using Bloch’s theorem. According to the results, with increasing the elastic modulus contrast of the auxiliary FGM resonators, the locations of the bandgaps move higher, while increasing the density ratio contrast moves them to lower frequencies. Additionally, the effect of adding auxiliary FGM resonators on the directionality of the wave propagation is studied using the iso-frequency contours of the first dispersion branches of each structure. The results of the present study can be a starting point for using FGM resonators to design tunable elastic/acoustic metamaterials with the ability to filter waves in predefined frequency ranges.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  1. Kushwaha, M.S., Halevi, P., Martínez, G., Dobrzynski, L., Djafari-Rouhani, B.: Theory of acoustic band structure of periodic elastic composites. Phys. Rev. B 49, 2313–2322 (1994). https://doi.org/10.1103/PhysRevB.49.2313

    Article  Google Scholar 

  2. Ruzzene, M., Scarpa, F., Soranna, F.: Wave beaming effects in two-dimensional cellular structures. Smart Mater. Struct. 12, 363 (2003)

    Article  Google Scholar 

  3. Zhang, S., Xia, C., Fang, N.: Broadband acoustic cloak for ultrasound waves. Phys. Rev. Lett. 106, 1–4 (2011). https://doi.org/10.1103/PhysRevLett.106.024301

    Article  Google Scholar 

  4. Richards, D., Pines, D.J.: Passive reduction of gear mesh vibration using a periodic drive shaft. J. Sound Vib. 264, 317–342 (2003). https://doi.org/10.1016/S0022-460X(02)01213-0

    Article  Google Scholar 

  5. Sánchez-Pérez, J.V., Caballero, D., Mártinez-Sala, R., Rubio, C., Sánchez-Dehesa, J., Meseguer, F., Llinares, J., Gálvez, F.: Sound attenuation by a two-dimensional array of rigid cylinders. Phys. Rev. Lett. 80, 5325–5328 (1998). https://doi.org/10.1103/PhysRevLett.80.5325

    Article  Google Scholar 

  6. Hussein, M.I., Leamy, M.J., Ruzzene, M.: Dynamics of phononic materials and structures: historical origins, recent progress, and future outlook. Appl. Mech. Rev. 66, 040802 (2014). https://doi.org/10.1115/1.4026911

    Article  Google Scholar 

  7. Schaedler, T.A., Carter, W.B.: Architected cellular materials. Annu. Rev. Mater. Res. 46, 187–210 (2016). https://doi.org/10.1146/annurev-matsci-070115-031624

    Article  Google Scholar 

  8. Bitzer, T.: Honeycomb Technology. Springer, Dordrecht (1997)

    Book  Google Scholar 

  9. Charlier, J.: Building carbon nanotube based network architectures. Smart Mater. Struct. 11, 1–4 (2018)

    MathSciNet  Google Scholar 

  10. Zok, F.W., Rathbun, H.J., Wei, Z., Evans, A.G.: Design of metallic textile core sandwich panels. Int. J. Solids Struct. 40, 5707–5722 (2003)

    Article  Google Scholar 

  11. Xue, Z., Hutchinson, J.W.: Crush dynamics of square honeycomb sandwich cores. Int. J. Numer. Methods Eng. 65, 2221–2245 (2006). https://doi.org/10.1002/nme.1535

    Article  MATH  Google Scholar 

  12. Gonella, S., Ruzzene, M.: Homogenization and equivalent in-plane properties of two-dimensional periodic lattices. Int. J. Solids Struct. 45, 2897–2915 (2008). https://doi.org/10.1016/j.ijsolstr.2008.01.002

    Article  MATH  Google Scholar 

  13. Mousanezhad, D., Haghpanah, B., Ghosh, R., Hamouda, A.M., Nayeb-Hashemi, H., Vaziri, A.: Elastic properties of chiral, anti-chiral, and hierarchical honeycombs: a simple energy-based approach. Theor. Appl. Mech. Lett. 6, 81–96 (2016). https://doi.org/10.1016/j.taml.2016.02.004

    Article  Google Scholar 

  14. Espo, M., Abolbashari, M.H., Hosseini, S.M.: Band structure analysis of wave propagation in piezoelectric nano-metamaterials as periodic nano-beams considering the small scale and surface effects. Acta Mech. (2020). https://doi.org/10.1007/s00707-020-02678-8

    Article  MathSciNet  MATH  Google Scholar 

  15. Bacigalupo, A., De Bellis, M.L., Gnecco, G.: Complex frequency band structure of periodic thermo-diffusive materials by Floquet–Bloch theory. Acta Mech. 230, 3339–3363 (2019)

    Article  MathSciNet  Google Scholar 

  16. Bacigalupo, A., Lepidi, M.: Acoustic wave polarization and energy flow in periodic beam lattice materials. Int. J. Solids Struct. 147, 183–203 (2018)

    Article  Google Scholar 

  17. Phani, A.S., Woodhouse, J., Fleck, N.A.: Wave propagation in two-dimensional periodic lattices. J. Acoust. Soc. Am. 119, 1995–2005 (2006)

    Article  Google Scholar 

  18. Kittel, C.: Introduction to Solid State Physics. Chapman & Hall, New York (1953)

    MATH  Google Scholar 

  19. Brillouin, L.: Wave Propagation in Periodic Structures: Electric Filters and Crystal Lattices. Dover Publications Inc, Mineola (1953)

    MATH  Google Scholar 

  20. Sheng, P., Zhang, X.X., Liu, Z., Chan, C.T.: Locally resonant sonic materials. Physica B 338, 201–205 (2003)

    Article  Google Scholar 

  21. Raghavan, L., Phani, A.S.: Local resonance bandgaps in periodic media: theory and experiment. J. Acoust. Soc. Am. 134, 1950–1959 (2013). https://doi.org/10.1121/1.4817894

    Article  Google Scholar 

  22. Hirsekorn, M.: Small-size sonic crystals with strong attenuation bands in the audible frequency range. Appl. Phys. Lett. 84, 3364–3366 (2004). https://doi.org/10.1063/1.1723688

    Article  Google Scholar 

  23. Chang, I.L., Liang, Z.X., Kao, H.W., Chang, S.H., Yang, C.Y.: The wave attenuation mechanism of the periodic local resonant metamaterial. J. Sound Vib. 412, 349–359 (2018). https://doi.org/10.1016/j.jsv.2017.10.008

    Article  Google Scholar 

  24. Lepidi, M., Bacigalupo, A.: Multi-parametric sensitivity analysis of the band structure for tetrachiral acoustic metamaterials. Int. J. Solids Struct. 136–137, 186–202 (2018). https://doi.org/10.1016/j.ijsolstr.2017.12.014

    Article  Google Scholar 

  25. Hsu, J.C.: Local resonances-induced low-frequency band gaps in two-dimensional phononic crystal slabs with periodic stepped resonators. J. Phys. D Appl. Phys. 44, 055401 (2011). https://doi.org/10.1088/0022-3727/44/5/055401

    Article  Google Scholar 

  26. Pelat, A., Gallot, T., Gautier, F.: On the control of the first Bragg band gap in periodic continuously corrugated beam for flexural vibration. J. Sound Vib. 446, 249–262 (2019). https://doi.org/10.1016/j.jsv.2019.01.029

    Article  Google Scholar 

  27. Wang, P., Casadei, F., Kang, S.H., Bertoldi, K.: Locally resonant band gaps in periodic beam lattices by tuning connectivity. Phys. Rev. B Condens. Matter Mater. Phys. 91, 2–5 (2015)

    Google Scholar 

  28. Schneider, D., Liaqat, F., El Boudouti, E.H., El Hassouani, Y., Djafari-Rouhani, B., Tremel, W., Butt, H.J., Fytas, G.: Engineering the hypersonic phononic band gap of hybrid Bragg stacks. Nano Lett. 12, 3101–3108 (2012). https://doi.org/10.1021/nl300982d

    Article  Google Scholar 

  29. Vadalà., F., Bacigalupo, A., Lepidi, M., Gambarotta, L.: Free and forced wave propagation in beam lattice metamaterials with viscoelastic resonators (2019). arXiv preprint arXiv.1911.00455

  30. Xiao, Y., Wen, J., Wang, G., Wen, X.: Theoretical and experimental study of locally resonant and Bragg band gaps in flexural beams carrying periodic arrays of beam-like resonators. J. Vib. Acoust. Trans. ASME (2013). https://doi.org/10.1115/1.4024214

    Article  Google Scholar 

  31. Krushynska, A.O., Miniaci, M., Bosia, F., Pugno, N.M.: Coupling local resonance with Bragg band gaps in single-phase mechanical metamaterials. Extreme Mech. Lett. 12, 30–36 (2017). https://doi.org/10.1016/j.eml.2016.10.004

    Article  Google Scholar 

  32. Kaina, N., Fink, M., Lerosey, G.: Composite media mixing Bragg and local resonances for highly attenuating and broad bandgaps. Sci. Rep. 3, 11–13 (2013). https://doi.org/10.1038/srep03240

    Article  Google Scholar 

  33. Liu, L., Hussein, M.I.: Wave motion in periodic flexural beams and characterization of the transition between Bragg scattering and local resonance. J. Appl. Mech. Trans. ASME 79, 1–17 (2012). https://doi.org/10.1115/1.4004592

    Article  Google Scholar 

  34. Olhoff, N., Niu, B., Cheng, G.: Optimum design of band-gap beam structures. Int. J. Solids Struct. 49, 3158–3169 (2012). https://doi.org/10.1016/j.ijsolstr.2012.06.014

    Article  Google Scholar 

  35. Trainiti, G., Rimoli, J.J., Ruzzene, M.: Wave propagation in undulated structural lattices. Int. J. Solids Struct. 97–98, 431–444 (2016). https://doi.org/10.1016/j.ijsolstr.2016.07.006

    Article  Google Scholar 

  36. Wen, S., Xiong, Y., Hao, S., Li, F., Zhang, C.: Enhanced band-gap properties of an acoustic metamaterial beam with periodically variable cross-sections. Int. J. Mech. Sci. 166, 105229 (2020). https://doi.org/10.1016/j.ijmecsci.2019.105229

    Article  Google Scholar 

  37. Gao, F., Wu, Z., Li, F., Zhang, C.: Numerical and experimental analysis of the vibration and band-gap properties of elastic beams with periodically variable cross sections. Waves Random Complex Media 29, 299–316 (2019). https://doi.org/10.1080/17455030.2018.1430918

    Article  Google Scholar 

  38. Zhang, K., Su, Y., Hou, X., Meng, J., Deng, Z.: Effect of pre-load on wave propagation characteristics of hexagonal lattices. Compos. Struct. 203, 361–372 (2018). https://doi.org/10.1016/j.compstruct.2018.07.033

    Article  Google Scholar 

  39. Spadoni, A., Ruzzene, M., Gonella, S., Scarpa, F.: Phononic properties of hexagonal chiral lattices. Wave Motion 46, 435–450 (2009). https://doi.org/10.1016/j.wavemoti.2009.04.002

    Article  MathSciNet  MATH  Google Scholar 

  40. Tee, K.F., Spadoni, A., Scarpa, F., Ruzzene, M.: Wave propagation in auxetic tetrachiral honeycombs. J. Vib. Acoust. Trans. ASME 132, 0310071–0310078 (2010). https://doi.org/10.1115/1.4000785

    Article  Google Scholar 

  41. Liu, W., Chen, J.W., Su, X.Y.: Local resonance phononic band gaps in modified two-dimensional lattice materials. Acta Mech. Sinica/Lixue Xuebao 28, 659–669 (2012). https://doi.org/10.1007/s10409-012-0031-9

    Article  Google Scholar 

  42. Lal, R., Saini, R.: On the use of GDQ for vibration characteristic of non-homogeneous orthotropic rectangular plates of bilinearly varying thickness. Acta Mech. 226, 1605–1620 (2015). https://doi.org/10.1007/s00707-014-1272-4

    Article  MathSciNet  MATH  Google Scholar 

  43. Jha, D.K., Kant, T., Singh, R.K.: A critical review of recent research on functionally graded plates. Compos. Struct. 96, 833–849 (2013). https://doi.org/10.1016/J.COMPSTRUCT.2012.09.001

    Article  Google Scholar 

  44. Jennings, J.M., Vaidyanathan, R., Kar, A.: Theoretical and experimental studies of electrical conductivity for functionally graded, heterogeneous surfaces. J. Appl. Phys. 125, 035106 (2019). https://doi.org/10.1063/1.5079556

    Article  Google Scholar 

  45. Akgöz, B., Civalek, Ö.: Effects of thermal and shear deformation on vibration response of functionally graded thick composite microbeams. Compos. B Eng. 129, 77–87 (2017). https://doi.org/10.1016/j.compositesb.2017.07.024

    Article  Google Scholar 

  46. Avcar, M.: Free vibration of imperfect sigmoid and power law functionally graded beams. Steel Compos. Struct. 30, 603–615 (2019). https://doi.org/10.12989/scs.2019.30.6.603

    Article  Google Scholar 

  47. Aydogdu, M., Taskin, V.: Free vibration analysis of functionally graded beams with simply supported edges. Mater. Des. 28, 1651–1656 (2007). https://doi.org/10.1016/j.matdes.2006.02.007

    Article  Google Scholar 

  48. Alshorbagy, A.E., Eltaher, M.A., Mahmoud, F.F.: Free vibration characteristics of a functionally graded beam by finite element method. Appl. Math. Model. 35, 412–425 (2011)

    Article  MathSciNet  Google Scholar 

  49. Azizi, S., Chorsi, M.T., Bakhtiari-Nejad, F.: On the secondary resonance of a MEMS resonator: a conceptual study based on shooting and perturbation methods. Int. J. Non-Linear Mech. 82, 59–68 (2016). https://doi.org/10.1016/j.ijnonlinmec.2016.02.003

    Article  Google Scholar 

  50. Akgöz, B., Civalek, Ö.: A microstructure-dependent sinusoidal plate model based on the strain gradient elasticity theory. Acta Mech. 226, 2277–2294 (2015). https://doi.org/10.1007/s00707-015-1308-4

    Article  MathSciNet  MATH  Google Scholar 

  51. Ebrahimi, F., Barati, M.R., Civalek, Ö.: Application of Chebyshev-Ritz method for static stability and vibration analysis of nonlocal microstructure-dependent nanostructures. Eng. Comput. 36, 953–964 (2020). https://doi.org/10.1007/s00366-019-00742-z

    Article  Google Scholar 

  52. Akgöz, B., Civalek, Ö.: A size-dependent beam model for stability of axially loaded carbon nanotubes surrounded by Pasternak elastic foundation. Compos. Struct. 176, 1028–1038 (2017). https://doi.org/10.1016/j.compstruct.2017.06.039

    Article  Google Scholar 

  53. Niknam, H., Akbarzadeh, A.H.: Thermo-mechanical bending of architected functionally graded cellular beams. Compos. B Eng. 174, 107060 (2019). https://doi.org/10.1016/j.compositesb.2019.107060

    Article  Google Scholar 

  54. Wu, M.-L., Wu, L., Yang, W., Chen, L.: Elastic wave band gaps of one-dimensional phononic crystals with functionally graded materials. Smart Mater. Struct. 18, 115013 (2009). https://doi.org/10.1088/0964-1726/18/11/115013

    Article  Google Scholar 

  55. Guo, X., Wei, P., Lan, M., Li, L.: Dispersion relations of elastic waves in one-dimensional piezoelectric/piezomagnetic phononic crystal with functionally graded interlayers. Ultrasonics 70, 158–171 (2016). https://doi.org/10.1016/J.ULTRAS.2016.04.025

    Article  Google Scholar 

  56. Sepehri, S., Jafari, H., Mashhadi, M.M., Yazdi, M.R.H., Fakhrabadi, M.M.S.: Tunable elastic wave propagation in planar functionally graded metamaterials. Acta Mech. 231, 3363–3385 (2020). https://doi.org/10.1007/s00707-020-02705-8

    Article  MathSciNet  Google Scholar 

  57. Chuang, K.C., Lv, X.F., Wang, D.F.: A tunable elastic metamaterial beam with flat-curved shape memory alloy resonators. Appl. Phys. Lett. 114, 051903 (2019). https://doi.org/10.1063/1.5084548

    Article  Google Scholar 

  58. Zhu, R., Huang, G.L., Huang, H.H., Sun, C.T.: Experimental and numerical study of guided wave propagation in a thin metamaterial plate. Phys. Lett. A 375, 2863–2867 (2011). https://doi.org/10.1016/j.physleta.2011.06.006

    Article  Google Scholar 

  59. De Sousa, V.C., Tan, D., De Marqui, C., Erturk, A.: Tunable metamaterial beam with shape memory alloy resonators: theory and experiment. Appl. Phys. Lett. 113, 143502 (2018). https://doi.org/10.1063/1.5050213

    Article  Google Scholar 

  60. Naebe, M., Shirvanimoghaddam, K.: Functionally graded materials: a review of fabrication and properties. Appl. Mater. Today 5, 223–245 (2016). https://doi.org/10.1016/j.apmt.2016.10.001

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mechanical Engineering, College of Engineering, University of Tehran, Tehran, Iran

    Hamid Jafari, Soroush Sepehri, Mohammad Reza Hairi Yazdi, Mahmoud Mosavi Mashhadi & Mir Masoud Seyyed Fakhrabadi

Authors
  1. Hamid Jafari
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Soroush Sepehri
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Mohammad Reza Hairi Yazdi
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Mahmoud Mosavi Mashhadi
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Mir Masoud Seyyed Fakhrabadi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mir Masoud Seyyed Fakhrabadi.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

The shape functions for the 6-degrees-of-freedom frame element with length l are presented in the current manuscript:

$$\begin{aligned} {\xi _1}= & {} \left( {1 - \frac{x}{l}} \right) , \end{aligned}$$
(A.1)
$$\begin{aligned} {\xi _2}= & {} \left( {\frac{1}{{{l^3}}}} \right) ( {2{x^3} - 3{x^2}l + {l^3}} ) ,\end{aligned}$$
(A.2)
$$\begin{aligned} {\xi _3}= & {} \left( {\frac{1}{{{l^3}}}} \right) ( {{x^3}l - 2{x^2}{l^2} + x{l^3}} ), \end{aligned}$$
(A.3)
$$\begin{aligned} {\xi _4}= & {} \frac{x}{l} ,\end{aligned}$$
(A.4)
$$\begin{aligned} {\xi _5}= & {} \left( {\frac{1}{{{l^3}}}} \right) ( { - 2{x^3} + 3{x^2}l} ) ,\end{aligned}$$
(A.5)
$$\begin{aligned} {\xi _6}= & {} \left( {\frac{1}{{{l^3}}}} \right) ( {{x^3}l - {x^2}{l^2}} ). \end{aligned}$$
(A.6)

where x denotes the local coordinate of the element.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jafari, H., Sepehri, S., Yazdi, M.R.H. et al. Hybrid lattice metamaterials with auxiliary resonators made of functionally graded materials. Acta Mech 231, 4835–4849 (2020). https://doi.org/10.1007/s00707-020-02799-0

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-020-02799-0

Search

Navigation