Skip to main content
SpringerLink
Log in

A polynomial chaos expansion approach for nonlinear dynamic systems with interval uncertainty

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

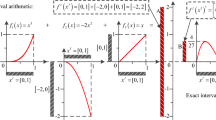
Abstract

This paper proposes a non-intrusive interval uncertainty analysis method for estimation of the dynamic response bounds of nonlinear systems with uncertain-but-bounded parameters using polynomial chaos expansion. The conventional interval arithmetic and Taylor series methods usually lead to large overestimation because of the intrinsic wrapping effect, especially for the multidimensional and non-monotonic problems. To overcome this drawback, a novel polynomial chaos inclusion function, based on the truncated polynomial chaos expansion, is proposed in the present work to evaluate interval functions. In this method, the Legendre polynomial in interval space is employed as the trial basis to expand the interval processes, and the polynomial coefficients are calculated through the collocation method. Two examples show that the polynomial chaos inclusion function is capable of determining tighter enclosures of the true solutions and effectively dealing with the wrapping effect. The response of nonlinear systems with respect to interval variables is approximated by the polynomial chaos inclusion function, through which the supremum and infimum of the dynamic responses over all time iteration steps can be easily estimated by an appropriate numerical solver. Four dynamics examples described by ordinary differential equations demonstrate the effectiveness, feasibility, and efficiency of the proposed interval uncertainty analysis method compared with other methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  1. Su-Huan, C., Zhong-Sheng, L., Zong-Fen, Z.: Random vibration analysis for large-scale structures with random parameters. Comput. Struct. 43, 681–685 (1992). https://doi.org/10.1016/0045-7949(92)90509-X

    Article  Google Scholar 

  2. Schuëller, G.I., Jensen, H.A.: Computational methods in optimization considering uncertainties—An overview Comput. Methods Appl. Mech. Eng. 198, 2–13 (2008). https://doi.org/10.1016/j.cma.2008.05.004

    Article  MATH  Google Scholar 

  3. McWilliam, S.: Anti-optimization of uncertain structures using interval analysis. Comput. Struct. 79, 421–430 (2001). https://doi.org/10.1016/S0045-7949(00)00143-7

    Article  Google Scholar 

  4. Qiu, Z., Xia, Y., Yang, J.: The static displacement and the stress analysis of structures with bounded uncertainties using the vertex solution theorem. Comput. Methods Appl. Mech. Eng. 196, 4965–4984 (2007). https://doi.org/10.1016/j.cma.2007.06.022

    Article  MATH  Google Scholar 

  5. Liu, Y., Wang, X., Wang, L.: Interval uncertainty analysis for static response of structures using radial basis functions. Appl. Math. Model. 69, 425–440 (2019). https://doi.org/10.1016/j.apm.2018.12.018

    Article  MathSciNet  MATH  Google Scholar 

  6. Chen, S.H., Lian, H.D., Yang, X.W.: Interval eigenvalue analysis for structures with interval parameters. Finite Element Anal. Des. 39, 419–431 (2003). https://doi.org/10.1016/S0168-874X(02)00082-3

    Article  Google Scholar 

  7. Williams, M.M.R.: A method for solving stochastic eigenvalue problems II. Appl. Math. Comput. 219, 4729–4744 (2013). https://doi.org/10.1016/j.amc.2012.10.089

    Article  MathSciNet  MATH  Google Scholar 

  8. Li, Q., Qiu, Z., Zhang, X.: Eigenvalue analysis of structures with interval parameters using the second-order Taylor series expansion and the DCA for QB. Appl. Math. Model. 49, 680–690 (2017). https://doi.org/10.1016/j.apm.2017.02.041

    Article  MathSciNet  MATH  Google Scholar 

  9. Gao, W.: Natural frequency and mode shape analysis of structures with uncertainty. Mech. Syst. Signal Process. 21, 24–39 (2007). https://doi.org/10.1016/j.ymssp.2006.05.007

    Article  Google Scholar 

  10. Gao, W., Zhang, N., Ji, J.: A new method for random vibration analysis of stochastic truss structures. Finite Element Anal. Des. 45, 190–199 (2009). https://doi.org/10.1016/j.finel.2008.09.004

    Article  MathSciNet  Google Scholar 

  11. Jiang, C., Bi, R.G., Lu, G.Y., Han, X.: Structural reliability analysis using non-probabilistic convex model. Comput. Methods Appl. Mech. Eng. 254, 83–98 (2013). https://doi.org/10.1016/j.cma.2012.10.020

    Article  MathSciNet  MATH  Google Scholar 

  12. Meng, Z., Hu, H., Zhou, H.: Super parametric convex model and its application for non-probabilistic reliability-based design optimization. Appl. Math. Model. 55, 354–370 (2018). https://doi.org/10.1016/j.apm.2017.11.001

    Article  MathSciNet  MATH  Google Scholar 

  13. Cheng, J., Liu, Z., Tang, M., Tan, J.: Robust optimization of uncertain structures based on normalized violation degree of interval constraint. Comput. Struct. 182, 41–54 (2017). https://doi.org/10.1016/j.compstruc.2016.10.010

    Article  Google Scholar 

  14. Wang, L., Xiong, C., Wang, X., Xu, M., Li, Y.: A dimension-wise method and its improvement for multidisciplinary interval uncertainty analysis. Appl. Math. Model. 59, 680–695 (2018). https://doi.org/10.1016/j.apm.2018.02.022

    Article  MathSciNet  MATH  Google Scholar 

  15. Astill, C.J., Imosseir, S.B., Shinozuka, M.: Impact loading on structures with random properties. J. Struct. Mech. 1, 63–77 (1972). https://doi.org/10.1080/03601217208905333

    Article  Google Scholar 

  16. Rajabalinejad, M., Meester, L.E., Van Gelder, P.H.A.J.M., Vrijling, J.K.: Dynamic bounds coupled with Monte Carlo simulations. Reliab. Eng. Syst. Saf. 96, 278–285 (2011). https://doi.org/10.1016/j.ress.2010.07.006

    Article  Google Scholar 

  17. Xia, B., Qin, Y., Yu, D., Jiang, C.: Dynamic response analysis of structure under time-variant interval process model. J. Sound Vib. 381, 121–138 (2016). https://doi.org/10.1016/j.jsv.2016.06.030

    Article  Google Scholar 

  18. Xiu, D., Em Karniadakis, G.: The Wiener-Askey polynomial chaos for stochastic differential equations. SIAM J. Sci. Comput. 24, 619–644 (2003). https://doi.org/10.1137/S1064827501387826

    Article  MathSciNet  MATH  Google Scholar 

  19. Culla, A., Carcaterra, A.: Statistical moments predictions for a moored floating body oscillating in random waves. J. Sound Vib. 308, 44–66 (2007). https://doi.org/10.1016/j.jsv.2007.07.018

    Article  Google Scholar 

  20. Kamínski, M.M.: A generalized stochastic perturbation technique for plasticity problems. Comput. Mech. 45, 349–361 (2010). https://doi.org/10.1007/s00466-009-0455-7

    Article  MathSciNet  MATH  Google Scholar 

  21. Xu, Y., Qian, Y., Chen, J., Song, G.: Stochastic dynamic characteristics of FGM beams with random material properties. Compos. Struct. 133, 585–594 (2015). https://doi.org/10.1016/j.compstruct.2015.07.057

    Article  Google Scholar 

  22. Kundu, A., Adhikari, S.: Dynamic analysis of stochastic structural systems using frequency adaptive spectral functions. Probabilistic Eng. Mech. 39, 23–38 (2015). https://doi.org/10.1016/j.probengmech.2014.11.003

    Article  Google Scholar 

  23. Sandu, A., Sandu, C., Ahmadian, M.: Modeling multibody systems with uncertainties. Part I: theoretical and computational aspects. Multibody Syst. Dyn. 15, 369–391 (2006). https://doi.org/10.1007/s11044-006-9007-5

    Article  MATH  Google Scholar 

  24. Sandu, C., Sandu, A., Ahmadian, M.: Modeling multibody systems with uncertainties. Part II: numerical applications. Multibody Syst. Dyn. 15, 241–262 (2006). https://doi.org/10.1007/s11044-006-9008-4

    Article  MATH  Google Scholar 

  25. Pishvaee, M.S., Fazli Khalaf, M.: Novel robust fuzzy mathematical programming methods. Appl. Math. Model. 40, 407–418 (2016). https://doi.org/10.1016/j.apm.2015.04.054

    Article  MathSciNet  MATH  Google Scholar 

  26. Certa, A., Hopps, F., Inghilleri, R., La Fata, C.M.: A Dempster-Shafer theory-based approach to the failure mode, effects and criticality analysis (FMECA) under epistemic uncertainty: application to the propulsion system of a fishing vessel. Reliab. Eng. Syst. Saf. 159, 69–79 (2017). https://doi.org/10.1016/j.ress.2016.10.018

    Article  Google Scholar 

  27. Alefeld, G., Mayer, G.: Interval analysis: theory and applications. J. Comput. Appl. Math. 121, 421–464 (2000). https://doi.org/10.1016/S0377-0427(00)00342-3

    Article  MathSciNet  MATH  Google Scholar 

  28. Nedialkov, N.S., Jackson, K.R., Corliss, G.F.: Validated solutions of initial value problems for ordinary differential equations. Appl. Math. Comput. 105, 21–68 (1999). https://doi.org/10.1016/S0096-3003(98)10083-8

    Article  MathSciNet  MATH  Google Scholar 

  29. Moens, D., Vandepitte, D.: A survey of non-probabilistic uncertainty treatment in finite element analysis. Comput. Methods Appl. Mech. Eng. 194, 1527–1555 (2005). https://doi.org/10.1016/j.cma.2004.03.019

    Article  MATH  Google Scholar 

  30. Berz, M., Makino, K.: Suppression of the wrapping effect by Taylor model—based verified integrators: long-term stabilization by shrink wrapping. Int. J. Differ. Equ. Appl. 10, 385–403 (2005)

    MathSciNet  MATH  Google Scholar 

  31. Wu, J., Luo, Z., Zhang, Y., Zhang, N., Chen, L.: Interval uncertain method for multibody mechanical systems using Chebyshev inclusion functions. Int. J. Numer. Methods Eng. 95, 608–630 (2013). https://doi.org/10.1002/nme.4525

    Article  MathSciNet  MATH  Google Scholar 

  32. Makino, K., Berz, M.: Efficient control of the dependency problem based on Taylor model methods. Reliab. Comput. 5, 3–12 (1999). https://doi.org/10.1023/A:1026485406803

    Article  MathSciNet  MATH  Google Scholar 

  33. Revol, N., Makino, K., Berz, M.: Taylor models and floating-point arithmetic: proof that arithmetic operations are validated in COSY. J. Log. Algebr. Program. 64, 135–154 (2005). https://doi.org/10.1016/j.jlap.2004.07.008

    Article  MathSciNet  MATH  Google Scholar 

  34. Jackson, K.R., Nedialkov, N.S.: Some recent advances in validated methods for IVPs for ODEs. Appl. Numer. Math. 42(1), 269–284 (2002)

    MathSciNet  MATH  Google Scholar 

  35. Qiu, Z., Wang, X.: Comparison of dynamic response of structures with uncertain-but-bounded parameters using non-probabilistic interval analysis method and probabilistic approach. Int. J. Solids Struct. 40, 5423–5439 (2003). https://doi.org/10.1016/S0020-7683(03)00282-8

    Article  MATH  Google Scholar 

  36. Qiu, Z., Wang, X.: Parameter perturbation method for dynamic responses of structures with uncertain-but-bounded parameters based on interval analysis. Int. J. Solids Struct. 42, 4958–4970 (2005). https://doi.org/10.1016/j.ijsolstr.2005.02.023

    Article  MATH  Google Scholar 

  37. Qiu, Z., Ma, L., Wang, X.: Non-probabilistic interval analysis method for dynamic response analysis of nonlinear systems with uncertainty. J. Sound Vib. 319, 531–540 (2009). https://doi.org/10.1016/j.jsv.2008.06.006

    Article  Google Scholar 

  38. Zhang, X.M., Ding, H., Chen, S.H.: Interval finite element method for dynamic response of closed-loop system with uncertain parameters. Int. J. Numer. Methods Eng. 70, 543–562 (2007). https://doi.org/10.1002/nme.1891

    Article  MATH  Google Scholar 

  39. Xia, B., Yu, D.: Interval analysis of acoustic field with uncertain-but-bounded parameters. Comput. Struct. 112–113, 235–244 (2012). https://doi.org/10.1016/j.compstruc.2012.08.010

    Article  Google Scholar 

  40. Han, X., Jiang, C., Gong, S., Huang, Y.H.: Transient waves in composite-laminated plates with uncertain load and material property. Int. J. Numer. Methods Eng. 75, 253–274 (2008). https://doi.org/10.1002/nme.2248

    Article  MATH  Google Scholar 

  41. Qiu, Z., Elishakoff, I.: Antioptimization of structures with large uncertain-but-non-random parameters via interval analysis. Comput. Methods Appl. Mech. Eng. 152, 361–372 (1998). https://doi.org/10.1016/S0045-7825(96)01211-X

    Article  MATH  Google Scholar 

  42. Xia, B., Yu, D.: Modified sub-interval perturbation finite element method for 2D acoustic field prediction with large uncertain-but-bounded parameters. J. Sound Vib. 331, 3774–3790 (2012). https://doi.org/10.1016/j.jsv.2012.03.024

    Article  Google Scholar 

  43. Wu, J., Zhang, Y., Chen, L., Luo, Z.: A Chebyshev interval method for nonlinear dynamic systems under uncertainty. Appl. Math. Model. 37, 4578–4591 (2013). https://doi.org/10.1016/j.apm.2012.09.073

    Article  MathSciNet  MATH  Google Scholar 

  44. Lin, Y., Stadtherr, M.A.: Validated solutions of initial value problems for parametric ODEs. Appl. Numer. Math. 57, 1145–1162 (2007). https://doi.org/10.1016/j.apnum.2006.10.006

    Article  MathSciNet  MATH  Google Scholar 

  45. Xia, Y., Qiu, Z., Friswell, M.I.: The time response of structures with bounded parameters and interval initial conditions. J. Sound Vib. 329, 353–365 (2010). https://doi.org/10.1016/j.jsv.2009.09.019

    Article  Google Scholar 

  46. Liu, N., Gao, W., Song, C., Zhang, N., Pi, Y.L.: Interval dynamic response analysis of vehicle-bridge interaction system with uncertainty. J. Sound Vib. 332, 3218–3231 (2013). https://doi.org/10.1016/j.jsv.2013.01.025

    Article  Google Scholar 

  47. Wang, L., Liu, Y., Liu, Y.: An inverse method for distributed dynamic load identification of structures with interval uncertainties. Adv. Eng. Softw. 131, 77–89 (2019). https://doi.org/10.1016/j.advengsoft.2019.02.003

    Article  Google Scholar 

  48. Wang, L., Liu, Y., Gu, K., Wu, T.: A radial basis function artificial neural network (RBF ANN) based method for uncertain distributed force reconstruction considering signal noises and material dispersion. Comput. Methods Appl. Mech. Eng. 364, 112954 (2020). https://doi.org/10.1016/j.cma.2020.112954

    Article  MathSciNet  MATH  Google Scholar 

  49. Muscolino, G., Sofi, A.: Stochastic analysis of structures with uncertain-but-bounded parameters via improved interval analysis. Probabilistic Eng. Mech. 28, 152–163 (2012). https://doi.org/10.1016/j.probengmech.2011.08.011

    Article  Google Scholar 

  50. Ran, X., Leng, S., Liu, K.: A novel affine arithmetic method with missed the triangular domain with uncertainties. IEEE Trans. Smart Grid. (2019). https://doi.org/10.1109/tsg.2019.2938080

    Article  Google Scholar 

  51. Adusumilli, B.S., Kumar, B.K.: Modified affine arithmetic based continuation power flow analysis for voltage stability assessment under uncertainty. IET Gener. Transm. Distrib. 12, 4225–4232 (2018). https://doi.org/10.1049/iet-gtd.2018.5479

    Article  Google Scholar 

  52. Buras, A.J., Jamin, M., Lautenbacher, M.E.: A 1996 analysis of the CP violating ratio ε′/ε. Phys. Lett. B 389, 749–756 (1996). https://doi.org/10.1016/s0370-2693(96)80019-0

    Article  Google Scholar 

  53. Wiener, N.: The homogeneous chaos. Am. J. Math. 60, 897–936 (1938). https://doi.org/10.2307/2371268

    Article  MathSciNet  MATH  Google Scholar 

  54. Wiener, N.: Nonlinear Problems in Random Theory. MIT Technology Press and Wiley, New York (1958)

    MATH  Google Scholar 

  55. Cameron, R.H., Martin, W.T.: The orthogonal development of non-linear functionals in series of Fourier-Hermite functionals. Ann. Math. 48, 385–392 (1947). https://doi.org/10.2307/1969178

    Article  MathSciNet  MATH  Google Scholar 

  56. Xu, M., Du, J., Wang, C., Li, Y.: Hybrid uncertainty propagation in structural-acoustic systems based on the polynomial chaos expansion and dimension-wise analysis. Comput. Methods Appl. Mech. Eng. 320, 198–217 (2017). https://doi.org/10.1016/j.cma.2017.03.026

    Article  MathSciNet  MATH  Google Scholar 

  57. Gerstner, T., Griebel, M.: Numerical integration using sparse grids. Numer. Algorithms. 18, 209–232 (1998). https://doi.org/10.1023/A:1019129717644

    Article  MathSciNet  MATH  Google Scholar 

  58. Hosder, S., Walters, R.W., Balch, M.: Efficient sampling for non-intrusive polynomial chaos applications with multiple uncertain input variables. In: Collection of Technical Papers—AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference. pp. 2946–2961 (2007)

  59. Isukapalli, S.S., Roy, A., Georgopoulos, P.G.: Stochastic response surface methods (SRSMs) for uncertainty propagation: application to environmental and biological systems. Risk Anal. 18, 351–363 (1998). https://doi.org/10.1111/j.1539-6924.1998.tb01301.x

    Article  Google Scholar 

  60. Park, J.: Optimal Latin-hypercube designs for computer experiments. J. Stat. Plan. Inference. 39, 95–111 (1994)

    MathSciNet  MATH  Google Scholar 

  61. Morris, M.D., Mitchell, T.J.: Exploratory designs for computational experiments. J. Stat. Plan. Inference. 43, 381–402 (1995). https://doi.org/10.1016/0378-3758(94)00035-T

    Article  MATH  Google Scholar 

  62. Gao, W., Zhang, N., Ji, J.C., Du, H.P.: Dynamic analysis of vehicles with uncertainty. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 222, 657–664 (2008). https://doi.org/10.1243/09544070JAUTO740

    Article  Google Scholar 

  63. Han, Z.: Exterior ballistics of projectiles and rockets. Beijing Institute of Technology Press, Beijing (2014)

    Google Scholar 

  64. Moens, D., Vandepitte, D.: Recent advances in non-probabilistic approaches for non-deterministic dynamic finite element analysis. Arch. Comput. Methods Eng. 13, 389–464 (2006). https://doi.org/10.1007/BF02736398

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This research was financially supported by the National Natural Science Foundation of China (Grant Nos. 301070603 and 11572158). Besides, the authors wish to express their many thanks to the reviewers for their useful and constructive comments.

Author information

Authors and Affiliations

  1. School of Mechanical Engineering, Nanjing University of Science and Technology, Nanjing, 210094, People’s Republic of China

    Liqun Wang & Guolai Yang

  2. Department of Mechanical Engineering, University of Alberta, Edmonton, T6G 1H9, Canada

    Zengtao Chen

Authors
  1. Liqun Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zengtao Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Guolai Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Guolai Yang.

Ethics declarations

Conflict of interest

The authors declare that they have no potential conflict of interest to this work.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, L., Chen, Z. & Yang, G. A polynomial chaos expansion approach for nonlinear dynamic systems with interval uncertainty. Nonlinear Dyn 101, 2489–2508 (2020). https://doi.org/10.1007/s11071-020-05895-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-020-05895-x

Keywords

Search

Navigation